Category: Inter 1st Year

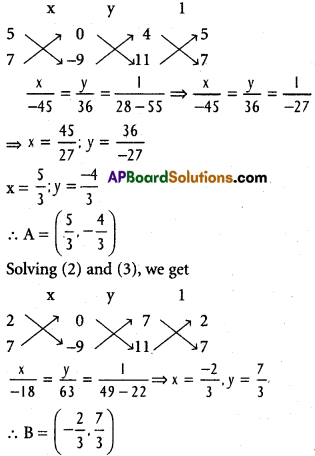
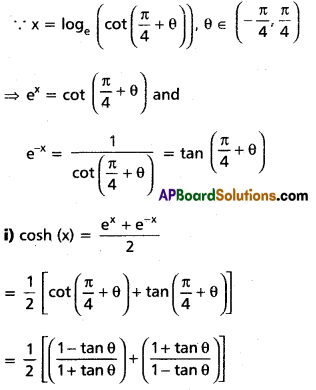
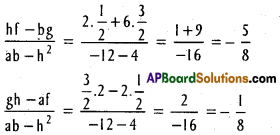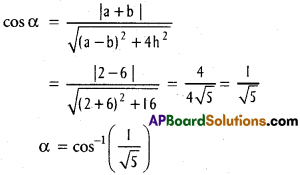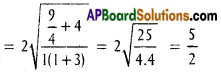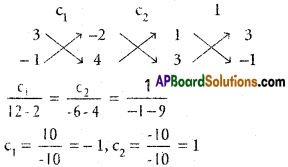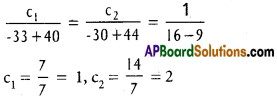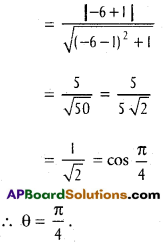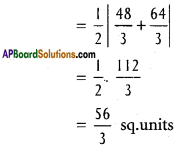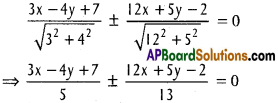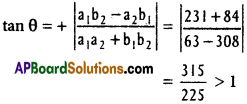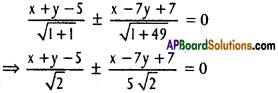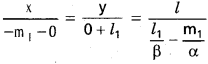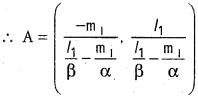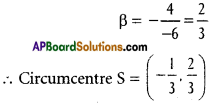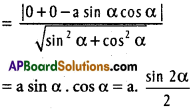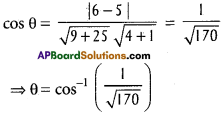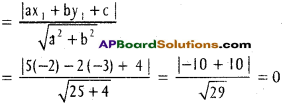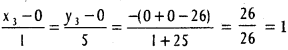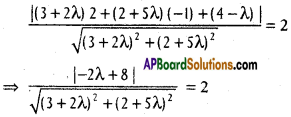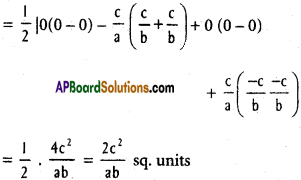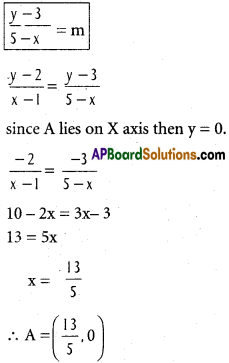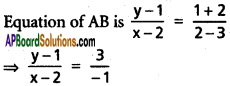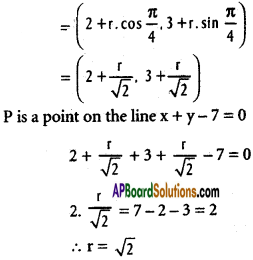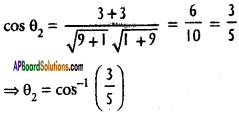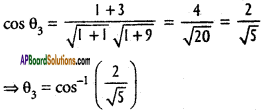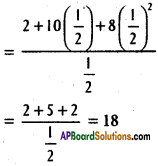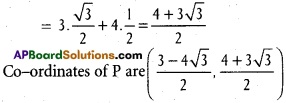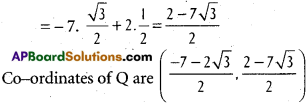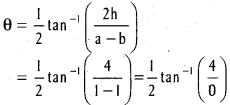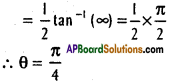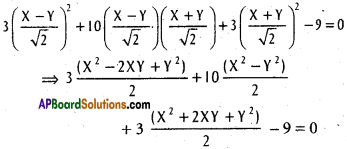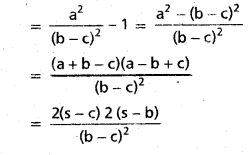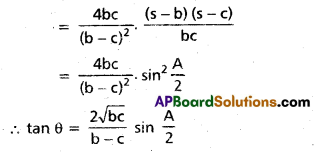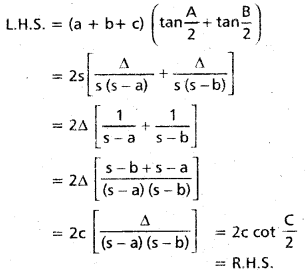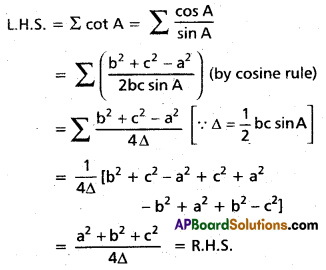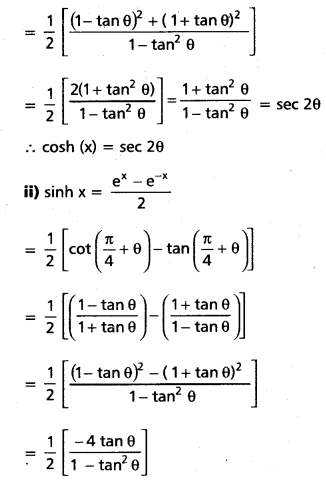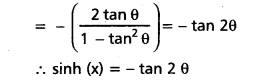Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Pair of Straight Lines Solutions Exercise 4(c) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Pair of Straight Lines Solutions Exercise 4(c)

I.

Question 1.
Find the equation of the lines joining the origin to the points of intersection of x² + y² = 1 and x + y = 1.
Solution:
The given curves are
x² + y² = 1 …….. (1)
x + y = 1 ……….. (2)
Homogenising (1) with the help of (2) Combined equation of OA and OB is

x² + y² = (x + y)²
= x² + y² + 2xy
i.e., 2xy = 0 ⇒ xy – 0

Question 2.
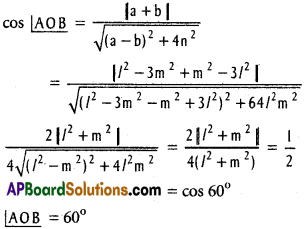
Find the angle between the lines joining the origin to the points of intersection of y2 = x and x + y = 1.
Solution:
Equation of the centre is y² = x …………. (1)
Equation of AB is x + y = 1 ………. (2)
Hamogonsing (1) with the help of O
Combined equation of A & OB is
y² = x(x + y) = x² + xy
x² + xy – y2 = 0
a + b = 1 – 1 = 0
OA, OB an perpendicular
∴ ∠AOB =90°

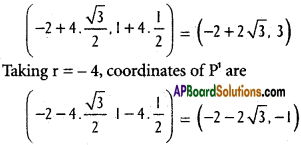
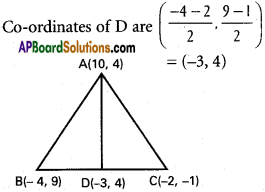
II.

Question 1.
Show that the lines joining the origin to the points of intersection of the curve x² – xy + y² + 3x + 3y – 2 = 0 and the straight line x – y – √2 = 0 are mutually perpendicular.
Solution:

Equation of the curve is
x² – xy + y² + 3x + 3y – 2 = 0 ……….. (1)
Equation of AB is x – y – √2 = 0
x – y = √2
\(\frac{x-y}{\sqrt{2}}\) = 1 ………. (2)
Homogenising, (1) with the help of (2) combined equation of OA, OB is
x² – xy + y² + 3x.1 + 3y.1 – 2.1² = 0
x² – xy + y² + 3(x + y)\(\frac{x-y}{\sqrt{2}}\) – 2\(\frac{(x-y)^{2}}{2}\) = 0
x² – xy + y² + \(\frac{3}{\sqrt{2}}\)(x² – y²) – (x² – 2xy + y²) = 0
x² – xy + y² + \(\frac{3}{\sqrt{2}}\)x² – \(\frac{3}{\sqrt{2}}\)y² – x² + 2xy – y² = 0
\(\frac{3}{\sqrt{2}}\)x² + xy – \(\frac{3}{\sqrt{2}}\)y² = 0
a + b = \(\frac{3}{\sqrt{2}}\) – \(\frac{3}{\sqrt{2}}\) = 0
∴ OA, OB are perpendicular.

Question 2.
Find the values of k, if the lines joining the origin to the points of intersection of the curve 2x² – 2xy + 3y² + 2x – y – 1 = 0 and the line x + 2y = k are muiually perpendicular.
Solution:
Given equation of the curve is
S ≡ 2x² – 2xy + 3y² + 2x – y – 1 = 0 ……….. (1)
Equation of AB is x + 2y = k

\(\frac{x+2y}{k}\) = 1
Homogenising, (1) with the help of (2), combined equation of OA, OB is
2x² – 2xy + 3y² + 2x.1 – y.1 – 1² = 0
2x² – 2xy + 3y² + 2x\(\frac{x+2y}{k}\) – y\(\frac{x+2y}{k}\) – \(\frac{(x+2y)^{2}}{k^{2}}\) = 0
Multiplying with k²
2k²x² – 2k²xy + 3k²y² + 2kx(x + 2y) – ky(x + 2y) – (x + 2y)² = 0
2k²x² – 2k²xy + 3k²y² + 2kx² + 4kxy – kxy – 2ky² – x² – 4xy – 4y² = 0
(2k² + 2k – 1)x² + (-2k² + 3k – 4) xy + (3k² – 2k – 4) y² = 0
Since OA, OB are perpendicular.
Co-efficient x² + Co-efficient of y² = 0
2k² + 2k – 1 + 3k² – 2k – 4 = 0
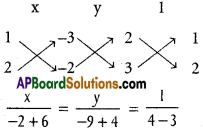
5k² = 5 ⇒ k² = 1
∴ k = ± 1

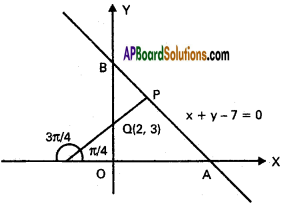
Question 3.
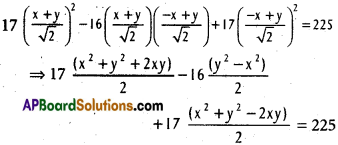
Find the angle between the lines joining the origin to the points of intersection of the curve x² + 2xy + y² + 2x + 2y – 5 = 0 and the line 3x – y + 1 = 0
Solution:

Equation of the curve is
x² + 2xy + y² + 2x + 2y- 5 = 0 …….. (1)
Equation of AB is 3x – y + 1 = 0
y – 3x = 1…………. (2)
Homogenising (1) with the help of (2), combined equation of OA, OB is
x² + 2xy + y² + 2x.1 + 2y.1 – 5.1² = 0
x² + 2xy + y² + 2x(y – 3x) + 2y(y – 3x) – 5 (y – 3x)² = 0
x² + 2xy + y² + 2xy – 6x² + 2y² – 6xy – 5(y² + 9x² – 6xy) = 0
– 5x² – 2xy + 3y² – 5y² – 45x² + 30 xy = 0
-50x² + 28xy – 2y² = 0
i.e, 25x² – 14xy + y² = 0
Suppose θ is the angle between OA and OB

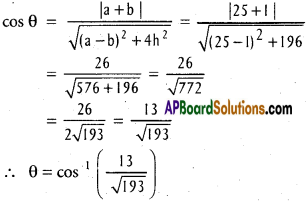
III.

Question 1.
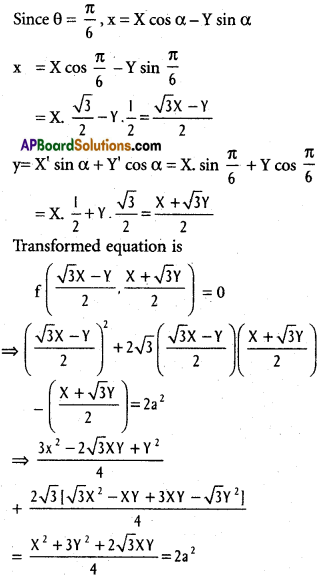
Find the condition for the chord lx + my = 1 of the circle x² + y² = a² (whose centre is the origin) to subtend a right angle at the origin.
Solution:

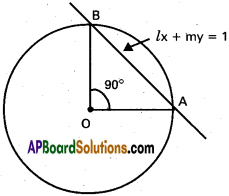
Equation of the circle is x² + y² = a² ………. (1)
Equation of AB is lx + my = 1 ……….. (2)
Homogenising (1) with the help of (2)
Gombined equation of OA, OB is
x² + y² = a².l²
x² + y² = a² (lx + my)²
= a²(l²x² + m²y² + 2lmxy)
= a²l²x² + a²m²y² + 2a²lmxy
i.e., a²l²x² + 2a²lmxy + a²m²y² – x² – y² = 0
(a²l² – 1) x² + 2a² lmxy + (a²m² – 1)y² = 0
Since OA, OB are perpendicular
Co-efficient of x² + co-efficient of y² = 0
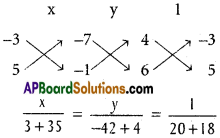
a²l² – 1 + a²m² – 1 = 0
a²(l² + m²) = 2
This is the required condition.

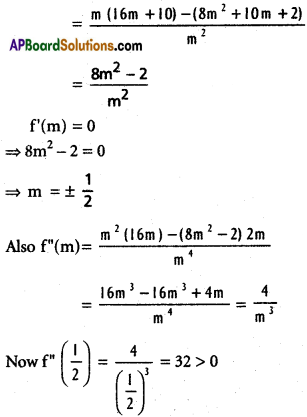
Question 2.
Find the condition for the lines joining the origin to the points of intersection of the circle x² + y² = a² and the line lx + my = 1 to coincide.
Solution:

Equation of the circle is
x² + y² = a² ………. (1)
Equation of AB is lx + my = 1 ……….. (2)
Homogenising (1) with the help of (2)
Combined equation of OA, OB is
x² + y² = a².l²
= a²(lx + my)²
= a²(l²x² + m²y² + 2lmxy)
i.e., x² + y² = a²l²x² + a²m²y² + 2a²lmxy
(a²l² – 1)x² + 2a²lmxy + (a²m² – 1)y² = 0
Since OA, OB are coincide
⇒ h² = ab
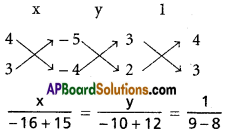
a⁴ l²m² = (a²l² – 1)(a²m² – 1)
a⁴ l²m² = a⁴ l²m² – a²l² – a² m² + 1
∴ a²l² – a²m² +1=0
a² (l² + m²) = 1
This is the required condition.

Question 3.
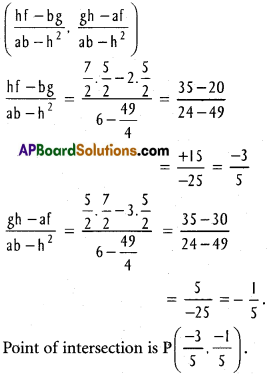
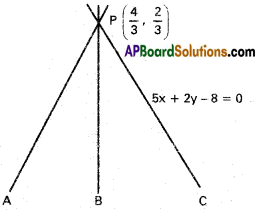
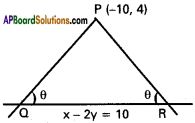
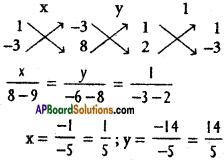
Write down the equation of the pair of straight lines joining the origin to the points of intersection of the line 6x – y + 8 = 0 with the pair of straight lines 3x² + 4xy – 4y² – 11x + 2y + 6 = 0. Show that the lines so obtained make equal angles with the coordinate axes.
Solution:
Given pair of lines is
3x² + 4xy – 4y² – 11x + 2y + 6 = 0 ……….. (1)
Given line is
6x – y + 8 = 0 ⇒ \(\frac{6x-y}{-8}\) = 1
⇒ \(\frac{y-6x}{8}\) = 1
Homogenising (1) w. r. t. (2)
3x² + 4xy – 4y² – (11x – 2y) (\(\frac{y-6x}{8}\)) = 6(\(\frac{y-6x}{8}\))² = 0
64[3x² + 4xy – 4y²] – 8[11xy – 66x² – 2y² + 12xy] + 6[y² + 36x² – 12xy] = 0
936x² + 256 xy – 256 xy – 234y² = 0
∴ 468x² – 117y² = 0 …….. (3)
⇒ 4x² – y² = 0
is eq. of pair of lines joining the origin to the point of intersection in.
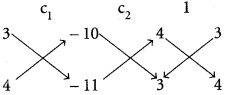
The eq. pair of angle bisectors to (3) is
h(x² – y²) – (a – b)xy = 0
0(x² – y²) – f4 – l)xy = 0
⇒ xy = 0
x = 0 or y = 0 [Eqs. is of coordinate axes]
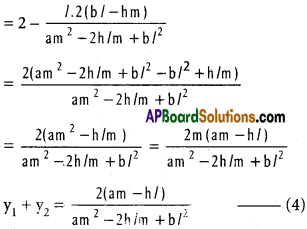
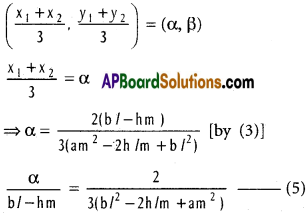
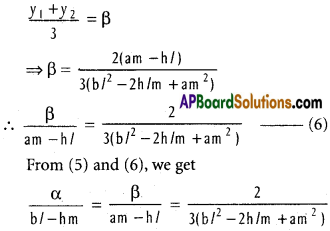
∴ The pair of lines are equally inclined to the co-ordinate axes.

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Pair of Straight Lines Solutions Exercise 4(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Pair of Straight Lines Solutions Exercise 4(b)

I.

Question 1.
Find the angle between the lines represented by 2x² + xy – 6y² + 7y – 2 = 0.
Solution:
Comparing with
ax² + 2hxy + by² + 2gx + 2fy + c = 0
a = 2, 2g = 0, g = 0
b = – 6, 2f = 7, f = 7/2
c = – 2, 2h = 1, h = 1/2
Angle between the lines is given by

Question 2.
Prove that the equation 2x² + 3xy – 2y² + 3x + y + 1 = 0 represents a pair of perpendicular lines.
Solution:
Given a = 2, b = -2
a + b = 0 ⇒ cos α = 0 ⇒ α = π/2
∴ The given lines are perpendicular.

II.

Question 1.
Prove that the equation 3x² + 7xy + 2y² + 5x + 5y + 2 = 0 represents a pair of straight lines and find the co-ordinates of the’point of intersection.
Solution:
The given equation is
3x² + 7xy + 2y² + 5x + 5y + 2 = 0
Comparing a = 3 2f = 5 ⇒ f = \(\frac{5}{2}\)
b = 2 2g = 5 ⇒ g = \(\frac{5}{2}\)
c = 2 2h = 7 ⇒ h = \(\frac{7}{2}\)
∆ = abc + 2fgh – af² – bg² – ch²
= 3(2)(2) + 2.\(\frac{5}{2}\).\(\frac{5}{2}\).\(\frac{7}{2}\) – 3.\(\frac{25}{4}\) – 2.\(\frac{25}{4}\) – 2.\(\frac{49}{4}\) = 0
= \(\frac{1}{4}\)(48 + 175 – 75 – 50 – 98)
= \(\frac{1}{2}\)(223 – 223) = 0

∴ The given equation represents a pair of lines point of intersection is

Question 2.
Find the value of k, if the equation 2x² + kxy – 6y² + 3x + y + l = 0 represents a pair of straight lines. Find the point of intersection of the lines and the angle between the straight lines for this value of k.
Solution:
The given equation is
2x² + kxy – by² + 3x + y+ 1 = 0
a = 2 2f = 1 ⇒ f = \(\frac{1}{2}\)
b = -6 2g = 3 ⇒ g = \(\frac{3}{2}\)
c = 1 2h = k ⇒ h = \(\frac{k}{2}\)
The given equation represents a pair of straight lines abc + 2fgh – af² – bg² – ch² = 0
-12 + 2.\(\frac{1}{2}\).\(\frac{3}{2}\)(+\(\frac{k}{2}\) -2.\(\frac{1}{4}\) + 6.\(\frac{9}{4}\) – \(\frac{k^{2}}{4}\) = 0
– 48 + 3k – 2 + 54 – k² = 0
-k² + 3k + 4 = 0 ⇒ k² – 3k – 4 = 0
(k – 4) (k + 1) = 0
k = 4 or – 1.

Case (i): k = – 1
Point of’intersection is \(\left(\frac{h f-b g}{a b-h^{2}}, \frac{g h-a f}{a b-h^{2}}\right)\)

Point of intersection is (\(\frac{-5}{7}\), \(\frac{1}{7}\))
Angle between the lines

Case (ii): k = 4

Point of intersection is P(-\(\frac{5}{8}\), –\(\frac{1}{8}\))

Question 3.
Show that the equation x² – y² – x + 3y – 2 = 0 represents a pair of perpendicular lines and find their equations.
Solution:
Comparing a= 1, f = \(\frac{3}{2}\)
b = -1, g = –\(\frac{1}{2}\)
c = -2, h = 0
abc + 2fgh – af² – bg² – ch²
= 1 (-1) (-2) + 0- 1.\(\frac{9}{4}\) + 1.\(\frac{1}{4}\) + 0
= + 2 – \(\frac{9}{4}\) + \(\frac{1}{4}\) = 0
h² – ab = 0 – 1(-1) = 1 > 0,
f² – be = \(\frac{9}{4}\) – 2 = \(\frac{1}{4}\) = 1 > 0
g² – ac = \(\frac{1}{4}\) + 2 = \(\frac{9}{4}\) > 0
a + b = 1 – 1 = 0
The given equation represent a pair of per-pendicular lines
Let x² – y² – x + 3y – 2
= (x + y + c₁) (x – y + c₂)
Equating the co-efficients of x
⇒ c₁ + c₂ = – 1
Equating the co-efficients of y
⇒ – c₁ + c₂ = 3
Adding 2c₂ = 2 ⇒ c₂ = 1
c₁ + c₂ = – 1 ⇒ c₁ + 1 = – 1
c₁ = – 2
Equations of the lines are x + y – 2 = 0 and x – y + 1 = 0

Question 4.
Show that the lines x² + 2xy – 35y² – 4x + 44y – 12 = 0 are 5x + 2y – 8 = 0 are concurrent.
Solution:
Equations of the given lines are
x² + 2xy- 35y² -4x + 44y- 12 = 0
a = 1, f = 22
b = – 35, g = – 2
c = – 12, h = 1
Point or intersection is \(\left(\frac{h f-b g}{a b-h^{2}}, \frac{g h-a f}{a b-h^{2}}\right)\)

Point of intersection of the given lines is P(\(\frac{4}{3}\), \(\frac{2}{3}\))
5x + 2y – 8 = 5.\(\frac{4}{3}\) + 2.\(\frac{2}{3}\) – 8
= \(\frac{20+4-24}{3}\) = 0
P lies on the third line 5x + 2y – 8 = 0
∴ The given lines are concurrent.

Question 5.
Find the distances between the following pairs of parallel straight lines :
i) 9x² – 6xy + y² + 18x – 6y + 8 = 0
Solution:
Distance between parallel lines = \(2 \sqrt{\frac{g^{2}-a c}{a(a+b)}}\)

ii) x² + 2√3xy + 3y² – 3x – 3√3y – 4 = 0
Solution:
Distance between parallel lines = \(2 \sqrt{\frac{g^{2}-a c}{a(a+b)}}\)

Question 5.
Show that the two pairs of lines 3x² + 8xy – 3y² = 0 and 3x² + 8xy – 3y² + 2x – 4y – 1 = 0 form a square.

Solution:
Combined equation of CA and CB is
3x² + 8xy – 3y²
(x + 3y) (3x – y) = 0
3x – y = 0, x + 3y = 0
Equation of OA is 3x – y = 0 ………. (1)
Equation of OB is x + 3y = 0 ……….(2)
Combined equation of CA and CB is
3x² + 8xy – 3y² + 2x – 4y + 1 =0
Let 3x² + 8xy – 3y² + 2x – 4y + 1 = (3x – y + c₁) (x + 3y + c₂)
Equating the co-efficients of
x, we get c₁ + 3c₂ = 2
y, we have 3c₁ + c₂ = -4

Equation of BC is 3x – y – 1 = 0 ………. (3)
Equation of AC is x + 3y + 1 = 0 ………. (4)
OA and BC differ by a constant ⇒ OA parallel to BC
OB and CA differ by a constant ⇒ OB parallel to AC
From combined equation of OA and OB
OACB is a rectangle a + b = 3 – 3 = 0
OA = Length of the -L lar from O to AC = \(\frac{|0+0+1|}{\sqrt{1+9}}=\frac{1}{\sqrt{10}}\)
OB = Length of the perpendicular from O to BC = \(\frac{|0+0-1|}{\sqrt{9+1}}=\frac{1}{\sqrt{10}}\)
OA = OB and OACB is a rectangle
OACB is a square.

III.

Question 1.
Find the product of the lengths of the perpendiculars drawn from (2, 1) upon the lines
12x² + 25xy + 12y² + 10x + 11y + 2 = 0

Solution:
Combined equation of AB, AC is
12x² + 25xy+ 12y² + 10x + 11y + 2 = 0
12x² + 25xy +12y²
= 12x² + 16xy + 9xy + 12y² = 0
= 4x (3x + 4y) + 3y (3x + 4y)
= (3x + 4y) (4x + 3y)
Let 12x² + 25xy + 12y² + 10x + 11y + 2
= (3x + 4y + c₁) (4x + 3y + c₂)
Equating the co-efficients of x, we get
4c₁ + 3c₂ = 10 ………. (1)
Equating the co-efficients of y, we get
3c₁ + 4c₂ = 11 ………… (2)
i.e., 4c₁ + 3c₂ – 10 = 0
3c₁ + 4c₂ – 11 = 0


Equation of AB is 3x + 4y + 1 = 0
Equation of AC is 4x + 3y + 2 = 0
PQ = Length of the perpendicular from P(2, 1) on
AB = \(\frac{6+4+1}{\sqrt{9+16}}=\frac{11}{5}\)
PQ = Length of the perpendiculars from P(2, 1) on
AC = \(\frac{|8+3+2|}{\sqrt{16+9}}=\frac{13}{5}\)
Product of the length of the perpendiculars
= PQ × PR= \(\frac{11}{5}\) × \(\frac{13}{5}\) = \(\frac{143}{25}\)

Question 2.
Show that the straight lines y² – 4y + 3 = 0 and
x² + 4xy + 4y² + 5x + 10y + 4 = 0 form a parallelogram and find the lengths of its sides.

Solution:
Equation of the first pair of lines is
y² – 4y + 3 = 0
(y – 1) (y – 3) = 0
y – 1 = 0 or y – 3 = 0
Equation of AB is y – 1 = 0 ……….. (1)
Equation of CD is y – 3 = 0 ………. (2)
Equations of AB and CD differ by a constant.
∴ AB and CD are parallel.
Equation of the second pair of lines is
x² + 4xy + 4y² + 5x + 10y + 4 = 0
(x + 2y)² + 5(x + 2y) + 4 = 0
(x + 2y)² + 4 (x + 2y) + (x + 2y) + 4 = 0
(x + 2y)(x + 2y + 4) + 1(x + 2y + 4) = 0
(x + 2y + 1) (x + 2y + 4) = 0
x + 2y + 1 = 0, x + 2y + 4 = 0
Equation of AD is x + 2y + 1 = 0 ……… (3)
Equation of BC is x + 2y + 4 = 0 ……… (4)
AD and BC are parallel.
Solving (1), (3) x + 2 + 1 = 0
x = – 3
Co-ordinates of A are (-3, 1)
Solving (2), (3) x + 6 + 1 = 0
x = -7
Co-ordinates of DC are (-7, 3)
Solving (1), (4) x + 2 + 4 = 0
x = – 6
Co-ordinates of B are (-6, 1)

Lengths of the sides of the parallelogram are 3, 2√5.

Question 3.
Show that the product of the perpendicular distances from the origin to the pair of straight lines represented by ax² + 2hxy + by² + 2gx + 2fy + c = 0 is \(\frac{|c|}{\sqrt{(a-b)^{2}+4 h^{2}}}\)
Solution:
Let ax² + 2hxy + by² + 2gx + 2fy + c = 0 represents the lines
l₁x + m₁y + n₁ = 0 ……….. (1)
l₂x + m₂y + n₂ = 0 ………. (2)
⇒ ax² + 2hxy + by² + 2gx + 2fy + c ≡ (l₁x + m₁y + n₁) (l₂x + m₂y + n₂)
l₁l₂ = a, m₁m₂ = b, l₁m₂ + l₂m₁ = 2h,
l₁n₂ + l₂n₁ = 2g, m₁n₂ + m₂n₁ = 2f, n₁n₂ = c
⊥^Ir distance from origin to (1) is = \(\frac{\left|n_{1}\right|}{\sqrt{l_{1}^{2}+m_{1}^{2}}}\)
⊥^Irdistance from origin to (2) is = \(\frac{\left|n_{2}\right|}{\sqrt{I_{2}^{2}+m_{2}^{2}}}\)
Product of perpendiculars

Question 4.
If the equation ax² + 2hxy + by² + 2gx + 2fy + c = 0 represents a pair of intersecting lines, then show that the square of the distance of their point of intersection from the origin is \(\frac{c(a+b)-f^{2}-g^{2}}{a b-h^{2}}\). Also slow that the square of this distance is \(\frac{f^{2}+g^{2}}{h^{2}+b^{2}}\) if the given lines are perpendicular.
Solution:
Let the equation
ax² + 2hxy + by² + 2gx + 2fy + c = 0
represent the lines
l₁x + m₁y + n₁ = 0 …….. (1)
l₂x + m₂y + n₂ = 0 …….. (2)
(l₁x + m₁y + n₁)(l₂x + m₂y + n₂) = ax² + 2hxy + by² + 2gx + 2fy + c
l₁l₂ = a, m₁ m₂ = b, n₁n₂ = c
l₁m₂ + l₂m₁ = 2h, l₁n₂ + l₁₂n₁ = 2g, m₁n₂ + m₂n₁ = 2f
Solving (1) and (2)
\(\frac{x}{m_{1} n_{2}-m_{2} n_{1}}=\frac{y}{I_{2} n_{1}-l_{1} n_{2}}=\frac{1}{l_{1} m_{2}-I_{2} m_{1}}\)
The point of intersection, P


If the given pair of lines are perpendicular, then a + b = 0
∴ a = -b
\(\mathrm{OP}^{2}=\frac{0-r^{2}-g^{2}}{(-b) b-h^{2}}=\frac{r^{2}+g^{2}}{h^{2}+b^{2}}\)

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Pair of Straight Lines Solutions Exercise 4(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Pair of Straight Lines Solutions Exercise 4(a)

I.

Question 1.
Find the acute angle between the pair of lines represented by the following equations.
(i) x² – 7xy + 12y² = 0
(ii) y² – xy – 6x² = 0
(iii) (x cos α – y sin α)² = (x² + y²) sin² α
(iv) x² + 2xy cot α – y² = 0
Solution:
(i) x² – 7xy + 12y² = 0
a = 1, b = 12, h = –\(\frac{7}{2}\)

(ii) y² – xy – x² = 0
a = -6, b = 1, h = –\(\frac{1}{2}\)
\(\cos \theta=\frac{|a+b|}{\sqrt{(a-b)^{2}+4 h^{2}}}\)

(iii) (x cos α – y sin α)² = (x² + y²) sin² α
x2 cos² α + y² sin² a – 2xy cos α sin α = x² sin² α + y² sin² α
∴ x² (cos² α – sin² α) – 2xy cos α sin α = 0
x².cos 2α – xy sin 2α = 0
a = cos 2α, b = 0, 2h = -sin 2α
\(\cos \theta=\frac{\|\cos 2 \alpha+0\|}{\sqrt{(\cos 2 \alpha-0)^{2}+\sin ^{2} 2 \alpha}}\)
= cos 2α
∴ θ = 2α

(iv) x² + 2xy cot a – y² = 0
a + b = 1 – 1 = 0
∴ θ = \(\frac{\pi}{2}\)

II.

Question 1.
Show that the following pairs of straight lines have the same set of angular bisectors (that is they are equally inclined to each other).
i) 2x² + 6xy + y² = 0,
4x² + 18xy + y² = 0.
ii) a²x² + 2h(a + b) xy + b²y² = 0,
ax² + 2hxy + by² = 0, a + b ≠ 0.
iii) ax² + 2hxy + by² + λ(x² + y²) = 0; (λ ∈ R),
ax² + 2hxy + by² = 0.

Solution:
(i) Combined equation of OA, OB is
2x² + 6xy + y² = 0
Equation of the pair of bisectors is
3(x² – y²) = (2 – 1) xy
3(x² – y2² = xy ………… (1)
Combined equation of OP, OQ is
4x² + 18xy + y² = 0
Equation of the pair of bisectors is
9(x² – y²) = (4 – 1) xy 9(x² – y²) = 3xy
3(x² – y²) = xy ………….. (2)
(1), (2) are same.
∴ OA, OB and OP, OQ are inclined to each other.

(ii) Combined equation of OA, OB is
a²x² + 2h(a + b) xy + b²y² = 0
Equation of the pair of bisectors is
h (a + b) (x² – y²) = (a² – b²) xy
h (a + b) (x² – y²) = (a + b)(a – b) xy
i.e., h(x² – y²) = (a – b) xy ………… (1)
Combined equation of OP, OQ is
ax² + 2hxy + by² = 0
Equation of the pair of bisectors is
h (x² – y²) = (a – b) xy …………. (2)
(1), (2) are same.
∴ OA, OB and OP, OQ are equally inclined to each other.

(iii) Combined equation of OA, OB is
ax² + 2hxy + by² + λ(x² + y²) = 0
(a + λ) x² + 2hxy + (b + λ) y² = 0
Equation of the pair of bisectors of OA, OB is
h (x2 – y2) = (a + λ – b – λ)xy
= (a – b)xy ……….. (1)
Combined equation of OP, OQ is
ax² + 2hxy + by² = 0
Equation of the pair of bisectors of OP, OQ is
h(x² – y²) = (a – b) xy …………. (2)
(1), (2) are same.
∴ OA, OB and OP, OQ are equally inclined to each other.

Question 2.
Find the value of h, if the slopes of the lines represented by 6x² + 2hxy + y² = 0 are in the ratio 1: 2.
Solution:
Combined equation of the lines is
6x² + 2hxy + y² = 0
Suppose individual equations of the given lines are y = m₁x and y = m₂x

Question 3.
If ax² + 2hxy + by² = 0 represents two straight lines such that the slope of one line is twice the slope of the other, prove that 8h² = 9ab.
Solution:
Combined equation of the lines is
ax² + 2hxy + by² =0
Suppose, y = m₁x and y = m₂x are the individual equations of the lines.
∴ m₁ + m₂ = –\(\frac{2h}{b}\), m₁m₂ = \(\frac{a}{b}\)
Given m₂ = 2m₁
∴ 3m₁ = –\(\frac{2h}{b}\) ; 2m₁² = \(\frac{a}{b}\)
m₁ = –\(\frac{2h}{3}\) ; m₁² = \(\frac{a}{2b}\)
∴ (-\(\frac{2h}{3b}\))² = \(\frac{a}{2b}\)
\(\frac{4 h^{2}}{9 b^{2}}=\frac{a}{2 b}\)
8h² = 9ab.

Question 4.
Show that the equation of the pair of straight lines passing through the origin and making an angle of 30° with the line 3x – y – 1 = 0 is 13x² + 12xy – 3y² = 0

Solution:
Equation of AB is 3x – y – 1 = 0
OA, OB make an angle of 30° with AB and pass through the origin.
Suppose slope of OA is m
∴ Equation of OA is
y – 0 = m (x – 0) = mx or mx – y = 0

∴ \(\frac{\sqrt{3}}{2}=\frac{|3 m+1|}{\sqrt{10} \sqrt{m^{2}+1}}\)
Squaring and cross multiplying
\(\frac{3\left(m^{2}+1\right)}{4}=\frac{(3 m+1)^{2}}{10}\)
15(m² + 1) = 2 (3m + 1)²
15m² + 15 = 2 (9m² + 6m + 1)
= 18m² + 12m + 2
3m² + 12m -13 = 0
Suppose m₁, m₂ are two roots of the equation
m₁ + m₂ = -4, m₁ m₂ = \(\frac{-13}{3}\)
Combined equation of OA and OB is
(m₁x – y) (m₂x – y) = 0
m₁m₂x² – (m₁ + m₂) xy + y² = 0
\(\frac{-13}{3}\)x² + 4xy + y² = 0
-13 x² + 12xy + 3y² = 0 or
13x² – 12xy – 3y² = 0

Question 5.
Find the equation to the pair of straight lines passing through the origin and making an acute angle a with the straight line x + y + 5 = 0.

Solution:
Equation of AB is x + y + 5 = 0 ……….. (1)
Slope of AB = – λ
Suppose OA and OB are the required lines
Suppose equation of OA is
y = mx ⇒ mx – y = 0

2(m² + 1) cos² α = (m – 1)²
2(m² + 1) = \(\frac{(m-1)^{2}}{\cos ^{2} \alpha}\) = (m – 1)² sec² α.
2m² + 2 = m² sec² α – 2m sec² α + sec² α.
m² (sec² α – 2) – 2m sec² α + (sec² α – 2) = 0
m₁ + m₂ = \(\frac{2 \sec ^{2} \alpha}{\sec ^{2} \alpha-2}\), m₁m₂ = 1
Combined equation of OA and OB is
(y – m₁x) (y – m₂x) = 0
y² (m₁ + m₂) xy + m₁m₂ x² = 0

Combined equation of OA and OB is
x² + 2xy sec 2a + y² = 0

Question 6.
Show that the straight lines represented by (x + 2a)² – 3y² = 0 and x = a form an equilateral triangle.
Solution:
Combined equation of OA, OB is
(x + 2a)² – 3y² = 0
(x + 2a)² – (√3y)² =0
(x + 2a + √3 y) (x + 2a – √3 y) = 0

Equation of OA is
x + √3y + 2a = 0 ………….. (1)
Equation of OB is
x – √3y + 2a = 0 ………. (2)
Equation of AB is x – a = 0

∴ ∠OBA =60°
∴ ∠AOB = 180°- (∠OAB + ∠OBA)
= 180° – (60° + 60°)
= 180° – 120°
= 60°
∴ ∆OAB is an equilateral triangle.

Question 7.
Show that the pair of bisectors of the angles between the straight lines (ax + by)² = c (bx – ay)², c > 0 are parallel and perpen-dicular to the line ax+ by + k= 0.
Solution:
Combined equation of the given lines is (ax + by)² = c (bx – ay)²
a²x² + b²y² + 2ab xy = c (b²x² + a²y² – 2abxy)
= cb²x² +ca²y² – 2cabxy
(a² – cb²)x² + 2ab (1 + c²) xy + (b² – ca²)y² = 0
Equation of the pair of bisectors is
h (x – y²) = (a – h) xy ^
ab (1 + c) (x² – y²)
= (a² – cb² – b² + ca²) (x² – y²) = 0
= (a² – b²)(1 + c) xy.
i.e., ab (x² – y²) – (a² – b²) xy = 0
(ax + by) (bx – ay) = abx² – a2xy +b²xy – aby²
= ab(x² – y²) – (a² – b²)xy
∴ The equation of the pair of bisectors are (ax + by) (bx – ay) = 0
The bisectors are ax + by = 0 and bx – ay = 0
ax + by = 0 is parallel to ax + by + k = 0
bx – ay = 0 is perpendicular to ax + by +k=0.

Question 8.
The adjacent sides of a parallelogram are 2x² – 5xy + 3y² = 0 and one diagonal is x + y + 2 = 0. Find the vertices and the other diagonal.

Solution:
Combined equation of OA and OB is 2x² – 5xy + 3y² = 0
Equation of AB is x + y+ 2 = 0
y = -(x + 2)
Substituting in (1)
2x² + 5x (x + 2) + 3(x +2)² = 0
2x² + 5x² + 10x + 3(x² + 4x + 4) = 0
7x² + 10x + 3x² + 12x + 12 = 0
10x² + 22x + 12 = 0
5x² + 11x + 6 = 0
(x + 1) (5x + 6) = 0
x + 1 = 0 or 5x + 6 = 0
x = -1 or 5x = -6
x = –\(\frac{6}{5}\)
y = -(x + 2)
x = -1 ⇒ y = – (-1 + 2) = – 1
⇒ co-ordinates of A are (-1, -1)
x = –\(\frac{6}{5}\) ⇒ y = -(\(\frac{6}{5}\)+ 2) = –\(\frac{4}{5}\)
⇒ co-ordinates of B are (-\(\frac{6}{5}\), –\(\frac{4}{5}\))
Suppose the diagonals AB, OC intersect in O’ O’ bisects AB and OC.
Suppose co-ordinates of C are (x, y)
Midpoint of OC = Midpoint of AB

∴ The vertices are O(0, 0), A(-1, -1)
C(-\(\frac{11}{5}\), –\(\frac{9}{5}\)), B(-\(\frac{6}{5}\), –\(\frac{4}{5}\))

Question 9.
Find the centroid and the area of the triangle formed by the following lines.
(i) 2y² – xy – 6x² = 0, x + y + 4 = 0
(ii) 3x² – 4xy + y² = 0, 2x – y = 6

Solution:
(i) Combined equation of OA, OB is
2y² – xy – 6x² = 0 ………… (1)
Equation of AB is x + y + 4 = 0
y = – (x + 4) ………… (2)
Substituting in (1)
2(x + 4)² + x (x + 4) – 6x² = 0
2(x² + 8x + 16) + x² + 4x – 6x² = 0
2x² + 16x + 32 + x² + 4x – 6×2 = 0
-3x² + 20x + 32 = 0
3x² – 20x – 32 = 0
(3x + 4) (x-8) = 0
3x + 4 = 0 or x – 8 = 0
x = –\(\frac{4}{3}\) or 8

Case (i) : x = –\(\frac{4}{3}\)
y = – (x + 4)
= -(\(\frac{-4}{3}\) + 4) = –\(\frac{8}{3}\)
Co – ordinates of A are (-\(\frac{4}{3}\), –\(\frac{8}{3}\))

Case (ii) : x = 8
y = -(x + 4) = – (8 + 4) = – 12
Co-ordinates of B are (8, – 12)
Suppose G is the centroid of ∆ AOB
Co-ordinates of G are

(ii) Combined equation of OA, OB is
3x² – 4xy + y² = 0 ……………. (1)
Equation of AB is 2x – y = 6
y = 2x – 6 ……………. (2)
Substituting in (1)
3x² – 4x (2x – 6) + (2x – 6)² = 0
3x² + 8x² + 24x + 4x² + 36 – 24x = 0
– x + 36 = 0
x² – 36 = 0
(x + 6) (x – 6) = 0
x + 6 = 0 or x – 6 = 0
x = – 6 or 6
y = 2x – 6
x = 6 ⇒ y = 12 – 6 = 6
Go -ordinates of A are (6, 6)
x = -6 ⇒ y = – 12 – 6 = -18
Co-ordinates of B are (-6, -18)
Co-ordinates of G ate
(\(\frac{0+6-6}{3}\), \(\frac{0+6-18}{3}\)) = (0, -4)
∆OAB = \(\frac{1}{2}\)|x₁y₂ – x₂y₁|
= \(\frac{1}{2}\)|16 (-18) – (-6). 6|
= \(\frac{1}{2}\)|- 108 + 36|
= \(\frac{1}{2}\) . 72 =36 sq.units

Question 10.
Find the equation of the pair of lines intersecting at (2, -1) and
(i) perpendicular to the pair
6x² – 13xy – 5y² = 0 and
(ii) parallel to the pair
6x² – 13xy – 5y² = 0.
Solution:
Equation of OA, OB is 6x² – 13xy – 5y² = 0
(i) Equation of the pair of lines through (x₁ y₁) and perpendicular to
ax² + 2hxy + by² = 0 is
b(x – x₁)² – 2h(x – x₁) (y – y₁) + a (y – y₁)² = 0
Equation of the perpendicular pair of lines is
-5(x – 2)² + 13(x – 2) (y + 1) + 6(y+ 1)² =0
-5(x² – 4x + 4) + 13(xy + x – 2y – 2) + 6(y² + 2y + 1) = 0
-5x² + 20x – 20 + 13xy + 13x – 26y – 26 + 6y² + 12y + 6 = 0
-5x² + 13xy + 6y² + 33x – 14y – 40 = 0
or 5x² – 13xy – 6y² – 33x + 14y + 40 = 0

(ii) Equation of the pair of lines through (x₁, y₁) and parallel to ax² + 2hxy + by² = 0 is
a(x – x₁)² + 2h (x – x₁) (y – y₁) + b (y – y₁)² = 0
Equation of the pair of parallel lines is
6(x- 2)² – 13(x – 2) (y + 1) – 5(y + 1)² = 0
6(x² – 4x + 4) – 13(xy + x – 2y – 2) – 5(y² + 2y + 1) = 0
6x² – 24x + 24 – 13xy – 13x + 26y + 26 – 5y² – 10y – 5 = 0
6x² – 13xy – 5y² – 37x + 16y + 45 = 0.

Question 11.
Find the equation of the bisector of the acute angle between the lines
3x – 4y + 7 = 0 and 12x + 5y – 2 = 0
Solution:
Given lines
3x – 4y + 7 = 0 ………….. (1)
12x + 5y – 2 = 0 ………… (2)
The equations of bisector’s angles between (1) & (2) is

13 (3x – 4y + 7) ± 5 (12x + 5y- 2) = 0
(39x – 52y + 51) ± (60x + 25y – 10) = 0

(i) 39x- 52y + 51 + 60x + 25y- 10 = 0
99x-27y + 41 = 0 ……… (3)

(ii) (39x – 52y + 51) – (60x + 25y- 10) = 0
39x – 52y + 51 – 60x – 25y +10 = 0
– 21x – 77y + 61 =0
21x + 77y- 61 = 0 ……… (4)
Let ‘θ’ be the angle between (1), (4)

∴ (4) is obtuse angle bisector, then other one (3) is the accute angle bisector.
∴ 99x – 27y + 41 = 0 is the accute angle bisector.

Question 12.
Find the equation of the bisector of the obtuse angle between the lines x + y – 5 = 0 and x – 7y + 7 = 0
Solution:
Given lines
x + y- 5 = 0 ………. (1)
x – 7y + 7 = 0 ……..(2)
The equations of bisectors of angles between (1), (2) is

⇒ (5x + 5y-25)±(x-7y + 7) = 0

(i) 5x + 5y – 25 + x – 7y + 7 = 0
6x – 2y – 18 = 0
3x-y-9 = 0 ………. (3)

(ii) (5x + 5y – 25) – (x – 7y + 7) = 0
4x+ 12y – 32 = 0
x + 3y – 8 = 0 ……… (4)
Let ‘θ’ be the angle between (1), (4)

∴ (4) is the ocute angle bisector, then other one 3x – y – 9 = 0 is the obtuse angle bisector.

III.

Question 1.
Show that the lines represented by (lx + my)² – 3(mx – ly)² = 0 and lx + my + n = 0 form an equilateral triangle with area \(\frac{n^{2}}{\sqrt{3}\left(l^{2}+m^{2}\right)}\).
Solution:
Combined equation of A and is
(lx + my)² – 3(mx – ly)² = 0
l²x² + m²y² + 2lmxy – 3m²x² – 3l²y² + 6 lmxy = 0
(l² – 3m²) x² + 8lmxy + (m² – 3l²) y² = 0

Combined equation of the bisectors of OA
and OB is h (x² – y²) = (a – b) xy
4 lm (x² – y²) = (l² – 3m² – m² + 3l²) xy
4 lm (x² – y²) = 4(l² – m²) xy
lmx² – (l² – m²)xy – lmy² = 0
(lx – my) (mx – ly) = 0
lx + my = 0 and mx – ly = 0
∴ The bisectors mx – ly = 0 is perpendicular to AB whose equation lx + my + n = 0
OAB is an scales triangle and ∠AOB = 60°
OAB is an equalated tringle
P = Length of the X lan prove P and AB

Question 2.
Show that the straight tines represented by 3x² + 48xy + 23y² = 0 and 3x – 2y + 13 = 0 form an equilateral triangle of area \(\frac{13}{\sqrt{3}}\) sq.umts.
Solution:
Combined equation of OA, OB is
3x² + 48xy + 23y² = 0 …………. (1)
Equation of AB is 3x – 2y + 13 = 0 …….. (2)
(1) can be written as
(9x² – 12xy + 4y²) – 3(4x² + 12xy + 9y²) = 0
i.e., (3x – 2y)² – 3(2x + 3y)² = 0
⇒ [(3x – 2y) + √3(2x +3y)] [(3x – 2y) – √3(2x+3y)] = 0
⇒ [(3 + 2√3)x+ (3√3 – 2)y] [(3 – 2√3)x – (3√3 + 2)y]=0
Equation of OA is
(3 + 2√3)x – (3√3 – 2)y = 0 ………….. (1)
Equation of OB is
(3 – 2√3)x – (3√3 +2)y =0 ………… (2)

∴ OAB is an equilateral triangle.

Question 3.
Show that the equation of the pair of lines bisecting the angles between the pair of bisectors of the angles between the pair of lines ax² + 2hxy + by² = 0 is (a – b) (x² – y²) + 4hxy = 0
Solution:
Equation of the given lines is
ax² + 2hxy + by² = 0
Equation of the pair of bisectors is
h(x² – y²) = (a – b)xy …………. (1)
hx² – hy² – (a – b) xy = 0
∴ A = h, B = -h, 2H = -(a – b)
Equation of the pair of bisectors of (1) is
H(x² – y²) = (A – B) xy
–\(\frac{(a-b)}{2}\)(x² – y²) = 2hxy
-(a – b) (x² – y²) = 4hxy
or (a – b) (x² – y²) + 4hxy = 0
∴ Equation of the pair of bisectors of the pair of bisectors of ax² + 2hyx + by² = 0 is
(a – b) (x² – y²) + 4hxy = 0.

Question 4.
If one line of the pair of lines ax² + 2hxy + by² = 0 bisects the angle between the co-ordinate axes, prove that (a + b)²= 4h².
Solution:
The angular bisectors of the co-ordinate axes are:
y = ±x
Case (i) :
y = x is one of the lines of
ax² + 2hxy + by² = 0
x²(a + 2h + b) = 0
a + 2h + b = 0 ………….. (1)

Case (ii) :
y = – x is one of the lines of
ax² + 2hxy + by² = 0
x² (a – 2h + b) = 0
a – 2h + b = 0 ………. (2)
Multiplying (1) and (2), we get
(a + b + 2h).(a + b – 2h) = 0
(a + b)² – 4h² = 0
(a + b)² = 4h².

Question 5.
If (α, β) is the centroid of the triangle formed by the lines ax² + 2hxy + by² =0 and lx + my = 1, prove that

Solution:
Combined equation of OA, OB is
ax² + 2hxy + by² = 0 …………. (1)
Equation of AB is lx + my = 1
my = 1 – lx
\(y=\frac{1-1 x}{m}\) …………. (2)
Substituting in (1)
ax² + 2hx\(\frac{(1-b)}{m}\) + b\(\frac{(1-1 x)^{2}}{m^{2}}\) = 0
am²x² + 2hmx(1 – lx) + b(1 + l²x² – 2lx) = 0
am²x² + 2hmx – 2hlmx² + b + bl²x² – 2blx = 0
(am² – 2hlm + bl²)x² – 2(bl – hm)x + b = 0
Suppose coordinates of A are (x₁, y₁) and B are (x₂, y₂)
x₁ + x₂ = \(\frac{2(b /-h m)}{a m^{2}-2 h / m+b l^{2}}\) …………. (3)
A and B are points on
lx + my = 1
lx₁ + my₁ = 1
lx₂ + my₂ = 1
l(x₁ + x₂) + m(y₁ + y₂) = 2
m(y₁ + y₂) = 2 – l(x₁ + x₂)

Co-ordinates of the vertices are
O(0, 0), A(x₁, y₁), B(x₂, y₂)
Co-ordinates of G are

Question 6.
Prove that the distance from the origin to the orthocentre of the triangle formed by the lines \(\frac{x}{\alpha}+\frac{y}{\beta}\) = 1 and ax² + 2hxy + by² = 0 is (α² + β²)^1/2 \(\left|\frac{(a+b) \alpha \beta}{a \alpha^{2}-2 h \alpha \beta+b \beta^{2}}\right|\).
Solution:
Let ax² + 2hxy + by² = 0 represent the lines
l₁x + m₁y = 0 ………… (1)
l₂x + m₂y = 0 ………… (2)
∴ (l₁x + m₁y) (l₂x + m₂y) = ax² + 2hxy + by²
Comparing both sides
l₁l₂ = a, m₁m₂ = b, l₁m₂ + l₂ m₁ = 2h
Given line is lx + my =1 ……….. (3)
Clearly the origin O is the point of intersection of (1) & (2)
Let A be the point of intersection of (1) & (3)

By the method of cross multiplication,


Let B be the point of intersection of (2) & (3)
Let P be the orthocentre of ∆ OAB.

⇒ yl₂(l₁α – m₁β) – αβl₁l₂ = m₂(x(l₁α – m₁β) + m₁αβ)
⇒ (l₁α – m₁P) (m₂x – l₂y) = m₁m₂αβ + l₁l₂αβ

Question 7.
The straight line lx + my + n = 0 bisects an angle between the pair of lines of which one is px + qy + r = 0. Show that the other line is (px + qy + r) (l² + m²) – 2(lp + mq) (lx + my + n) = 0.
Solution:
lx + my + n – 0 is a bisector and let (a, P) be any point on it so that
lα + mβ + n = 0 …………….. (1)
The other line will pass through the intersection of given lines and given bisector and hence by p +λq = 0
Its equation is
(px + qy + r) + λ(lx + my + n) = 0 …………….. (2)
Also px + qy + r = 0
If (α, β) be a point on the bisector then its perpendicular distance from the lines (2) and (3) is same.

Putting lα + mβ + n = 0 by (1) in the above and cancelling pα + qβ + r and then squaring both sides, we get
(p + lλ)² + (q + mλ)² = p² + q² or
2λ(pl + qm)+ λ²(l² + m²) = 0
∴ λ = -2\(\frac{p l+Q m}{l^{2}+m^{2}}\)
Substitute X value in (2),
(px + qy + r) + \(\left(\frac{-2(p /+Q m)}{l^{2}+m^{2}}\right)\) lx + my + n = 0
⇒ (px + qy + r)(l² + m²) -2(pl + qm) (lx + my + n ) = 0

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B The Straight Line Solutions Exercise 3(e) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B The Straight Line Solutions Exercise 3(e)

I.

Question 1.
Find the incentre of the triangle whose vertices are (1, √3) (2, 0) and (0, 0)
Solution:
0(0, 0), A (1, √3), B (2, 0) are the vertices of ∆ ABC

∴ ABC is an equilateral triangle
Co-ordinates of the in centre are

Question 2.
Find the orthocentre of the triangle whose sides are given by x + y + 10 = 0, x – y – 2 = 0 and 2x + y – 7 = 0
Solution:
Equation of AB is x + y + 10 = 0 ………… (1)
Equation of BC is x – y – 2 = 0 ………… (2)
Equation of AC is 2x + y – 7 = 0 ………… (3)

Solving (1) and (2) co – ordinates of B are (-4, -6)
Solving (1) and (3) co – ordinates of A are (17, -27)
Equation of BC is x – y – 2 = 0
AD is perpendicular to BC.
Equation of AD is x + y + k = 0
AD passes through A (17, -27)
17 – 27 + k = 0 ⇒ k = 10
∴ Equation of AB is x + y + 10 = 0 ………….. (1)
Equation of AC is 2x + y – 7 = 0
BE is perpendicular to AC.
Equation of DE can be taken as x – 2y = k
BE passes through D (-4, -6)
-4 + 12 = k ⇒ k = 8
Equation of BE is x – 2y = 8

3y = -18 ⇒ y = – 6 x + y+10 = 0
⇒ x – 6+10 = 0
x = 6 – 10 = -4
Orthocentre of A ABC is (- 4, – 6)

Question 3.
Find the orthocentre of the triangle whose sides are given by 4x – 7y + 10 = 0, x + y = 5 and 7x + 4y = 15
Solution:
Equation of AB is. 4x – 7y + 10 = 0 …………. (1)
Equation of BC is x + y = 5 …………. (2)
Equation of AC is 7x + 4y – 15 = 0 …………. (3)
AB and AC are perpendicular and ∠A = 90°
∴ ABC is a right angled triangle
Right angled vertex A is the orthocentre
Solving (1) and (3)

Orthocentre is O (1,2)

Question 4.
Find the circumcentre of the triangle whose sides are x = 1, y = 1 and x + y = 1
Solution:
Equation of AB is x = 1
Equation of BC is y = 1
Equation of AC is x + y = 1

AB and BC are perpendicular
∴ ABC is a right angled triangle and ∠B = 90°
Mid point of AC is the circumcentre
Co-ordinates of A are (1,0) and C are (0,1)
Circumcentre is (\(\frac{1}{2}\), \(\frac{1}{2}\))

Question 5.
Find the incentre of the triangle formed by the lines x = 1, y = 1 and x + y = 1
Solution:
Equation of AB is x = 1
Equation of BC is y = 1
Equation of AC is x + y = 1
Solving the vertices are A(1, 0), B(1, 1), C (0, 1)

Question 6.
Find the circumcentre of the triangle whose vertices are (1,0),(-l, 2) and (3,2)
Solution:
A (1, 0), B (-1, 2), C (3, 2) are the vertices of ∆ ABC

S (x, y) is the circumcentre of ∆ ABC
SA = SB = SC
SA = SB ⇒ SA² = SB²
(x – 1)² + y² = (x + 1)² + (y – 2)²
x² – 2x + 1 + y² = x² + 2x + 1 + y² – 4y + 4
4x – 4y = – 4 ⇒ x – y = -1 …….. (1)
SB = SC ⇒ SB² = SC²
(x+ 1)² + (y – 2)² = (x-3)² + (y – 2)²
x² + 2x + 1 = x² – 6x + 9
8x = 8 ⇒ x = 1
From (1), 1 – y = -1
y = 2
∴ Circumcentre is (1, 2)

Question 7.
Find the value of k, if the angle between the straight lines kx + y + 9 =0 and 3x – y + 4 = 0 is π/4
Solution:
Equations of the given lines are
kx + y + 9 = 0
3x – y + 4 = 0

Squaring and cross-multiplying
5k² + 5 = (3k – 1)² = 9k² – 6k + 1
4k² – 6k – 4 = 0
2k² – 3 k – 2 = 0
(k – 2) (2k + 1) =0
k = 2 or -1/2

Question 8.
Find the equation of the straight line passing through the origin and also through the point of intersection of the lines 2x – y + 5 = 0 and x + y + 1 = 0.

Solution:
Equation of AB is L₁ = 2x – y + 5 = 0
Equation of AC is L₂ = x + y+ l = 0
Equation of any line passing through A is
L₁ + kL₂ = 0
(2x – y + 5) +k (x + y + 1) = 0 ……….. (1)
This line passes through O (0, 0)
5 + k = 0 ⇒ k = -5
Substituting in (1), equation of OA is
(2x – y + 5) – 5(x + y + 1) =0
2x – y + 5 – 5x – 5y – 5 = 0
-3x- 6y = 0 ⇒ x + 2y = 0

Question 9.
Find the equation of the straight line parallel to the line 3x + 4y = 7 and passing through the point of intersection of the lines x – 2y – 3 = 0 and x + 3y – 6 = 0.
Solution:
Equation of the given lines are
L₁ = x – 2y – 3 = 0 and
L₂ = x + 3y – 6 = 0
Equation of any line passing through the intersection is L₁ + kL₂ = 0
(x – 2y – 3) + k(x + 3y – 6) = 0
(1 + k)x + (-2 + 3k)y + (-3 – 6k) = 0
This line is parallel to 3x + 4y = 7
a₁b₂ = a₂b₁
3(-2 + 3k) = (1+ k) 4
-6 + 9k = 4 + 4k ⇒ 5k=10 ⇒ k = 2
Equation of the required line is 3x + 4y – 15 = 0

Question 10.
Find the equation of the straight line perpendicular to the line 2x + 3y = 0 and passing through the point of intersection of the lines x + 3y – 1 = 0 and x – 2y + 4 = 0.
Solution:
Equation of AB is x + 3y – 1 = 0
Equation of AC is x – 2y + 4 = 0

Equation of any line through A is
(x + 3y – 1) + k(x – 2y + 4) = 0
(1 + k)x + (3 – 2k)y + (4k – 1) = 0 ………… (1)
This line is perpendicular to 2x + 3y = 0
a₁a₂ + b₁b₂ = 0
2(1 +k) + 3(3 – 2k) = 0
2 + 2k + 9 – 6k = 0
4k = 11 ⇒ k = \(\frac{11}{4}\)
Substituting in (1), equation of the required line AD is
(1 + \(\frac{11}{4}\))x + (3 – \(\frac{11}{2}\))y + (11 – 1) = 0
\(\frac{15}{4}\) x – \(\frac{5}{2}\)y + 10 = 0
15x – 10y + 40 = 0
3x – 2y + 8 = 0

Question 11.
Find the equation of the straight line making non-zero equal intercepts on the coordinate axes and passing through the point of intersection of the lines 2x – 5y + 1 = 0 and x – 3y – 4 = 0.
Solution:
Equation of the given lines are
L₁ = 2x – 5y + 1 = 0
L₂ = x – 3y – 4 = 0
Equation of any line passing through the intersection of these lines is L₁ + kL2 = 0
(2x – 5y + 1) + k(x – 3y – 4) = 0
(2 + k)x – (5 + 3k)y + (1 – 4k) = 0 …………. (1)
Intercepts on co-ordinate axes are equal
2 + k = -5 – 3k
4k = – 7 ⇒ k = -7/4
Substituting in (1)
Equation of the required line is
(2 – \(\frac{7}{4}\))x – (s – \(\frac{21}{4}\))y+ (1 + 7) = 0
\(\frac{1}{4}\) x + \(\frac{1}{4}\)y + 8 = 0
⇒ x + y + 32 = 0

Question 12.
Find the length of the perpendicular drawn from the point of intersection of the lines 3x + 2y + 4 = 0 and 2x + 5y – 1 = 0 to the straight line 7x + 24y -15 = 0.
Solution:
Equation of the given lines
3x + 2y + 4 = 0
2x + 5y – 1 = 0

x = \(\frac{-22}{11}\) = -2, y = \(\frac{11}{11}\) = 1
Co-ordinates of P are (-2, 1)
Equation of the line is 7x + 24y- 15 = 0
Length of the perpendicular

Question 13.
Find the value of ‘a’ if the distances of the points (2, 3) and (-4, a) from the straight line 3x + 4y – 8 = 0 are equal.
Solution:
Equation of P’Q’ is 3x + 4y – 8 = 0 P (2, 3), Q (- 4, a) are the given points
PP’ and QQ’ are the perpendicular from P and Q.

PP’ = QQ’
\(\frac{|3.2+4.3-8|}{\sqrt{9+16}}=\frac{|3 .(-4)+4 a-8|}{\sqrt{9+16}}\)
10 = |4a – 20|
4a – 20 = ±10 ⇒ 4a = 20 ± 10 = 30 or 10
a = \(\frac{30}{4}\) or \(\frac{10}{4}\)
i.e., a = \(\frac{15}{2}\) oe \(\frac{5}{2}\)

Question 14.
Find the circumcentre of the triangle formed by the straight lines x + y = 0, 2x + y + 5 = 0 and x – y = 2.
Solution:
Equation of AB is x + y = 0 ………….. (1)
Equation of BC is 2x + y + 5 = 0 ………….. (2)
Equation of AC is x – y = 2 ………….. (3)

Solving (1) and (2) co-ordinates of D are (-5, 5)
Solving (2) and (3) co-ordinates of C are (-1, -3)
Solving (1) and (3) co-ordinates of A are (1, -1)
Suppose S(x, y) is the circumcentre
SA = SB = SC
SA = SB ⇒ SA² = SB²
(x + 5)² + (y – 5)² = (x + 1)² + (y + 3)²
x² + 10x + 25 + y² – 10y + 25
= x² + 2x + 1 + y² + 6y + 9
8x – 16y = -40
x – 2y = -5 ………… (1)
SB = SC ⇒ SB² = SC²
(x+ 1)² + (y + 3)² = (x -1)² + (y + 1)²
x² + 2x + 1 + y² + 6y+9
= x² – 2x + 1 + y² + 2y + 1
4x + 4y = -8
x + y = -2 …………… (2)
(2) – (1) gives 3y = 3 ⇒ y = 1
x + 1 = -2 ⇒ x = -3
Circumcentre is S(-3, 1)

Question 15.
If 6 is the angle between the lines \(\frac{x}{a}+\frac{y}{b}=1\) and \(\frac{x}{b}+\frac{y}{a}=1\) , find the value of sin 0 when a > b.
Solution:
Equation of AB is. \(\frac{x}{a}+\frac{y}{b}=1\) ⇒ bx + ay = ab
Equation of AC is. \(\frac{x}{b}+\frac{y}{a}=1\) ⇒ ax + by = ab

II.

Question 1.
Find the equation of the straight lines passing through the point (-10, 4) and making an angle 0 with the line x – 2y = 10 such that tan θ = 2.
Solution:
Equation of QR is x – 2y = 10

Suppose slope of PQ is m, PQ passes through P(-10, 4)
Equation Of PQ is y – 4 = m(x + 10)
= mx + 10m
i.e., mx-y + (10m + 4) = 0 …………… (1)
tan θ = 2 ⇒ cos θ = \(\frac{1}{\sqrt{5}}\)

Squaring and cross multiplying
m² + 1 = (m + 2)²
= m² + 4m + 4
4m + 3 = 0
m = –\(\frac{3}{4}\)

Case (i) : Co-efficient of m² = 0
⇒ One of the roots is ∞
Hence PR is a vertical line
∴ Equation of PR is x + 10 = 0

Case (ii): m = –\(\frac{3}{4}\)
Substituting in (1)
Equation of PQ is – \(\frac{3}{4}\)x – y + (\(\frac{30}{4}\) + 4) = 0
\(\frac{-3 x-4 y-14}{4}=0\)
⇒ 3x + 4y + 14 = 0

Question 2.
Find the equation of the straight lines pass-ing through the point (1, 2) and making an angle of 60° with the line √3x + y + 2 = 0.
Solution:
Equation of QR is √3x + y + 2 = 0

PQ and PR passes through P( 1, 2) and makes an angle of 60° with QR
Suppose slope of PQ is m.
Equation of PQ is
y – 2 = m(x – 1)
= mx – m
mx – y + (2 – m) = 0 ……………. (1)

Squaring and cross multiplying
m² + 1 = (√3 – 1)²
= 3m² + 1 – 2√3m
2m² – 2√3m = 0
2m(m – √3) = 0
m = 0 or √3

Case (i) : m = 0
Equation of PQ is -y+2 = 0 or y-2 = 0

Case (ii): m = √3
Equation of PQ is √3x – y + (2 – √3) = 0

Question 3.
The base of an equilateral triangle is x + y – 2 = 0 and the opposite vertex is (2, -1). Find the equation of the remaining sides.
Solution:
ABC is an equilateral triangle.
∴ ∠B = C = 60°

Equation of BC is x + y – 2 = 0
AB passes through A(2, -1)
Suppose slope of AB = m
Equation of AB is
y + 1 = m(x – 2)
= mx – 2m
mx – y – (2m + 1) = 0 …………. (1)

Squaring and cross multiplying
m² + 1 = 2 (m – 1)² = 2 (m² – 2m + 1)
= 2m² – 4m + 2
m² – 4m +1 = 0
\(m=\frac{4 \pm \sqrt{16-4}}{2}=\frac{4 \pm 2 \sqrt{3}}{2}=2 \pm \sqrt{3}\)
Substituting in (1)
Equation of AB is y + 1 = (2 + √3) (x – 2)
Equation of AC is y + 1 = (2 – √3) (x – 2)

Question 4.
Find the orthocentre of the triangle with following vertices.
i) (-2, -1), (6, -1) and (2, 5)
ii) (5,-2), (-1, 2) and (1, 4)
Solution:
i) A(-2, -1), B(6, -1), C(2,5) are the vertices of ∆ ABC.

Slope of BC = \(\frac{5+1}{2-6}=\frac{6}{-4}=-\frac{3}{2}\)
AD is perpendicular to BC
Slope of AD = \(\frac{2}{3}\)
Equation of AD is
y + 1 = \(\frac{2}{3}\) (x + 2)
2x – 3y + 1 = 0 …………….. (1)
Slope of AC = \(\frac{5+1}{2+2}=\frac{6}{4}=\frac{3}{2}\)
BE is ⊥^lr to AC
Slope of BE = –\(\frac{2}{3}\)
Equation of BE is
y + 1 = – \(\frac{2}{3}\)(x – 6)
2x + 3y – 9 = 0 …………. (2)
By solving (1),(2)

∴ Co-ordinates of the orthocentre
O are = (2, \(\frac{5}{3}\))

ii) (5, -2), (-1, 2) and (1, 4)
A(5, -2), B(-1, 2), C(1, 4) are the vertices of ∆ ABC.
Slope of BC = \(\frac{2-4}{-1-1}=\frac{-2}{-2}=1\)
AD is perpendicular to BC

Slope of AD = –\(\frac{1}{m}\) = -1 m
Equation of AD is
y + 2 = -(x – 5)
= -x + 5
x + y – 3 = 0 …………. (1)
Slope of AC = \(\frac{4+2}{1-5}=\frac{6}{-4}=-\frac{3}{2}\)
BE is perpendicular to AC
Slope of BE is –\(\frac{1}{m}\) = \(\frac{2}{3}\)
Equation of BE is y – 2 = j(x + 1)
3y – 6 = 2x + 2
2x – 3y + 8 = 0 ……….. (2)
From (1), (2) by cross – multiplication law

Co-ordinates of the Orthocentre O are (\(\frac{1}{5}\), \(\frac{14}{5}\))

Question 5.
Find the clrcumcentre of the triangle whose vertices are given below.
i) (-2, 3) (2, -1) and (4, 0)
ii) (1, 3), (0, -2) and (-3, 1)
Solution:
i) A(-2, 3), B(2, -1), C(4, 0) are the vertices of ∆ ABC.
D is the midpoint of BC

Co-ordinates of D are (\(\frac{2+4}{2}\), \(\frac{-1+0}{2}\)
= (3, \(\frac{-1}{2}\))
Slope of BC = \(\frac{-1-1}{2-4}=\frac{-1}{-2}=\frac{1}{2}\)
SD is perpendicular to BC
Slope of SD = –\(\frac{1}{m}\) = -2
Equation of SD is y + \(\frac{1}{2}\) = -2(x – 3)
2y + 1 = -4(x – 3)
= -4x+ 12
4x + 2y – 11 = 0 ………….. (1)
E is the midpoint of AC
Co-ordinates of E are (\(\frac{-2+4}{2}\), \(\frac{3+0}{2}\)) = (1, \(\frac{3}{2}\))
Slope of AC = \(\frac{3-0}{-2-4}=-\frac{3}{6}=-\frac{1}{2}\)
SE is perpendicular to AC
Slope of SE = –\(\frac{1}{m}\) = 2
Equation of SE is y – \(\frac{3}{2}\) = 2(x – 1)
2y- 3 = 4(x- 1)
= 4x – 4
4x – 2y – 1 = 0 ……………. (2)
4x + 2y – 11 = 0 ……………. (1)
Adding (1), (2) ⇒ 8x- 12 = 0
8x = 12
x = \(\frac{12}{8}\) = \(\frac{3}{2}\)
Substituting in (1)
2y = 11 – 4x = 11 – 4 . \(\frac{3}{2}\) = 11 – 6 = 5
y = \(\frac{5}{2}\)
∴ Co-ordinates of S are (\(\frac{3}{2}\), \(\frac{5}{2}\))

ii) (1, 3), (0, -2) and (-3, 1)

In ∆ ABC,
A = (1, 3), B = (0, -2), C = (-3, 1)
D is the midpoint of BC

SD is ⊥^lr to BC
Slope of SD = 1
Equation of SD is
y + \(\frac{3}{2}\) = 1(x + \(\frac{3}{2}\))
⇒ 2y + 1 = 2x + 3
⇒ 2x – 2y + 2 = 0
⇒ x – y + 1 = 0 …………… (1)
E is the midpoint of CA
⇒ E = (\(\frac{-3+1}{2}\), \(\frac{1+3}{2}\)) = (-1, 2)
Slope of CA = \(\frac{1-3}{-3-1}\) = \(\frac{1}{2}\)
SE is ⊥^lr to CA
Slope of SE = -2
Equation of SE is
y – 2 = -2(x + 1)
⇒ y – 2 = -2x – 2
⇒ 2x + y = 0 …………….. (2)
By solving (1), (2)

Question 6.
Let \(\overline{\mathrm{P S}}\) be the median of the triangle with vertices P(2, 2) Q(6, -1) and R(7, 3). Find the equation of the straight line passing through (1, -1) and parallel to the median \(\overline{\mathrm{P S}}\).

Solution:
P(2, 2), Q(6, -1), R(7, 3) are the vertices of ∆ ABC.
S is the mid point of QR

AB is parallel to PS and passes through A(1, -1)
Equation of AB is y + 1 = – \(\frac{2}{9}\) (x – 1)
9y + 9 = -2x + 2
2x + 9y + 7 = 0

III.

Question 1.
Find the orthocentre of the triangle formed by the lines.
x + 2y = 0, 4x + 3y – 5 = 0 and 3x + y = 0.

Solution:
Equation of AB is x + 2y = 0 ………….. (1)
Equation of BC is 4x + 3y – 5 = 0 ………….. (2)
Equation of AC is 3x + y = 0 ………….. (3)
Solving (1) and (2) Co-ordinates of A are (0, 0)

4x – 8 = 0 ⇒ 4x = 8, x=2
Co-ordinates of B are (2, -1)
Equation of BC is 4x + 3y – 5 = 0
AB is perpendicular to BC and passes through A(0, 0)
Equation of AB is 3x – 4y = 0 ……….. (1)
BE is perpendicular to AC
∴ Equation of BE is x – 3y = k
BE passes through B (2, -1)
2 + 3 = k ⇒ k = 5
Equation of BE is x – 3y – 5 = 0 …………………. (2)

x = – 4, y = -3
∴ Orthocentre is O (- 4, -3)

Question 2.
Find the circumcentre of the triangle whose sides are given by x + y + 2 = 0, 5x – y – 2 = 0 and x – 2y + 5 = 0.
Solution:
Given lines are
x + y + 2 = 0 ………………. (1)
5x – y – 2 = 0 ………………. (2)
x – 2y + 5 = 0 ……………… (3)
Point of intersection of (1) and (2) is A = (0, -2)
Point of intersection of (2) and (3) is B = (1, 3)
Point of intersection of (1) and (3) is C = (-3, 1)
Let S = (α, β) the orthocentre of ∆ ABC then
SA = SB = SC
⇒ SA² = SB² = SC²
⇒ (α – 0)² + (β + 2)² = (α – 1)² + (β – 3)² = (α + 3)² + (β – 1)²

(a) = (b) ⇒ α² + β² + 4β + 4 = α² + β² – 2α – 6β + 10
⇒ 2α + 10β – 6 = 0
⇒ α + 5β – 3 = 0 ………. (4)

(a) = (c) => α² + β² + 4β + 4 = α² + β² + 6α – 2β + 10
⇒ 6α – 6β + 6 = 0
⇒ α – β + 1 = 0 ………….. (5)
From (4) and (5)

Question 3.
Find the equation of the straight lines passing through (1, 1) and which are at a distance of 3 units from (-2, 3).
Solution:
AB passes through A(1, 1)
Suppose slope of AB is m
Equation of AB is y – 1 = m(x – 1)
= mx – m
mx – y + (1 – m) = 0 ………….. (1)
Distance from (-2, 3) on AB = 3
\(\frac{|-2 m-3+1-m|}{\sqrt{m^{2}+1}}=3\)
Squaring and cross multiplying
(3m + 2)² = 9(m² + 1)
9m² + 4 + 12m = 9m² + 9
12m = 5 ⇒ m = \(\frac{5}{12}\)
Co-efficient of m² = 0 ⇒ m = ∞

i) m = ∞
AB is a vertical line
Equation of AB is x = a
AB passes through A(1, 1)
∴ a = 1
Equation of AB is x = 1 S

ii) m = \(\frac{5}{12}\)
substituting in (1),
Equation of AB is \(\frac{5}{12}\)x – y + [1 – \(\frac{5}{12}\)] = 0
\(\frac{5}{12}\)x – y + \(\frac{7}{12}\) = 0
5x – 12y + 7 = 0

Question 4.
If P and q are the lengths of the perpen-diculars from the origin to the straight lines x sec α + y cosec α = a and x cos α – y sin α = a cos 2α, prove that 4p² + q² = a².
Solution:
Equation of AB is x sec α + y cosec α = a
\(\frac{x}{\cos \alpha}+\frac{y}{\sin \alpha}=a\)
x sin α + y cos α = a sin α cos α
x sin α + y cos α – a sin α cos α = 0
p = length of the perpendicular from O on AB

2p = asin 2α …………. (1)
Equation of CD is x cos α – y sin α = a cos 2α
x cos α – y sin α – a cos 2α = 0
q = Length of the perpendicular from O on CD
\(\frac{|0+0-a \cos 2 \alpha|}{\sqrt{\cos ^{2} \alpha+\sin ^{2} \alpha}}\) = cos2α ………. (2)
Squaring and adding (1) and (2)
4p² + q² = a² sin² 2α + a² cos² 2α
= a2 (sin² 2α + cos² 2α)
= a².1
= a²

Question 5.
Two adjacent sides of a parallelogram are given by 4x + 5y = 0 and 7x + 2y = 0 and one diagonal is 11x + 7y = 9. Find the equations of the remaining sides and the other diagonal.
Solution:
Let 4x + 5y = 0 ………… (1) and
7x + 2y – 0 ………… (2) respectively
denote the side \(\stackrel{\leftrightarrow}{O A}\) and \(\stackrel{\leftrightarrow}{O B}\) of the parallelo-gram OABC.
Equation of the diagonal \(\stackrel{\leftrightarrow}{A B}\) is 1 lx + 7y – 9 = 0 ………. (3)

Solving (1) and (2) vertex O = (0, 0)
Solving (1) and (3), we get
Solving (2) and (3), we get

Midpoint of AB is P (\(\frac{1}{2}\), \(\frac{1}{2}\)), Slope of OP is 1.
Equation to OC is y = (1)x = x – y = 0
x = y

Question 6.
Find the incenter of the triangle formed by the following straight line.
i) x + 1 = 0, 3x- 4y = 5 and 5x + 12y = 27
ii) x + y – 7 =0, x – y + 1 = 0 and x – 3y + 5 = 0
Solution:
i)

In ∆ ABC,
Equation of side AC is
x + 1 = 0 ……… (1)
Equation of side AB is
3x – 4y – 5 = 0 ……………. (2)
Equation of side BC is
5x + 12y – 27 = 0 ………… (3)
From (1), x = – 1
Substitute in (2) ⇒ 3 (-1) – 4y – 5 = 0
4y = -8
y = -2
The point of intersection of (1), (2) is A =(-1, -2)
By solving (2), (3)

The point of intersection of (2), (3) is B = (3, 1)
From (1) x = -1
Substitute in (3)
-5 + 12y – 27 =0
12y = 32
y = \(\frac{32}{12}=\frac{8}{3}\)
The point of intersection of (3), (1) is

Incentre = I =

ii)

In ∆ ABC,
Equation of AC is x + y – 7 = 0 ………… (1)
Equation of AB is x – y + 1 = 0 ………… (1)
Equation of BC is x – 3y + 5 = 0 ………… (1)
solving (1) (2) and (3)
verti and A (3, 4) B (1, 2) and C (4, 3)

Question 7.
A ∆^le is formed by the lines ax + by + c = 0, lx + my + n = 0 and px + qy + r = 0. Given that the ∆^le is not right angled, show that the straight line \(\frac{a x+b y+c}{a p+b Q}=\frac{l x+m y+n}{I p+m Q}\) passes through the orthocentre of the ∆^le.
Solution:
Given sides of triangle are

ax+by+c /x+mv+y
ax + by + c = 0 ………… (1)
lx + my + n = 0 ………… (2)
px + qy + r = 0 ………… (3)
Equation of line passing through intersecting points of (1), (2) is
ax + by + c + k (lx + my + n) = 0 ………… (4)
(a + ki) x + (b + km)y + (c + nk) = 0
It is ⊥^lr to (3), then
p(a + kl) + q(b + km) = 0
⇒ k = –\(\frac{a P+b Q}{l p+m Q}\)
Substitute in (4)

is the required straight line equation of AD This is the altitude passing throught A.

Passes through the othrocentre of the triangle.

Question 8.
The Cartesian equations of the sides \(\overrightarrow{B C}\) , \(\overrightarrow{C A}\) and \(\overrightarrow{A B}\) of a ∆^le are respectively u₁ = a₁x + b₁y + c1 = 0, r = 1, 2, 3. Show that the equation of the straight line passing through A and bisecting the side \(\overline{\mathrm{B C}}\) is \(\frac{u_{3}}{a_{3} b_{1}-a_{1} b_{3}}=\frac{u_{2}}{a_{3} b_{1}-a_{1} b_{3}}\)
Solution:
A is a point of intersection of the lines u₂ = 0 and u₃ = 0
∴ Equation to a line passing through A is
u₂ + λu₃ = 0 ⇒ (a₂x + b₂y + c₂) + λ(a₃x + b₃y + c₃) = 0 ………….. (1)
⇒ (a₂ + λa₃)x + (b₂ + λb₃) y + (c₂ + λc₃) = 0
If this is parallel to a₁x + b₁y + c₁ = 0, we get
\(-\frac{\left(a_{2}+\lambda a_{3}\right)}{\left(b_{2}+\lambda b_{3}\right)}=-\frac{a_{1}}{b_{1}}\)
⇒ (a₂ + λa₃) b₁ = (b₂ + λb₃) a₁
⇒ a₂b₁ + λa₃b₁ = a₁b₂ + λa₁b₃
⇒ λ(a₃b₁ – a₁b₃) = – (a₂b₁ – a₁b₂) .
⇒ λ = \(\frac{\left(a_{2} b_{1}-a_{1} b_{2}\right)}{a_{3} b_{1}-a_{1} b_{3}}\)
Substituting this value of X in (1), the required equation is
(a₂x + b₂y + c₂) – \(\frac{\left(a_{2} b_{1}-a_{1} b_{2}\right)}{\left(a_{3} b_{1}-a_{1} b_{3}\right)}\) (a₃x + b₃y + c₃) = 0
⇒ (a₃b₁ – a₁b₃) (a₂x + b₂y + c₂) – (a₂b₁ – a₁b₂) (a₃x + b₃y + c₃) = 0
⇒ (a₃b₁ – a₁b₃)u₂ – (a₂b₁ – a₁b₂) u₃ = 0
⇒ (a₃b₁ – a₁b₃)u₂ = (a₂b₁ – a₁b₂)u₃
⇒ \(\frac{u_{3}}{\left(a_{3} b_{1}-a_{1} b_{3}\right)}=\frac{u_{2}}{\left(a_{2} b_{1}-a_{1} b_{2}\right)}\)

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B The Straight Line Solutions Exercise 3(d) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B The Straight Line Solutions Exercise 3(d)

I. Find the angle between the following straight lines.

Question 1.
y = 4 – 2x, y = 3x + 7
Solution:
y = 4 – 2x ⇒ 2x + y – 4 = 0
3x – y + 7 = 0

Question 2.
3x + 5y = 7, 2x – y + 4 = 0
Solution:

Question 3.
y = – √3 + 5, y = \(\frac{1}{\sqrt{3}} x-\frac{2}{\sqrt{3}}\)
Solution:
m₁ = √3, m₂= \(\frac{1}{\sqrt{3}}\)
m₁m₂ = (-√3).\(\frac{1}{\sqrt{3}}\) = 1
The lines are perpendicular θ = \(\frac{\pi}{2}\)

Question 4.
ax + by = a + b, a(x-y) + b(x + y) = 2b
Solution:
ax + by = a + b, (a + b) x + (- a + b) y = 2b

Find the length of the perpendicular drawn from the point given against the following straight lines.

Question 5.
5x – 2y + 4 = 0, (-2, -3)
Solution:
Length of the perpendicular

Question 6.
3x – 4y + 10 = 0, (3, 4)
Solution:
Length of the perpendicular
\(=\frac{|3.3-4.4+10|}{\sqrt{9+16}}=\frac{3}{5}\)

Question 7.
x – 3y – 4 = 0, (0,0)
Solution:
Length of the perpendicular
\(=\frac{|0-0-4|}{\sqrt{1+9}}=\frac{4}{\sqrt{10}}\)

Find the distance between the following parallel lines.

Question 8.
3x – 4y = 12, 3x – 4y = 7
Solution:
Equation of the lines are
3x – 4y – 12 = 0
3x – 4y – 7 = 0

Distance between parallel lines
\(=\frac{\left|c_{1}-c_{2}\right|}{\sqrt{a^{2}+b^{2}}}=\frac{|-| 2+7 \mid}{\sqrt{9+16}}=\frac{5}{5}=1\)

Question 9.
5x – 3y – 4 = 0, 10x – 6y – 9 = 0
Solution:
Equations of the lines can be taken as
10x – 6y – 8 = 0
10x – 6y – 9 = 0

Distance between parallel lines
\(=\frac{|-8+9|}{\sqrt{100+36}}=\frac{1}{2 \sqrt{34}}\)

Question 10.
Find the equation of the straight line parallel to the line 2x + 3y 4- 7 = 0 and passing through the point (5, 4).
Solution:
Equation of the given line is 2x + 3y + 7 = 0
The required line is parallel to this line
Equation of the parallel line is 2x + 3y = k
This line passes through p (5, 4)
10 + 12 = k ⇒ k = 22
Equation of the required line is
2x + 3y = 22
2x + 3y — 22 = 0

Question 11.
Find the equation of the straight line per-pendicular to the line 5x – 3y + 1 = 0 and passing through the point (4, – 3).
Solution:
Equation of the given line is 5x – 3y + 1 = 0
Equation of the perpendicular line is 3x + 5y + k = 0
This line passes through P (4, -3)
12 – 15 + k = 0 ⇒ k = 3
Equation of the required line is
3x + 5y + 3 = 0

Question 12.
Find the value of k, if the straight lines 6x – 10y + 3 = 0 and kx – 5y + 8 = 0 are parallel.
Solution:
Equations of the given lines are
6x – 10y + 3 = 0
kx – 5y + 8 = 0
These lines are parallel
a₁b₂ = a₂b₁
-30 = -10 k
k = 3

Question 13.
Find the value of p, if the straight lines 3x 4- 7y – 1 = 0 and 7x – py + 3 = 0 are mutually perpendicular.
Solution:
Equations of the given lines are
3x + 7y – 1 = 0
7x – py + 3 = 0
These lines are perpendicular
⇒ a₁a₂ + b₁b₂ = 0
3.7 + 7(- p) = 0
7p = 21 ⇒ p = 3

Question 14.
Find the value of k, if the straight lines y – 3kx + 4 = 0 and (2k-1) x-(8k-1) y – 6 = 0 are perpendicular.
Solution:
Equations of the given lines are
-3kx + y + 4 = 0
(2k- 1)x- (8k- 1)y- 6 = 0
These lines are perpendicular
⇒ -3k(2k – 1) – 1(8k – 1) = 0
– 6k² +.3k – 8k +1 = 0
6k² + 5k – 1 = 0
(k + 1) (6k – 1) = 0
k = -1 or 1/6

Question 15.
(- 4,5) is a vertex of a square and one of its diagonal is 7x – y + 8 = 0. Find the equation of the other diagonal.

Solution:
ABCD is a square Equation of the diagonal AC is 7x – y + 8 = 0
The other diagonal BD is perpendicular to AC.
Equation of BD can be taken asx + 7y + k = 0
BD passes through D (- 4, 5)
-4 + 35 + k = 0 ⇒ k = 4-35 = -31
Equation of BD is x + 7y- 31 = 0

II.

Question 1.
Find the equations of the straight lines passing through (1, 3) and i) parallel to ii) perpendicular to the line passing through the points (3, -5) and (-6,1).

Solution:
A (3, -5), B(- 6, 1) are the given points
Slope of AB = \(\frac{-5-1}{3+6}=\frac{-6}{9}=\frac{-2}{3}\)

i) The required line is parallel to AB and passes through (1, 3)
Equation of the required line is
y – 3 = \(\frac{-2}{3}\)(x – 1)
3y – 9 = -2x + 2
2x + 3y – 11 = 0

ii) The required line AE is perpendicular to AD.
Equation of AE can be taken as 3x – 2y + k = 0
This line passes through A (1, 3)
3 – 6 + k = 0 ⇒ k = 6 – 3 = 3
Equation of AE is 3x – 2y + 3 = 0.

Question 2.
The line \(\frac{x}{a}-\frac{y}{b}\) = 1 meets the X-axis at P. Find the equation of the line perpen-dicular to this line at P.
Solution:

Equation of PQ is \(\frac{x}{a}-\frac{y}{b}\) = 1
Equation of X-axis is y = 0
\(\frac{x}{a}\) = 1 ⇒ x = a
Co-ordinates of P are (a, 0)
PR is perpendicular to PQ
Equation of PR = \(\frac{x}{b}+\frac{y}{a}\) = k
This line PR passes through P is (a, 0) a
\(\frac{a}{b}\) + 0 = k ⇒ k = a/b
Equation of PR is \(\frac{x}{b}+\frac{y}{a}=\frac{a}{b}\) = 1

Question 3.
Find the equation of the line perpendi-cular to the line 3x + 4y + 6 = 0 and making an intercept – 4 on X-axis.
Solution:
Equation of the given line is 3x + 4y + 6 = 0
Equation of the perpendicular line is
4x – 3y = k
\(\frac{4x}{k}-\frac{3y}{k}\) = 1
\(\frac{x}{\left(\frac{k}{4}\right)}+\frac{y}{\left(-\frac{k}{3}\right)}=1\)
x – intercept = \(\frac{k}{4}\) = – 4 ⇒ k = -16
Equation of the required line is 4x – 3y = -16
⇒ 4x – 3y + 16 = 0

Question 4.
A (-1,1), B (5, 3) are opposite vertices of a square in the XY plane. Find the equation of the other diagonal, (not passing through A, B) of the square.
Solution:
A (-1, 1), B (5, 3) are opposite vertices of the square.
Slope of AB \(\frac{1-3}{-1-5}=\frac{-2}{-6}=\frac{1}{3}\)

The other diagonal perpendicular to AB
Slope of CD = – \(\frac{1}{m}\) = -3 m
‘O’ is the point of intersection of the diagonals
Co-ordinates of O are\(\left(\frac{-1+5}{2}. \frac{1+3}{2}\right)\) = (2, 2)
CD passes through O (2, 2)
Equation of CD is y – 2 = -3 (x – 2)
= -3x + 6
3x + y – 8 = 0

Question 5.
Find the foot of the perpendicular drawn from (4, 1) upon the straight line 3x – 4y + 12 = 0.
Solution:
Equation of the line is 3x – 4y + 12 = 0
If (x₂, y₂) is the foot of the perpendicular from (x₁, y₁) on the line
ax + by + c = 0, then

Question 6.
Find the foot of the perpendicular drawn from (3, 0) upon the straight line 5x + 12y – 41 = 0.
Solution:
Equation of the line is 5x + 12y – 41 = 0
If (x₂, y₂) is the foot of the perpendicular from (x₁, y₁) on the line ax + by + c = 0,

Question 7.
x – 3y – 5 = 0 is the perpendicular bisector of the line segment joining the points A, B. If A = (-1, -3), find the co-ordinates of B.

Solution:
If PQ is the perpendicular bisector of AB, then B is the image of A in the line PQ.
Equation of PQ is x – 3y – 5 = 0

Co-ordinates of A are (-1, -3)
If (x₂, y₂) is the image of (x₁, y₁) about the line ax + by + c = 0, then

Question 8.
Find the image of the point (1, 2) in the straight line 3x + 4y – 1 = 0,
Solution:
Equation of the line is 3x + 4y – 1 = 0 ‘
If (x₂, y₂) is the image of (x₁, y₁) in the line ax + by + c = 0, then

Question 9.
Show that the distance of the point (6, – 2) from the line 4x + 3y = 12 is half the distance of the point (3,4) from the line 4x – 3y = 12.
Solution:

Equation of AB is 4x + 3y – 12 = 0
PQ = Length of the perpendicular from
\(P=\frac{|24-6-12|}{\sqrt{16+9}}=\frac{6}{5}\)
Equation of CD is 4x – 3y – 12 = 0
RS = Length of the perpendicular from
\(R=\frac{|12-12-12|}{\sqrt{16+9}}=\frac{12}{5}\)
∴ PQ = \(\frac{1}{2}\)RS

Question 10.
Find the locus of the foot of the perpendi¬cular from the origin to a variable straight line which always passes through a fixed point (a, b).

Solution:
Suppose m is the slope of the line AB
Equation of AB is y – b = m (x – a)
= mx – ma
mx – y + (b – ma) = 0 ……………. (1)

OK is perpendicular to A B and passes through the origin O. Suppose co-ordinates of K are (x, y)
Equation of AB is x + my – 0 ……………. (2)

m = -x/y
Substituting in (1)
\(-\frac{x^{2}}{y}-y+b+\frac{x}{y} \cdot a=0\).
– x² – y² + by + ax = 0
or x² + y² – ax – by = 0
Locus of K is x² + y² – ax – by = 0

III.

Question 1.
Show that the lines x – 7y – 22 = 0, 3x + 4y + 9 = 0 and 7x + y – 54 = 0 form a right angled isosceles triangle.
Solution:
Given lines x – 7y – 22 = 0 …………. (1)
3x + 4y + 9 = 0 …………. (2)
7x + y – 54 = 0 …………. (3)

Let ‘A’ be the angle between (1), (2)

Let B be the angle between (2), (3)

Let ‘C’ be the angle between (3), (1)

∴ Given lines form a right angled isosceles triangle.

Question 2.
Find the equation of the straight lines passing through the point (-3, 2) and making an angle of 45° with the straight line 3x – y + 4 = 0.
Solution:
Given point
P(x₁, y₁) = (-3, 2)
Given line 3x – y + 4 = 0 ………….. (1)
Slope = m = – \(\frac{a}{b}\) = 3

m – 3 = -1 + 3m
2m = – 4 or m = -2
\(\frac{m-3}{1+3 m}\) = -1 ⇒ m – 3 = -1 – 3m
4m = 2 ⇒ m = 1/2
Case (i) m = – 2
Equation of the line PQ is
y – 2 = -2(x+3)
= -2x – 6
2x + y + 4 = 0

Case (ii) m =\(\frac{1}{2}\)
Equation of the line PR is
y – 2 = \(\frac{1}{2}\) (x + 3)
2y – 4 = x + 3
x – 2y + 7 = 0

Question 3.
Find the angles of the triangle whose sides are x + y- 4 = 0,2x + y- 6 = 0, 5x + 3y -15 = 0.
Solution:
Equation of AB is x + y – 4 = 0
Equation of BC is 2x + y – 6 = 0
Equation of AC is 5x + 3y – 15 = 0

Question 4.
Proye that the feet of the perpendiculars from the origin on the lines x + y = 4, x + 5y = 26 and 15x – 27y = 424 are collinear.
Solution:
Given lines
x + y – 4 = 0 ……… (1)
x + 5y – 26 = 0 ……… (2)
15x – 27y – 424 = 0 ……… (3)

Let P(x₂, y₂) be the foot of the ⊥^le of (x₁, y₁) = (0, 0) on (1)

⇒ x₂ – 0 = 2, y₂ – 0 = 2
⇒ x₂ = 2, y₂ = 2
∴ P = (2, 2)
Let Q(x₃, y₃) be the foot of the ⊥^le of (x₁ y₁) = (0, 0) on (2)

x₃ = 1, y₃ = 5 ⇒ Q = (1, 5)
Let R(x₄, y₄) be the foot of the ⊥^le of (x₁, y₁) = (0, 0) on (3)

Equation of the line through P,Q is
\(\frac{x-2}{1-2}=\frac{y-2}{5-2}\) ⇒ 3x + y – 8 = 0 ………. (4)
Substitute R (x₄, y₄) in (4)
⇒ 3\(\frac{1060}{159}\) – 12 – 8
= 20 – 20 = 0
∴ Foot of perpendiculars of origin on the lines lies on a straight line.

Question 5.
Find the equations of the straight lines passing through the point of intersection of the lines 3x + 2y + 4 = 0,2x + 5y = 1 and whose distance from (2, -1) is 2.
Solution:
Equations of the lines passing through the point of intersection of the line
L₁ ≡ 3x + 2y + 4 = 0, L₃ ≡ 2x + 5y – 1 = 0 is
L₁ + λL₂ = 0
(3x + 2y + 4) + λ (2x + 5y- 1) = 0
(3 + 2λ)x + (2 + 5λ) y + (4 – λ) = 0 ………….. (1)
Given distance from (2, -1) to (1) = 2

⇒ (-λ + 4)2 = 9 + 4λ2 + 12λ + 4 + 25λ2 + 20λ
⇒ 28λ2 + 40λ – 3 = 0
⇒ 28λ2 – 2λ + 42λ – 3 = 0
⇒ (2λ + 3) (14λ – 1) = 0
⇒ λ = \(\frac{1}{14}\), λ = – \(\frac{3}{2}\)
From (1)
If λ = \(\frac{1}{14}\) ⇒ 4x + 3y + 5 = 0
If λ = – \(\frac{3}{2}\)
⇒ y – 1 = 0 are the required line equations.

Question 6.
Each side of a square is of length 4 units. The centre of the square is (3, 7) and one of its diagonals is parallel to y = x. Find the co-ordinates of its vertices.
Solution:
Let ABCD be the square.
Point of intersection of the diagonals is the centre P(3, 7)

From P draw PM⊥AB. Then M is midpoint ofAB
∴ AM = MB = PM = 2
Since a diagonal is parallel to y = x, its sides are parallel to the co-ordinate axes.
M(3, 5) ⇒ A(1, 5), B(5, 5), C(5, 9), D(1, 9)

Question 7.
If ab > 0, find the area of the rhombus enclosed by the four straight lines ax ± by ± c = 0.
Solution:
Equation of AB is ax + by + c = 0 ………….. (1)
Equation of CD is ax + by – c = 0 ………….. (2)
Equation of BC is ax – by + c = 0 ………….. (3)
Equation of AD is ax – by – c = 0 ………….. (4)

Solving (1) and (3), co-ordinates of B are (-\(\frac{c}{a}\) 0)
Solving (1) and (4) co-ordinates of A are (0, –\(\frac{c}{b}\)
Solving (2) and (3) co-ordinates of C are (0, \(\frac{c}{b}\)
Solving (2) and (4) co-ordinates of D are (\(\frac{c}{a}\), 0)
Area of rhombus ABCD = \(\frac{1}{2}\)|∑x₁ (y₂ – y₄)|

Question 8.
Find the area of the parallelogram whose sides are 3x + 4y + 5 = 0, 3x + 4y – 2 = 0, 2x + 3y + 1 = 0 and 2x + 3y – 7 = 0
Solution:
Given sides are 3x + 4y + 5 = 0 ……….. (1)
3x + 4y – 2 = 0 ……….. (2)
2x + 3y + 1 = 0 ……….. (3)
2x + 3y – 7 = 0 ……….. (4)

Area of parallelogram formed by (1), (2), (3), (4)

Question 9.
A person standing at the junction (crossing) of two straight paths represented by the equations 2x – 3y + 4 = 0 and 3x + 4y – 5 = 0 wants to reach the path whose equation is 6x – 7y + 8 = 0 in the least time. Find the equation of the path the he should follow.
Solution:
By solving
2x – 3y + 4 = 0
3 x + 4y – 5 = 0

Given line 6x – 7y + 8 = 0
Required line is 7x + 6y + k = 0 …….. (1)

From (1) 7x + 6y – \(\frac{125}{17}\)
119x + 102y – 125 = 0

Question 10.
A ray of light passing through the point (1, 2) reflects on the X – axis at a point A and the reflected ray passes through the point (5, 3). Find the coordinates of A.
Solution:
Let m be the slope then equation of line passing through (1, 2).
y – 2 = m(x – 1)
\(\frac{y-2}{x-1}=m\)
Let -m be the slope the equation of line passing through (5, 3)
y – 3 = -m(x – 5)

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B The Straight Line Solutions Exercise 3(c) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B The Straight Line Solutions Exercise 3(c)

I.

Question 1.
Find the ratio in which the following straight lines divide the line segment joining the given points. State whether the points lie on the same side or on either side of the straight line, i) 3x – 4y = 7, (2, -7) and (-1, 3)
Solution:
3x – 4y – 7= 0

L₁₁, L₂₂ are of opposite signs. The given points lie on opposite sides of the line.

ii) 3x + 4y = 6, (2, -1) and (1, 1)
Solution:
Equation of the line is 3x + 4y – 6 = 0

The points lie on the opposite side of the line.

iii) 2x + 3y = 5, (0, 0) and (-2, 1)
Solution:
\(\frac{I}{m}=\frac{-(0+0-5)}{-4+3-5}\)
\(\frac{-5}{6}\)
The points lie on same side of the line.

Question 2.
Find,the point of intersection of the follow- ing lines.
i) 4x + 8y – 1 = 0, 2x – y + 1 = 0
Solution:
4x + 8y – 1 = 0, 2x – y + 1 = 0
point of intersection is

ii) 7x + y + 3 = 0, x + y = 0
Solution:
7x + y+ 3 = 0, x + y = 0 x y 1

Question 3.
Show that the straight lines (a – b) x + (b – c) y = c – a, (b – c)x + (c – a) y = (a – b) and (c – a)x + (a – b) y = b – c are concurrent.
Solution:
Equations of the given lines are
(a – b) x + (b – c) y = c – a …………… (1)
(b – c) x +(c – a) y = a – b …………… (2)
(c – a) x + (a – b) y = b – c …………… (3)
From (1) and (2)


Point of intersection of (1) and (2) is P (-1, -1)
Substituting in (3)
(c – a) (-1) + (a – b) (-1) = -c + a – a + b = b – c
∴ P (-1, -1) is a point on (3)
The given lines are concurrent.

Question 4.
Transform the following equation into the form L₁ + λL₂ = 0 and find the point of concurrency of the family of straight lines represented by the equation.
i) (2 + 5k) x – 3(1 + 2k)y + (2 – k) = 0
Solution:
(2 + 5k)x – 3(1 + 2k)y + (2 – k) = 0
(2x – 3y + 2) + k (5x – 6y – 1) = 0
This is of the form L₁ + λL₂ = 0
L₁ = 2x – 3y + 2 = 0
L₂ = 5x – 6y – 1 = 0

The point of concurrency is P (5, 4).

ii) (k + 1) x + (k + 2) y + 5 = 0
Solution:
(k + 1) x + (k + 2) y + 5 = 0
k (x + y) + (x + 2y + 5) = 0
i.e., (x + 2y + 5) + k (x + y) = 0
This is of the form L₁ + λL₂ = 0
∴ L₁ = x + 2y + 5 = 0
L₂ = x + y = 0

Point of concurrency is P (5, – 5).

Question 5.
Find the value of P, If the straight lines. x + p = 0, y + 2 = 0 and 3x + 2y + 5 = 0 are concurrent.
Solution:
Equations of the given lines are
x + p = 0 …………. (1)
y + 2 = 0 …………. (2)
3x + 2y + 5 = 0 …………. (3)
From (2), y = -2
Substituting in (3)
3x – 4 + 5 = 0
3x = 4 – 5 = -1
x = –\(\frac{1}{3}\)
Point of intersection of (2) and (3) is P (-\(\frac{1}{3}\), – 2)
The given lines are concurrent
P is a point on x + p = 0
–\(\frac{1}{3}\) + p = o ⇒ p = \(\frac{1}{3}\)

Question 6.
Find the area of the triangle formed by the following straight lines and the coordinate axes,
i) x – 4y + 2 = 0
Solution:
Equation of AB is
x – 4y + 2 = 0
– x + 4y = 2
\(\frac{x}{-2}+\frac{y}{\left(\frac{1}{2}\right)}=1\)
a = -2 b = \(\frac{1}{2}\)
Area of ∆OAB = \(\frac{1}{2}\)|ab|
= \(\frac{1}{2}\)|-2 × \(\frac{1}{2}\)| = \(\frac{1}{2}\) sq.units

ii) 3x – 4y + 12 = 0
Solution:
Equation of AB is
3x – 4y + 12 = 0
– 3x + 4y = 12
\(\frac{x}{-4}+\frac{y}{3}\) = 1
Area of ∆OAB = \(\frac{1}{2}\)|ab|
= \(\frac{1}{2}\)|(-4) (3)| = \(\frac{1}{2}\)(12)
= 6 sq. units

II.

Question 1.
A straight line meets the co-ordinate axes at A and B. Find the equation of the straight line, when
i) \(\overline{\mathrm{AB}}\) is divided in the ratio 2:3 at (-5, 2).

Solution:
Let OA = a and OB = b
Co-ordinates of A are (a, 0) and B are (0, b)
P divides AB in the ratio 2:3

-3x + 5y = 25
3x – 5y + 25 = 0

ii) \(\overline{\mathrm{AB}}\) is divided in the ratio 1:2 at (-5,4)
Solution:
Let OA = a and OB = b
Co-ordinates of A are (a, 0) and B are (0, b)
P divides AB in the ratio 1 : 2

– 8x + 5y = 60
8x – 5y + 60 = 0

iii) (p, q) bisects \(\overline{\mathrm{AB}}\)
Solution:
Let OA a and OB = b
Co-ordinates of A are (a, 0) and B are (0, b)

Question 2.
Find the equation of the straight line passing through the points (-1, 2) and (5, -1) and also find the area of the triangle formed by it with the axes of coordinates.
Solution:
P (-1, 2) and Q (5, -1) are the given points. Equation of
PQ is (y – y₁) (x₁ – x₂) = (x – x₁) (y₁ – y₂)
(y – 2) (-1 -5) = (x + 1) (2 + 1)
-6 (y – 2) = 3 (x+ 1)
-2y + 4 = x+ 1
x + 2y – 3 = 0

Question 3.
A triangle of area 24 sq. units is formed by a straight line and the co-ordinate axes is the first quadrant. Find the equation of the straight line, if it passes through (3, 4).

Solution:
Equation of AB in the
intercept form is \(\frac{x}{a}+\frac{y}{b}\) = 1
This line passes through P (3, 4)

Area of ∆ OAB = 24 ⇒ \(\frac{1}{2}\) |ab|= 24
\(\frac{1}{2} \frac{4 a^{2}}{a-3}=24\)
a² = 12 (a – 3)
= 12a – 36
a² – 12a + 36 = 0
(a – 6)² = 0 ⇒ a = 6
b = \(\frac{4a}{a-3}=\frac{24}{3}\) = 8
Equation of AB is \(\frac{x}{6}+\frac{y}{8}\) = 1
4x + 3y = 24
4x + 3y – 24 = 0

Question 4.
A straight line with slope 1 passes through Q(-3, 5) and meets the straight line x + y – 6 = 0 at P. Find the distance PQ.

Solution:
Given slope = 1
tan α = 1 = tan 45°
α = 45°
The line passes through Q (-3, 5)
Co-ordinates of P are (x₁ + r cos α, y₁ + r sin α)
= (-3 + r cos 45°, 5 + r sin 45°)

PQ = 2√2

Question 5.
Find the set of values of ‘a’ if the points (1, 2) and (3, 4) lie to the same side of the straight line 3x – 5y + a = 0
Solution:
P (1, 2), Q (3, 4) are the given points.
Equation of the given line is 3x – 5y + a = 0
L₁₁ = 3.1 – 5.2 + a = a – 7
L₂₂ = 3.3 – 5.4 + a = a – 11
a – 7 and a – 11 must both be positive or both negative.

Case (i) :
a – 7 > 0, a – 11 > 0
a > 7 and a > 11
∴ a > 7.11 ⇒ a∈ (11, α)

Case (ii) :
a – 7 < 0, a – 17 < 0
a < 7, a < 17
⇒ a < 17, ⇒ a∈ (-α, 27)
∴ a ∈ (-α, 7) U (11, α)

Question 6.
Show that the lines 2x + y – 3 = 0,
3x + 2y – 2 = 0 and 2x – 3y – 23 = 0 are concurrent and find the point of concurrency.
Solution:
Equations of the given lines are
2x + y – 3 = 0 ……………. (1)
3x + 2y – 2 = 0 ……………. (2)
2x – 3y – 23 = 0 ……………. (3)

From (1) and (2)

x = 4, y = – 5
Co-ordinates of P are (4, -5)
2x – 3y – 23 = 2(4) – 3(-5) – 23
= 8 + 15 – 23 = 0
P lies on (3)
The given lines are concurrent Point of concurrency is P (4, -5)

Question 7.
Find the value of p, if the following lines are concurrent.
(i) 3x + 4y = 5, 2x + 3y = 4, px + 4y = 6
(ii) 4x – 3y – 7 = 0, 2x + py + 2 = 0, 6x + 5y – 1 = 0
Solution:
(i) Equations of the lines are
3x + 4y – 5 = 0
2x + 3y – 4 = 0

x = -1, y = 2

Point of intersection of (1) and (2) is P (-1, 2)
The given lines are concurrent.
P lies on px + 4y = 6
-p + 8 = 6 ⇒ p = 8- 6 = 2

(ii) Equations of the lines
4x – 3y – 7 = 0
6x + 5y- 1 = 0

x = 1, y = -1
Co-ordinates of P are (1, -1)
The given lines are concurrent.
P is a point on
2x + py + 2 = 0
2 – p + 2 = 0
p = 4

Question 8.
Determine whether or not the four straight lines with equations
x + 2y – 3 = 0, 3x + 4y – 7 = 0,
2x + 3y – 4 = 0 and 4x + 5y – 6 = 0 are concurrent.
Solution:
Equations of the given lines are
x + 2y – 3 = 0 ………. (1)
3x + 4y – 7 = 0 ………. (2)
2x + 3y – 4 = 0 ………. (3)
4x + 5y – 6 = 0 ………. (4)
Solving (1) and (2)

x = 1, y = 1
Point of intersection of (1) and (2) is P (1, 1)
2x + 3y – 4 = 2.1 + 3.1 – 4 = 5 – 4 = 1 ≠ 0
4x + 5y- 6 = 4.1 + 5.1 – 6 = 9 – 6 = 3 ≠ 0
∴ P (1,1) is not a point on (3) and (4)
∴ The given lines are not concurrent.

Question 9.
If 3a + 2b + 4c = 0, then show that the equation ax + by + c = 0 represents a family of concurrent straight lines and find the point of concurrency.
Solution:
Given condition is 3a + 2b + 4c = 0
\(\frac{3}{4}\)a + \(\frac{1}{2}\)b + c = 0
For all values of a, b the line ax + by + c = 0
passes through the point (\(\frac{3}{4}\), \(\frac{1}{2}\))
The equation ax + by + c = 0 represents a family of concurrent lines.
Point of concurrency is (\(\frac{3}{4}\), \(\frac{1}{2}\))

Question 10.
If non-zero numbers a, b, c are in harmonic progression then show that the equation \(\frac{x}{a}+\frac{y}{b}+\frac{1}{c}\) = o represents a family of concurrent lines and find the point of concurrency.
Solution:
Given a, b, c are in H.P.
∴ \(\frac{2}{b}=\frac{1}{a}+\frac{1}{c}\)
\(\frac{1}{a}+\frac{(-2)}{b}+\frac{1}{c}\) = o
∴ For all values of a,b,c the equation \(\frac{x}{a}+\frac{y}{b}+\frac{1}{c}\) = o
represents a line passing through the point (1, -2)
∴ The equation, \(\frac{x}{a}+\frac{y}{b}+\frac{1}{c}\) = o represents a family of concurrent lines.
Point of concurrency is P (1, – 2)

III.

Question 1.
Find the point on the straight lines
3x + y 4- 4 = 0 which is equidistant from the points (-5, 6) and (3, 2).
Solution:
P(x₁, y₁) is the point on 3x + y + 4 = 0
3x₁ + y₁ + 4 = 0 …………. (1)
Given PA = PB ⇒ PA² = PB²

(x₁+ 5)² + (y₁ – 6)² = (x₁ – 3)² + (y₁ – 2)²
x²₁ + 10x₁ + 25 + y²₁ – 12y₁ + 36 = x²₁ -6x₁ + 9 + y²₁ – 4y₁ + 4
16x₁ – 8y₁ + 48 = 0
2x₁ – y₁+ 6 = 0 ……….. (2)
3x₁ + y₁ +4 = 0 ………… (1)
Adding 5x₁ + 10 = 0 ⇒ x₁ = – 2
From (1) – 6 + y₁ + 4 = 0
y₁ = 6 – 4 = 2
Co-ordinates of P are (-2, 2)

Question 2.
A straight line through P (3, 4) makes an angle of 60° with the positive direction of the X-axes. Find the co-ordinates of the points on that line which are 5 units away from P.
Solution:
Co-ordinates of any point on the line Q are (x₁ + r cos θ, y₁ + r sin θ)
Given (x₁, y₁) = (3, 4) i.e., x₁ = 3, y₁ = 4
θ = 60° ⇒ cos θ = cos 60° = \(\frac{1}{2}\), sin θ = sin 60° \(\frac{\sqrt{3}}{2}\)

Question 3.
A straight line through Q (√3, 2) makes an angle \(\frac{\pi}{6}\) with the positive direction of the X-axis. If the straight line intersects the line √3x – 4y + 8 = 0 at P; find the distance of PQ.
Note : AB and PQ are not perpendicular.
So we have to follow the first method only.
Solution:

PQ makes an angle \(\frac{\pi}{6}\) with the positive direction of X – axis.
m = Slope of PQ = tan 30° = \(\frac{1}{\sqrt{3}}\)
PQ passes through Q (√3, 2)
Equation of PQ is y – 2 = \(\frac{1}{\sqrt{3}}\)(x – √3)
√3y – 2√3 = x – √3
x – √3y = – √3 ………… (1)
Equation of AB is √3x – 4y + 8 = 0
√3x – 4y = -8 ……………. (2)
(1) × √3 ⇒ √3x – 3y = -3
Subtracting -y = -5
y = 5
From (1), x = √3y – √3
= 5√3 – √3 = 4√3
Equation of AB is
Co-ordinates of P are ( 4√3, 5)
Q are (√3, 2)
PQ²= (4√3 – √3)² +(5 – 2)²
= 27 + 9 = 36
PQ =6 units.

Question 4.
Show that the origin is with in the triangle whose angular points are (2,1), (3,-2) and (-4,-1).
Solution:
The vertices of the ∆ le ABC are
A = (2, 1), B = (3, -2), C = (-4, -1)

The equation of BC is

⇒ 7y + 7 = -x -4
L = x + 7y + 11 = 0 …………. (1)
The equation of CA is

⇒ 3y + 3 = x + 4
⇒ L’ = x – 3y + 1 = 0 ………. (2)

⇒ 3x – 6 = -y + 1
L” = – 3x + y – 7 = 0 …………… (3)
L” (- 4, -1)= 3 (- 4) – 1 – 7
= – 20 is negative.
L” (0,0) = 3(0) + 0 – 7
= – 7 is negative
So (- 4, -1), (0,0) lie on the same side of AB
Hence O (0,0) lies to the left of AB ………… (4)
L’ (3, -2) = 3 – 3 (- 2) + 1
= 10 is positive

L” (0, 0) =0 – 3 (0) + 1
= 1 is positive
So (0, 0), (3, -2) lie on the same side of AC
both down of AC ………… (5)
L (2, 1) = 2 + 7(1) + 11
= 20 is positive

L (0, 0) =0 + 7(0)+ 11
= 11 is positive
So (0, 0) and (2, 1) lie on the same side of BC.
So (0, 0) lie upwards from BC ……… (6)

From (4), (5), (6) it follows O (0,0) lies down-wards of AC, upwards of BC, and to the left of AB. So O (0, 0) will lie inside the ∆ ABC.

Question 5.
A straight line through Q(2, 3) makes an angle \(\frac{3 \pi}{4}\) with negative direction of the X – axis. If the straight line intersects the line x+ y – 7 = 0 at P, find the distance PQ.

Solution:
The line PQ makes an angle \(\frac{3 \pi}{4}\) with the negative direction of X – axis i.e., PQ makes and angle π – \(\frac{3 \pi}{4}\) = \(\frac{\pi}{4}\) with the positive direction of X – axis.
Co-ordinates of Q are (2, 3)
Co-ordinates of P are (x₁ + r cos θ, y₁ + r sin θ)

PQ = r = √2 units.

Question 6.
Show that the straight lines x + y = 0, 3x + y – 4 = 0 and x + 3y – 4 = 0 form an isosceles triangle.
Solution:
Given lines
x + y = 0 ………….. (1)
3x + y – 4 = 0 ………….. (2)
x + 3y – 4 = 0 ………….. (3)
Let θ₁ be the angle between (1), (2)

Let θ₂ be the angle between (2), (3)

Let 0, be the angle between (3), (1)

Since θ₁ = θ₃
∴ The ∆^le formed by the lines is an Isosceles triangle.

Question 7.
Find the area of the triangle formed by the straight lines 2x – y – 5 = 0, x – 5y + 11 = 0 and x + y – 1 = 0.
Solution:
Given lines
2x – y – 5 = 0 ………….. (1)
x – 5y + 11 =0 …………. (2)
x + y – 1 = 0 ………….. (3)
By solving (1), (2)

Let A = (4, 3)
By solving (2), (3)

∴ B = (- 1,2)
By solving (3), (1)

∴ C = (2, -1)

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B The Straight Line Solutions Exercise 3(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B The Straight Line Solutions Exercise 3(b)

I.

Question 1.
Find the sum of the squares of the inter¬cepts of the line 4x – 3y = 12 on the axes, of co-ordinates.
Solution:
Equation of the given line is
\(\frac{4 x}{12}-\frac{3 y}{12}=1\)
\(\frac{x}{3}+\frac{y}{-4}=1\)
a = 3, b = -4
Sum of the squares = a² + b²
= 9 + 16 = 25

Question 2.
If the portion of a straight line inter¬cepted between the axes of co-ordinates is bisected at (2p, 2q), write the equation of the straight line.
Solution:
Equation of AB in the intercept form is
\(\frac{x}{a}+\frac{y}{b}=1\) …………… (1)

Co-ordinates of A are (a, 0) and B are (0, b)
M is the mid-point of AB
Co-ordinates of M are (\(\frac{a}{2}\), \(\frac{b}{2}\)) = (2p, 2q)
\(\frac{a}{2}\) = 2p, \(\frac{b}{2}\) = 2q
a = 4p, b = 4q
Substituting in (1), equation of AB is
\(\frac{x}{4 P}+\frac{y}{4 Q}=1\)
\(\frac{x}{P}+\frac{y}{Q}=4\)

Question 3.
If the linear equation ax + by + c = 0
(a,b,c ≠ 0) and lx + my + n = 0
represent the same line and r = \(\frac{l}{a}\) = \(\frac{n}{c}\)
write the value of r in terms of m and b.
Solution:
ax + by + c — 0 and
lx + my + n = 0 represent the same line
∴ \(\frac{1}{a}=\frac{m}{b}=\frac{n}{c}=r\)
\(\frac{m}{b}\) = r

Question 4.
Find the angle made by the straight line y = – √3x + 3 with the positive direction of the X-axis mea-sured in the counter clock-wise direction.
Solution:
Equation of the given line isy = -√3x + 3 Suppose a is the angle made by this line with positive X – axis in the counter clock – wise direction.
tan α = – √3 = tan \(\frac{2 \pi}{3}\)
α = \(\frac{2 \pi}{3}\)

Question 5.
The intercepts of a straight line on the axes of co-ordinates are a and b. If p is the length of the perpendicular drawn from the origin to this line. Write the value of p in terms of a and b.
Solution:
Equation of the line in the intercept form is

p = length of the perpendicular from origin

II.

Question 1.
In what follows, p denotes the distance of the straight line from the origin and a denotes the angle made by the normal ray drawn from the origin to the straight line with \(\stackrel{\leftrightarrow}{O X}\) measured in the anticlockwise sense. Find the equations of the straight lines with the following values of p and a.
i) p = 5, a = 60°
ii) p = 6, a = 150°
iii) p = 1, α = \(\frac{7 \pi}{4}\)
iv) p = 4, α = 90°
v) p = 0, α = 0
vi) p = 2√2, α = \(\frac{5 \pi}{4}\)
Solution:
Equation of the line in the normal form is x cos α + y sin α = p

i) p = 5, α = 60°
cos α = cos 60° = \(\frac{1}{2}\)
sin α = sin 60° = \(\frac{\sqrt{3}}{2}\)
Equation of the line is x. \(\frac{1}{2}\) + y. \(\frac{\sqrt{3}}{2}\) = 5
⇒ x + √3y= 10

ii) p = 6, α = 150°
cos α = cos 150° = cos (180° – 30°)
= -cos 30° = – \(\frac{\sqrt{3}}{2}\)
sin α = sin 150°
= sin (180° – 30°)
= sin 30° = \(\frac{1}{2}\)
Equation of the line is
x.(-\(\frac{\sqrt{3}}{2}\)) + y.\(\frac{1}{2}\) = 6
-√3x + y = 12
or √3x – y + 12 = 0

iii) p = 1, α = \(\frac{7 \pi}{4}\)
cos α = cos 315° = cos (360° – 45°)
= cos 45° = \(\frac{1}{\sqrt{2}}\)
sin α = sin 315° = sin (360° – 45°)
= -sin45° = \(\frac{1}{\sqrt{2}}\)
Equation of the line is
x.\(\frac{1}{\sqrt{2}}\) – y.\(\frac{1}{\sqrt{2}}\) = 1
x – y = √2
x – y – √2 = 0

iv) p = 4, α = 90°
cos α = cos 90° = 0, sin α = sin 90° = 1
Equation of the line is
x.0 + y.1 = 4
y = 4

v) p = 0, α = 0
cos α = cos 0 = 1, sin α = sin 0 = 0
Equation of the line is
x.1 + y.0 = 0
x = 0

vi) p = 2√2 , α = \(\frac{5 \pi}{4}\)
cos α = cos 225° = cos (180° + 45°)
= -cos 45° = – \(\frac{1}{\sqrt{2}}\)
sin α = sin 225° = sin (180° + 45°)
=-sin 45° = – \(\frac{1}{\sqrt{2}}\)
Equation of the line is
x(-\(\frac{1}{\sqrt{2}}\)) + y(-\(\frac{1}{\sqrt{2}}\)) = 2√2
– x – y = 4
or x + y + 4 = 0

Question 2.
Find the equations of the straight line in the symmetric form, given the slope and a point on the line in each part of the question.
i) √3, (2, 3)
ii) –\(\frac{1}{\sqrt{3}}\), (-2, 0)
iii) -1, (1,1)
Solution:
i) Equation of the line in the symmetric form is
\(\frac{x-x_{1}}{\cos \alpha}=\frac{y-y_{1}}{\sin \alpha}=r\)
(x₁, y₁) = (2, 3)
m = tan α = √3 ⇒ α = 60°
cos α = cos 60° = \(\frac{1}{2}\)
sin α = sm 60° = \(\frac{\sqrt{3}}{2}\)
Equation of the line in symmetric form is
\(\frac{x-2}{\cos \frac{\pi}{3}}=\frac{y-3}{\sin \frac{\pi}{3}}\)

ii) (x₁, y₁) = (-2, 0)
tan α = –\(\frac{1}{\sqrt{3}}\) ⇒ α = 180° – 30° = 150°
Equation of the line is \(\frac{x+2}{\cos 150^{\circ}}=\frac{y}{\sin 150^{\circ}}\)

iii) tan α = -1, α =180°- 45° = 135°
(x₁, y₁) = (1, 1)
Equation of the line is \(\frac{x-1}{\cos \left(\frac{3 \pi}{4}\right)}=\frac{y-1}{\sin \left(\frac{3 \pi}{4}\right)}\)

Question 3.
Transform the following equation into
a) Slope-intercept form
b) Intercept form and
c) Normal form
i) 3x + 4y = 5
ii) 4x – 3y + 12 = 0
iii) √3x + y = 4
iv) x + y + 2 = 0
v) x + y – 2 = 0
vi) √3x + y + 10 = 0
Solution:
i) 3x + 4y = 5
Slope-intercept form
4y = -3x + 5
\(y=\left(-\frac{3}{4}\right) x+\left(\frac{5}{4}\right)\)
Intercept form :
3x + 4y = 5

x cos α + y sin α = 1

ii) 4x – 3y + 12 = 0
Slope-intercept form :
3y = 4x + 12
y = (\(\frac{4}{3}\))x + 4
Intercept form:
4x – 3y+ 12 = 0
-4x + 3y = 12
\(\frac{-4 x}{12}+\frac{3 y}{12}=1\)
\(\frac{x}{(-3)}+\frac{y}{4}=1\)
Normal form :
4x – 3y + 12 = 0
– 4x + 3y = 12

x cos α + y sin α = \(\frac{12}{5}\)

iii) √3x + y = 4
Slope-intercept form :
√3x + y = 4
y = -√3x + 4
Intercept form :
√3x + y = 4

iv) x + y + 2 = 0
Slope-intercept form
x + y+.2 = 0
y = -x – 2
= (-1)x + (-2)

Intercept form:
x + y + 2 = 0
-x – y = 2
\(\frac{x}{(-2)}+\frac{y}{(-2)}=1\)
Normal form:
x + y + 2 = 0
-x – y = 2

v) x + y – 2 = 0
Slope-intercept form :
x + y – 2 = 0
y = – x + 2
Intercept form:
x + y – 2 = 0
x + y = 2
\(\frac{x}{2}+\frac{y}{2}\) = 1
Normal form :
x + y-2 = 0
x + y = 2

vi) √3x + y + 10 = 0
Slope-intercept form :
√3x + y + 10 = 0
y = -√3x – 10
Intercept form :
√3x + y = -10
Normal form :
√3x + y = -10
Dividing with \(\sqrt{3+1}=2\), we get
\(\frac{-\sqrt{3}}{2} \cdot x+\frac{-1}{2} \cdot y=5\)
x cos 30° + y sin 30° = 5

Question 4.
If the product of the intercepts made by the straight line x tan α + y sec α = 1 (0 ≤ α < \(\frac{\pi}{2}\)), on the co-ordinate axes is equal to sin α, find α.
Solution:
Equation of the line is x tan α + y sec α = 1
\(\frac{x}{\cot \alpha}+\frac{y}{\cos \alpha}=1\)
a = cot α, b = cos α
Given ab = sin α
cot α. cos α = sin α
\(\frac{\cos ^{2} \alpha}{\sin \alpha}\) = sin α ⇒ cos² α = sin α
tan² α = 1 ⇒ tan α = ±1
α = 45°

Question 5.
If the sum of the reciprocals of the intercepts made by a variable straight line on the axes of co-ordinate is a constant, then prove that the line always passes through a fixed point.
Solution:
Equation of the line in the intercept form is
\(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}=1\) ………….. (1)
Sum of the reciprocals of the intercepts

The line (1) passes through the fixed point
\(\left(\frac{1}{k}, \frac{1}{k}, \frac{1}{k}\right)\)

Question 6.
Line L has intercepts a and b on the axes of coordinates. When the axes are rota¬ted through a given angle, keeping the origin fixed, the same line L has intercepts p and q on the transformed axes. Prove that \(\frac{1}{a^{2}}+\frac{1}{b^{2}}=\frac{1}{P^{2}}+\frac{1}{Q^{2}}\).
Solution:
Equation of the line in the old system in the intercept form is
\(\frac{x}{a}+\frac{y}{b}=1 \Rightarrow \frac{x}{a}+\frac{y}{b}-1=0\)
Length of the perpendicular from origin

Equation of the line in the second system in the intercept form is
\(\frac{x}{p}+\frac{y}{Q}=1 \Rightarrow \frac{x}{p}+\frac{y}{Q}-1=0\)
Length of the perpendicular from origin

Since the origin and the given line remain unchanged we have from (1) and (2)

Question 7.
Transform the equation \(\frac{x}{a}+\frac{y}{b}=1\) into the normal form when a > 0 and b > 0. If the perpendicular distance of the straight line from the origin is p, deduce that \(\frac{1}{p^{2}}=\frac{1}{a^{2}}+\frac{1}{b^{2}}\).
Solution:

III.

Question 1.
A straight line passing through A(-2, 1) makes an angle 30° with \(\overrightarrow{O X}\) in the positive direction. Find the points on the straight line whose distance from A is 4 units.
Solution:
Co-ordinates of any point on the given line are (x₁ + r cos α, y₁ + r sin α)
α = 30° ⇒ cos α = cos 30° = \(\frac{\sqrt{3}}{2}\),
sin α = sin = 30° = \(\frac{1}{2}\)
(x₁, y₁) = (-2, 1) ⇒ x₁ = -2 y₁ = 1
Taking r = 4 ⇒ co-ordinates of P are

Question 2.
Find the points on the line 3x – 4y – 1 = 0 which are at a distance of 5 units from the point (3, 2).
Solution:
Equation of the line in the symmetric form is
\(\frac{x-3}{\cos \alpha}=\frac{y-2}{\sin \alpha}=r\)
Co-ordinates of the point P are
(3 + r cos α, 2 + r sin α) = (3 + 5 cos α, 2 + 5 sin α)
P is a point on 3x – 4y – 1 = 0
3(3 + 5 cos α) – 4(2 + 5 sin α) -1 = 0
9 + 15 cos α – 8 – 20 sin α – 1 = 0
15 cos α – 20 sin α = 0
15 cos α = + 20 sin α
tan α = + T
Case i) : cos α = +\(\frac{4}{5}\), sin α \(\frac{3}{5}\)
Case ii) : cos α = –\(\frac{4}{5}\), sin α \(\frac{3}{5}\)

Case i) : Co-ordinates of P are
\(\left(3+5 \cdot \frac{4}{5}, 2+5 \cdot \frac{3}{5}\right)=(7,5)\)

Case ii) : Co-ordinates of P are
\(\left(3-5 \cdot \frac{4}{5}, 2-5 \cdot \frac{3}{5}\right)=(-1,-1)\)

Question 3.
A straight line whose inclination with the positive direction of the X-axis measured in the antidock wise sense is it/3 makes positive intercept on the Y-axis. If the straight line is at a distance of 4 from the origin, find its equation.
Solution:
Given α = π/3, p = 4
m = tan α = tan 60° = √3
Equation of the line in the slope – intercept form is
y = √3 x + c
√3x – y + c = 0
Distance from the origin = 4
\(\frac{|0-0+c|}{\sqrt{3+1}}=4\)
|c| = 4 × 2 = 8
c = ± 8
Given c > 0 ∴ c = 8
Equation of the line is √3x – y + 8 = 0

Question 4.
A straight line L is drawn through the point A (2, 1) such that its point of intersection with the straight line x + y = 9 is at a distance of 3√2 from A. Find the angle which the line L makes With the positive direction of the X – axis.
Solution:
Suppose a is the angle made by L with the positive X – axis
Any point on the line is
(x₁ + r cos α₁, y₁ + r sin α) = (2 + 3√2 cos α 1 + 3√2 sin α)
This is a point on the line x + y = 9
2+ 3√2 cos α+ 1 + 3√2 sin α = 9
3√2 (cos α + sin α) = 6
cos α + sm α = \(\frac{6}{3 \sqrt{2}}\) = √2
\(\frac{1}{\sqrt{2}}\). cos α + \(\frac{1}{\sqrt{2}}\) sin α = 1
cos α. cos 45° + sin α. sin 45° = 1
cos (α – 45°) = cos 0°
α – 45° = 0 ⇒ α = 45° = \(\frac{\pi}{3}\)

Question 5.
A straight line L with negative slope passes through the point (8, 2) and cuts positive co-ordinate axes at the points P and Q. Find the minimum value of OP + OQ as L varies, when O is the origin.
Solution:
Equation of the line passing through A(8, 2) with negative slope ‘-m’ is y – 2 = -m(x – 8)
mx + y – (2 + 8m) = 0
mx + y = 2 + 8m

OP =X Intercept = \(\frac{2+8 m}{m}\)
OQ = Y – Intercept = 2 + 8m 2 + 8m

For f(m) to have minimum or maximum, we must have f'(m) = 0

and ‘f’ has minimum at m = \(\frac{1}{2}\)
∴ Minimum value of f(m) = Minimum Value I of OP + OQ at m = \(\frac{1}{2}\)

∴ Minimum value of OP + OQ as L varies, where ‘O’ is the origin is 18.

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B The Straight Line Solutions Exercise 3(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B The Straight Line Solutions Exercise 3(a)

I.

Question 1.
Find the slope of the line x + y = 0 and x – y = 0.
Solution:
Slope of x + y = 0 is – \(\frac{a}{b}\) = -1
Slope of x – y = 0 is 1

Question 2.
Find the equation of the line containing the points (2, -3) and (0, -3).
Solution:
Equation of the line is
(y – y₁) (x₁ – x₂) = (x – x₁) (y₁ – y₂)
(y + 3)(2 – 0) = (x – 2)(-3 + 3)
2(y + 3) = 0
⇒ y + 3 = 0

Question 3.
Find the equation of the line containing the points (1, 2) and (1, -2).
Solution:
Equation of the line is
(y – y₁) (x₁ – x₂) = (x – x₁) (y₁ – y₂)
(y – 2)(1 – 1) = (x – 1) (2 + 2)
0 = 4(x – 1) ⇒ x – 1 =0

Question 4.
Find the angle which the straight line y = √3x – 4 makes with the Y-axis.
Solution:
Equation of the line is y = √3x – 4
Slope = m = √3 = tan \(\frac{\pi}{6}\)
Angle made with X-axis = \(\frac{\pi}{6}\)
Angle made with Y – axis = \(\frac{\pi}{2}-\frac{\pi}{6}=\frac{\pi}{3}\)

Question 5.
Write the equation of the reflection of the line x = 1 in the Y-axis.
Solution:

Equation of PQ is x = 1
Reflection about Y – axis is x = – 1
i.e., x + 1 = 0

Question 6.
Find the condition for the points (a, 0), (h, k) and (0, b) when ab ≠ 0 to be collinear.
Solution:
A(a, 0), B(h, k), C(0, b) are collinear.
⇒ Slope of AB = Slope of AC
\(\frac{k-0}{h-a}=\frac{-b}{a}\)
ak = -bh + ab
bh + ak = ab
\(\frac{h}{a}+\frac{k}{b}=1\)

Question 7.
Write the equations of the straight lines parallel to X-axis is
i) at a distance of 3 units above the X-axis and ii)at a distance of 4 units below the X-axis.
Solution:
I)

Equation of the required line AB is y = 3

ii)

Equation of A’B’ is y = — 4 ; y + 4 = 0

8. Write the equations of the straight line parallel to Y – axis and
i) at a distance of 2 units from the Y-axis to the right of it.
ii) at a distance of 5 units from the Y-axis to the left of it.
Solution:
i)

Equation of the required line AB is x = 2

ii)

Equation of the required line A’B’
x = -5
x + 5 = 0

II.

Question 1.
Find the slopes of the straight line passing through the following pairs of points.
i) (-3, 8) (10, 5)
ii) (3, 4) (7, -6)
iii) (8,1), (-1, 7)
iv) (-P, q) (q, -p) (pq ≠ 0)
Solution:

Question 2.
Find the value of x, if the slope of the line passing through (2, 5) and (x, 3) is 2.
Solution:
Slope = \(\frac{y_{1}-y_{2}}{x_{1}-x_{2}}=\frac{5-3}{2-x}=2\)
2 = 2(2 – x)
x = 2 – 1 = 1

Question 3.
Find the value of y if the line joining the points (3, y) and (2, 7) is parallel to the line joining the points (-1, 4) and (0,6).
Solution:
A(3, y), B(2, 7), P(-1, 4) and Q(0, 6) are the given points.
m₁ = Slope of AB = \(\frac{y-7}{3-2}\) = y – 7
m₂ = Slope of PQ = \(\frac{4-6}{-1-0}=\frac{-2}{-1}=2\)

AB and PQ are parallel
m₁ = m₂ ⇒ y – 7 = 2
y = 2 + 7 = 9

Question 4.
Find the slopes of the lines i) parallel to and ii) perpendicular to the line passing through (6, 3) and (- 4,5).
Solution:
A(6, 3) and B(-4, 5) are the given points.
m = Slope of AB = \(\frac{3-5}{6+4}=\frac{-2}{10}=-\frac{1}{5}\)

PQ is parallel to AB
(i) Slope of PQ = m = – \(\frac{1}{5}\)
RS is perpendicular to AB

(ii)Slope of RS = – \(\frac{1}{m}\) =5 m

Question 5.
Find the equation of the straight line which makes the following angles with the positive X-axis in the positive direction and which pass through the points given below
i) \(\frac{\pi}{4}\) and (0,0)
ii) \(\frac{\pi}{3}\) and(1, 2)
iii) 135° and (3, -2)
iv) 150° and (-2, -1)
Solution:
i) m = Slope = tan 45° = 1
Equation of the line is y – y₁ = m(x – x₁)
y – 0 = 1(x – 0)
i.e., y = x
or x – y = 0

ii) m = tan 60° – √3
Equation of the line is
y – 2 = √3 (x – 1)
= √3x – √3
√3x – y+(2 – √3) = 0

iii) m = tan 135° = tan (180° -45°)
= – tan 45° = -1
Equation of the line is y + 2 = -1(x – 3)
= – x + 3
i.e., x + y – 1 = 0

iv) m = tan 150° = tan (180° – 30°)
= – tan 30° = – \(\frac{1}{\sqrt{3}}\)
Equation of the line is
y + 1 = – \(\frac{1}{\sqrt{3}}\) (x + 2)
√3y + √3 = – x – 2
x + √3y + (2 + √3) = 0

Question 6.
Find the equations of the straight lines passing through the origin and making equal angles with the co-ordinate axes.
Solution:
Case (i) : PP1 makes an angle 45° with positive X-axis
m = tan 45° = 1
PP’ passes through 0(0, 0)
Equation of PP’ is y – 0 = 1 (x – 0)

Case ii) : QQ’ makes an angle 135° with positive X-axis
m = tan 135° = tan (180° – 45°) = -tan 45°
Equation of QQ’ is y – 0 = -1 (x – 0)
y = -x

Question 7.
The angle made by a straight line with the positive X-axis in the positive direction and the Y-intercept cut off by it are given below. Find the equation of the straight line.
i) 60°, 3
ii) 150°, 2
iii) 45°, -2
iv) Tan^-1(\(\frac{2}{3}\)), 3
Solution:
i) Equation of the line is y = mx + c
m = tan 60° = √3, c = 3
Equation of the line is y = √3x + 3
√3x – y + 3 = 0

ii) m = tan 150° = tan (180°-30°)
= – tan 30° = \(\frac{-1}{\sqrt{3}}\), c = 2
Equation of the line is y =- \(\frac{1}{\sqrt{3}}\)x + 2
√3 y = -x + 2 √3x
x + √3y – 2 – √3 =0

iii) m = tan 45° = 1
c = -2
Equation of the line is
y = x – 2
x – y – 2=0

iv) θ = tan^-1(\(\frac{2}{3}\)) ⇒ m = tan θ = \(\frac{2}{3}\),c = 3
Equatidn of the line is y = \(\frac{2}{3}\) x + 3
3y = 2x + 9
2x – 3y + 9 = 0

Question 8.
Find the equation of the straight line passing through (-4, 5) and cutting off equal and non-zero intercepts on the coordinate axes.
Solution:
Equation of the line in the intercept form is
\(\frac{x}{a}+\frac{y}{b}\) = 1
Given a = b
Equation of the line is \(\frac{x}{a}+\frac{y}{b}\) = 1
⇒ x + y = a
This line passes through P(- 4, 5)
-4 + 5 = a ⇒ a = 1
Equation of the required line is x + y = 1 or x + y – 1 = 0

Question 9.
Find the equation of the straight line passing through (-2, 4) and making non¬zero intercepts whose sum is zero.
Solution:
Equation of the line in the intercept form is
\(\frac{x}{a}+\frac{y}{b}\) = 1
Given a + b = 0 ⇒ b = -a
Equation of the line is \(\frac{x}{a}-\frac{y}{b}\)
⇒ x – y = a
This line passes through P(-2,4)
∴ -2 – 4 = a ⇒ a = -6
Equation of the required line is x – y = -6
⇒ x – y + 6 = 0

III.

Question 1.
Find the equation of the straight line passing through the point (3, -4) and making X and Y-intercepts which are in the ratio 2 : 3.
Solution:
Equation of the line in the intercept form is x y
\(\frac{x}{a}+\frac{y}{b}\) = 1
Given \(\frac{a}{b}\) = \(\frac{2}{3}\) ⇒ b = \(\frac{3a}{2}\)
Equation of the line is \(\frac{x}{a}+\frac{2 y}{3 a}=1\)
⇒ 3x + 2y = 3a
This line passes through P(3, – 4)
9 – 8 = 3a ⇒ 3a = 1

Equation of the required line is 3x + 2y = 1
⇒ 3x + 2y – 1 = 0

Question 2.
Find the equation of the straight line passing through the point (4, -3) and perpendicular to the line passing through the points (1, 1) and (2, 3).
Solution:
A(1, 1), B(2, 3) are the given points.
m = Slope of AB = \(\frac{1-3}{1-2}=\frac{-2}{-1}=2\)

PQ is perpendicular to AB
Slope of PQ = –\(\frac{1}{m}\) = – \(\frac{1}{2}\)
PQ passes through P(4, -3)
Equation of PQ is y – y₁ = m(x – x₁)
y + 3 = –\(\frac{1}{2}\)(x – 4)
2y + 6 = -x + 4 ⇒ x + 2y + 2 = 0

Question 3.
Show that the following sets of points are collinear and find the equation of the line L containing them.
i) (-5, 1), (5, 5), (10, 7)
ii) (1, 3), (-2, – 6), (2, 6)
iii) (a, b + c), (b, c + a), (c, a + b)
Solution:
i) A(-5, 1), B(5, 5), C(10, 7) are the given points.
Equation of AB is
(y – y₁) (x₁ – x₂) = (x – x₁) (y₁ – y₂)
(y- 1) (-5 – 5) = (x + 5) (1 – 5)
– 10y + 10 = -4x – 20
4x – 10y + 30 = 0
or 2x – 5y + 15 = 0

C(10, 7)
2x – 5y + 15 = 2.10 – 5.7 + 15
= 20 – 35 + 15 = 0

A, B, C are collinear.
Equation of the line containing them is 2x – 5y + 15 = 0

ii) A(1, 3), B(-2, -6), C(2, 6)
Equation of AB is
(y – 3) (1 + 2) = (x – 1) (3 + 6)
3(y – 3) = 9(x – 1)
y – 3 = 3x – 3
3x – y = 0

C(2, 6)
3x – y = 3.2 – 6 = 6 – 6 = 0

∴ The given points A, B, C are collinear.
Equation of the line containing A,B,C is 3x – y = 0

iii) A(a, b f c), B(b, c + a), C(c, a + b)
Equation of AB is
(y – (b + c)) (a-b) = (x – a)(b + c – c – a)
(y – b – c) (a – b) = -(a – b) (x – a)
y – b – c = -x + a
or x + y – (a + b + c) = 0
C (c, a + b)
c + a + b – a – b – c = 0
C lies on AB
A, B, C are collinear.
Equation of the line containing them is x + y = a + b + c

Question 4.
A(10, 4), B(-4, 9) and C(-2, -1) are the vertices of a triangle. Find the equations of
i) \(\stackrel{\leftrightarrow}{A B}\)
ii) the median through A
iii) the altitude through B
iv) the perpendicular bisector the side of \(\stackrel{\leftrightarrow}{A B}\)
Solution:
i) A(10,4), B(-4, 9) are the given points.
Equation of AB is
(y – 4) (10 + 4) = (x – 10) (4 – 9)
14y – 56 = -5x + 50
5x + 14y – 106 = 0

ii) D is the mid-point of BC

A (10,4) is the other vertex Equation of AD is
(y – 4) (10 + 3) = (x + 3) (4 – 4)
13(y – 4) = 0 ⇒ y – 4 = 0 (or) y = 4

iii)

Slope of AC = \(\frac{4+1}{10+2}=\frac{5}{12}\)
BE is perpendicular to AC
Slope of BE = \(\frac{-1}{m}=\frac{-12}{5}\)

BE passes through B(-4, 9)
Equation of the altitude BE is
y – 9 = \(\frac{-12}{5}\)(x + 4)
5y – 45 = -12x – 48
12x + 5y + 3 = 0

iv) O is the mid-point of AB

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Transformation of Axes Solutions Exercise 2(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Transformation of Axes Solutions Exercise 2(a)

I.

Question 1.
When the origin is shifted to (4, -5) by the translation of axes, find the coordinates of the following points with reference to new axes.
i) (0, 3), ii) (-2, 4) iii) (4, -5)
Solution:
i) New origin = (4, -5); h = 4, k = -5
Old co-ordinates are (0, 3)
x = 0, y = 3
x’ = x – h = 0 – 4 = -4
y’=y-k = 3 + 5 = 8
New co-ordinates are (—4, 8)

ii) Old co-ordinates are (-2, 4)
x = -2, y = 4
x’ = x- h = -2 – 4 = -6
y’=y-k=4 + 5 = 9
New co-ordinates are (-6, 9)

iii) Old co-ordinates are (4, -5)
x = 4, y = -5
x’ = x – h = 4- 4 = 0
y = y – k = -5 + 5 = 0
New co-ordinates are (0,0)

Question 2.
The origin is shifted to (2, 3) by the translation of axes. If the coordinates of a point P changes as follows, find the coordinates of P in the original system.
i) (4, 5) ii) (-4, 3), iii) (0, 0)
Solution:
i) New co-ordinates are (4, 5)
x’ = 4, y’ = 5
x = x’ + h = 4 + 2 = 6
y = y’ + k = 5 + 3 = 8
Old co-ordinates are (6, 8)

ii) New co-ordinates are (-4, 3)
x’ = – 4, y’ = 3
x = x’ + h = -4 + 2 = -2
y = y’ + k = 3 + 3 = 6
Old co-ordinates are (-2, 6)

iii) New co-ordinates are (0, 0)
x’ = 0, y’ = 0
x = x’ + h = 0 + 2 = 2
y = y’ + k = 0 + 3 = 3
Old co-ordinates are (2, 3)

Question 3.
Find the, point to which the origin is to be shifted so that the point (3, 0) may change to (2, -3).
Solution:
(x, y) = (3, 0)
(x’, y’) = (2, -3)
Let (h, k) be the shifting origin.
h = x – x’= 3- 2 = 1
k = y – y’ = 0 + 3 = 3
∴ (h, k) = (1, 3)

Question 4.
When the origin is shifted to (-1, 2) by the translation of axes, find the transformed equations of the following.
i) x² + y² + 2x – 4y.+ 1 = 0
ii) 2x² + y² – 4x + 4y = 0
Solution:
i) The given equation is
x² + y² + 2x – 4y + 1 = 0
Origin is shifted to (-1, 2)
h = -1, k = 2
Equation of transformations are
x = x’ + h, y = y’ + k
i.e., x = x’ – 1, y = y’ + 2
The new equation is
(x’ – 1)² + (y’ + 2)² + 2(x’ – 1) – 4(y’ + 2) + 1 = 0
⇒ (x’)² + 1 – 2x’ + (y’)² + 4 + 4y’ + 2x’ – 2 -4y’ – 8 + 1 = 0
(x’)² + (y’)² -4 = 0
The transformed equation is x² + y² – 4 = 0

ii) Old equation is
2x² + y² – 4x + 4y = 0
New equation is 2(x’ – 1)² + (y’ + 2)² —4(x’ – 1) + 4(y’ + 2) = 0
2[(x’)² + 1 – 2x’] + (y’)² + 4 + 4y’ – 4x’ + 4 + 4y’ + 8 = 0
2(x’)² + 2 – 4x’ + (y’)² + 4 + 4y’ – 4x’ + 4 + 4y’ + 8 = 0
2(x’)² + (y’)² – 8x’ + 8y’ + 18 = 0
The transformed equation is
2x² + y² – 8x + 8y+18 = 0

Question 5.
The point to which the origin is shifted and the transformed equation are given below. Find the original equation.
i) (3,-4);x² + y² = 4
ii) (-1, 2); x² + 2y² + 16 = 0
Solution:
i) Given shifting origin = (3, – 4) = (h, k)
x’ = x – h,
= x – 3

y’ = y – k
= y + 4

The original equation of (x’)² + (y’)² = 4 is
(x – 3)² + (y + 4)2 = 4
x² – 6x + 9 + y² + 8y + 16 = 4
x² + y² – 6x + 8y + 21 =0

ii) Given shifting origin = (h, k) = (-1,2)
x’ = x – h,
= x + 1

y’ = y – k
y = y – 2

The original equation of
x’² + 2y’² + 16 = 0 is
(x + 1)² + 2(y – 2)² + 16 = 0
x² + 2x + 1 + 2y² – 8y + 8 + 16 = 0
x² + 2y² + 2x – 8y + 25 = 0

Question 6.
Find the point to which the origin is to be shifted so as to remove the first degree terms from the equation.
4x² + 9y² – 8x + 36y + 4 = 0
Solution:
The given equation is
4x² + 9y² – 8x + 36y + 4 = 0
a = 4 g = -4
b = 9 f = 18

Origin should be shifted to (1, -2)

Question 7.
When the axes are rotated through an angle 30°, find the new coordinates of the following points,
i) (0, 5) ii) (-2, 4) hi) (0, 0)
Solution:
i) Given 0 = 30°
Old co-ordinates are (0, 5)
i.e., x = 0, y = 5
x‘ = x. cos θ + y. sin θ
= 0. cos 30° + 5. sin 30° = \(\frac{5}{2}\)
= – x sin θ + y cos θ
= – 0. sin 30° + 5 cos 30° =
New co-ordinates are \(\left(\frac{5}{2}, \frac{5 \sqrt{3}}{2}\right)\)

ii) Old co-ordinates are (-2, 4)
x= -2, y = 4
x’ = x cos θ + y sin θ
= (-2). cos 30°+ 4. sin 30°
= -2. \(\frac{\sqrt{3}}{2}\) + 4. \(\frac{1}{2}\) = – √3 +2
y’ = -x sin θ + y cos θ
= – (-2) sin 30° + 4 cos 30°
= 2 . \(\frac{1}{2}\) + 4. \(\frac{\sqrt{3}}{2}\)
= 1 + 2 √3
New co-ordinates are (- √3 + 2, 1 + 2√3)

iii) Given (x, y) = (0,0) and 0 = 30°
x = (0, y) ⇒ x = x’. cos 30° – y’ sin 30°
= 0. \(\frac{\sqrt{3}}{2}\) – 0. \(\frac{1}{2}\) =0
y = x’. sin 30° + y’.cos 30°
= 0.\(\frac{1}{2}\) + 0.\(\frac{\sqrt{3}}{2}\) = 0
New co-ordinates of the point are (0, 0)

Question 8.
When the axes are rotated through an angle 60°, the new co-ordinates of three points are the following
i) (3, 4) ii) (-7, 2) iii) (2, 0) Find their original coordinates.
Solution:
i) Given 0 = 60°
New co-ordinates are (3, 4)
x’ = 3, y’ = 4
x = x’ cos θ – y’ sin θ
= 3. cos 60° – 4. sin 60°
\(=3 \cdot \frac{1}{2}-\frac{4 \cdot \sqrt{3}}{2}=\frac{3-4 \sqrt{3}}{2}\)
y = x’ sin θ + y’ cos θ
= 3 sin 60° + 4. cos 60°

ii) New co-ordinates are (-7, 2)
x’= -7, y’ = 2
x = x’ cos θ – y’ sin θ
= (-7) cos 60° – 2. sin 60°
\(=-7 \cdot \frac{1}{2}-2 \cdot \frac{\sqrt{3}}{2}=\frac{-7-2 \sqrt{3}}{2}\)
y = x’ sin θ + y’. cos θ
= – 7. sin 60° + 2. cos 60°

iii) New co-ordinates are (2, 0)
x’ = 2, y’ = 0
x = x’ cos θ – y’ sin θ
= 2. cos 60° – 0. sin 60°
= 2.\(\frac{1}{2}\) – 0.\(\frac{\sqrt{3}}{2}\) =1 – 0 = 1
y = x’ sin θ + y’ cos θ
= 2. sin 60° + 0. cos 60°
= 2.\(\frac{\sqrt{3}}{2}\) + 0.\(\frac{1}{2}\) = √3
Co-ordinates of R are (1, √3)

Question 9.
Find the angle through which the axes are to be rotated so as to remove the xy term in the equation.
x² + 4xy + y² – 2x + 2y – 6 = 0.
Solution:
Compare the equation
x² + 4xy + y² – 2x + 2y – 6 = 0
with ax² + 2hxy + by² + 2gx + 2fy + c = 0
a = 1, h = 2, b = 1, g = -1, f = 1, c = -6
Let ‘θ’ be the angle of rotation of axes, then

II.

Question 1.
When the origin is shifted to the point (2, 3), the transformed equation of a curve is x² + 3xy – 2y² + 17x – 7y – 11= 0. Find the original equation of the curve.
Solution:
Equations of transformation are
x = x’ + h, y = y’ + k
x’ = x – h = x – 2, y’ = y – 3
Transformed equation is
x² + 3xy – 2y² + 17x – 7y – 11 = 0
Original equation is
(x – 2)² + 3(x – 2) (y – 3) – 2(y – 3)² + 17(x – 2) – 7(y – 3) – 11 = 0
⇒ x² – 4x + 4 + 3xy – 9x – 6y + 18 – 2y² + 12y – 18 + 17x – 34 – 7y + 21 -11 = 0
⇒ x² + 3xy – 2y² + 4x – y – 20 = 0
This is the required original equation.

Question 2.
When the axes are rotated through an angle 45°, the transformed equation of acurveis 17x² – 16xy + 17y² = 225. Find the original equation of the curve.
Solution:
Angle of rotation = θ = 45
x’ = x cos θ + y sin θ = x cos 45 + y sin 45 = \(\frac{x+y}{\sqrt{2}}\)
y’ = – x sin θ + y cos θ = – x sin 45 + y cos 45 = \(\frac{-x+y}{\sqrt{2}}\)

The original equation of
17x’²- 16x’y’ + 17y’² = 225 is

⇒ 17x² + 17y² + 34xy – 16y² + 16x² + 17x² + 17y² – 34xy = 450
⇒ 50x² + 18y² = 450
∴ x² + y² = 9 is the original equation.

Question 3.
When the axes are rotated through an angle a, find the transformed equation of x cos a + y sin a = p. iMM&mmn
Solution:
The given equation is x cos α + y sin α = p
∵ The axes are rotated through an angle α
x = x’ cos α – y’ sin α
y = x’ sin α + y’ cos α

The given equation transformed to
(x’ cos α – y’ sin α) cos α +
(x’ sin α + y’ cos α) sin α = p
⇒ x’ (cos² α + sin² α) = p
⇒ x’ = p
The equation transformed to x = p

Question 4.
When the axes are rotated through an angle n/6. Find the transformed equation of x² + 2 √3xy – y² = 2a².
Solution:

⇒ 3X² – 2√3 XY + Y² + 2√3|√3X² +2XY – √3Y²|- (x² +3Y² +2√3XY) =8a²
⇒ 3X² -2V3XY +Y² +6X² + W3XY – 6Y² – X² – 3Y² – 2√3 XY = 8a²
⇒ 8X² – 8Y² = 8a² ⇒ X² – Y² = a²
The transformed equation is x² – y² = a²

Question 5.
When the axes are rotated through an angle \(\frac{\pi}{4}\), find the transformed equation of 3x² + 10xy + 3y² = 9.
Solution:
Given equation is
3x² + 10xy + 3y² – 9 = 0 ………….. (1)
Angle of rotation of axes = θ = \(\frac{\pi}{4}\)
Let (X, Y) be the new co-ordinates of (x, y)
x = X cos θ – Y sin θ

Transformed equation of (1) is

⇒ 3X² – 6XY + 3Y² + 10X² – 10Y² + 3X² + 6XY + 3Y² – 18 = 0
∴ 16X² – 4Y² -18 = 0
∴ 8X² – 2Y² = 9
∴ 8X² – 2Y² = 9
The transformed equation is 8x² – 2y² = 9

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Locus Solutions Exercise 1(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Locus Solutions Exercise 1(a)

I.

Question 1.
Find the equation of the locus of a point that is at a distance 5 from A (4, – 3).
Solution:
A (4, -3) is the given point P(x, y) is any point on the locus.

Given condition is CP = 5
CP² = 25
(x – 4)² + (y + 3)² = 25
x² – 8x + 16 + y² + 6y + 9 – 25 = 0
Equation of the locus of P is x²+ y² – 8x + 6y = 0.

Question 2.
Find the equation of locus of a point which is equidistant from the points A(-3, 2) and B(0, 4).
Solution:
A (-3, 2), B (0,4) are the given points
P (x, y) is any point on the locus

Given condition is PA = PB
PA² = PB²
(x+3)² + (y – 2)² = (x – 0)² + (y – 4)²
x² + 6x + 9 + y² – 4y + 4 = x² + y² – 8y +16
6x + 4y = 3 is the equation of the locus.

Question 3.
Find the equation of locus of a point P such that the distance of P from the origin is twice the distance of P from A (1, 2).
Solution:
O(0,0), A (1,2) are the given points
P (x, y) is any point on the locus

Given condition is OP = 2AP
OP² = 4 AP²
x² + y² = 4 [(x – 1)² + (y – 2)²]
= 4 (x² – 2x + 1 + y² – 4y + 4)
x² + y² = 4x² + 4y² – 8x – 16y + 20
Equation to the locus of P is
3x² + 3y² – 8x – 16y + 20 = 0.

Question 4.
Find the equation of locus of a point which is equidistant from the coordi¬nate axes.
Solution:
P(x, y) is any point on the locus.
PM and PN are perpendiculars from P on X and Y – axes.

Given PM = PN ⇒ PM² = PN²
y² = x²
Locus of P is x² – y² = 0

Question 5.
Find the equation of locus of a point equidistant from A (2, 0) and the Y- axis.
Solution:
A (2,0) is the given point.
P (x, y) is any point on the locus.

Draw PN perpendicular to Y – axis.
Given condition is PA = PN
PA² = PN²
(x – 2)² + (y – 0)² = x²
x² – 4x + 4 + y² = x²
Locus of P is y² – 4x + 4 = 0

Question 6.
Find the equation of locus of a point P, the square of whose distance from the origin is 4 times its y coordinate.

Solution:
P(x, y) is any point on the locus
Given condition is OP² = 4y ⇒ x² + y² = 4y
Equation of the locus of P is x² + y² – 4y = 0

Question 7.
Find the equation of locus of a point ‘P’ such that PA² + PB² = 2c², where A = (a, 0), B = (-a, 0) and 0 < |a| < |c|.
Solution:
Let P (x, y) be a point in locus.
A = (a, 0); B = (-a, 0)
Given condition is PA² + PB² = 2c²
(x – a)² + (y – 0)² + (x + a)² + (y – 0)² = 2c²
x² – 2ax + a² + y² + x² + 2ax + a² + y² = 2c²
2x² + 2y² = 2c² – 2a²
∴ x² + y² = c² – a² is the locus.

II.

Question 1.
Find the equation of locus of P, if the line segment joining (2, 3) and (-1, 5) subtends a right angle at P.
Solution:
A(2,3), B (-1, 5) are the given points.
P(x, y) is any point on the locus.

Given condition is, ∠APB = 90°
AP² + PB² = AB²
(x – 2)² + (y – 3)² + (x + 1)² + (y – 5)² = (2 + 1)² + (3 – 5)²
x² – 4x + 4 + y² – 6y + 9 + x² + 2x + 1+ y² – 10y + 25 = 9 + 4
2x² + 2y² – 2x – 16y + 26 = 0
Locus of P is x² + y² – x – 8y + 13 = 0
(x, y) ≠ (2,3) and (x, y) ≠ (-1, 5)

Question 2.
The ends of the hypotenuse of a right angled triangle are (0, 6) and (6, 0). Find the equation of locus of its third vertex.
Solution:
A(0,6), B (6,0) are the ends of the hypotenuse,
P (x, y) is the third vertex.
∴ The given condition is ∠APB = 90°

AP² + PB² = AB²
(x – 0)² + (y – 6)² + (x – 6)² + (y – 0)² = (6 – 0)² + (0 – 6)²
x² + y² – 12y + 36 + x² – 12x + 36 + y² = 36 + 36
2x² + 2y² -12x -12y = 0
Locus of P is x² + y² – 6x – 6y = 0
(x, y) ≠ (0,6) and (x, y) ≠ (6,0).

Question 3.
Find the equation of the locus of a point, the difference of whose distances from (-5, 0) and (5, 0) is 8.
Solution:
A(5,0), B(-5,0) are the given points.

P(x, y) is any point on the locus.
Given condition is |PA – PB| = 8
PA – PB = 8 ………..(1)
PA² – PB² = [(x – 5)² + (y – 0)²] – [(x + 5)² + (y – 0)²]
= x² – 10x + 25 + y² – x² – 10x – 25 – y²
= – 20x
(PA + PB) (PA – PB) = -20x
(PA + PB) 8 = -20x
PA + PB = –\(\frac{5}{2}\)x …………..(2)
Adding (1) and (2),
2PA = –\(\frac{5x}{2}\) + 8 = \(\frac{-5 x+16}{2}\)
4PA = – 5x + 16
16PA² = (- 5x + 16)²
16 [(x – 5)² + y²] = (- 5x + 16)²
16 [x² – 10x + 25 + y²] = [- 5x + 16]²
16x² + 16y²2 – 160x + 400 = 25x² + 256 – 160x
9x² – 16y² = 144
Dividing with 144, locus of P is
\(\frac{9 x^{2}}{144}-\frac{16 y^{2}}{144}=1\) i.e., \(\frac{x^{2}}{16}-\frac{y^{2}}{9}=1\)

Question 4.
Find the equation of locus Of P, if A=(4,0), B = (- 4,0) and |PA- PB| =4.
Solution:
A = (4, 0), B = (-4, 0) are the given points.
P (x, y) is any point on the locus.
The given condition is |PA – PB| = 4 …………… (1)
PA² – PB²= [(x- 4)² + (y – 0)²] – [(x + 4)² + y²]
= x² – 8x+ 16 + y² – x² – 8x – 16 – y²
= -16x

(PA + PB) (PA – PB) = -16x
(PA + PB) 4 = – 16x
PA + PB = – 4x ………….. (2)
Adding (1) and (2),
2PA = 4 – 4x
PA = 2 – 2x
PA² = (2 – 2x)²
(x – 4)² + (y – 0)² = (2 – 2x)²
x² – 8x + 16 + y² = 4 + 4x² – 8x
3x² – y² = 12
Dividing with 12, locus of P is \(\frac{3 x^{2}}{12}-\frac{y^{2}}{12}=1\)
i.e., \(\frac{x^{2}}{4}-\frac{y^{2}}{13}=1\)

Question 5.
Find the equation of the locus of a point, the sum of whose distances from (0, 2) and (0, -2) is 6.
Solution:
A (0,2), B (0, -2) are the given points.

P(x, y) is any point on the locus.
Given condition is PA + PB = 6 ……….. (1)
PA² – PB² = [(x – 0)² + (y – 2)²] – [(x – 0)² + (y + 2)²]
= x² + y² – 4y + 4 – x² – y² – 4y – 4 = – 8y
(PA + PB) (PA – PB) = -8y
6(PA – PB) = – 8y
PA – PB = –\(\frac{8y}{6}\)
PA-PB = –\(\frac{4y}{3}\) ……. (2)
Adding (1) and (2), 2PA = 6 –\(\frac{4y}{3}\)

9x² + 9y² + 36 = 81 + 4y²
9x² + 5y² = 45
Dividing with 45,

Question 6.
Find the equation (rf the locus of P, if A= (2,3), B = (2, -3) and PA + PB = 8.
Solution:
A (2, 3), B (2, -3) are the given points.

P(x, y) is any point on the locus.
Given condition is PA + PB = 8 ……….. (1)
PA² – PB² = [(x – 2)² + (y – 3)²] – [(x – 2)² + (y + 3)²]
= (x – 2)² + (y – 3)² – (x – 2)² – (y + 3)² = (y – 3)² – (y + 3)² = -12y
(PA + PB) (PA – PB) = -12y
8(PA – PB) = -12y
PA – PB = \(\frac{-12 y}{8}\)
PA – PB = \(\frac{-3 y}{2}\) ……….. (2)
Adding (1) and (2),
2PA = 8 – \(\frac{3 y}{2}\) = \(\frac{16 -3y}{2}\)
4PA = 16 – 3y
16PA² = (16 – 3y)²
16 [(x – 2)² + (y – 3)²] = (16 – 3y)²
16(x² – 4x + 4 + y² – 6y + 9) = (16 – 3y)²
16x² + 16y² – 64x – 96y + 208 = 256 + 9y² – 96y
16x² + 7y² – 64x – 48 = 0
Locus of P is 16x² + 7y² – 64x – 48 = 0.

Question 7.
A(5, 3) and B (3, -2) are two fixed points. Find the equation of the locus of P, so that the area of triangle PAB is 9.
Solution:
A(5, 3), B(3, -2) are the given points.
P(x, y) is any point on the locus.

Given condition is ∆PAB = 9
\(\frac{1}{2}\)|5(-2, -y) + 3(y – 3) + x(3 + 2)|=9
|-10 – 5y + 3y – 9 + 5x| = 18
5x – 2y – 19 = ± 18
5x – 2y – 19 = 18 or 5x – 2y – 19 = – 18
5x – 2y – 37 = 0 or 5x – 2y – 1 = 0
Locus of P is (5x- 2y- 37) (5x- 2y- 1) = 0

Question 8.
Find the equation of the locus of a point, which forms a triangle of ar5a 2 with the points A(l, 1) and B (-2, 3).
Solution:
A (1, 1), B(-2, 3) are the given points.

P(x, y) is any point on the locus.
Given condition is ∆PAB = 2
\(\frac{1}{2}\)|1(3 – y) – 2 (y – 1) + x (1 – 3)| = 2
|3 – y – 2y + 2 – 2x| = 4
-2x – 3y + 5 = ± 4
-2x – 3y + 5 = 4 or – 2x – 3y + 5 = – 4
2x + 3y – 1 = 0 or 2x + 3y – 9 = 0
Locus of P is (2x + 3y – 1) (2x + 3y – 9) = 0.

Question 9.
If the distance from ‘P’ to the points (2, 3) and (2, -3) are in the ratio 2 : 3, then find the equation of locus of P.
Solution:
Let P (x, y) be a point on locus.
Given points A = (2, 3), B = (2, -3)
Given condition is
PA : PB = 2 : 3
⇒ 3PA = 2PB
⇒ 9PA² = 4PB²
⇒ 9[(x – 2)² + (y – 3)²] = 4[(x – 2)² + (y + 3)²]
⇒ 9[x² – 4x + 4 + y² – 6y + 9] = 4 [x² – 4x + 4 + y² + 6y + 9]
∴ 5x² + 5y² – 20 x – 78 y + 65 = 0 is the equation of locus.

Question 10.
A (1, 2), B (2, -3) and C (-2, 3) are three points. A point ‘P’ moves such that PA² + PB² = 2PC². Show that the equation to the locus of ‘P’ is 7x – 7y + 4 = 0.
Solution:
Let P (x, y) be a point on locus.
Given points A = (1, 2), B = (2) -3) and C = (-2, 3)
Given condition is PA² + PB² = 2 PC²
⇒ (x – 1)² + (y – 2)² + (x – 2)² + (y + 3)² = 2 [(x + 2)² + (y-3)²]
⇒ 2x² + 2y² – 6x + 2y + 18 = 2x² + 2y² + 8x – 12y + 26
⇒ 14x – 14y + 8 = 0
∴ 7x – 7y + 4 = 0 is the equation of locus.

SOLVED PROBLEMS

Question 1.
Find the equation of the locus of a point which is at a distance 5 from (-2, 3) in the xoy plane.
Solution:
Let the given point be A = (-2, 3) and P(x, y) be a point on the plane.
The geometric condition to be satisfied by P to be on the locus is that
AP = 5 …………… (1)
Expressing this condition algebraically, we get
\(\sqrt{(x+2)^{2}+(y-3)^{2}}=5\)
i.e., x² + 4x + 4 + y² – 6y + 9 = 25
i.e., x² + y² + 4x – 6y – 12 = 0 ……………. (2)
Let Q(x₁, y₁) satisfy (2).

Hence AQ = 5.
This means that Q(x₁, y₁) satisfies the geometric condition (1).
∴ The required equation of locus is
x² + y² + 4x – 6y – 12 = 0.

Question 2.
Find the equation of locus of a point P, if the distance of P from A(3,0) is twice the distance of P from B(-3, 0).
Solution:
Let P(x, y) be a point on the locus. Then the geometric condition to be satisfied by P is
PA = 2PB …………. (1)
i.e., PA² = 4PB²
i.e., (x – 3)² + y² = 4 [(x + 3)² + y²]
i.e., x² – 6x + 9 + y² = 4 [x² + 6x + 9 + y²]
i.e., 3x² + 3y² + 30x + 27 = 0
i.e., x2 + y2 + lOx + 9 = 0 ………….. (2)
Let Q(x₁, y₁) satisfy (2).

∴ QA = 2QB.
This means that Q(x₁, y₁) satisfies (1).
Hence, the required equation of locus is
x² + y² + 10x + 9 = 0.

Question 3.
Find the locus of the third vertex of a right angled triangle, the ends of whose hypotenuse are (4, 0) and (0, 4).
Solution:
Let A = (4, 0) and B = (0, 4).
Let P(x, y) be a point such that PA and PB are
perpendicular. Then PA² + PB² = AB².
i.e., (x – 4)² + y² + x² + (y – 4)² = 16 + 16
i.e., 2x² + 2y² – 8x – 8y = 0
or x² + y² – 4x – 4y = 0
Let Q(x₁, y₁) satisfy (2) and Q be different from A and B.

Hence QA² + QB² = AB², Q ≠ A and Q ≠ B.
This means that Q(x₁, y₁) satisfies (1).
∴ The required equation of locus is (2), which is the circle with \(\overline{\mathrm{AB}}\) as diameter, deleting the points A and B.
Though A and B satisfy equation (2), they do not satisfy the required geometric condition.

Question 4.
Find the equation of the locus of P, if the ratio of the distances from P to A(5, -4) and B(7, 6) is 2 : 3.
Solution:
Let P(x, y) be any point on the locus.
The geometric condition to be satisfied by P
is\(\frac{AP}{PB}\) = \(\frac{2}{3}\).
i.e., 3AP = 2PB ………. (1)
i.e., 9AP² = 4PB²
i.e., 9[(x – 5)² + (y + 4)²] = 4[(x – 7)² + (y – 6)²]
i.e., 9[x² + 25 – 10x + y² + 16 + 8y] = 4[x² + 49 – 14x +y² + 36 – 12y]
i.e, 5x² + 5y² – 34x + 120y + 29 = 0 ……… (2)
Let Q(x₁ y₁) satisfy (2). Then

(by using (3))
– 4 [(x₁ – 7)² + (y₁ – 6)²] = 4PB²
Thus 3AQ = 2PB. This means that Q(x₁, y₁) satisfies (1).
Hence, the required equation of locus is
5(x² + y²) – 34x + 120y + 29 = 0.

Question 5.
A(2, 3) and B(-3, 4) are two given points. Find the equation of locus of P so that the area of the triangle PAB is 8.5.
Solution:
Let P(x, y) be a point on the locus.
The geometric condition to be satisfied by P is that.
area of ∆PAB = 8.5 ……….. (1)
i.e., \(\frac{1}{2}\)|x(3 – 4) + 2(4 – y) – 3(y – 3)| = 8.5
i.e., |-x + 8-2y-3y+9| = 17
i.e., |-x- 5y + 17| = 17
i.e., -x- 5y + 17 = 17 or-x-5y + 17 = -17
i.e., x + 5y = 0 or x + 5y = 34
∴ (x + 5y) (x + 5y – 34) = 0
i.e., x² + 10xy + 25y² – 34x – 170y = 0 …………. (2)
Let Q(x₁, y₁) satisfy (2). Then
x₁ + 5y₁ = 0 or x₁ + 5y₁ = 34 ………… (3)
Now, area of ∆QAB
= \(\frac{1}{2}\)|x₁(3-4) + 2(4-y₁) – 3(y₁ – 3)|
= \(\frac{1}{2}\) |-x₁ + 8 – 2y₁ – 3y₁ + 9|
= \(\frac{1}{2}\) |-x₁ – 5y₁ + 17|
= \(\frac{17}{2}\) =8.5 (by using (3))
This means that Q(x₁, y₁) satisfies (1).
Hence, the required equation of locus is
(x + 5y) (x + 5y – 34) = 0 or
x² + 10xy + 25 – y² – 34x- 170y = 0.

Students get through Maths 1A Important Questions Inter 1st Year Maths 1A Properties of Triangles Important Questions which are most likely to be asked in the exam.

Intermediate 1st Year Maths 1A Properties of Triangles Important Questions

Question 1.
In ∆ABC, if a = 3, b = 4 and sinA = \(\frac{3}{4}\), find angle B.
Solution:
By sine Rule \(\frac{a}{\sin A}\) = \(\frac{b}{\sin B}\)
⇒ sin B = \(\frac{\text { b. } \sin A}{a}\) = \(\frac{4}{3}\) (\(\frac{3}{4}\)) = 1
⇒ sin B = 1 ⇒ B = 90°

Question 2.
If the lengths of the sides of a triangle are 3, 4, 5 find the circumradius of the triangle.
Solution:
∴ 3² + 4² = 5²
∴ The triangle is right angled and its hypotenuse = 5 = circum diameter.
∴ Circum radius = \(\frac{1}{2}\) (hypotenu) = \(\frac{5}{2}\) cms.

Question 3.
If a = 6, b = 5, c = 9, then find angle A.
Solution:
∵ cos A = \(\frac{\mathrm{b}^{2}+c^{2}-a^{2}}{2 b c}\)
= \(\frac{5^{2}+9^{2}-6^{2}}{2(5)(9)}\) = \(\frac{25+81-36}{2(5)(9)}\)
= \(\frac{70}{90}\) = \(\frac{7}{9}\)
∴ A = cos^-1(\(\frac{7}{9}\))

Question 4.
If ∆ABC, show that ∆ (b + c) cos A = 2s.
Solution:
L.H.S. = (b + c) cos A + (c + a) cos B + (a + b) cos C
= (b cos A + a cos B) + (c cos B + b cos C) + (a cos C + c cos A)
= c + a + b = 2S = R.H.S.

Question 5.
If the sides of a triangle are 13, 14, 15, then find the circum diameter.
Solution:
Let a = 13, b = 14, c = 15
Then 2s = a + b + c = \(\frac{13+14+15}{2}\) = \(\frac{42}{2}\)
∴ s = 21
s – a = 21 – 13 = 8
s – b = 21 – 14 = 7
s – c = 21 – 15 = 6
Now ∆ = \(\sqrt{s(s-a)(s-b)(s-c)}\)
= \(\sqrt{21 \times 8 \times 7 \times 6}\)
= \(\sqrt{21 \times 21 \times 16}\) = 21 × 4 = 84
∵ ∆ = \(\frac{a b c}{4 R}\) ⇒ 4R = \(\frac{a b c}{\Delta}\)
= \(\frac{13 \times 14 \times 15}{84}\) = \(\frac{65}{2}\)
∴ R = \(\frac{65}{8}\)
∴ Circum diameter(2R) = 2 × \(\frac{65}{8}\) = \(\frac{65}{4}\)cms.

Question 6.
In a ∆A B C, if (a + b + c) (b + c – a) = 3 be, find A.
Solution:
(2s – sa) = 3bc ⇒ \(\frac{s(s-a)}{b c}\) = \(\frac{3}{4}\)
⇒ cos² \(\frac{A}{2}\) = \(\frac{3}{4}\) ⇒ cos \(\frac{A}{2}\) = \(\frac{1}{2}\) = cos 30°
∴ \(\frac{A}{2}\) = 30° = A = 60°

Question 7.
If a = 4, b = 5, c = 7, find cos \(\frac{B}{2}\).
Solution:
2s = a + b + c = 4 + 5 + 7 = 16
⇒ s = 8 and s – b = 8 – 5 = 3
Now cos \(\frac{B}{2}\) = \(\sqrt{\frac{s(s-b)}{a c}}\) = \(\sqrt{\frac{8 \times 3}{4 \times 7}}\) = \(\sqrt{\frac{6}{7}}\)

Question 8.
In ∆ ABC, find b cos² \(\frac{C}{2}\) + c cos² \(\frac{B}{2}\).
Solution:
b cos² \(\frac{C}{2}\) + c cos ² \(\frac{B}{2}\) = b[latex]\frac{s(s-c)}{a b}[/latex] + c[latex]\frac{s(s-b)}{c a}[/latex]
= \(\frac{s(s-c)}{a}\) + \(\frac{s(s-b)}{a}\) = \(\frac{\mathrm{s}}{\mathrm{a}}\)[s – c + s – b]
= \(\frac{\mathrm{s}}{\mathrm{a}}\) • a = s

Question 9.
If tan \(\frac{A}{2}\) = \(\frac{5}{6}\) and tan \(\frac{C}{2}\) = \(\frac{2}{5}\), determine the relation between a, b, c.    [Mar 05]
Solution:
tan \(\frac{A}{2}\) • tan \(\frac{C}{2}\) = \(\frac{5}{6}\) • \(\frac{2}{5}\)
\(\sqrt{\frac{(s-b)(s-c)}{s(s-a)}} \sqrt{\frac{(s-a)(s-b)}{s(s-c)}}\) = \(\frac{2}{6}\)
⇒ \(\frac{s-b}{s}\) = \(\frac{1}{3}\) ⇒ 3s – 3b = s ⇒ 2s = 3b
⇒ a + b + c = 3b ⇒ a + c = 2b Hence a, b, c are in A.P.

Question 10.
If cot \(\frac{A}{2}\) = \(\frac{b+c}{a}\), find angle B.
Solution:
cot \(\frac{A}{2}\) = \(\frac{b+c}{a}\) ⇒ \(\frac{\cos \frac{A}{2}}{\sin \frac{A}{2}}\) = \(\frac{\cos \left(\frac{B-C}{2}\right)}{\sin \frac{A}{2}}\) (by Mollweide rule)
⇒ \(\frac{A}{2}\) = \(\frac{B-C}{2}\)
⇒ A = B – C ⇒ A + C = B ⇒ A + B + C = 2B
∴ 2B = 180° ⇒ B = 90°

Question 11.
If tan (\(\frac{C-A}{2}\)) = k cot \(\frac{B}{2}\), find k.
Solution:
Comparing with tan (\(\frac{C-A}{2}\)) = \(\frac{c-a}{c+a}\) cot \(\frac{B}{2}\), (by tangent law)
we get that k = \(\frac{c-a}{c+a}\)

Question 12.
In ∆ABC, show that \(\frac{b^{2}-c^{2}}{a^{2}}\) = \(\frac{\sin (B-C)}{\sin (B+C)}\).
Solution:

Question 13.
Show that a² cot A + b² cot B + c² cot C = \(\frac{a b c}{R}\).    [Mar 14]
Solution:
L.H.S. = a² cot A + b² cot B + c² cot C
= 4R² sin² A. \(\frac{\cos A}{\sin A}\) + 4R² sin²B. \(\frac{\cos B}{\sin B}\) + 4R² sin²C. \(\frac{\cos C}{\sin C}\) (by sine rule)
= 2R² (2 sin A cos A + 2 sin B cos B + 2 sin C cos C)
= 2R² (sin 2A + sin 2B + sin 2C) .
= 2R² (4 sin A sin B sin C) (from transformations)
= \(\frac{1}{R}\) (2R sin A) (2R sin B) (2R sin C)
= \(\frac{abc}{R}\) = R.H.S

Question 14.
Show that (b – c)² cos² \(\frac{A}{2}\) + (b + c)² sin² \(\frac{A}{2}\) = a².
Solution:
L.H.S. = (b² + c² – 2bc) cos² \(\frac{A}{2}\) + (b² + c² + 2bc) sin² \(\frac{A}{2}\)
= (b² + c²) [cos² \(\frac{A}{2}\) + sin² \(\frac{A}{2}\)] – 2bc (cos² \(\frac{A}{2}\) – sin² \(\frac{A}{2}\))
= b² + c² – 2bc cos A = a²

Question 15.
Prove that a (b cos C – c cos B) = b² – c²     [Mar 07]
Solution:
L.H.S. = ab cos C – ca cos B
= (\(\frac{a^{2}+b^{2}-c^{2}}{2}\)) – (\(\frac{c^{2}+a^{2}-b^{2}}{2}\)) (by cosine rule)
= \(\frac{1}{2}\)[a² + b² – c² – c² – a² + b²]
= b² – c² = R.H.S.

Question 16.
Show that \(\frac{c-b \cos A}{b-c \cos A}\) = \(\frac{\cos B}{\cos C}\).
Solution:
From projection rule c = a cos B – b cos A and b = c cos A + a cos C

Question 17.
In ∆ABC, if \(\frac{1}{a+c}\) + \(\frac{1}{b+c}\) = \(\frac{3}{a+b+c}\) show that C = 60°.
Solution:
\(\frac{1}{a+c}\) + \(\frac{1}{b+c}\) = \(\frac{3}{a+b+c}\)
⇒ \(\frac{b+c+a+c}{(a+c)(b+c)}\) = \(\frac{3}{a+b+c}\)
⇒ 3(a + c) (b + c) = (a + b + 2c) (a + b + c)
⇒ 3(ab + ac + bc + c²) = (a² + b² + 2ab) + 3c(a + b) + 2c²
⇒ ab = a² + b² – c²
⇒ 2ab cos C (from cosine rule)
⇒ cos C = \(\frac{1}{2}\) ⇒ C = 60°

Question 18.
If a = (b – c) sec θ, prove that tan θ = \(\frac{2 \sqrt{b c}}{b-c}\) sin \(\frac{A}{2}\).    [Mar 16]
Solution:
a = (b – c) sec θ ⇒ sec θ = \(\frac{a}{b-c}\)
tan² θ = sec² θ – 1

Question 19.
In ∆ABC, show that (a + b + c) (tan \(\frac{A}{2}\) + tan \(\frac{B}{2}\)) = 2c cot \(\frac{C}{2}\).
Solution:

Question 20.
Show that b² sin 2C + c² sin 2B = 2bc sin A.
Solution:
L.H.S. = b² sin 2C + c² sin 2B
= 4R² sin² B (2 sin C cos C) + 4R² sin² C (2 sin B cos B)
= 8R² sin B sin C (sin B cos C + cos B sin C)
= 8R² sin B sin C sin (B + C)
= 2(2R sin B) (2R sin C) sin A
= 2bc sin A = R.H.S.

Question 21.
Prove that cot A + cot B + cot C = \(\frac{a^{2}+b^{2}+c^{2}}{4 \Delta}\)    [Mar 15]
Solution:

Question 22.
Show that a cos² \(\frac{A}{2}\) + b cos² \(\frac{B}{2}\) + c cos ² \(\frac{C}{2}\) = s + \(\frac{\Delta}{R}\).
Solution:
L.H.S. = Σ a cos² \(\frac{A}{2}\) = \(\frac{1}{2}\) Σ a (1 + cos A)
= \(\frac{1}{2}\) Σ (a + a cos A) = \(\frac{1}{2}\) (a + b + c) + \(\frac{1}{2}\) Σ (2R sin A cos A)
= \(\frac{1}{2}\) (2s) + \(\frac{R}{2}\) Σ sin 2A
= s + \(\frac{R}{2}\) (sin 2A + sin 2B + sin 2C)
= s + \(\frac{R}{2}\) (4 sin A sin B sin C)
= s + \(\frac{1}{R}\) (2R² sin A sin B sin C)
= s + \(\frac{\Delta}{R}\) (∵ ∆ = 2R² sin A sin B sin C)
= R.H.S.

Question 23.
In ∆ ABC, if a cos A = b cos B, prove that the triangle is either isosceles or right angled.
Solution:
a cos A = b cos B
⇒ 2R sin A cos A = 2R sin B cos B
⇒ sin 2A = sin 2B (or) = sin (180° – 2B)
Hence 2A = 2B or 2A = 180° – 2B
⇒ A = B or A = 90° – B ⇒ A = B or A + B = 90°
⇒ C = 90°
∴ The triangle is isosceles or right angled.

Question 24.
If cot \(\frac{A}{2}\) : cot \(\frac{B}{2}\) : cot \(\frac{C}{2}\) = 3 : 5 : 7, show that a : b : c = 6 : 5 : 4.
Solution:

Then s – a = 3k, s – b = 5k, s – c = 7k
Adding 3s – (a + b + c) = 3k + 5k+ 7k
⇒ 3s – 2s = 15k ⇒ s = 15k
Now a = 12k, b = 10k, c = 8k
∴ a : b : c = 12k : 10k : 8k = 6 : 5 : 4

Question 25.
Prove that a³ cos (B – C) + b³ cos (C – A) + c³ cos(A – B) = 3abc.
Solution:
L.H.S. = Σ a³ cos (B – C)
= Σ a² (2R sin A) cos (B – C)
= R Σ a² [2 sin (B + C) cos (B- C)]
= R Σ a² (sin 2B + sin 2C)
= R Σ a² (2 sin B cos B + 2 sin C cos C)
= Σ [a²(2R sin B) cos B + a²(2R sin C) cos C] Σ (a² b cos B + a²c cos C)
= (a²b cos B + a²c cos C) + (b²c cos C + b² a cos A) + (c² a cos A + c²b cos B)
= ab (a cos B + b cos A) + bc (b cos C + c cos B) + ca (c cos A + a cos C)
= ab(c) + bc(a) + ca(b) = 3 abc = R.H.S

Question 26.
If p₁, p₂, p₃ are the altitudes of the ∆ A, B, C show that \(\frac{1}{p_{1}^{2}}+\frac{1}{p_{2}^{2}}+\frac{1}{p_{3}^{2}}\) = \(\frac{\cot A+\cot B+\cot C}{\Delta}\)
Solution:
Since p₁, p₂, p₃ are the altitudes of ∆ ABC,

Question 27.
The angle of elevation of the top point P of the vertical tower PQ of height h from a point A is 45° and from a point B is 60°, where B is a point at a distance 30 meters from the point A measured along the line AB which makes an angle 30° with AQ. Find the height of the tower.
Solution:

PQ = h, ∠PAQ = 45°
∠BAQ = 30° and ∠PBC = 60°
Also AB = 30 mts.
∴ ∠BAP = ∠APB = 15°.
This gives BP = AB = 30 and h = PC + CQ = BP sin 60° + AB sin 30°
= 15 \(\sqrt{3}\) + 15 = 15(\(\sqrt{3}\) +1) metres.

Question 28.
Two trees A and B are on the same side of a river. From a point C in the river the distances of the trees A and B are 250m and 300m respectively. If the angle C is 45°, find the distance between the trees (use \(\sqrt{2}\) = 1.414).
Solution:

From the triangle ABC, using the cosine rule
AB² = 250² + 300² – 2(250) (300) cos 45°
= 100 (625 + 900 – 750\(\sqrt{2}\)) = 46450.
∴ AB = 215.5m. (approximately).

Question 29.
In A ABC, prove that \(\frac{1}{r_{1}}\) + \(\frac{1}{r_{2}}\) + \(\frac{1}{r_{3}}\) = \(\frac{1}{r}\).
Solution:
L.H.S. = \(\frac{1}{r_{1}}\) + \(\frac{1}{r_{2}}\) + \(\frac{1}{r_{3}}\) = \(\frac{s-a}{\Delta}\) + \(\frac{s-b}{\Delta}\) + \(\frac{s-c}{\Delta}\)
= \(\frac{3 s-(a+b+c)}{\Delta}\) = \(\frac{3 s-2 s}{\Delta}\) = \(\frac{s}{\Delta}\) = \(\frac{1}{r}\)
= R.H.S

Question 30.
Show that rr₁r ₂r₃ = ∆²
Solution:
L.H.S. = rr₁r ₂r₃ = \(\frac{\Delta}{s} \cdot \frac{\Delta}{s-a} \cdot \frac{\Delta}{s-b} \cdot \frac{\Delta}{s-c}\)
= \(\frac{\Delta^{4}}{\Delta^{2}}\) = ∆² = R.H.S

Question 31.
In an equilateral triangle, find the value of \(\frac{r}{R}\).
Solution:
\(\frac{r}{R}\) = \(\frac{4 R \sin \frac{A}{2} \sin \frac{B}{2} \sin \frac{C}{2}}{R}\)
= 4 sin³ 30° (∵ A = B = C = 60°)
= 4 . (\(\frac{1}{2}\))³ = \(\frac{1}{2}\)

Question 32.
The perimeter of ∆ ABC is 12 cm. and its in radius is 1 cm. Then find the area of the triangle.
Solution:
Given that 2s = 12 ⇒ s = 6 cm.
and r = 1 cm
Area of ∆ ABC = ∆ = rs = (1) (6) = 6 sq.cms.

Question 33.
Show that rr₁ = (s – b) (s – c).
Solution:
L.H.S. = rr₁
= [(s – b) tan \(\frac{B}{2}\)] [(s – c) cot \(\frac{B}{2}\)]
= (s – b) (s – c) = R.H.S.
ans:

Question 34.
Express \(\frac{a \cos \mathbf{A}+\mathbf{b} \cos \mathbf{B}+\cos \mathbf{C}}{\mathbf{a}+\mathbf{b}+\mathbf{c}}\) in terms of R and r.
Solution:

Question 35.
In ∆ABC, ∆ = 6sq.cm and s = 1.5 cm, find r.
Solution:
r = \(\frac{\Delta}{s}\) = \(\frac{6}{1.5}\) = 4 cm.

Question 36.
Show that rr₁ cot\(\frac{A}{2}\) = ∆.
Solution:
rr₁ cot\(\frac{A}{2}\) = \(\frac{\Delta}{s}\) (s tan \(\frac{A}{2}\)) cot \(\frac{A}{2}\) = ∆

Question 37.
If a = 13, b = 14, c = 15, find r₁.
Solution:
2s = a + b +c = 42
⇒ s = 21
s – a = 8, s – b = 7, s – c = 6
∆² = 21 × 8 × 7 × 6
⇒ ∆ = 7 × 12 = 84 sq. units
∴ r₁ = \(\frac{\Delta}{s-b}\) = \(\frac{84}{8}\) = 10.5 units

Question 38.
If rr₂ = r₁r₃, then find B.
Solution:

Question 39.
In a ∆ABC, show that the sides a, b, c are in A.P if and only if r₁, r₂, r₃ are in H.P.
Solution:
r₁, r₂, r₃ are in A.P.
⇔ \(\frac{1}{r_{1}}\), \(\frac{1}{r_{2}}\), \(\frac{1}{r_{3}}\) are in A.P.
⇔ \(\frac{s-a}{\Delta}\), \(\frac{s-b}{\Delta}\), \(\frac{s-c}{\Delta}\) are in A.P.
⇔ s – a, s – b, s – c are in A.P.
⇔ -a, -b, -c are in A.P.
⇔ a, b. c, are in A.R

Question 40.
If A = 90°, show that 2(r + R) = b + c.
Solution:
L.H.S = 2r + 2R
= 2(s – a) tan \(\frac{A}{2}\) + 2R.1
= 2(s – a) tan 45°+ 2RsinA
(∵A = 90°)
= (2s – 2a). 1 + a
= b + c = R.H.S.

Question 41.
If (r₂ – r₁) (r₃ – r₁) = 2r₂r₃, show that A = 90°.
Solution:
(r₂ – r₁) (r₃ – r₁) = 2r₂r₃

⇒ 2(bc – ca – ab + a²) = b² + c² + a² + 2bc – 2ca – 2ab
⇒ 2a² = b² + c² + a²
⇒ b² + c² = a²
Hence △ABC is right angled and A = 90°.

Question 42.
Prove that \(\frac{r_{1}\left(r_{2}+r_{3}\right)}{\sqrt{r_{1} r_{2}+r_{2} r_{3}+r_{3} r_{1}}}\) = a
Solution:

Question 43.
Show that \(\frac{1}{r^{2}}+\frac{1}{r_{1}^{2}}+\frac{1}{r_{2}^{2}}+\frac{1}{r_{3}^{2}}\) = \(\frac{a^{2}+b^{2}+c^{2}}{\Delta^{2}}\)
Solution:

Question 44.
Prove that Σ(r + r₁) tan \(\left(\frac{B-C}{2}\right)\) = 0
Solution:
r + r₁ = 4R sin \(\frac{A}{2}\) sin \(\frac{B}{2}\) sin \(\frac{C}{2}\) + 4R sin \(\frac{A}{2}\) cos \(\frac{B}{2}\) cos \(\frac{C}{2}\)
= 4R sin\(\frac{A}{2}\)[sin \(\frac{B}{2}\) sin \(\frac{C}{2}\) + cos \(\frac{B}{2}\) cos \(\frac{C}{2}\)]

Question 45.
Show that \(\frac{r_{1}}{b c}+\frac{r_{2}}{c a}+\frac{r_{3}}{a b}=\frac{1}{r}-\frac{1}{2 R}\) .    [May 07]
Solution:

Question 46.
If r: R: r₁ = 2 : 5 : 12. then prove that the triangle ¡s right angled at A.
Solution:
r : R : r₁ = 2 : 5 : 12 then r = 2k. R = 5k and r₁ = 12K
r₁ – r = 12k – 2k = 10k = 2(5k) = 2R
⇒ 4R sin \(\frac{A}{2}\)[cos \(\frac{B}{2}\) cos \(\frac{C}{2}\) – sin \(\frac{B}{2}\) sin \(\frac{C}{2}\)] = 2R
⇒ 2 sin\(\frac{A}{2}\) cos\(\left(\frac{B+C}{2}\right)\) = 1
⇒ 2 sin \(\frac{A}{2}\) = \(\frac{1}{2}\) [cos \(\left(\frac{B+C}{2}\right)\) = sin \(\frac{A}{2}\)]
⇒ sin \(\frac{A}{2}\) = \(\frac{1}{\sqrt{2}}\) = sin 45°
⇒ \(\frac{A}{2}\) = 45° ⇒ A = 90°
Hence the triangle is right angled at A.

Question 47.
Show that r + r₃ + r₁ – r₂ = 4R cos B.
Solution:
r + r₃

Question 48.
If A, A₁, A₂, A₃ are the areas of incircle and excircles of a triangle respectively, then prove that \(\frac{1}{\sqrt{A_{1}}}+\frac{1}{\sqrt{A_{2}}}+\frac{1}{\sqrt{A_{3}}}=\frac{1}{\sqrt{A}}\) .
Solution:
r, r₁, r₂, r₃ are the in radius and ex-radii of the circles whose areas are A, A₁, A₂, A₃ respectively, then
A = itr², A₁ = πr₁², A₂ = πr₂², A₃ = πr₃²

Question 49.
Show that (r₁ + r₂) sec² \(\frac{C}{2}\) = (r₂ + r₃) sec² \(\frac{A}{2}\) = (r₃ + r₁) sec² \(\frac{B}{2}\).
Solution:
r₁ + r₂ = 4R cos \(\frac{C}{2}\) [sin\(\frac{A}{2}\) cos\(\frac{B}{2}\) + cos \(\frac{A}{2}\) sin \(\frac{B}{2}\)]
= 4R cos\(\frac{C}{2}\) sin\(\left(\frac{A+B}{2}\right)\)
= 4R cos²\(\frac{C}{2}\) ___________ (1)
⇒ (r₁ + r₂) sec²\(\frac{C}{2}\) = 4R
Similarly, we can show that
(r₂ + r₃) sec²\(\frac{A}{2}\) = (r₃ + r₁) sec²\(\frac{B}{2}\) = 4R
∴ (r₁ + r₂)sec²\(\frac{C}{2}\) = (r₂ + r₃)
sec²\(\frac{A}{2}\) = (r₃ + r₁) sec²\(\frac{B}{2}\) = 4R

Question 50.
In ∆ABC, if AD, BE, CF are the perpendiculars drawn from the vertices A, B, C to the opposite sides, show that
i) \(\frac{1}{A D}+\frac{1}{B E}+\frac{1}{C F}=\frac{1}{r}\) and
ii) AD. BE. CF = \(\frac{(a b c)^{2}}{8 R^{3}}\)
Solution:
Since AD ⊥r to BC,

Question 51.
In ∆ ABC, if r₁ = 8, r₂ = 12, r₃ = 24, find a, b, c.
Solution:
∵ \(\frac{1}{r}\) = \(\frac{1}{r_{1}}\) + \(\frac{1}{r_{2}}\) + \(\frac{1}{r_{3}}\)
⇒ \(\frac{1}{r}\) = \(\frac{1}{8}\) + \(\frac{1}{12}\) + \(\frac{1}{24}\)
⇒ \(\frac{1}{r}\) = \(\frac{3+2+1}{24}\) ⇒ r = 4
But ∆² = rr₁r₂r₃ = 4 × 8 × 12 × 24
= (8 × 12)²
⇒ ∆ = 96 sq. cm
∵ r = \(\frac{\Delta}{\mathrm{s}}\) ⇒ 4 = \(\frac{96}{s}\) ⇒ s = 24
∵ r₁ = \(\frac{\Delta}{s-a}\)
⇒ δ = \(\frac{96}{s-a}\) ⇒ s – a = \(\frac{96}{8}\) ⇒ 24 – a
= 12 ⇒ a = 12
Similarly s – b = \(\frac{96}{12}\) ⇒ 24 – b = 8 ⇒ b = 16
s – c = \(\frac{96}{24}\) ⇒ 24 – c = 4 ⇒ c = 20

Question 52.
Show that \(\frac{a b-r_{1} r_{2}}{r_{3}}\) = \(\frac{b c-r_{2} r_{3}}{r_{1}}\) = \(\frac{c a-r_{3} r_{1}}{r_{2}}\)   [May 08; Mar 08]
Solution:

Students get through Maths 1A Important Questions Inter 1st Year Maths 1A Hyperbolic Functions Important Questions which are most likely to be asked in the exam.

Intermediate 1st Year Maths 1A Hyperbolic Functions Important Questions

Question 1.
Prove that for any x ∈ R, sinh (3x) = 3 sinh x + 4sinh x
Answer:
LHS = sinh (3x)
= sinh (2x + x)
= sinh (2x) . cosh(x) + cosh (2x) . sinh (x) = (2sinh x cosh x)cosh x (1 +2sinh² x)sinh x
= 2 sinh x (cosh² x) + (1 + 2 sinh² x) sinh x
= 2 sinh x (1 + sinh² x) + (1 + 2 sinh² x) sinh x
∵ cosh² x – sinh² x = 1
= 3 sinh x + 4 sinh³ x
∴ sinh (3x) = 3 sinh x + 4 sinh³ x

Question 2.
Prove that for any x ∈ R, tanh 3x = \(\frac{3 \tanh x+\tanh ^{3} x}{1+3 \tanh ^{2} x}\)
Answer:
tanh 3x = tan (2x + x)

Question 3.
If cosh x = \(\frac{5}{2}\), find the values of
i) cosh (2x) and
ii) sinh (2x)
Answer:
cosh (x) = \(\frac{5}{2}\)
(i) cosh (2x) = 2 cosh² (x) – 1
= 2(\(\frac{5}{2}\))² – 1 = \(\frac{25}{2}\) – 1 = \(\frac{23}{2}\)

ii) sinh² (2x) = cosh² (2x) – 1
= (\(\frac{23}{2}\))² – 1 = \(\frac{529-4}{2}\) = \(\frac{525}{4}\)
∴ sinh (2x) = ±\(\sqrt{\frac{525}{4}}\) = ±\(\frac{5 \sqrt{21}}{2}\)

Question 4.
If coshx = sec θ then prove that tan h²\(\frac{x}{2}\) = tan²\(\frac{\theta}{2}\)
Answer:
tan h²\(\frac{x}{2}\) = \(\frac{\cosh x-1}{\cosh x+1}\)
= \(\frac{\sec \theta-1}{\sec \theta+1}\) = \(\frac{1-\cos \theta}{1+\cos \theta}\) = tan²\(\frac{\theta}{2}\)

Question 5.
If θ ∈ (-\(\frac{\pi}{4}\), \(\frac{\pi}{4}\)) and x = log_e(cot(\(\frac{\pi}{4}\) + θ) then prove that
i) cosh x = sec 2θ and
ii) sinh x = -tan 2θ
Answer:

Question 6.
If sinh x = 5, show that x = log_e (5 + \(\sqrt{26}\))
Answer:
∴ sinh (x) = 5
⇒ x = sinh^-1 (5)
= log_e (5 + \(\sqrt{5^{2}+1}\))
= log_e (5 + \(\sqrt{26}\))
[sin^-1 (x) = log_e (x + \(\sqrt{x^{2}+1}\)) for all x ∈ R]

Question 7.
Show that tanh^-1(\(\frac{1}{2}\)) = \(\frac{1}{2}\) log_e3 (A.P) [Mar 15; May 07, 05; Mar 08, 05]
Answer:
∵tanh^-1 (x) = \(\frac{1}{2}\)log_e(\(\frac{1+x}{1-x}\)) for all x ∈ (-1, 1)
∵ tanh^-1 (\(\frac{1}{2}\)) = \(\frac{1}{2}\)log_e(\(\frac{1+\frac{1}{2}}{1-\frac{1}{2}}\))
= \(\frac{1}{2}\)log_e(3)