Category: Inter 1st Year

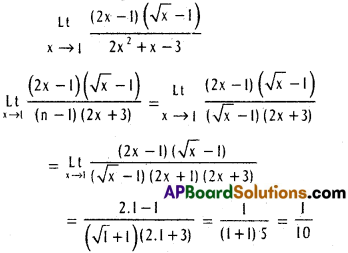
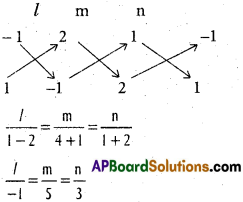
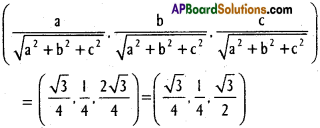
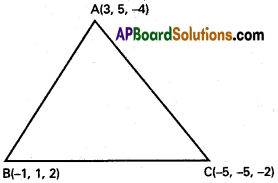
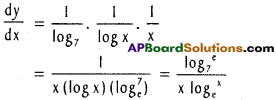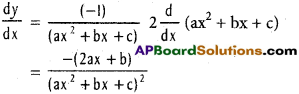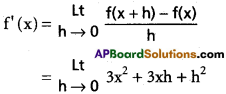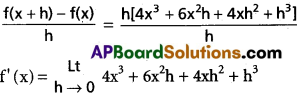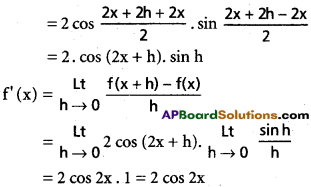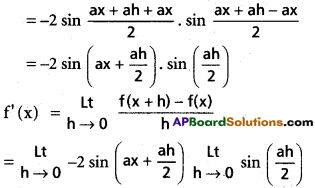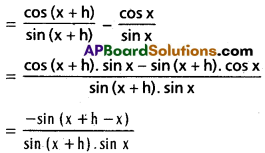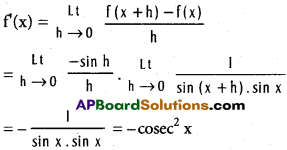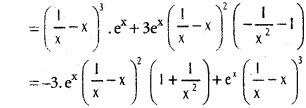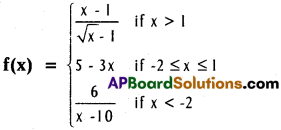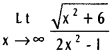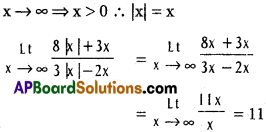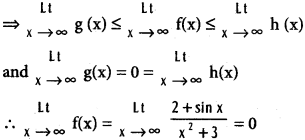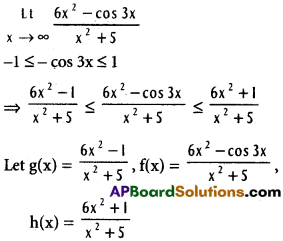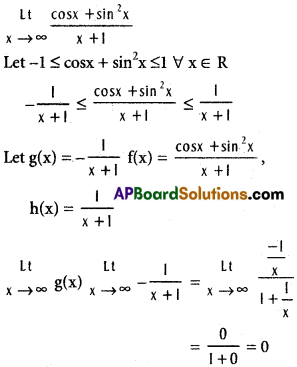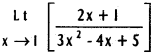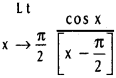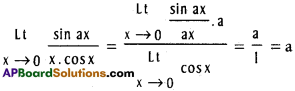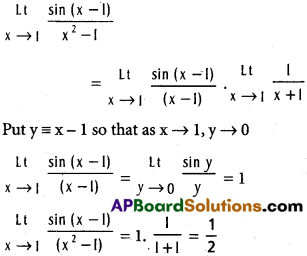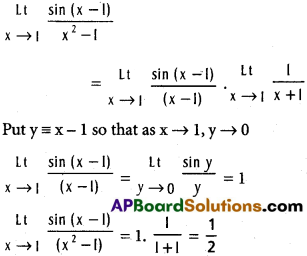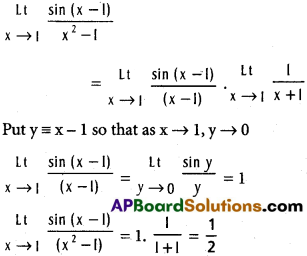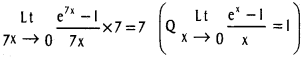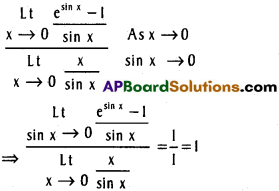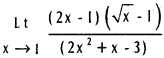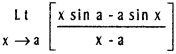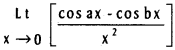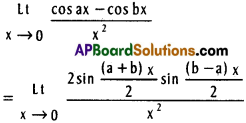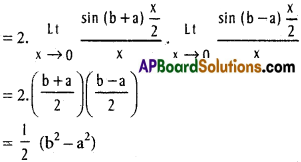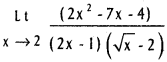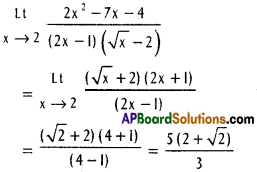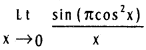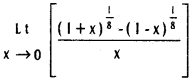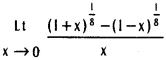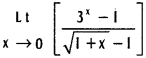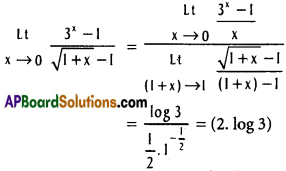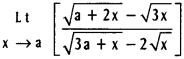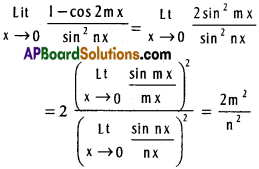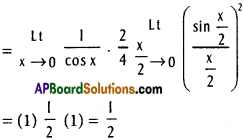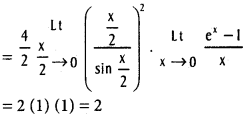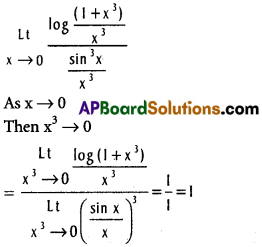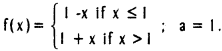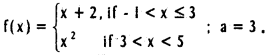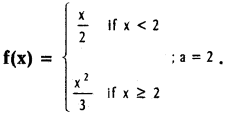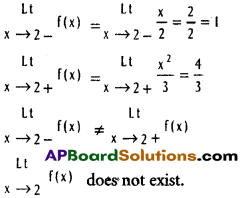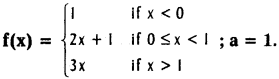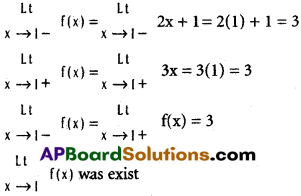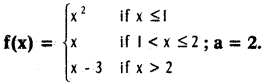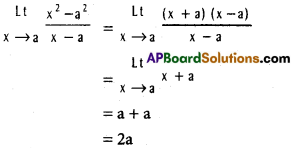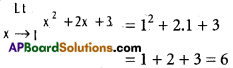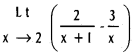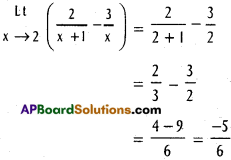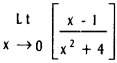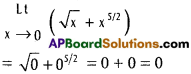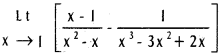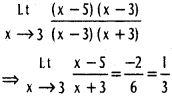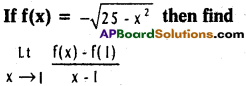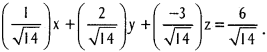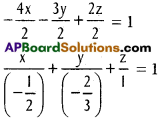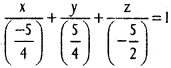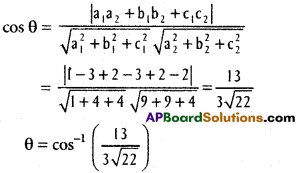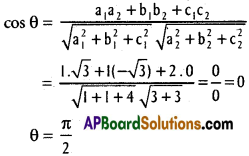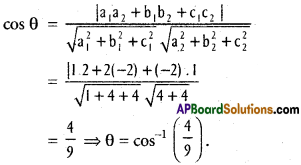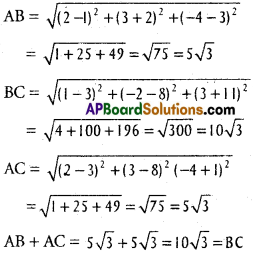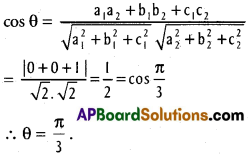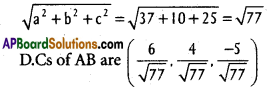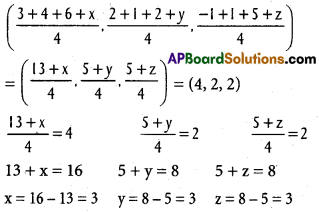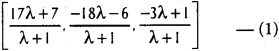Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Differentiation Solutions Exercise 9(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Differentiation Solutions Exercise 9(b)

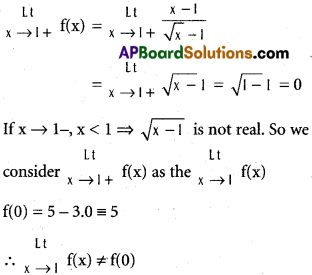
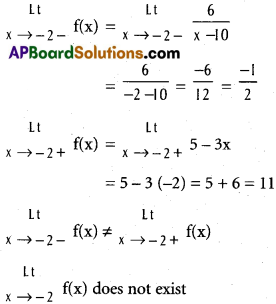
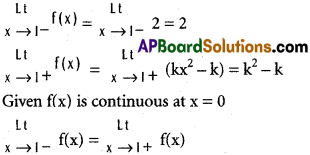
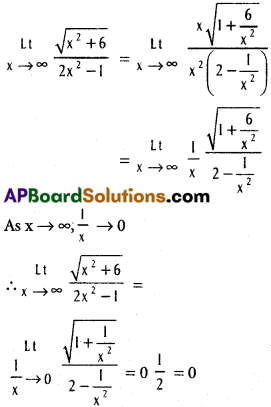
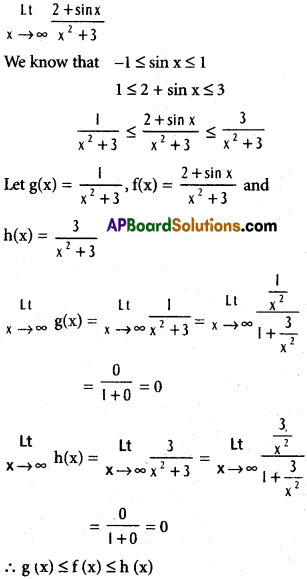
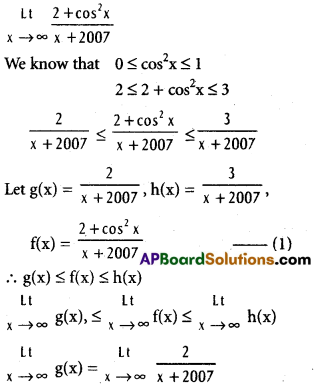
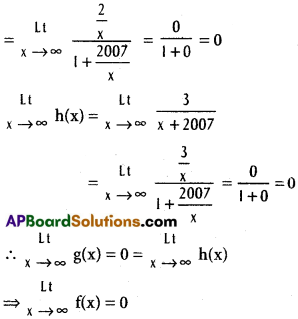
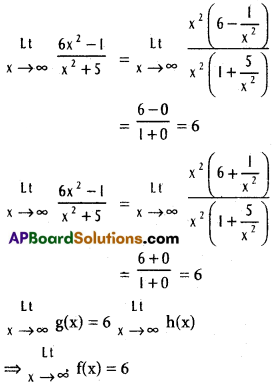
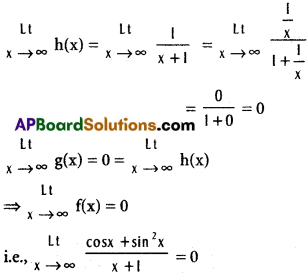
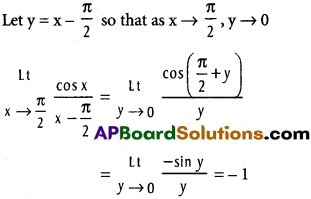
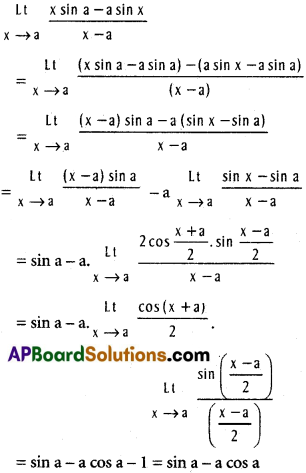
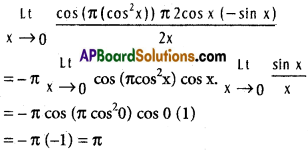
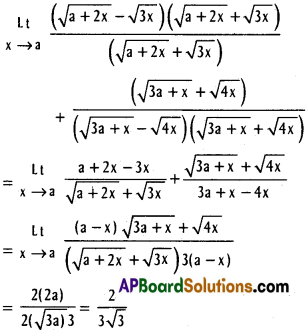
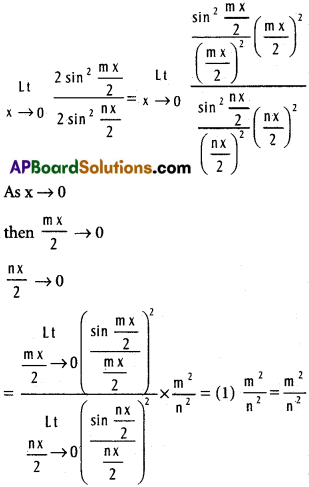
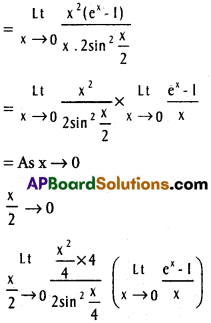
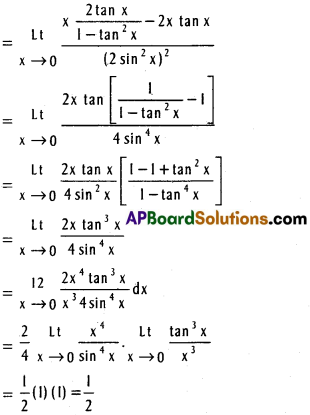
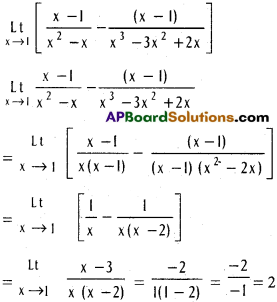
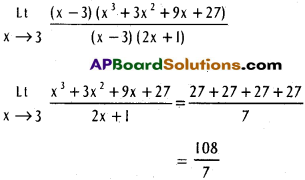
I. Compute the following limits.

Question 1.
Find the derivatives of the following function.
i) cotⁿ x
Solution:
\(\frac{dy}{dx}\) = n. cot^n-1 x. \(\frac{d}{dx}\) (cot x)
= n. cot^n-1 x (- cosec² x)
= – n. cot^n-1 x. cosec² x

ii) cosec⁴ x
Solution:
\(\frac{dy}{dx}\) = 4.cosec³ x. \(\frac{d}{dx}\)(cosec x)
= 4. cosec³ x (- cosec x. cot x)
= – 4. cosec⁴ x. cot x

iii) tan (e^x)
=sec 2(e^x).(e^x)¹
= e^x. sec² (e^x)

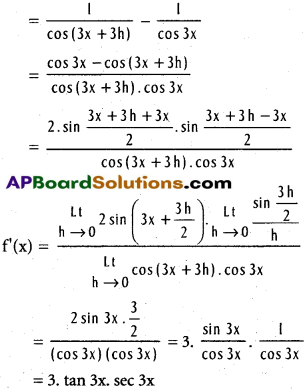
iv) \(\frac{1-\cos 2 x}{1+\cos 2 x}\)
Solution:
\(\frac{2 \sin ^{2} x}{2 \cos ^{2} x}\) = tan² x \(\frac{dy}{dx}\) = 2 tan x . sec² x

v) sin^m x cosⁿ x
Solution:
\(\frac{dy}{dx}\) = (sin^m x). \(\frac{d}{dx}\) (cosⁿ x) + (cosⁿ x) \(\frac{d}{dx}\) (sin^m x)
= sin^m xn + cos^n-1 x(-sin x) + cosⁿ x. m sin^m-1 x. cos x
= m. cosⁿ⁺¹ x. sin^m-1 x – n. sin^m+1 x. cos^n-1 x.

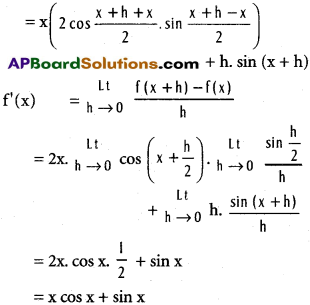
vi) sin mx. cos nx
Solution:
\(\frac{dy}{dx}\) = sin mx \(\frac{d}{dx}\) (cos nx) + (cos nx) \(\frac{dy}{dx}\) (sin mx)
= sin mx (-n sin nx)+cos nx (m cos mx)
= m. cos mx. cos nx – n. sin mx . sin nx

vii) x tan^-1 x
Solution:
\(\frac{dy}{dx}\) = x. \(\frac{d}{dx}\) (tan^-1 x) + (tan^-1 x) \(\frac{d}{dx}\) (x)
= \(\frac{x}{1+x^{2}}\) + tan^-1 x.

viii) sin^-1 (cos x)
Solution:
= sin^-1 [sin (\(\frac{\pi}{2}\) – x)] = \(\frac{\pi}{2}\) – x
\(\frac{dy}{dx}\) = 0 – 1 = -1

ix) log (tan 5x)
Solution:

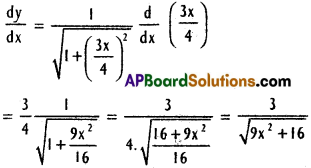
x) sinh^-1 (\(\frac{3x}{4}\))
Solution:

xi) tan^-1 (log x)
Solution:

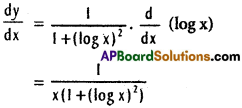
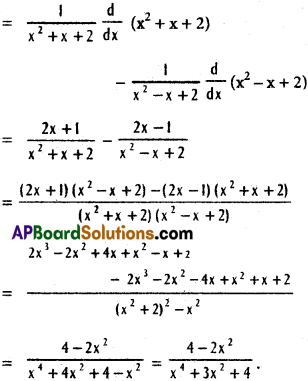
xii) log (\(\frac{x^{2}+x+2}{x^{2}-x+2}\))
Solution:
\(\frac{dy}{dx}\) = log(x² + x + 2) – log(x² – x + 2)

xiii) log (sin^-1 (e^x))
Solution:

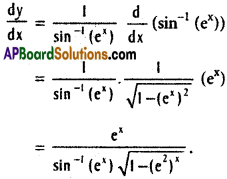
xiv) (sin x)² (sin^-1 x)²
Solution:

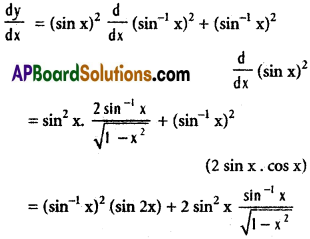
xv) y = \(\frac{\cos x}{\sin x+\cos x}\)
Solution:

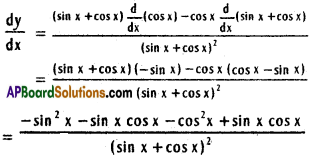
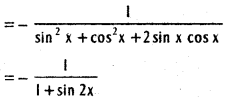
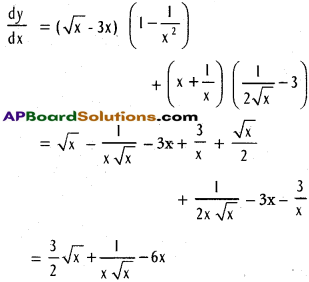
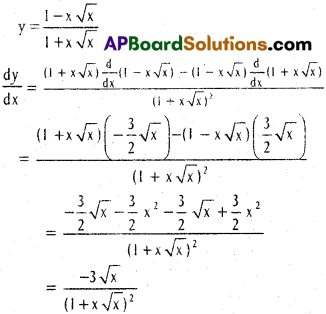
xvi) \(\frac{x\left(1+x^{2}\right)}{\sqrt{1-x^{2}}}\)
Solution:

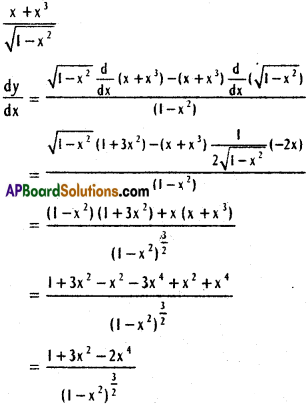
xvii) y = e^sin-1x
Solution:

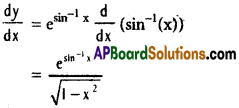
xviii) y = cos (log x + e^x)
Solution:
\(\frac{dy}{dx}\) = -sin(log x + e^x) = \(\frac{d}{dx}\) (log x + e^x)
= -sin (log x + e^x) (\(\frac{1}{x}\) + e^x)

xix) y = \(\frac{\sin (x+a)}{\cos x}\)
Solution:

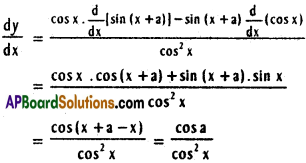
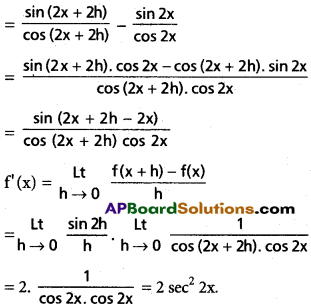
xx) y = cot^-1 (coses 3x)
Solution:

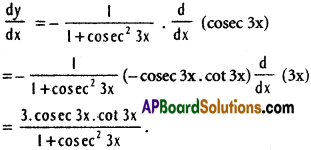
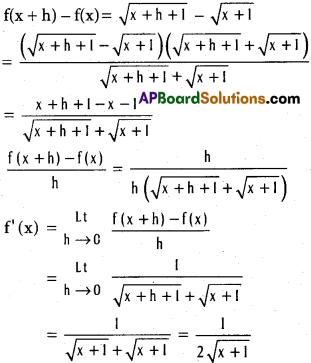
Question 2.
Find the derivatives of the following fountion.
i) x = sinh² y
Solution:
\(\frac{dy}{dx}\) = 2 sinh y . cosh y

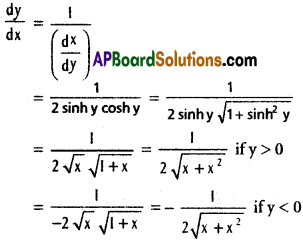
ii) x = tanh² y
Solution:
\(\frac{dy}{dx}\) = 2 tanh y . sech² y

iii) x = e^{sinh y}
Solution:
\(\frac{dy}{dx}\) = e^{sinh y} \(\frac{d}{dx}\) (sinh y)
= e^{sinh y} . cosh y
= x. cosh y
\(\frac{d y}{d x}=\frac{1}{\left(\frac{d x}{d y}\right)}=\frac{1}{x \cdot \cosh y}\)

iv) x =tan (e^-y)
Solution:
\(\frac{dy}{dx}\) = sec² (e^-y) . (e^-y)¹ = -e^-y . sec² e^-y
= -e^-y(1 + tan² (e^-y) = – e^-y(1 + x²)
\(\frac{d y}{d x}=\frac{1}{\left(\frac{d x}{d y}\right)}=-\frac{1}{e^{-y}\left(1+x^{2}\right)}=-\frac{e^{y}}{1+x^{2}}\)

v) x = log (1 + sin² y)
Solution:

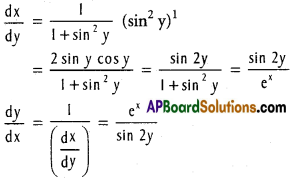
vi) x = log (1 + √y)
Solution:
1 + √y = e^x
√y = e^x – 1
y = (e^x – 1)²
\(\frac{dy}{dx}\) = 2(e^x – 1) . e^x = 2 √y . e^x
= 2 √y (√y + 1)
= 2(y + √y)

II. Find the derivativies of the following functions.

i) y = cos [log (cot x)]
Solution:

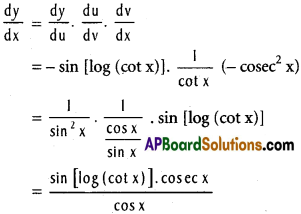
ii) y = sin^-1 \(\frac{1-x}{1+x}\)
Solution:

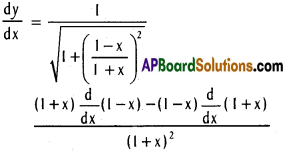
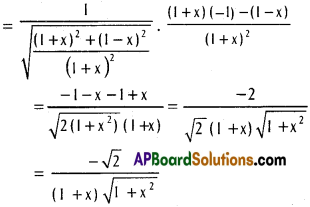
iii) log (cot (1 – x²))
Solution:

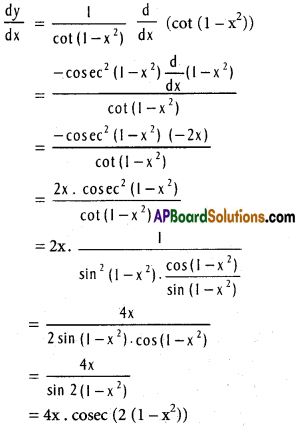
iv) y = sin [cos (x²)]
Solution:
\(\frac{dy}{dx}\) = cos [cos (x²)].\(\frac{d}{dx}\)[cos (x²)]
= cos [cos (x²)](sin (x²)).\(\frac{d}{dx}\)(x²)
= – 2x. sin (x²). cos [cos (x²)]

v) y = sin [tan^-1 (e^x)]
Solution:

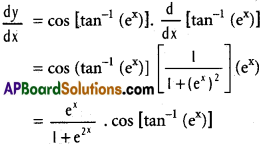
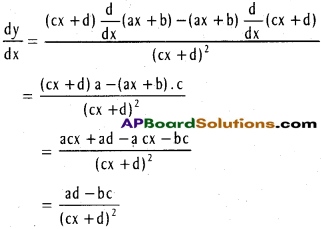
vi) y = \(\frac{\sin (a x+b)}{\cos (c x+d)}\)
Solution:

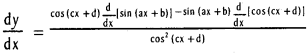
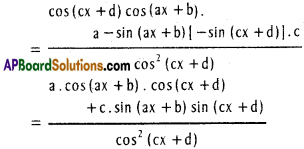
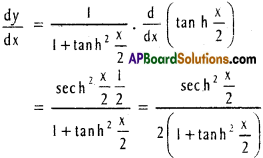
vii) y = tan^-1 (tan h \(\frac{x}{2}\))
Solution:

viii) y = sinx . (Tan^-1x)²
Solution:

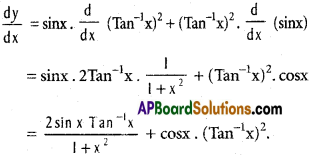
III. Find the derivatives of the following functions.

Question 1.
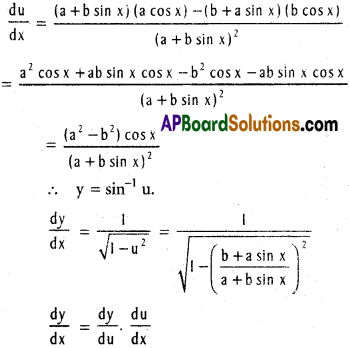
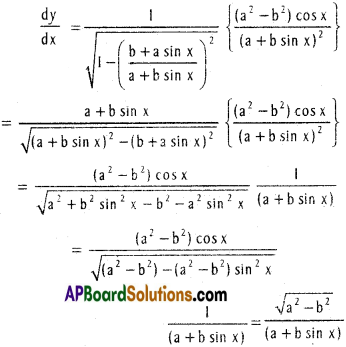
y = sin^-1 \(\left(\frac{b+a \sin x}{a+b \sin x}\right)\) (a > 0, b > 0)
Solution:
Let u = \(\frac{b+a \sin x}{a+b \sin x}\)

Question 2.
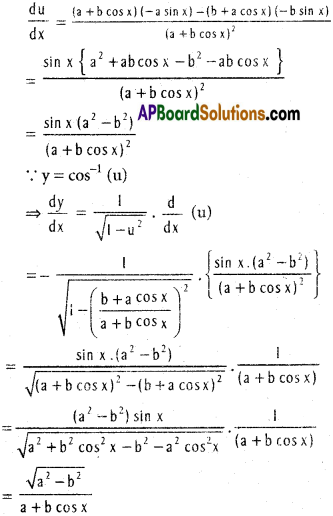
cos^-1\(\left(\frac{b+a \cos x}{a+b \cos x}\right)\) (a > 0, b > 0)
Solution:
Let u = \(\frac{b+a \cos x}{a+b \cos x}\)

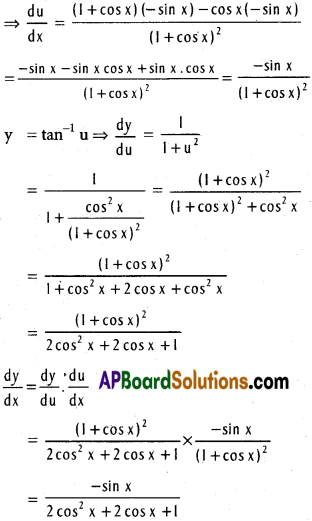
Question 3.
tan^-1 \(\left[\frac{\cos x}{1+\cos x}\right]\)
Solution:
Let u = \(\frac{\cos x}{1+\cos x}\)

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Differentiation Solutions Exercise 9(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Differentiation Solutions Exercise 9(a)

I. Compute the following limits.

Question 1.
Find the derivatives of the following functions f(x).
i) \(\sqrt{x}+2 x^{\frac{3}{4}}+3 x^{\frac{5}{6}}\) (x > 0)
Solution:
y = \(\sqrt{x}+2 x^{\frac{3}{4}}+3 x^{\frac{5}{6}}\) (x>0)
\(\frac{dy}{dx}\) = \(\frac{1}{2}\).x^-1/2 + 2.\(\frac{3}{4}\).x^-1/4 + 3.\(\frac{5}{6}\).^x-1/6 dx
= \(\frac{1}{2}\)[x^-1/2 + 3.x^-1/4 + 5.x^-1/6]

ii) \(\sqrt{2 x-3}+\sqrt{7-3 x}\).
Solution:

iii) (x² – 3) (4x³ + 1)
Solution:
y = (x² – 3) (4x³ + 1)
\(\frac{dy}{dx}\) = (x² – 3) \(\frac{d}{dx}\) (4x³ + 1) + (4x³ + 1) \(\frac{d}{dx}\)(x² – 3)
= (x² – 3) (12x²) + (4x³ + 1) (2x)
= 12x⁴ – 36x² + 8x⁴ + 2x
= 20x⁴ – 36x² + 2x

iv) (√x – 3x) (x + \(\frac{1}{x}\))
Solution:
y = (√x – 3x) (x + \(\frac{1}{x}\))

v) (√x + 1) (x² – 4x + 2) (x > 0)
Solution:
y = (√x + 1) (x² – 4x + 2) (x > 0)
Differentiating w.r.to x
\(\frac{dy}{dx}\) = (√x + 1) \(\frac{d}{dx}\)(x² – 4x + 2) + (x² – 4x + 2) \(\frac{d}{dx}\)(√x +1)
= (√x + 1) (2x – 4) + \(\frac{x^{2}-4 x+2}{2 \sqrt{x}}\)

vi) (ax + b)ⁿ. (cx + d)^m.
Solution:
y = (ax + b)ⁿ. (cx + d)^m
\(\frac{dy}{dx}\) = (ax + b)ⁿ \(\frac{d}{dx}\)(cx +d)^m + (cx + d)^m \(\frac{d}{dx}\)(ax + b)ⁿ
= (ax + b)ⁿ [m(cx + d)^m-1. c] + (cx + d)^m [n(ax + b)^n-1. a]
= (ax + b)^n-1 (cx + d)^m-1 [cm (ax + b) + an (cx + d)]
= (ax + b)ⁿ (cx + d)^m [\(\frac{an}{ax+b} + \frac{cm}{cx+d}\) ]

vii) 5 sin x + ex log x
Solution:
y = 5 sin x + e^x. log x
\(\frac{dy}{dx}\) = 5 cos x + e^x. \(\frac{d}{dx}\) (log x) + log x \(\frac{d}{dx}\)(e^x)
= 5 cos x + e^x. \(\frac{1}{x}\) + (log x) (e^x)

viii) 5^x + log x + x³ e^x
Solution:
y = 5^x + log x + x³ e^x
\(\frac{dy}{dx}\) = 5^x . log 5 + \(\frac{1}{x}\) + x³.e^x + e^x.3x²
= 5^x. log 5 + – + x³ e^x + 3x² e^x

ix) e^x + sin x cos x
Solution:
y = e^x + sin x . cos x
\(\frac{dy}{dx}\) = \(\frac{d}{dx}\) (e^x) + \(\frac{d}{dx}\) (sin x . cos x)
= e^x + sin x \(\frac{d}{dx}\) (cos x) + cos x \(\frac{d}{dx}\) (sin x)
= e^x – sin² x + cos² x
= e^x + cos 2x

x) \(\frac{p x^{2}+e x+r}{a x+b}\)(|a| + |b| ≠ 0)
Solution:

xi) log₇ (log x) (x > 0)
Solution:
y = log₇ (log x) (x > 0)

xii) \(\frac{1}{a x^{2}+b x+c}\) (|a| + |b| + |c| ≠ 0)
Solution:
\(\frac{1}{a x^{2}+b x+c}\) (|a| + |b| + |c| ≠ 0)

xiii) e^2x log (3x + 4) (x > –\(\frac{4}{3}\))
Solution:
y = e^2x. log (3x + 4) (x > –\(\frac{4}{3}\))
Differentiating w.r.to x
\(\frac{dy}{dx}\) = e^2x \(\frac{d}{dx}\)[log (3x + 4) + log (3x + 4) \(\frac{d}{dx}\) (e^2x)]
= e^2x.\(\frac{1}{3x+4}\) 3 + log (3x + 4). e^2x . 2
= e^2x (\(\frac{3}{3x+4}\) + 2 log (3x + 4))

xiv) (4 + x²) e²xy
Solution:
y = (4 + x²). e^2x
Differentiating w.r.to x
\(\frac{dy}{dx}\) = (4 + x²) \(\frac{d}{dx}\) (e^2x) + e^2x \(\frac{d}{dx}\)(4 + x²)
= (4 + x²). 2e^2x + e^2x (0 + 2x)
= 2e^2x [4 + x² + x]
= 2e^2x (x² + x + 4)

xv) \(\frac{ax+b}{cx+d}\) [|c| + |d|≠0]
Solution:
y = \(\frac{ax+b}{cx+d}\) [|c| + |d|≠0]
Differentiating w.r.to x

xvi) a^x. e^x²
Solution:
y = a^x. e^x²
Differentiating w.r.to x
\(\frac{dy}{dx}\) = (a^x) \(\frac{d}{dx}\)(e^x²) + (e^x²)\(\frac{d}{dx}\)(a^x)
= a^x. e^x². 2x + e^x². a^x. log a
= ax. ^x² (2x + log a)
= y(2x + log a)

Question 2.
If f(x) = 1 + x + x² + + x¹⁰⁰, then find f’ (1).
Solution:
f'(x) = 1 + 2x + 3x² + 100 x⁹⁹
f'(1) = 1+2 + 3 ….+ 100
= \(\frac{100 \times 101}{2}=5050\left(\Sigma x=\frac{x(x+1)}{2}\right)\)

Question 3.
If f (x) = 2x² + 3x – 5, then prove that f(0) + 3f (-1) = 0.
Solution:
f'(x) = 4x + 3
f'(0) = 0 + 3 = 3
f'(-1) = – 4 + 3 = -1
f'(0) + 3f'(-1) = 3 + 3(-1) = 3 – 3 = 0 n.

II.

Question 1.
Find the derivatives of the following functions from the first principles.
i) x³
Solution:


= 3x² + 0 + 0 = 3x²

ii) x⁴ + 4
Solution:
f(x + h) – f(x) = ((x + h)⁴ + 4) – (x⁴ + 4)
= (x + h)4 + 4 – x⁴ – 4 .
= x⁴ + 4x³h + 6x²h² + 4xh³ + h⁴ – x⁴

= 4x³ + 0 + 0 + 0 = 4x³

iii) ax² + bx + c
Solution:
f(x + h) = a(x + h)² + b(x + h) + c
= a(x² + 2hx + h²) + b(x + h) + c
= ax² + 2ahx + ah² + bx + bh +c

f(x + h) – f(x) = ax² + 2ahx + ah² + bx + bh + c – ax² – bx – c
= h [2ax + ah + b]

= 2ax + 0 + b = 2ax + b

iv) \(\sqrt{x+1}\)
Solution:

v) sin 2x
Solution:
f(x + h) – f(x) = sin 2(x + h) – sin 2x

vi) cos ax
Solution:
f(x + h) – f(x) = cos a (x + h) – cos ax

= – 2 sin ax. \(\frac{a}{2}\)
=-a. sin ax

vii) tan 2x
Solution:
f(x + h) – f(x) = tan 2(x + h) – tan 2x

viii) cot x
Solution:
f(x + h) – f(x) = cot (x + h) – cot x

ix) sec 3x
Solution:
f(x + h) – f(x)= sec 3(x + h) – sec 3x

x) x sin x
Solution:
f(x + h) – f(x) = (x + h) sin (x + h) – x sin x
= x (sin (x + h) – sin x) + h. sin (x + h)

xi) cos² x
Solution:
f(x + h) – f(x) = cos² (x + h) – cos² x
= -(cos² x – cos² (x + h))
= -sin (x + h + x) sin (x + h – x)

= -sin 2x. 1 = -sin 2x

Question 2.
Find the derivatives of the following function.
i) \(\frac{1-x \sqrt{x}}{1+x \sqrt{x}}\) (x > 0)
Solution:

ii) xⁿ n^x log (nx) (x > 0, n ∈ N)
Solution:
y = xⁿ. n^x. log nx
\(\frac{dy}{dx}\) = xⁿ. n^x (log nx) + nⁿ. log xn (xⁿ) + xⁿ. log nx (n^x)
= xⁿ. n^x \(\frac{n}{log nx}\) + n^x. log xⁿ (nx^n-1) + xⁿ . log nx. (n^x . log nx)
= x^n-1. n^x[\(\frac{nx}{log nx}\) + log nx. (nⁿ. log nx)]

iii) ax²ⁿ. log x + bxⁿ e^-x
Solution:
y = ax²ⁿ. log x + bxⁿ e^-x
\(\frac{dy}{dx}\) = a (x²ⁿ.\(\frac{1}{x}\) + log x (2nx^2n-1)) + b n (-e^-x) + e^-x. nx^n-1)
= a. x^2n-1 + 2an. x^2n-1. log x – bxⁿ e^-x + bn. x^n-1 . e^-x

iv) (\(\frac{1}{x}\) – x)³ e^x
Solution:
y = (\(\frac{1}{x}\) – x)³ . e^x
\(\frac{dy}{dx}\) = (\(\frac{1}{x}\) – x)³ \(\frac{d}{dx}\)(e^x) + e^x \(\frac{d}{dx}\){(\(\frac{1}{x}\) – x)³}

Question 3.
Show that the function f(x) = |x| + |x – 1|, x ∈ R is differentiable for all real numbers except for 0 and 1.
Solution:
f(x) = |x| + |x – 1| ∀ x ∈ R
f(x) = x + x – 1 = 2x – 1, x ≥ 1
= x – (x – 1) = x – x + 1, = 1, 0 < x < 1
= -x – (x – 1) =-x – x + 1 = 1 – 2x, x < 0 ∴ f(x) = 2x – 1, x > 1
= 1, 0 < x < 1 = 1 – 2x, x ≤ 0 If x > 1, then f(x) = 2x – 1 = polynomial in x f(x) is differentiable for all x > 1
If 0 < x < 1, then f(x) = 1 – constant
∴ f(x) is differentiable if 0 < x < 1.
If x < 1, then f(x) = 1 – 2x = polynomial in x.
∴ f(x) is differentiable for all x < 1

Case (i) : x = 0

R f'(0) ≠ Lf'(0)
∴ f'(0) does not exist.
f(x) is not differentiable at x = 0.

Case (ii): x = 1

R f'(1) ≠ L f'(1)
f(1) does not exist.
f(x) is not differentiable at x = 1
∴ f(x) is differentiable for all real x except zero and one.

Question 4.
Verify whether the following function is differentiable at 1 and 3.

Solution:
Case (i): x = 1

R f'(1) ≠ L f'(1)
f(x) is not differentiable at x = 1

Case (ii) : x = 3

f(3⁺) ≠ f'(3^–)
f(x) is not differentiable at x = 3.

Question 5.
Is the following function f derivable at 2?

Solution:

f'(2^–) ≠ f(2⁺); f(x) is not derivable at x = 2.

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(e) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(e)

I.

Question 1.
Is the function f, defined by \(f(x)=\left\{\begin{array}{l}
x^{2} \text { if } x \leq 1 \\
x \text { if } x>1
\end{array}\right.\) continuous on R?
Solution:

f is continuous at x = 1
f is continuous on R.

Question 2.
Is f defined by f(x) = \(=\left\{\begin{array}{cc}
\frac{\sin 2 x}{x}, & \text { if } x \neq 0 \\
1 & \text { if } x=0
\end{array}\right.\) continuous at 0?
Solution:

f is not continuous at 0

Question 3.
Show that the function f(x) = [cos (x¹⁰ + 1)]^1/3, x ∈ R is a continuous function.
Solution:
We know that cos x is continuous for every x ∈ R
∴ The given function f(x) is continuous for every x ∈ R.

II.

Question 1.
Check the continuity of the following function at 2.

Solution:

f(x) is not continuous at 2.

Question 2.
Check the continuity of f given by f(x) = \(\begin{cases}\frac{\left[x^{2}-9\right]}{\left[x^{2}-2 x-3\right]} & \text { if } 0<x<5 \text { and } x \neq 3 \\ 1.5 & \text { if } x=3\end{cases}\) at the point 3.
Solution:

f(x) is continuous at x = 3.

Question 3.
Show that f, given by f(x) = \(\frac{x-|x|}{x}\) (x ≠ 0) is continuous on R – {0}.
Solution:
Case (i) : a > 0 ⇒ |a| = a

If x = 0, f(a) is not defined
f(x) is not continuous at ’0′
∴ f(x) is continuous on R – {0}

Question 4.
If f is a function defined by

then discuss the continuity of f.
Solution:
Case (i) : x = 1

f(x) is not continuous at x > 1

Case (ii) : x = -2

f(x) is not continuous at x = -2.

Question 5.
If f is given by f(x) = \(=\left\{\begin{array}{cl}
k^{2} x-k & \text { if } x \geq 1 \\
2 & \text { if } x<1
\end{array}\right.\) is a continuous function on R, then find the values of k.
Solution:

2 = k² – k
k² – k – 2 = 0
(k – 2) (k + 1) = 0
k = 2 or – 1

Question 6.
Prove that the functions ‘sin x’ and ‘cos x’ are continuous on R.
Solution:
i) Let a ∈ R

∴ f is continuous at a.

ii) Let a ∈ R

∴ f is continuous at a.

III.

Question 1.
Check the continuity of ‘f given by

at the points 0, 1 and 2.
Solution:
i)
∴ f(x) is continuous at x = 0

ii)
∴ f(x) is continuous at x = 1

iii)
∴ f(x) is continuous at x = 2

Question 2.
Find real constant a, b so that the function f given by

is continuous on R.
Solution:

Since f(x) is continuous on R
LHS = RHS ⇒ a = 0

Since f(x) is continuous on R.
LHS = RHS
3b + 3 = -3
3b = – 6 ⇒ b = -2

Question 3.
Show that

where a and b are real constants, is continuous at 0.
Solution:

∴ f(x) is continuous at x = 0

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(d) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(d)

I. Compute the following limits.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.

Solution:

Question 6.

Solution:

II.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.

Solution:

Question 6.

Solution:

Question 7.

Solution:

Question 8.

Solution:

III.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.

Solution:

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(c) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(c)

I. Computer the following limits.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.

Solution:

Question 6.

Solution:

Question 7.

Solution:
As x → 0
⇒ 7x → 0

Question 8.

Solution:

Question 9.

Solution:

Question 10.

Solution:

II.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.

Solution:

Question 6.

Solution:
By L. Hospital rule

III.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.

Solution:

Question 6.

Solution:

Question 7.

Solution:

Question 8.

Solution:

Question 9.

Solution:

Question 10.

Solution:

Question 11.

Solution:

Question 12.

Solution:

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(b)

Find the right and left-hand limits of the functions in 1,2,3 of I and 1,2, of II at the point mentioned against them. Hence, check whether the functions have limits at those a’s.

I.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

II.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:
x → 2 – ⇒ x < 2
x – 2 < 0
|x – 2| = – (x – 2)

Question 4.

Solution:
x → 0 + ⇒ x > 0
|x| = x

Question 5.

Solution:

Question 6.

Solution:

III.

Question 1.

Solution:

∴ LHL ≠ RHL
Limit does not exists.

Question 2.

Solution:

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Limits and Continuity Solutions Exercise 8(a)

I. Compute the following limits.

Question 1.

Solution:

Question 2.

Solution:

Question 3.

Solution:

Question 4.

Solution:

Question 5.

Solution:

Question 6.

Solution:

Question 7.

Solution:

Question 8.

Solution:

Question 9.

Solution:

Question 10.

Solution:

Question 11.

Solution:

Question 12.

Solution:

Question 13.

Note : Text Book answer will come, when the problem changes like.
Solution:

Question 14.

Solution:

Question 15.

Solution:

Question 16.

Solution:

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B The Plane Solutions Exercise 7(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B The Plane Solutions Exercise 7(a)

I.

Question 1.
Find the equation of the plane if the foot of the perpendicular from origin to the plane is (1, 3, -5).
Solution:
OP is the normal to the plane D. Rs of op are 1, 3, -5
The plane passes through P( 1, 3, -5) equation of the plane is
1(x – 1) + 3(y – 3) – 5(z + 5) = 0
x – 1 + 3y – 9 – 5z – 25 = 0
x + 3y – 5z – 35 = 0

Question 2.
Reduce the equation x + 2y – 3z – 6 = 0 of the plane to the normal form.
Solution:
Equation of the plane is x + 2y – 3z – 6 = 0
i.e., x + 2y – 3z = 6
Dividing in the
\(\sqrt{1^{2}+2^{2}+(-3)^{2}}=\sqrt{1+4+9}=\sqrt{14}\)
The equation of the plane in the normal form is

Question 3.
Find the equation of the plane. Whose intercepts on X, Y, Z – axis are 1, 2, 4 respectively.
Solution:
Equation of the plane in the intercept form is
\(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) = 1
Given a = 1, b = 2, c = 4.
Equation of the required plane in the intercept form is
\(\frac{x}{1}+\frac{y}{2}+\frac{z}{4}\) = 1
Multiplying with 4, we get
4x + 2y + z = 4

Question 4.
Find the intercepts of the plane 4x + 3y – 2z + 2 = 0 on the co-ordinate axes.
Solution:
Equation of the plane is 4x + 3y – 2z + 2 = 0
– 4x – 3y + 2z = 2

x – intercept = -1/2, y – intercept = -2/3, z intercept =1.

Question 5.
Find the d.c.’s of the normal to the plane x + 2y + 2z – 4 = 0.
Solution:
Equation of the plane is x + 2y + z- 4 = 0
d.r.’s of the normal are (1, 2, 2)
Dividing with \(\sqrt{1+4+4}\) = 3,
d.c.’s of the normal to the plane are (\(\frac{1}{3}, \frac{2}{3}, \frac{2}{3}\))

Question 6.
Find the equation of the plane passing through the point (-2, 1, 3) and having (3, -5, 4) as d.r.’s of its normal.
Solution:
d.r.’s of the normal are (3, -5, 4) and the plane passes through (-2, 1, 3).
Equation of the plane is 3(x + 2) – 5(y – 1) + 4(z – 3) = 0
3x + 6 – 5y + 5 + 4z – 12 = 0
3x -5y+ 4z – 1 = 0.

Question 7.
Write the equation of the plane 4x – 4y + 2z + 5 = 0 in the intercept form.
Solution:
Equation of the plane is
4x – 4y + 2z + 5 = 0
– 4x + 4y – 2z = 5
\(-\frac{4x}{5}, \frac{4y}{5}, \frac{2z}{5}\)
Intercept form is

x – intercept = \(\frac{5}{4}\), y – intercept = \(\frac{5}{4}\), z – intercept = –\(\frac{5}{2}\)

Question 8.
Find the angle between the planes x + 2y + 2z-5 = 0 and 3x + 3y + 2z – 8 = 0.
Solution:
Equation of the planes are
x + 2y + 2z – 5 = 0
3x + 3y + 2z – 8 = 0

II.

Question 1.
Find the equation of the plane passing through the point (1,1,1) and parallel to the plane x +.2y + 3z – 7 = 0.
Solution:
Equation of the given plane is x + 2y + 3z – 7 = 0.
Equation of the parallel plane is x + 2y + 3z = k.
This plane passes through the point P (1, 1, 1)
1 + 2 + 3 = k ⇒ k = -6
Equation of the required plane is x + 2y + 3z = 6

Question 2.
Find the equation of the plane passing through (2, 3, 4) and perpendicular to x – axis.
Solution:
The plane is perpendicular to x – axis
∴ x – axis is the normal to the plane
d.c.’s of x -axis are 1, 0, 0
Equation of the required plane is x = k
This plane passes through the point P(2, 3, 4)
∴ 2 = k
Equation of the required plane is x = 2.

Question 3.
Show that 2x + 3y + 7 = 0 represents a plane perpendicular to XY – plane.
Solution:
Equation of the given plane is 2x + 3y + 7 = 0
Equation of xy – plane is z = 0
a₁a₂ + b₁b₂ + c₁c₂ = 2.0 + 3.0 + 0.1 = 0
+0+0=0
The plane 2x + 3y + 7 = 0 is perpendicular to XY – plane.

Question 4.
Find the constant k so that the planes x – 2y + kz = 0 and 2x + 5y – z = 0 are at right angles. Find the equation of the plane through (1, -1, -1) and perpen-dicular to these planes.
Solution:
Equations of the given planes are x – 2y + kz = 0 and 2x + 5y – z = 0
These the planes are perpendicular
1.2 – 2.5 + k (-1) = 0
2 – 10 = k ⇒ k = -8
Equation of the planes
x – 2y – 8z = 0 ………. (1)
2x + 5y – z = 0 ……….. (2)
The required plane passes through (1, -1, -1)
∴ Equation of the plane can be taken as
a(x – 1) + b(y + 1) + c(z + 1) = 0 ………… (3)
This plane is perpendicular to the planes (1) and (2)
a – 2b – 8c = 0
2a + 5b – c = 0

Substituting in (3), equation of the required planes
42 (x – 1) – 15(y + 1) + 9(z + 1) = 0
42x – 42 – 15y – 15 + 9z + 9 = 0
42x – 15y + 9z – 48 = 0.

Question 5.
Find the equation of the plane through (-1, 6, 2) and perpendicular to the join of (1, 2, 3) and (-2, 3, 4).
Solution:
The plane is perpendicular to the line joining A(1, 2, 3) and B(-2, 3, 4).
d.r.’s of AB are 1 + 2, 2 – 3, 3 – 4
i.e., 3, -1, -1

AB is normal to the plane and the plane passes through the point P(-1, 6, 2)
Equation of the required plane is 3(x + 1) – 1(y – 6) -1(z – 2) = 0
3x + 3 – y + 6- z + 2 = 0
3x – y – z + 11 = 0

Question 6.
Find the equation of the plane bisecting the line segment joining (2, 0, 6) and (-6, 2, 4) and perpendicular to it.
Solution:
A (2, 0, 1), B(-6, 2, 4) are the given points ‘o’ is the mid point of AB

Co-ordinates of O are
\(\left(\frac{2-6}{2}, \frac{0+2}{2}, \frac{6+4}{2}\right)\) = (-2, 1, 5)
The plane is perpendicular to AB
d.r.’s of the normal to the plane are
2 + 6, 0 – 2, 6 – 4
8, -2, 2
Equation of the required plane is
+8 (x + 2) – 2(y – 1) + 2 (z – 5) = 0
8x + 16 – 2y + 2 + 2z – 10 = 0
8x – 2y + 2z + 8 = 0

Question 7.
Find the equation of the plane passing through (0, 0, -4) and perpendicular to the line joining the points (1, -2, 2) and (-3, 1, -2).
Solution:
A(1, -2, 2), B(-3, 1, -2) are the given points
d.r.’s of AB are 1 + 3, -2 -1, 2 + 2 i.e., 4, -3, 4
AB is normal to the plane and the plane passes through the point P(0, 0, -4).
Equation of the required plane is
4(x – 0) – 3(y – 0) + 4(z + 4) = 0
4x – 3y + 4z + 16 = 0

Question 8.
Find the equation of the plane through (4, 4, 0) and perpendicular to the planes 2x + y + 2 z + 3 = 0 and 3x + 3y + 2z – 8 = 0.
Solution:
Equation of the plane passing through P(4, 4, 0) is
a(x – 4) + b(y – 4) + c(z – 0) = 0 ………. (1)
This plane is perpendicular to
2x + y + 2z – 3 = 0
3x + 3y + 2z – 8 = 0
∴ 2a + b + 2c = 0 ………… (2)
3a + 3b + 2c = 0 ………….(3)

Substituting in (1), equation of the required plane is
-4 (x – 4) + 2(y – 4) + 3(z – 0) = 0
-4x + 16 + 2y – 8 + 3z = 0
-4x + 2y + 3z + 8 = 0

III.

Question 1.
Find the equation of the plane through the points (2, 2, -1), (3, 4, 2), (7, 0, 6).
Solution:
A(2, 2, -1), B(3, 4, 2), C(7, 0, 6) are the given points.
Equation of the plane passing through A(2, 2, -1) is
a(x – 2) + b(y – 2) + c(z + 1) = 0
This plane passes through B(3, 4, 2) and C(7, 0, 6)
a(3 – 2) + b(4 – 2) +c(2 + 1) = 0
a + 2b + 3c = 0 ……….. (2)
a(7 – 2) + b(0 – 2) + c(6 + 1) = 0
5a – 2b + 7c = 0 ……….. (3)
From (2) and (3)

Substituting in (1) equation of the required plane is
5(x- 2) + 2(y- 2) – 3(z +1) = 0
5x – 10 + 2y – 4 – 3z – 3 = 0
5x + 2y – 3z -17 = 0 or 5x + 2y- 3z = 17

Question 2.
Show that the points (0, -1, 0), (2, 1, -1), (1, 1, 1), (3, 3, 0) are coplanar.
Solution:
Equation of the plane through A(0, -1, 0) is
ax + b(y + 1) + cz = 0 …….. (1)
This plane passes through B(2, 1, -1) and C(1, 1, 1)
2a + 2b – c = 0 …………. (2)
a + 2b + c = 0 ………….. (3)
(2) – (3) gives a – 2c = 0 ⇒ a = 2c ⇒ \(\frac{a}{2}=\frac{c}{1}\)
(2) + (3) gives 3a + 4b = 0 ⇒ 3a = – 4b
⇒ \(\frac{a}{4}=\frac{b}{-3}\)
∴ \(\frac{a}{4}=\frac{b}{-3}=\frac{c}{2}\)
Substitutes in (1) equation of the plane ABC is
4x – 3(y + 1) + 2(z – 0) = 0
4x – 3y + 2z – 3 = 0
4x – 3y + 2z – 3 = 4.3 – 3.3. + 0.3
= 12 – 9 – 3 = 0
The given points A, B, C, D are coplanar.

Question 3.
Find the equation of the plane through (6, – 4, 3), (0, 4, -3) and cutting of inter-cepts whose sum is zero.
Solution:
Suppose a, b, c are the intercepts of the plane.
Equation of the plane is \(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) = 1
Given a + b + c = 0
c = – (a + b)
The plane passes through
P(6, -4, 3), Q(0, 4, -3)

c = -a – b = -3 – b
4(-3 – b) – 3b = b(-3 – b)
-12 – 4b – 3b = – 3b – b²
b² – 4b – 12 = 0
(b – 6) (b + 2) = 0 ⇒ b = 6 – 2

Case i) b = 6
c = -3 – b = -3 – 6 = -9
Equation of the plane is
\(\frac{x}{3}+\frac{y}{6}+\frac{z}{9}\) = 1
6x + 3y – 2z = 18

Case ii) b = -2
c = -3 – b = -3 + 2 = -1
Equation of the plane is
\(\frac{x}{3}+\frac{y}{-2}+\frac{z}{-1}\) = 1

Question 4.
A plane meets the co-ordinate axes in A, B, C. If the centroid of ∆ABC is (a, b, c). Show that the equation of the plane is
\(\frac{x}{a}+\frac{y}{b}+\frac{z}{c}\) = 1
Solution:
Suppose α, β, γ are the intercepts of the plane ABC.
Equation of the plane is the intercept form is
\(\frac{x}{\alpha}+\frac{y}{\beta}+\frac{z}{\gamma}=1\) ………… (1)

Co-ordinates of A are (α, 0, 0), B are (0, β, 0) and C are (0, 0, γ)
G is the centroid of ∆ABC
Co-ordinates of Gare =

α = 3a, β = 3b, γ = 3c
Substituting in (1), equation of the plane ABC is

Question 5.
Show that the plane through (1, 1, 1) , (1, -1, 1) and (-7, -3, -5) is parallel . to Y-axis.
Solution:
Equation of the plane through A (1, 1, 1) can be taken as
a(x – 1) +b(y – 1) + c(z – 1) = 0 ……….. (1)
This plane passes through B(1, -1, 1) and C(-7, -3, -5)
0 – 2b + 0 = 0 ⇒ b – 0
Equation of zy – plane is y = 0
0.x + 1.y + 0.z = 0
a.0 + 0.1 + c.0 = 0
The required plane is perpendicular to zx – plane hence it is parallel to Y – axis.

Question 6.
Show that the equations ax + by + r =0, by + cz + p = 0, cz + ax + q = 0 represent planes perpendicular to xy,yz, zx planes respectively.
Solution:
Equation of the given plane is
ax + by + c = Q
d.rs of the normal are (a, b, c)
Equation of XY – plane is z = 0
d.rs of the normal are (0, 0, 1)
a.0 + b.0 + 0.1 = 0
∴ ax + by + r = 0 is perpendicular to xy – plane.
Similarly we can show that by + cz + p = 0 is perpendicular to yz – plane and cz + ax + q – 0 is perpendicular to zx – plane.

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Direction Cosines and Direction Ratios Solutions Exercise 6(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Direction Cosines and Direction Ratios Solutions Exercise 6(b)

I.

Question 1.
Find the direction ratios of the line joining the points (3, 4, 0) and (4, 4, 4).
Solution:
A(3, 4, 0) and B(4, 4, 4) are the given points.
d.rs of AB are (x₂ – x₁, y₂ – y₁, z₂ – z₁)
(4-3, 4-4, 4-0) i.e.,(1, 0, 4)

Question 2.
The direction ratios of a line are (-6, 2, 3). Find its direction cosines.
Solution:
D.Rs of the line are -6, 2, 3
Dividing with \(\sqrt{36+4+9}\) = 7
Direction cosines of the line are – \(\frac{6}{7}, \frac{2}{7}, \frac{3}{7}\)

Question 3.
Find the cosine of the angle between the lines whose direction cosines are (\(\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\)) and (\(\frac{1}{\sqrt{2}}, \frac{1}{\sqrt{2}}\), 0)
Solution:
cos θ = l₁ + l₂ + m₁ m₂ + n₁ n₂

Question 4.
Find the angle between the lines whose direction ratios are (1, 1, 2) (√3, -√3, 0).
Solution:

Question 5.
Show that the lines with direction cosines (\(\frac{12}{13}, \frac{-3}{13}, \frac{-4}{13}\)) and (\(\frac{4}{13}, \frac{12}{13}, \frac{3}{13}\)) are perpendicular to each other.
Solution:
If two lines, are perpendicular, then
l₁l₂ + m₁m₂ + n₁n₂ = 0
l₁l₂ + m₁m₂ + n₁n₂
\(\frac{12}{13} \cdot \frac{4}{13}-\frac{3}{13} \cdot \frac{12}{13}-\frac{4}{13} \cdot \frac{3}{13}\)
∴ The given lines are perpendicular.

Question 6.
O is the origin, P(2, 3, 4) and Q(1, k, 1) are points such that \(\overline{\mathrm{O P}}\) ⊥ \(\overline{\mathrm{O Q}}\). Find k.
Solution:
d.rs of OP and 2, 3,4
d.rs of OQ are 1, k, 1
OP and OQ are perpendicular
⇒ a₁a₂ + b₁b₂ + c₁c₂ = 0
2 + 3k + 4 = 0
3k = -6
k = -2.

II.

Question 1.
If the direction ratios of a line are (3, 4, 0), find its direction cosines are also the angles made with the co-ordinate axes.
Solution:
Direction ratios of the line are (3, 4, 0)
Dividing with \(\sqrt{9+16+0}\) = 5
D.C’s of the line are (\(\frac{3}{5}, \frac{4}{5}\), 0)
If α_c, βc² are the angles made By the line with the co-ordinate axes, then
cos α = \(\frac{3}{5}\) cos β = \(\frac{4}{5}\) cos γ = 0
α = cos^-1\(\frac{3}{5}\), β = cos^-1\(\frac{4}{5}\), γ = \(\frac{\pi}{2}\)
Angles made with co-ordinate axes are
cos^-1\(\frac{3}{5}\), cos^-1\(\frac{4}{5}\), γ = \(\frac{\pi}{2}\)

Question 2.
Show that the line through the points (1, -1,2) (3, 4, -2) is perpendicular to the line through the points (0, 3, 2) and (3, 5, 6).
Solution:
A(1, -1 2) B(3, 4, -2) C(0,3,2) and D(3, 5,6) are the given points
d.rs of AB are 3 – 1, 4 + 1, -2 -2 i.e., 2, 5, -4
d.rs of CD are 3 – 0, 5 – 3, 6 – 2 i.e., 3, 2 4
a₁a₂ + b₁b₂ + c₁c₂ = 2.3 + 5.2 – 4.4
= 6 + 10 – 16 = 0
AB and CD are perpendicular.

Question 3.
Find the angle between \(\overline{\mathrm{D C}}\) and \(\overline{\mathrm{A B}}\) where A = (3, 4, 5), B =(4, 6, 3) C = (-1, 2, 4) are D(l, 0, 5).
Solution:
A(3, 4, 5), B(4, 6, 3), C(-1, 2, 4), D(1, 0, 5) are the given points.
d.rs of AB are 4 – 3, 6 – 4, 3 – 5 i.e., 1, 2, -2
d.rs of CD are 1 + 1, 0 – 2, 5 – 4 i.e., 2, -2, 1

Question 4.
Find the direction cosines of a line which is perpendicular to the lines, whose direction ratios are (1, -1, 2) and (2, 1, -1).
Solution:
Let l, m, n be the d.cs of the required line it is perpendicular to the lines with
d.rs (1, -1, 2) and (2, 1, -1)
l – m + 2n = 0
2l + m – n = 0

d.rs of the line are – 1, 5, 3
Dividing with \(\sqrt{1+25+9} =\sqrt{35}\)
d.cs of the required line are ’
– \(\frac{1}{\sqrt{35}}, \frac{5}{\sqrt{35}}, \frac{3}{\sqrt{35}}\)

Question 5.
Show that the points (2, 3, -4), (1, -2, 3) and (3, 8, -11) are collinear.
Solution:
A(2, 3, -4),B( 1, -2, 3) and C(3, 8, -11) are the given points.

∴ A, B, C are collinear.

Question 6.
Show that the points (4, 7, 8), (2, 3, 4), (-1, -2,1), (1,2,5) are vertices of apare- llelogram.
Solution:
A(4, 7, 8), B(2, 3, 4), C(-1, -2, 1) and D (1, 2, 5) . are the given points.

∴ AB = CD and BC = DA
∴ A, B, C, D are the vertical of parallelogram.

III.

Question 1.
Show that the lines whose direction co – sines are given by l + m + n = 0, 2mn + 3nZ – 5Zm = 0 are perpendicular to each other.
Solution:
Given l + m + n = 0 ………… (1)
2mn + 3nl – 5lm = 0 …………… (2)
From (1), l = -(m + n)
Substituting in (2)
2mn – 3n(m + n) + 5m(m + n) = 0
2mn – 3mn – 3n2 + 5m2 + 5mn = 0
5m² + 4mn – 3n² = 0
\(\frac{m_{1} m_{2}}{n_{1} n_{2}}=-\frac{3}{5} \Rightarrow \frac{m_{1} m_{2}}{-3}=\frac{n_{1} n_{2}}{5}\) ………. (1)
From (1), n = -(l + m).
Substituting is (2)
-2m (l + m) – 3l(l + m) – 5lm = 0
– 2lm – 2m2 – 3l² – 3lm – 5lm = 0
3l² + 10lm + 2m² = 0
\(\frac{l_{1} l_{2}}{m_{1} m_{2}}=\frac{2}{3} \Rightarrow \frac{l_{1} l_{2}}{2}=\frac{m_{1} m_{2}}{3}\) ……… (2)
From (1) and (2) we get
\(\frac{I_{1} I_{2}}{2}=\frac{m_{1} m_{2}}{3}=\frac{n_{1} n_{2}}{-5}=1\)
l₁l₂ = 2k, m₁m₂ = 3k, n₁ n₂ = -5k
∴ l₁l₂ + m₁m₂ + n₁n₂ = 2k + 3k – 5k = 0
The two lines are perpendicular.

Question 2.
Find the angle between the lines whose direction cosines satisfy the equations l + m + n = 0, l² + m² – n² = 0.
Solution:
Given l + m + n = 0
l² + m² – n² = 0
From (1), l = – (m + n)
Substituting in (2)
(m + n)² + m² – n² = 0
m² + n² + 2mn + m² – n² = 0
2m² + 2mn = 0
2m(m + n) = 0
∴ m = 0 or m + n = 0

Case (i) : m = 0, Substituting in (1) l + n = 0
l = -n ⇒ \(\frac{l}{1}\) = \(\frac{n}{-1}\)
D.Rs of the first line l₁ are (1, 0, – 1)

Case (ii) :m + n = 0 ⇒ m = -n ⇒ \(\frac{m}{1}\) = \(\frac{n}{-1}\)
Substituting in (1) l = 0
D.Rs of the second line l₂ are (0, 1 – 1)
Suppose θ is the angle between the two lines

Question 3.
If a ray makes angles α, α, γ and δ with the four diagonals of a cube find cos² α + cos² β + cos² γ + cos² δ.
Solution:
Let each side of the cube be of length a. Let one of the vertices of the cube be the origin O and the co-ordinate axes be along the three edges \(\overline{\mathrm{O A}}\), \(\overline{\mathrm{O B}}\) and \(\overline{\mathrm{O C}}\) passing through the origin. The co-ordinates of the vertices of the cube with respect to the frame of reference OABC are as shown in figure. The diagonals of he cubeare \(\overline{\mathrm{O P}}\) \(\overline{\mathrm{C D}}\), \(\overline{\mathrm{A E}}\) and \(\overline{\mathrm{B F}}\) .(a, a, a), (a, a, -a), (-a, a, a) and (a, -a, a) are direction . ratios of these diagonals respectively.

Let the direction cosines of the given ray be (l, m, n). If this fay makes the angles α, β, γ and δ with the four diagonals of the cube, then

cos² α + cos² β + cos² γ + cos² δ
\(\frac{1}{3}\){|l + m + n|² +|l + m – n|² + |-l + m + n|² + |l – m + n|²}
\(\frac{1}{3}\)[(l + m + n)² + (l + m – n)² + (-l + m + n)² + (l – m + n)²]
\(\frac{1}{3}\)[4(l² + m² + n²)] = \(\frac{4}{3}\) (since l² + m² + n² = 1)

Question 4.
If (l₁, m₁, n₁), (l₂, m₂, n₂) are d.c.s of two intersecting lines, show that d.c.s of two lines, bisecting the angles between them are proportional to l₁ ± l₂, m₁ ± m₂ n₁ ± n₂.

Solution:
OA and OB are the given lines
A and B are points at unit distances from O
Co-ordinates of A are (l₁, m₁, n₁)
Co-ordinates of B are (l₂, m₂, n₂)
P is the mid point of AB
Co-ordinates of P are \(\left(\frac{l_{1}+l_{2}}{2}, \frac{m_{1}+m_{2}}{2}, \frac{n_{1}+n_{2}}{2}\right)\)
∴ OP is the bisector of ∠AOB
D.Rs of OP are l₁ + l₂, m₁+ m₂, n₁+ n₂
Suppose B’ is a point are OB such that OB’ OB = 1
Co-ordinates of B’ are (-l₂, -m₂, -n₂)
Q is the mid point of AB’
Co-ordinates of Q are \(\left(\frac{l_{1}-l_{2}}{2}, \frac{m_{1}-m_{2}}{2}, \frac{n_{1}-n_{2}}{2}\right)\)
OQ is the other bisector of ∠AOB
D.Rs of OQ are l₁ – l₁, m₁ – m₂, n₁ – n₂

Question 5.
A (-1, 2, -3), B(5, 0, -6), C(0, 4, -1) are three points. Show that the direction cosines of the bisectors of ∠BAC are propotional to (25, 8, 5) and (-11, 20, 23).
Solution:
A (-1, 2, -3), B (5, 0, -6) and C (0, 4, -1) are the given points.
D.Rs of AB are 5 +1, 0 -2, -6 +3 ie., 6, -2, -3
D.Rs of AB are \(\frac{6}{7}, \frac{-2}{7}, \frac{-3}{7}\)
D.Rs of AC are 0 + 1, 4 – 2, -1 + 3 i.e., 1, 2, 2
D.Rs of AC are \(\frac{1}{3}, \frac{2}{3}, \frac{2}{3}\)
D.Rs of one of the bisectors are

D.Rs of one of the bisectos are (25, 8, 5)
D.Rs of the other bisectors are

D.Rs of the second bisector are (-11, 20, 23)

Question 6.
If (6,10,10), (1, 0, -5), (6, -10, 0) are vertices of a triangle, find the direction ratios of its sides. Determine whether it is right angled or isosceles.
Solution:
A (6, 10, 10), B (1, 0, -5), C (6, -10, 0) are the vertices of ∆ABC
D.Rs of AB are 5, 10, 15 i.e., 1, 2, 3
D.Rs of BC are -5, 10, -5 i.e., 1, -2, 1
D.Rs of AC are 0,20,10, i.e., 0, 2, 1

∴ The given triangle is right angle a.

Question 7.
The vertices of a triangle are A (1, 4, 2), B (-2, 1, 2) C (2, 3, -4). Find ∠A, ∠B, ∠C.

Solution:
A (1, 4, 2), B (-2, 1, 2), C (2, 3, -4) and the vertices of OABC.
D.Rs of AB are 3, 3, 0 i.e., 1, 1, 0
D.Rs of BC are -4, -2, 6 i.e., 2, 1, -3
D.Rs of AC are -1, 1, 6.

Question 8.
Find the angle between the lines whose direction cosines are given by the equation 3l + m + 5n = 0 and 6mn – 2nl + 5lm = 0
Solution:
Given 3l + m + 5n = 0 ……….. (1)
6mn – 2nl + 5lm = 0 …………. (2)
From (1), m = – (3l + 5n)
Substituting in (2)
-6n(3l + 5n) -2nl – 5l(3l + 5n) = 0
-18ln – 30n² – 2nl – 15l² – 25ln = 0
-15l² – 45ln – 30n² = 0
l² + 3ln + 2n² = 0
(l + 2n) (l + n) — 0
l + 2n = 0 or l + n = 0

Case (i) : l₁ + n₁ = 0 ⇒ n₁ = – l₁; ⇒ \(\frac{l_{1}}{1}=\frac{n_{1}}{-1}\)
But m₁ = -(3l₁ + 5n₁) = -(-3n₁ + 5n₁) = -2n₁
∴ \(\frac{m_{1}}{+2}=\frac{n_{1}}{-1}\)
∴ \(\frac{l_{1}}{1}=\frac{m_{1}}{2}=\frac{n_{1}}{-1}\)
D.Rs of the first line lx are (1,2, -1)
Case (ii): l₂ + 2n₂ = 0
l₂ = —2n₂ ⇒ \(\frac{l_{2}}{-2}=\frac{n_{2}}{1}\)
m₂ = -(3l₂ + 5n₂) = – (-6n₂ + 5n₂) = n₂
\(\frac{m_{2}}{1}=\frac{n_{2}}{1}\)
∴ \(\frac{l_{2}}{-2}=\frac{m_{2}}{1}=\frac{n_{2}}{1}\)
D.Rs of the second line Z2 are (-2, 1, 1)
Suppose ‘θ’ is the angle between the lines l₁ and l₂

Question 9.
If a variable line in two adjecent positions has direction cosines (l, m, n) and (l + δl, m + δm, n + δn), show that the small angle δθ between two position. Is given by (δθ)² = (δl)² + (δm)² + (δn)².
Solution:
Since (l, m, n) and (l + δl, m + δm, n + δn) are direction cosines, we have
l² + m² + n² = 1 …………. (1)
(l + δl)² + (m + δm)² + (n + δn)² — 1 ………. (2)
(2) – (1) gives (l + δl)² + (m + δm)² + (n + 8n)² – (l² + m² + n²) = 0
2(l.δl + m.δm + n.δn) = -((δl)² + (δm)² + (δn)²) …………. (3)
cos θ . δθ = l(l + δl) + m(m + δm) + n(n + δn)
= (l² + m² + n²) + (l.δl + m.δm + n.δn)
= 1 – \(\frac{1}{2}\) [(δl)² + (δm)² + (δn)²]
(δl)² + (δm)² + (δn)² = 1 = ² (1 – cos θ.δ θ)
δθ being small, sin \(\frac{\delta \theta}{2}\) = \(\frac{\delta \theta}{2}\)
∴ sin²θ \(\frac{\delta \theta}{2}\) = 4(\(\frac{\delta \theta}{2}\))² = (δθ)²
∴ (δθ)² = (δl)² + (δm)² + (δn)².

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Direction Cosines and Direction Ratios Solutions Exercise 6(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Direction Cosines and Direction Ratios Solutions Exercise 6(a)

I.

Question 1.
A line makes angles 90°, 60° and 30° with positive directions of X, Y, Z – axes respectively. Find the direction cosines.
Solution:
Suppose l, m, n are the direction cosines of the line.
l = cos α = cos 90° = 0
m = cos β = cos 60° = \(\frac{1}{2}\)
n = cos γ = cos 30° = \(\frac{\sqrt{3}}{2}\)
Direction cosines of the line are (0, \(\frac{1}{2}\), \(\frac{\sqrt{3}}{2}\))

Question 2.
If a line makes angles α, β, γ with the positive direction of x, y, z axes, what is the value of sin² α + sin² β + sin² γ?
Solution:
We know that cos² α + cos² β + cos² γ = 1
1 – sin² α + 1 – sin² β + 1 – sin² γ = 1
sin² a + sin² P + sin² γ = 3 – 1 = 2.

Question 3.
If P(√3, 1, 2√3) is a point in space, find the direction cosines of \(\overrightarrow{O P}\).
Solution:
Direction ratios of P are (√3, 1, 2√3)
a² + b² + c² = 3 + 1 + 12 = 16
⇒ \(\sqrt{a^{2}+b^{2}+c^{2}}\) = 4
Direction cosines of \(\overrightarrow{O P}\) are

Question 4.
Find the direction cosines of the line joining the points (-4, 1, 7) are (2, -3, 2).
Solution:
A(- 4, 1, 2) and B(2, -3, 2) are the given points
d.rs of PQ are (x₂ – x₁, y₂ – y₁, z₂ – z₁)
(2 + 4, 1 + 3, 2 – 7) ie., (6, 4, -5)
Dividing with

II.

Question 1.
Find the direction cosines of the sides of the triangle whose vertices are (3, 5, -4), (-1, 1, 2) and (-5, -5, -2).
Solution:
A(3, 5, -4), B(-1, 1, 2) and C(-5, -5, -2) are the vertices of ∆ABC

d.rs of AB are (-1 -3, 1 – 5, 2 + 4) (-4, -4, 6)
Dividing with \(\sqrt{16+16+36}=\sqrt{68}=2 \sqrt{17}\)
D.Rs of AB are \(\frac{-4}{2 \sqrt{17}}, \frac{-4}{2 \sqrt{17}}, \frac{6}{2 \sqrt{17}}\)
ie., \(\frac{-2}{\sqrt{17}}, \frac{-2}{\sqrt{17}}, \frac{3}{\sqrt{17}}\)
D.Rs of BC are (-5 + 1, -5 -1, -2 -2)
i.e., (-4, -6, -4)

Dividing with \(\sqrt{16+16+36}=\sqrt{68}=2 \sqrt{17}\)
d.cs of BC are \(\frac{-4}{2 \sqrt{17}}, \frac{-6}{2 \sqrt{17}}, \frac{-4}{2 \sqrt{17}}\)
ie., \(\frac{-2}{\sqrt{17}}, \frac{-3}{\sqrt{17}}, \frac{-2}{\sqrt{17}}\)

d.rs of CA are 3 + 5, 5 + 5, -4 + 2
= 8, 10, -2
Dividing with \(\sqrt{64+100+4}=\sqrt{168}=2 \sqrt{42}\)
d.cs of CA are \(\frac{8}{2 \sqrt{42}}, \frac{10}{2 \sqrt{42}}, \frac{-2}{2 \sqrt{42}}\)
ie., \(\frac{4}{\sqrt{42}}, \frac{5}{\sqrt{42}}, \frac{-1}{\sqrt{42}}\)

Question 2.
Show that the lines \(\stackrel{\leftrightarrow}{P Q}\) and \(\stackrel{\leftrightarrow}{R S}\) are parallel where P, Q, R, S are two points (2, 3, 4), (4, 7, 8), (-1, -2, 1) and (1, 2, 5) respectively.
Solution:
P(2, 3, 4), Q(4, 7, 8), R(-1, -2, 1)
and S(1, 2, 5) are the given points.
d.rs of PQ are 4 -2, 7 -3, 8 – 4 i.e., 2, 4, 4
d.rs of RS are 1 + 1, 2 + 2, 5 – 1 i.e., 2, 4, 4
d.rs of PQ are RS are proportional
∴ PQ and RS are parallel.

III.

Question 1.
Find the direction cosines of two lines which are connected by the relations l – 5m + 3n = 0 and 7l² + 5m² – 3n² = 0.
Solution:
Given l – 5m + 3n = 0
⇒ l = 5m – 3n ………….. (1)
7l² + 5m² – 3n² = 0 …………. (2)
Substituting the value of l in (2)
7(5m – 3n)² + 5m² – 3n² = 0
7(25m² + 9n² – 30 mn) + 5m² – 3n² = 0
175 m² + 63n² – 210 mn + 5m² – 3n² = 0
180m² – 210mn + 60n² = 0
Dividing with 30.
6m² – 7mn + 2n² = 0
(3m – 2n) (2m – n) = 0
3m = 2n or 2m = n

Case (i): 3m₁ = 2n₁ ⇒ \(\frac{m_{1}}{2}=\frac{n_{1}}{3}\)
and m₁ = \(\frac{2}{3}\) n₁
From (1) l₁ = 5m₁ – 3n₁ = \(\frac{10}{3}\)n₁ – 3n₁
d.rs of the first line are (1, 2, 3)
Dividing with \(\sqrt{1+4+9}=\sqrt{14}\)
d.cs of the first line are (\(\frac{1}{\sqrt{14}}, \frac{2}{\sqrt{14}}, \frac{3}{\sqrt{14}}\))

Case (ii): 2m₂ = n₂
From (1) l₂ – 5m₂ + 3n₂ = 0
l₂ – 5m₂ + 6m₂ = 0
l₂ = m₂
∴ \(\frac{l_{2}}{-1}=\frac{m_{2}}{1}=\frac{n_{2}}{2}\)
d.rs of the second line are -1, 1, 2
Dividing with \(\sqrt{1+1+4}=\sqrt{6}\)
d.cs of the second line are (\(\frac{-1}{\sqrt{6}}, \frac{1}{\sqrt{6}}, \frac{2}{\sqrt{6}}\))

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Three Dimensional Coordinates Solutions Exercise 5(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Three Dimensional Coordinates Solutions Exercise 5(b)

I.

Question 1.
Find the ratio in which the XZ-plane divides the line joining A(-2, 3, 4) and B(l, 2,3).
Solution:
Ratio in which XZ plane divides
AB = -y₁ : y₂
= -3 : 2

Question 2.
Find the co-ordinates of the vertex ‘C’ of ∆ABC if its centroid is the origin and the vertices A, B are (1, 1, 1) and (-2, 4, 1) respectively.
Solution:
A(1, 1, 1) B(-2, 4, 1) and (x, y, z) are the vertices of ∆ABC.
G is the centroid of ∆ABC
Co-ordinates of G are
(\(\frac{1-2+x}{3}\), \(\frac{1+4+y}{3}\), \(\frac{1+1+z}{3}\)) = (0, 0, 0)
\(\frac{x-1}{3}\) = 0, \(\frac{y+5}{3}\) = 0, \(\frac{z+2}{3}\) = 0
x – 1 = 0, y +5 =0, z + 2 = 0
x = 1, y = – 5, z = -2
∴ Co-ordinates of c are (1, -5, -2)

Question 3.
If (3, 2, -1), (4, 1, 1) and (6, 2, 5) are three vertices and (4, 2, 2) is the centroid of a tetrahedron, find the fourth vertex.
Solution:
A(3, 2, -1), B(4, 1, 1), C(6, 2, 5), D(x, y, z) are the vertices of the tetrahedron.
Co-ordinates of the centroid G are

Co-ordinates of D are (3, 3, 3)

Question 4.
Find the distance between the mid point of the line segment \(\overline{\mathrm{A B}}\) and the point (3, -1, 2) where A = (6, 3, -4) and B = (-2, -1, 2).
Solution:
A(6, 3, – 4) B(-2, -1,2) are the given points.
Q is the midpoint of AB.
Co-ordinates of Q are (\(\frac{6-2}{2}\), \(\frac{3-1}{2}\), \(\frac{-4+2}{2}\)) = (2, 1, -1)
Co-ordinates of P are (3, -1, 2)

II.

Question 1.
Show that the points (5, 4,2) (6,2, -1) and (8, -2, -7) are collinear.
Solution:
A(5, 4, 2), B(6, 2, -1) c(8, -2, -7) are the given points.
AB = \(\sqrt{(5-6)^{2}+(4-2)^{2}+(2+1)^{2}}\)

∴ A, B, C are collinear.

Question 2.
Show that the points A(3, 2, -4), B(5, 4, -6) and C(9, 8, -10) are collinear and find the ratio in which B divides \(\overline{\mathrm{A C}}\).
Solution:
A(3, 2, -4), B(5, 4, -6) and C(9, 8, -10) are the given points.

A, B, C are collinear
Ratio in which B divides AC = AB : BC
= 2√3 : 4√3 = 1 : 2

III.

Question 1.
If A(4, 8, 12) B(2, 4, 6) C(3, 5, 4) and D(5, 8, 5) are four points, show that the line \(\stackrel{\leftrightarrow}{A B}\) and \(\stackrel{\leftrightarrow}{C D}\) intersect.
Solution:
A(4, 8, 12), B(2, 4, 6) C(3, 5, 4) and D(5, 8, 5) are the given points
Co-ordinates of the point dividing AB in the ratio λ : 1 is
\(\left[\frac{2 \lambda+4}{\lambda+1} \cdot \frac{4 \lambda+8}{\lambda+1} \cdot \frac{6 \lambda+12}{\lambda+1}\right]\) ……….. (1)
Co-ordinates of the point dividing CD in the ratio p : 1 is
\(\left[\frac{5 \mu+3}{\mu+1}, \frac{8 \mu+5}{\mu+1}, \frac{5 \mu+4}{\mu+1}\right]\) ……….. (2)
If the given lines intersects these two points must coincide.
\(\frac{2 \lambda+4}{\lambda+1}=\frac{5 \mu+3}{\mu+1}\)
(2λ + 4) (µ + 1) = (5µ + 3) (λ + 1)
2λµ + 2λ + 4µ + 4 = 5λµ + 5µ + 3λ + 3
3λ + λ + µ – 1 = 0
λ(3µ+1) = -(µ – 1)
λ = –\(\frac{(\mu-1)}{3 \mu+1}\)
\(\frac{4 \lambda+8}{\lambda+1}=\frac{8 \mu+5}{\mu+1}\)
(4λ + 8) (µ + 1) = (8µ + 5) (λ + 1)
4λµ + 4λ + 8µ + 8 = 8λµ + 8µ + 5λ + 5
4Aµ + λ – 3 = 0
(4µ + 1) λ = 3
\(-\frac{(4 \mu+1)(\mu-1)}{3 \mu+1}=3\)
4µ2 – 4µ + µ – 1 = -9µ – 3
4µ2 4- 6µ + 2 = 0
2µ2 + 3µ + 1=0
(2µ + 1) (µ – 1) = 0
µ = – \(\frac{1}{2}\) or – 1
µ = -1 is not possible
µ = – \(\frac{1}{2}\)

Co-ordinates of (2) are

Co-ordinates of (1) are
\(\frac{-6+4}{-3+1}, \frac{-12+8}{-3+1}, \frac{-18+12}{-3+1}\) = (1, 2, 3)
Since these two points coincide the given lines intersect.

Question 2.
Find the point of instersection of the lines \(\stackrel{\leftrightarrow}{A B}\) and \(\stackrel{\leftrightarrow}{C D}\) where A = (7, -6, 1) B = (17, -18, -3), C = (1, 4, -5) and D = (3, -4, 11)

Solution:
A(7, -6, 1), B(17, -18, -3), C(1, 4, -5) and D(3, -4, 11) are the given points.
Co-ordinates of the point dividing AB in the ratio λ: 1 are

Co-ordinates of the point dividing CD in the ratio µ : 1 are

(17λ + 7) (µ + 1) = (3µ + 1) (λ + 1)
17λµ + 17λ + 7µ + 7 = 3λµ + 3µ + λ + 1
14λµ + 16λ + 4µ + 6 = 0.

-18λµ – 6µ – 18λ – 6
= -4λµ + 4λ – 4µ + 4
14λµ + 22λ + 2µ + 10 = 0
14λµ + 16λ + 4µ + 6 = 0 ………. (1)
14λµ + 22λ + 2µ + 10 = 0……….(2)
Subtraction – 6λ + 2µ – 4 = 0
2µ = 6λ + 4
µ = 3λ + 2
Substituting in (3)
14λ(3λ + 2) + 16λ + 4(3λ + 2) + 6 = 0
42λ² + 28λ + 16λ + 12λ+ 8 + 6 = 0
42λ² + 56λ + 14 = 0
3λ² + 4λ + 1 = 0
(λ + 1) (3λ + 1) = 0
λ = -1 or λ = –\(\frac{1}{3}\)
λ = -1 is not possible
λ = –\(\frac{1}{3}\)

µ = 3λ + 2 = -1 + 2 = 1
µ = 1 ⇒
\(\frac{3+1}{1+1}, \frac{-4+4}{1+1}, \frac{11-5}{1+1}\) = (2, 0, 3)
∴ These two points coincide
⇒ The given lines AB and CD intersect
Point of intersection is (2, 0, 3)

Question 3.
A(3, 2, 0), B(5, 3, 2), C(-9, 6, -3) are vertices of a triangle. \(\overline{\mathrm{A D}}\), the bisector of ∠BAC meets \(\overline{\mathrm{B C}}\) at D. Find the co-ordinates of D.
Solution:
A(3, 2, 0),B(5, 3, 2) C(-9, 6, -3) are the vertices of ∆ABC


AB is the bisector of ∠DAC
‘D’ divides BC in the ratio 3 : 13
Co-ordinates of D are

Question 4.
Show that the points 0(0,0,0), A(2, -3, 3), B(-2, 3, -3) are collinear. Find the ratio in which each point divides the segment joining the other two.
Solution:
0(0, 0, 0), A(2, -3, 3), B(-2, 3, -3) are the given points.

∴ O, A, B are collinear.

Ratio in which ‘O’ divides AB
= OA : OB = √22 : √22 = 1 : 1
Ratio is which A divides OB
= OA:AB = -√22 : √22 = -1 : 2
Ratio in which B divides OA
= AB : BO = -2√22 : √22 =-2 : 1
A and B divide externally.

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Three Dimensional Coordinates Solutions Exercise 5(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Three Dimensional Coordinates Solutions Exercise 5(a)

I.

Question 1.
Find the distance of P (3, -2,4) from the origin.
Solution:
OP = \(\sqrt{x^{2}+y^{2}+z^{2}}=\sqrt{9+4+16}\)
= √29 units

Question 2.
Find the distance between the points (3,4,¬2) are (1,0,7).
Solution:

II.

Question 1.
Find x if the distance between (5, -1,7) and (x, 5,1) is 9 units.
Solution:
P(5, -1, 7), Q(x, 5,1) are the given points
PQ = 9

(5 – x)² + 36 + 36 = 81
(5 -x)² = 81 – 72 = 9
5 – x = ± 3
5 – x = 3 or 5 – x = -3
x = 5 – 3 or x = 5 + 3
= 2 or 8

Question 2.
Show that the points (2,3,5), (-1,5, -1) and (4, -3, 2) form a right angled iso-sceles triangle.
Solution:
A(2, 3, 5) B(-1, 5, -1), C(4, -3, 2) are the given points
AB² = (2 + 1)² + (3 – 5)² + (5 + 1)²
= 9 + 4 + 36 = 49

BC² = (-1- 4)² + (5 + 3)² + (-1 – 2)²
= 25 + 64 + 9 = 98

CA² = (4 – 2)² + (-3 -3)² + (2 – 5)²
= 4 + 36 + 9 = 49

AB² = CA² ⇒ AB² + CA² = 49 + 49 = 98 = BC²
ABC is a right angled isosceles triangle.

Question 3.
Show that the points (1, 2, 3), (2, 3, 1) and (3, 1, 2) form an equilateral triangle.
Solution:
A( 1, 2, 3), B(2, 3, 1) and C (3, 1, 2) are the given points
AB² = (1 – 2)² + (2 – 3)²+ (3 – 1)²
= 1 + 1+ 4 = 6

BC² = (2 – 3)² + (3 – 1)² + (1 – 2)²
= 1 + 4 + 1 = 6

CA² = (3 – 1)² + (1 – 2)² + (2 – 3)²
= 4 + 1 + 6

AB² = BC² = CA² ⇒ AB = BC = CA
∴ ABC is an equilateral triangle

Question 4.
P is a variable point which moves such that 3PA = 2PB. If A = (-2, 2,3) and B = (13, -3,13) prove that P satisfies the equation.
x² + y² + z² + 28x – 12y + 10z – 247 = 0.
Solution:
A(-2, 2, 3) and B(13, -3, 13) are the given points. P(x, y, z) is any point on the locus.
Given condition is 3P A = 2P B
⇒ 9 PA² = 4PA²
9[(x + 2)² + (y – 2)² + (z – 3)²] = 4[(x – 13)²+(y + 3)² + (z – 13)²]
⇒ 9(x² + 4x +4 + y² – 4y + 4 + z² – 6z + 9) = 4(x² – 26x + 169 + y² + 6y + 9 + z² – 26z + 169)
⇒ 9x² + 9y² + 9z² + 36x – 36y – 54z +153 = 4x² + 4y² + 4z² – 104x + 24y – 104z + 1388
5x² + 5f + 5z² + 140x – 60y + 50z – 1235 =0
Dividing with 5 locus of P is
x² + y² + z² + 28x – 12y + 10z – 247 = 0

Question 5.
Show that the points (1, 2, 3) (7, 0, 1) and (-2, 3, 4) are collinear.
Solution:
A (1, 2, 3), B(7, 0, 1) C(-2, 3, 4) are the given points.

AB + AC = 2√11 + √11 = 3√11 = BC
A, B, C are collinear

Question 6.
Show that ABCD is a square where A, B, C, D are the points (0, 4, 1), (2, 3 -1) (4, 5, 0) and (2, 6, 2) respectively.
Solution:
A(0, 4, 1), B(2, 3, -1) C(4, 5, 0), D(2, 6, 2) are the given points.
AB² = (0 – 2)² + (4 – 3)² + (1 + 1)²
=4 + 1 + 4 = 9

BC² = (2 – 4)² + (3 – 5)² + (-1 – 0)²
= 4 + 4 + 1 = 9

CD² = (4- 2) ²+ (5 – 6)² + (0 – 2)²
= 4 + 1 – 4 = 9

DA² = (2- 0) ²+ (6 – 4)² + (2 – 1)²
= 4 + 4 + 1 = 9

AB² = BC² = CD² = DA²
⇒ AB = BC = CD = DA
AC² = (0 – 4)² + (4 – 5)² + (1 – 0)² = 16 + 1 + 1 = 18
BD² = (2 – 2)² + (3 – 6)² + (-1 -2)² = 9 + 9 = 18
AC² = BD² ⇒ AC = BD
AB² + BC² = 9 + 9 = 18 = AC²
⇒ ∠ABC = 90°
A, B, C, D are the vertices of a square.