Category: Inter 1st Year

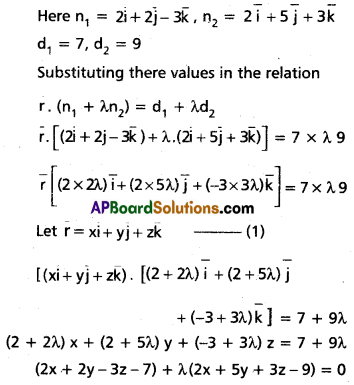
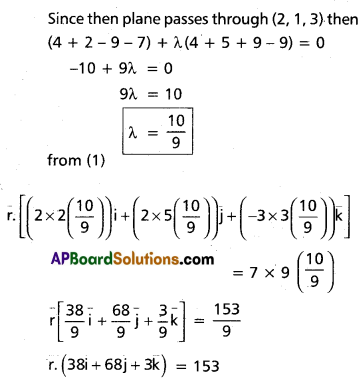
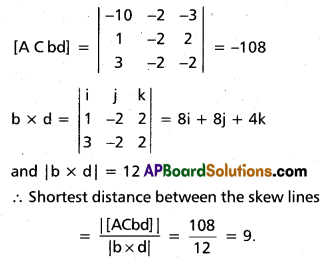
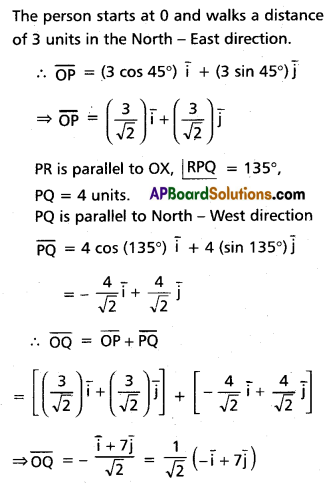
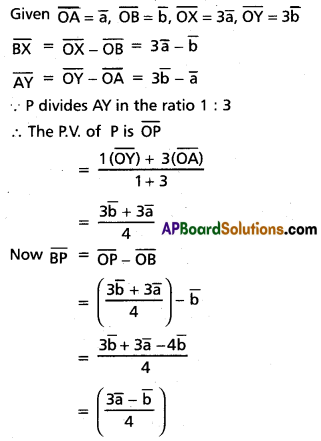
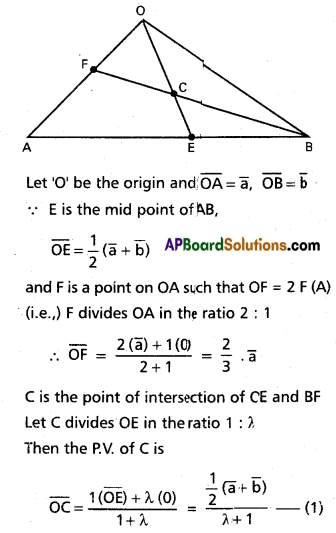
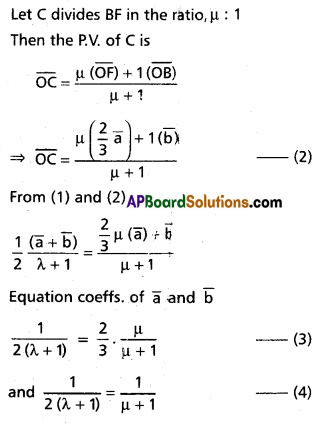
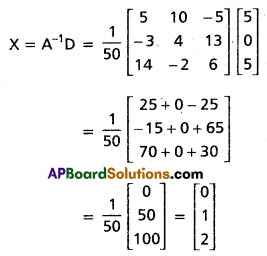
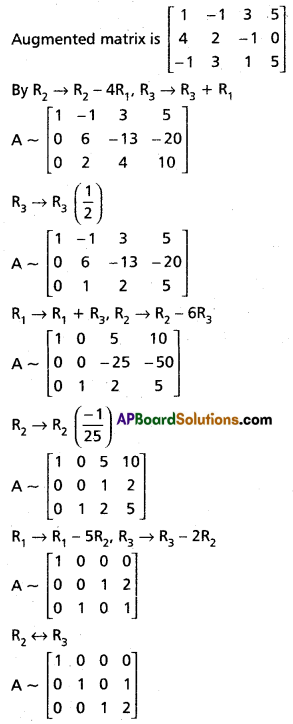
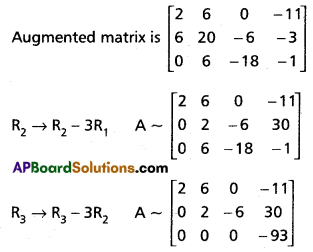
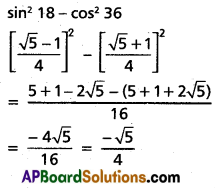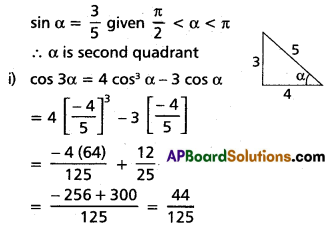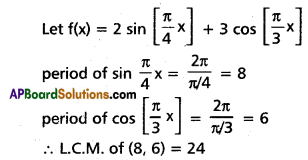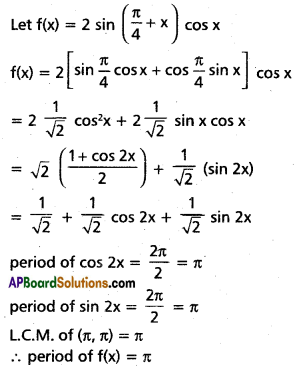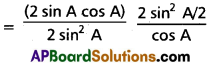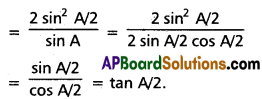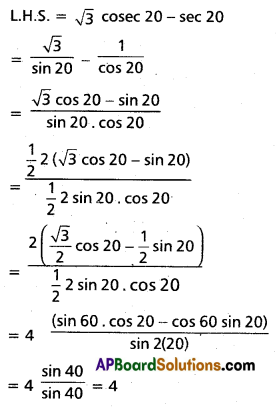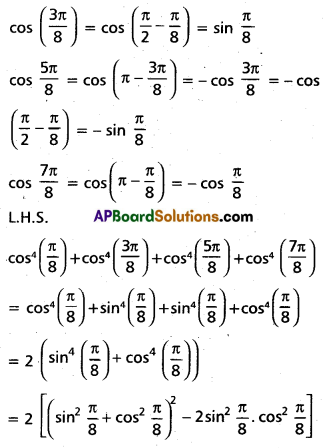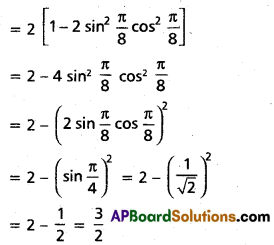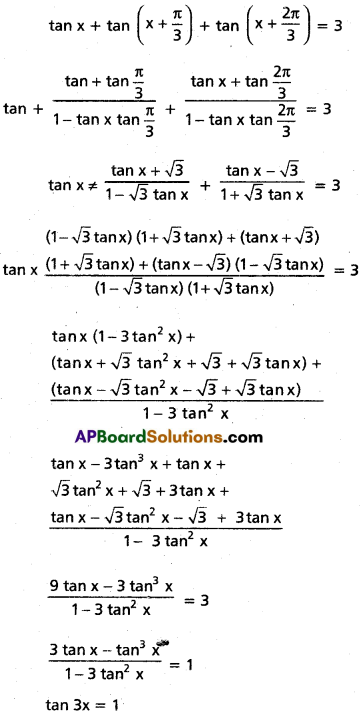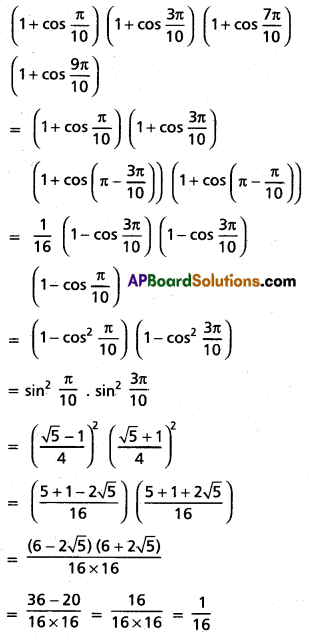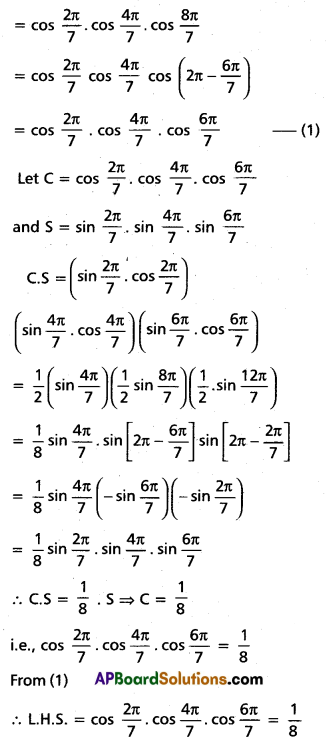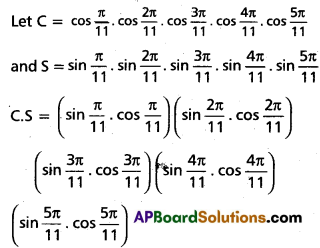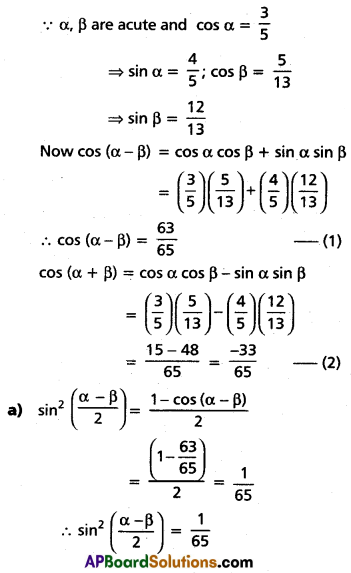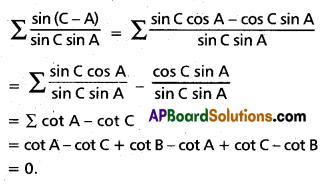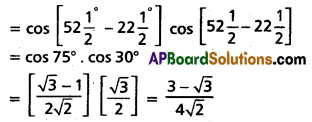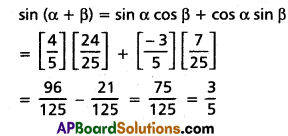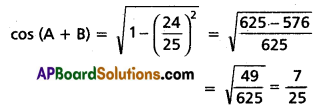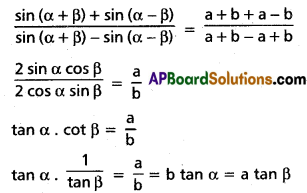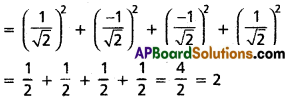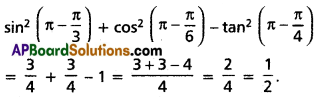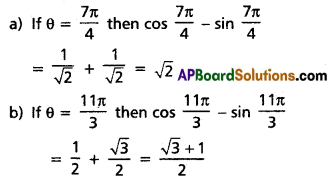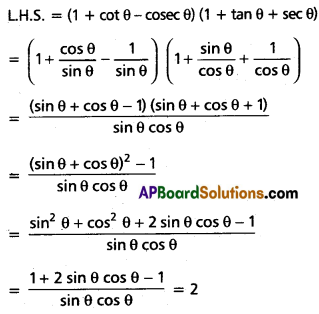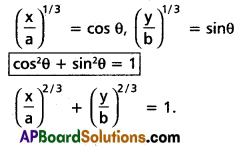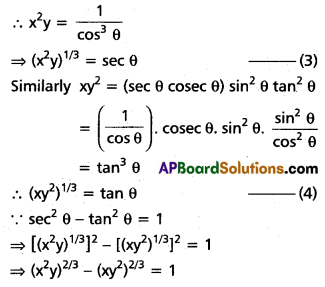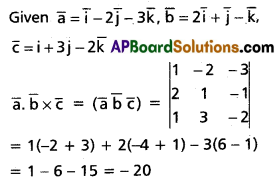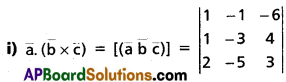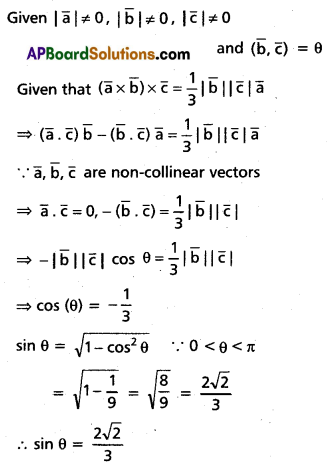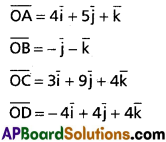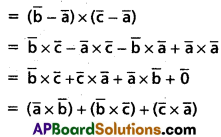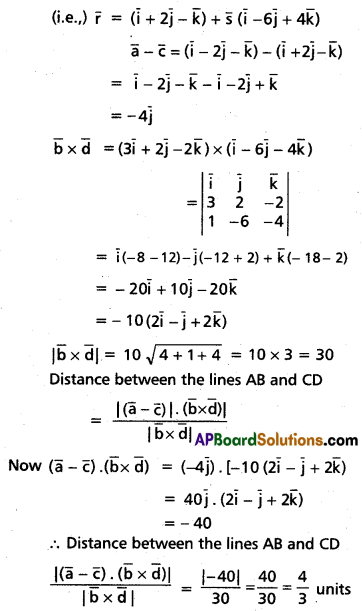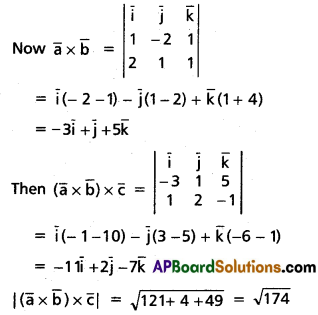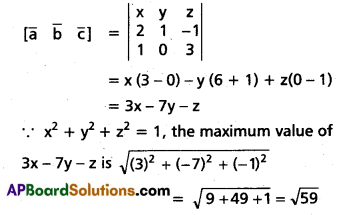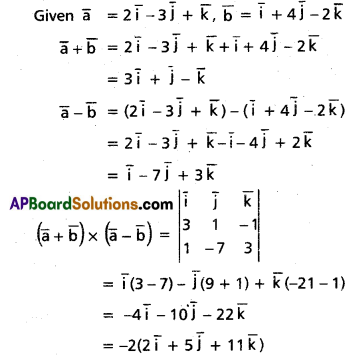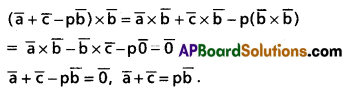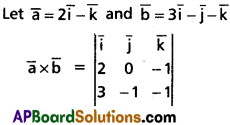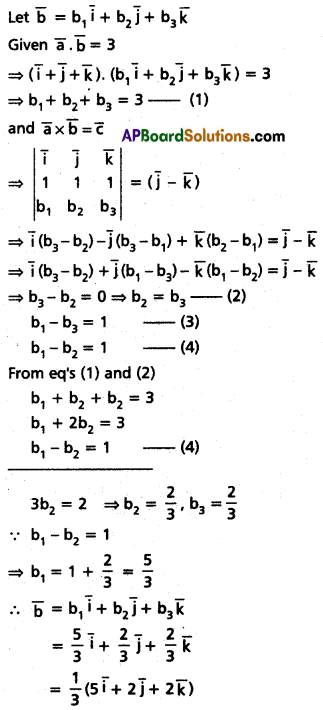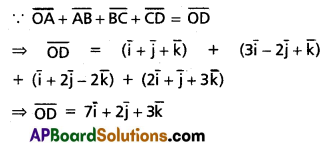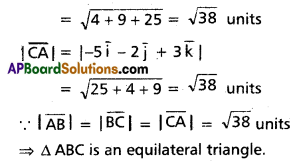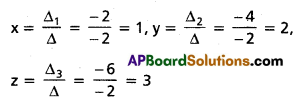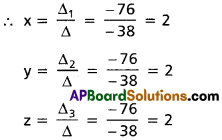Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(e) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(e)

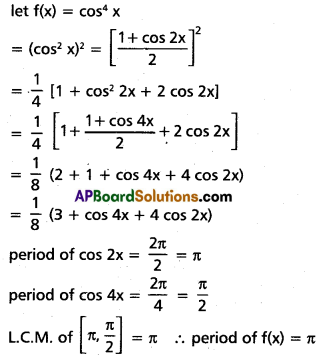
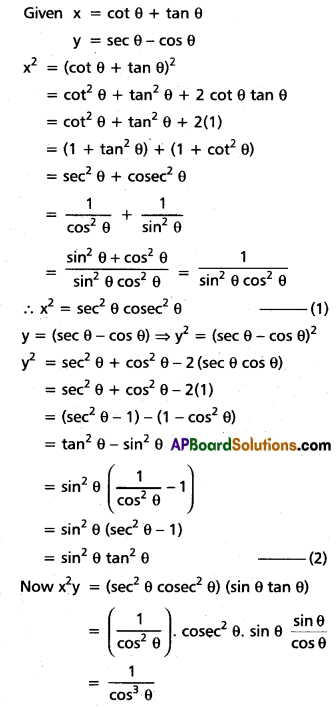
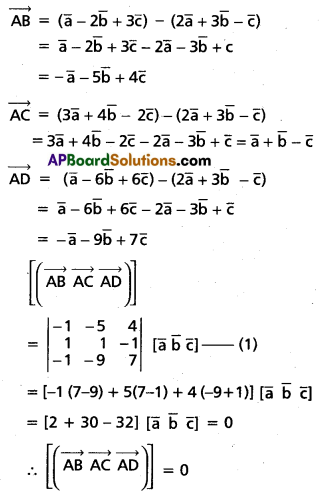
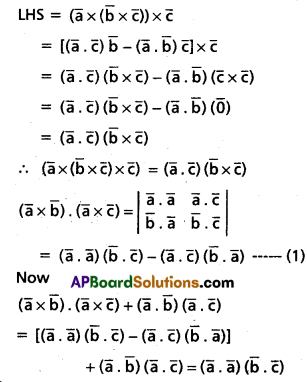
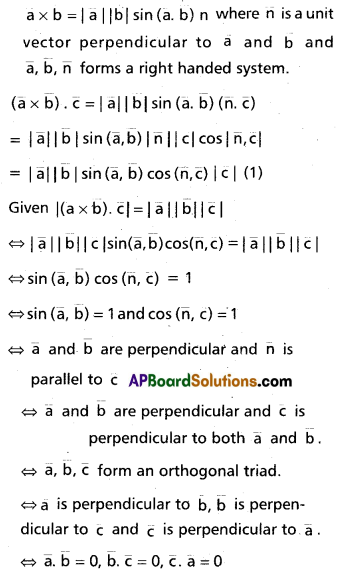
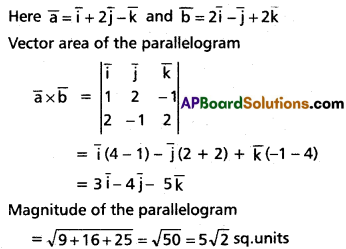
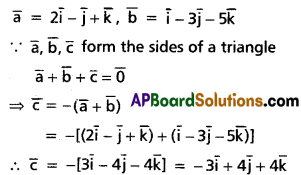
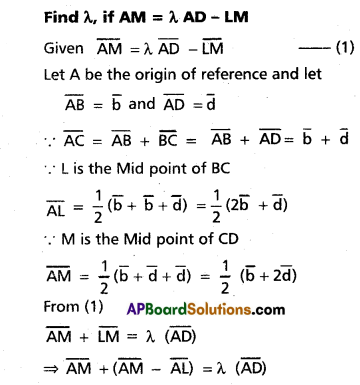
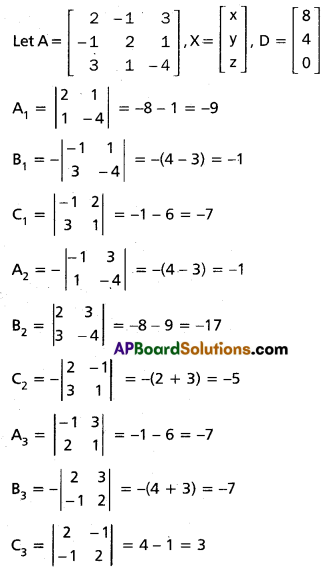
I.

Question 1.
Prove that sin 50° – sin70° + sin 10° = 0
Solution:
LHS = sin 50° – sin 70° + sin 10°
= 2 cos(\(\frac{50^{\circ}+70^{\circ}}{2}\)) . sin(\(\frac{50^{\circ}-70^{\circ}}{2}\)) + sin 10°
= 2 cos 60° . sin (-10°) + sin 10°
= 2(\(\frac{1}{2}\)) (-sin 10°) + sin 10°
= -sin 10° + sin 10°
= 0
= RHS
∴ sin 50° – sin 70° + sin 10° = 0

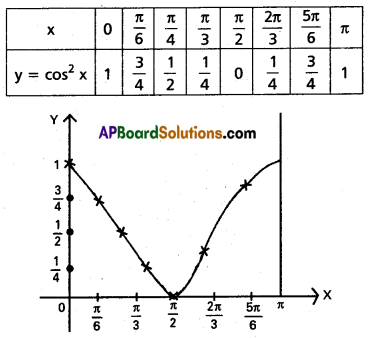
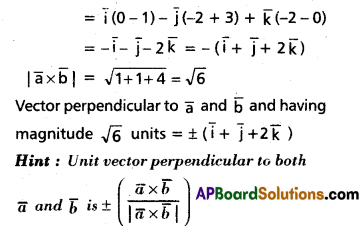
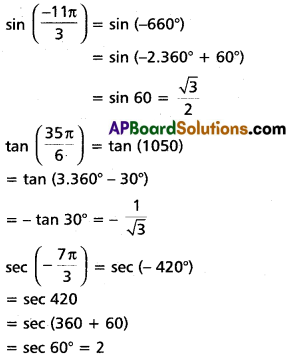
Question 2.
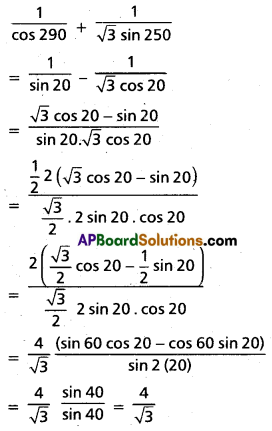
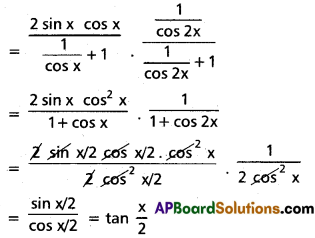
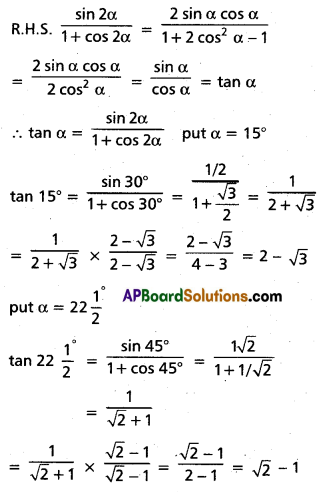
Prove that \(\frac{\sin 70^{\circ}-\cos 40^{\circ}}{\cos 50^{\circ}-\sin 20^{\circ}}=\frac{1}{\sqrt{3}}\)
Solution:

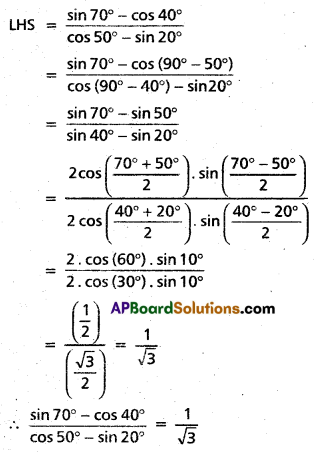
Question 3.
Prove that cos 55° +cos 65° + cos 175° = 0
Solution:
LHS = cos 55° + cos 65° + cos 175°
= cos 65° + cos 55° + cos (180° – 5°)
= 2 cos(\(\frac{65^{\circ}+55^{\circ}}{2}\)) . cos(\(\frac{65^{\circ}-55^{\circ}}{2}\)) – cos 5°
= 2 cos (60°) . cos (5°) – cos 5°
= 2(\(\frac{1}{2}\)) cos 5° – cos 5°
= cos 5° – cos 5°
= 0
= RHS
∴ cos 55° + cos 65° + cos 175° = 0

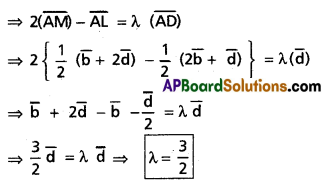
Question 4.
Prove that 4(cos 66° + sin 84°) = √3 + √15
Solution:

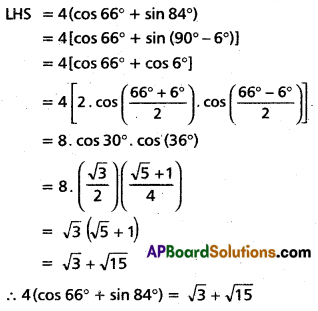
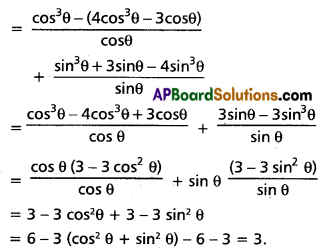
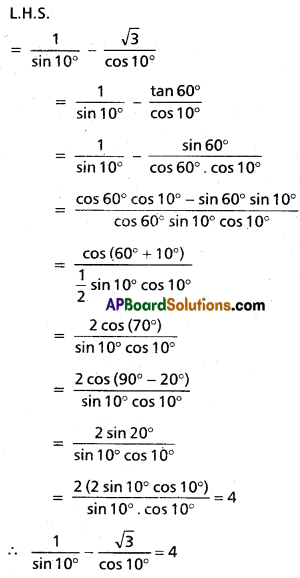
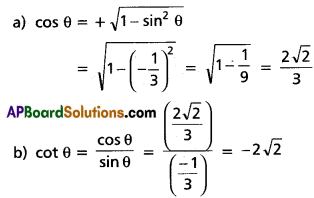
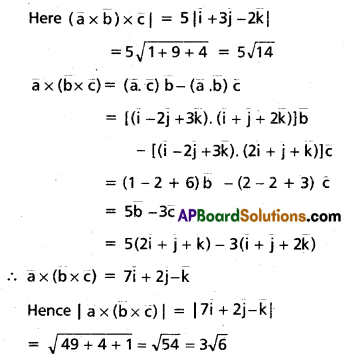
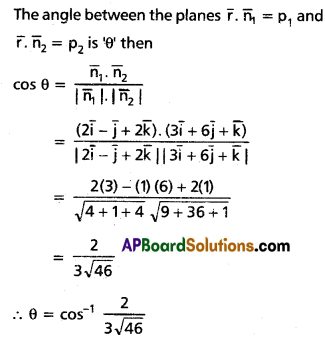
Question 5.
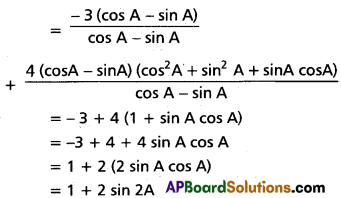
Prove that cos 20° cos 40° – sin 5° sin 25° = \(\frac{\sqrt{3}+1}{4}\)
Solution:
cos 20° cos40° – sin 5° sin 25°
= \(\frac{1}{2}\) [2 cos 20° cos 40° – 2 sin 5° sin 25°]
= \(\frac{1}{2}\) [cos (20° + 40°) + cos (20° – 40°) – {cos (5° – 25°) – cos (5° + 25°)}]
= \(\frac{1}{2}\) [cos 60° + cos 20° – cos 20° + cos 30°]
= \(\frac{1}{2}\left[\frac{1}{2}+\frac{\sqrt{3}}{2}\right]\)
= \(\frac{\sqrt{3}+1}{4}\)
= RHS
∴ cos 20° cos 40° – sin 5° sin 25° = \(\frac{\sqrt{3}+1}{4}\)

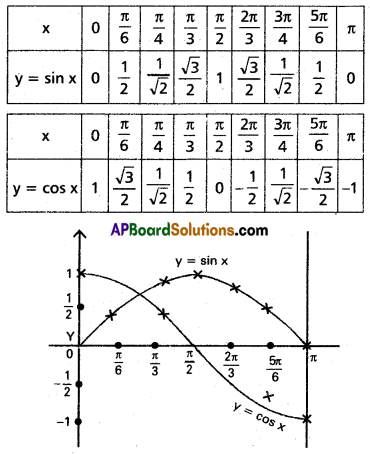
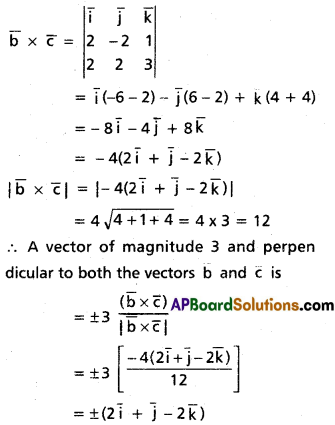
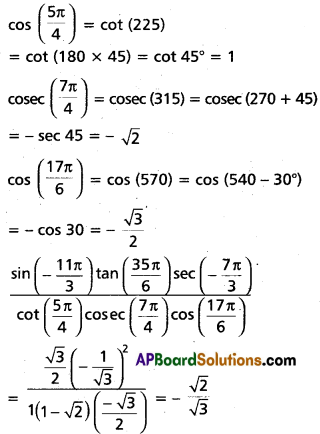
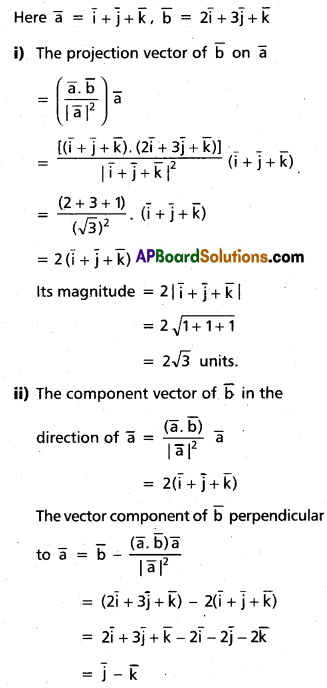
Question 6.
Prove that cos 48° . cos 12° = \(\frac{3+\sqrt{5}}{8}\)
Solution:
LHS = cos 48° . cos 12°
= \(\frac{1}{2}\) (2 cos 48°. cos 12°)
= \(\frac{1}{2}\) [cos (48° + 12°) + cos (48° – 12°)]
= \(\frac{1}{2}\) [cos 60° + cos 36°]
= \(\frac{1}{2}\left[\frac{1}{2}+\frac{\sqrt{5}+1}{4}\right]\)
= \(\frac{1}{2}\left[\frac{2+\sqrt{5}+1}{4}\right]\)
= \(\frac{3+\sqrt{5}}{8}\)
= RHS
∴ cos 48° . cos 12° = \(\frac{3+\sqrt{5}}{8}\)

II.

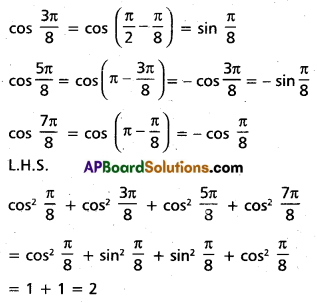
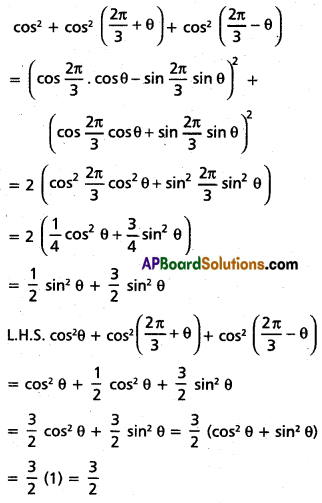
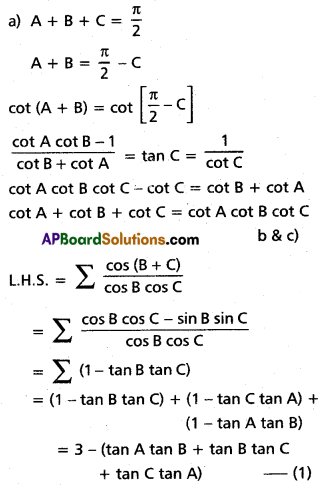
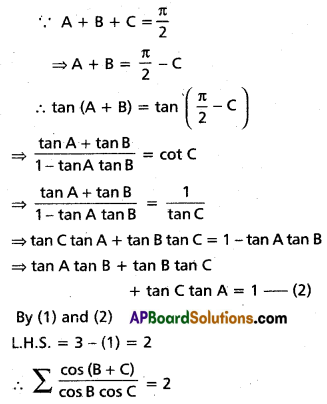
Question 1.
Prove that cos θ + cos[\(\frac{2 \pi}{3}\) + θ] + cos[\(\frac{4 \pi}{3}\) + θ] = 0
Solution:

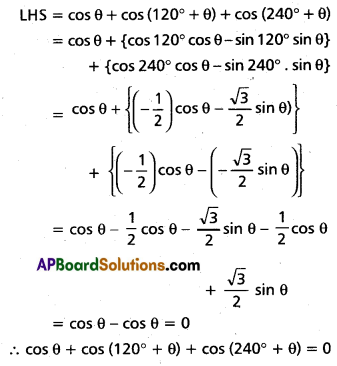
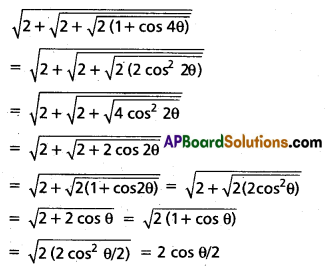
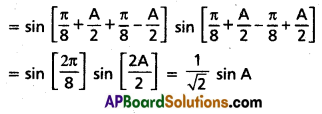
Question 2.
Prove that sin²(α – π/4) + sin²(α + π/2) – sin²(α – π/2) = \(\frac{1}{2}\)
Solution:

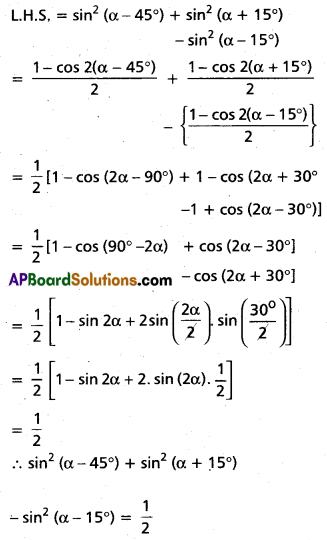
Question 3.
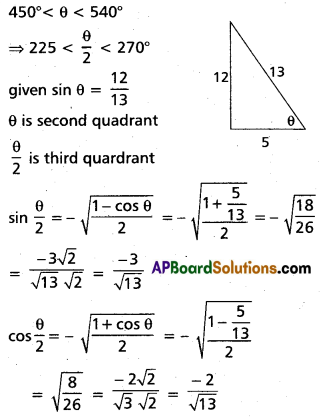
If sin x + sin y = \(\frac{1}{4}\) and cos x + cos y = \(\frac{1}{3}\), then show that
(i) \(\tan \left(\frac{x+y}{2}\right)=\frac{3}{4}\)
(ii) cot (x + y) = \(\frac{7}{24}\)
Solution:

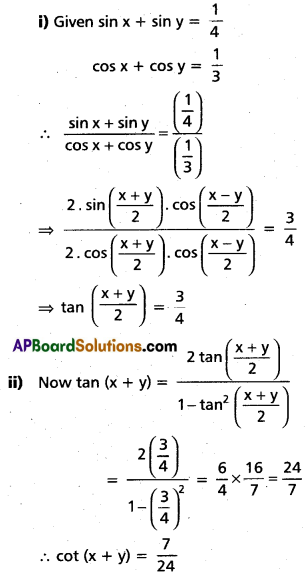
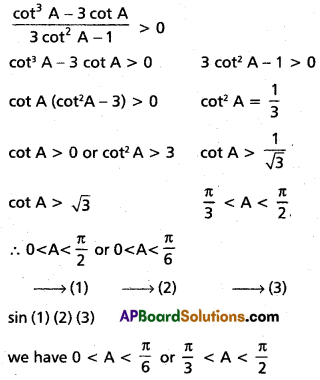
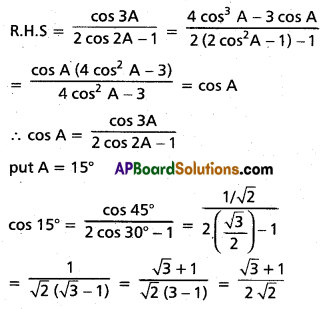
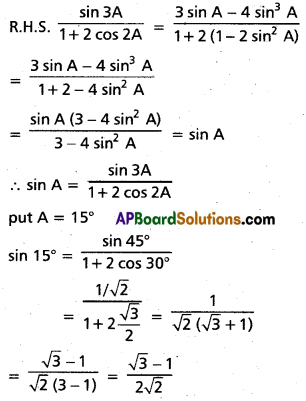
Question 4.
If neither [A – \(\frac{\pi}{12}\)] nor [A – \(\frac{5 \pi}{12}\)] is an integral multiple of π. Prove that cot(π/2 – A) + tan(π/12 + A) = \(\frac{4 \cos 2 A}{1-2 \sin 2 A}\)
Solution:

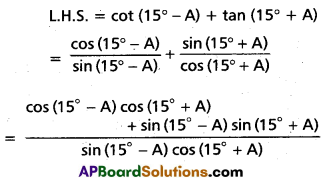
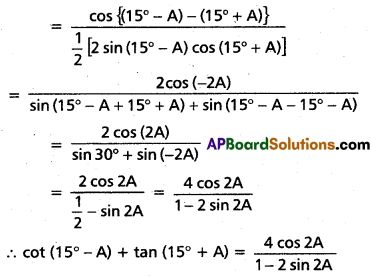
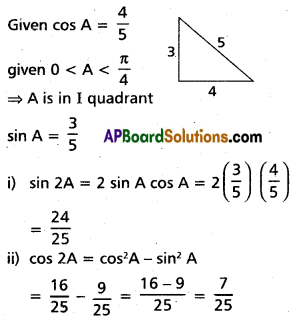
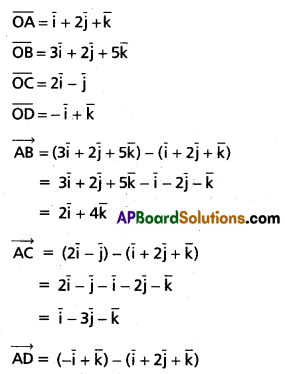
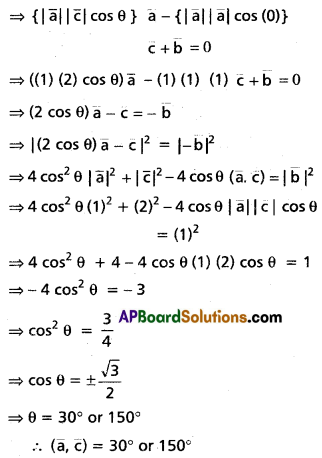
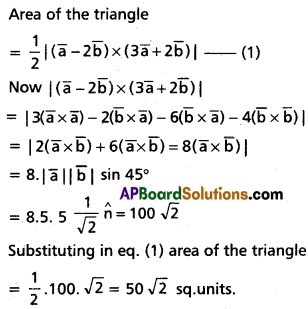
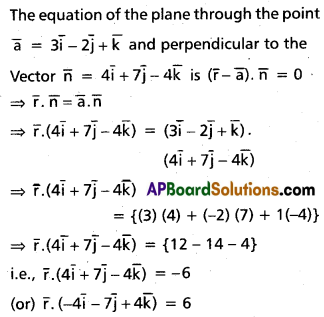
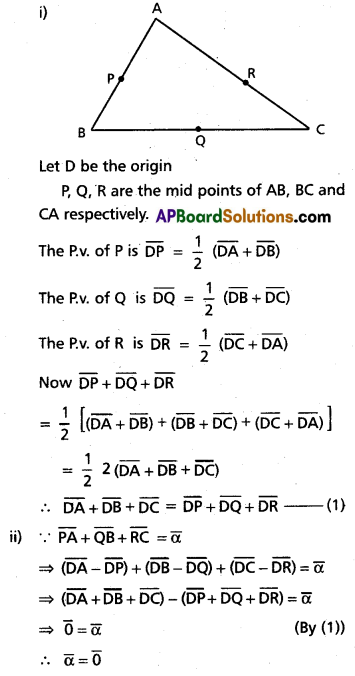
Question 5.
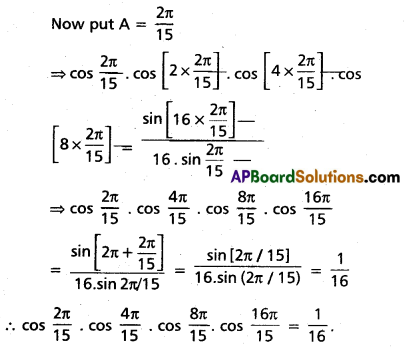
Prove that 4 cos 12° cos 48° cos 72° = cos 36°.
Solution:
LHS = 4 cos 12° cas 48° cos 72°
= 2 cos 12° {2 cos 72° cos 48°}
= 2 cos 12° {cos (72° + 48°) + cos (72° – 48°)}
= 2 cos 12° {cos (120°) + cos 24°)
= 2 cos 12° {\(\frac{1}{2}\) + cos 24°}
= 2 cos 12° \(\left\{\frac{-1+2 \cos 24^{\circ}}{2}\right\}\)
= -cos 12° + 2 cos 24° cos 12°
= -cos 12° + {cos(24° + 12°) + cos (24° – 12°))
= -cos 12° + cos 36° + cos 12°
= cos 36°
= RHS
∴ 4 cos 12° cos 48° cos 72° = cos 36°

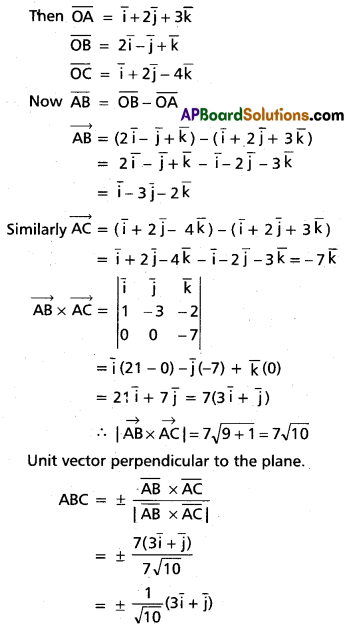
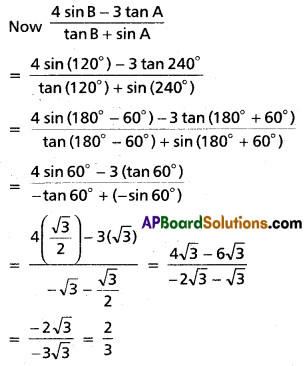
Question 6.
Prove that sin 10° + sin 20° + sin 40° + sin 50° = sin 70° + sin 80°
Solution:
LH.S. = sin 10° + sin 20° + sin 40° + sin 50°
= (sin 50°+ sin 10°) + (sin 40° + sin 20°)
= 2 sin(\(\frac{50^{\circ}+10^{\circ}}{2}\)) . cos(\(\frac{50^{\circ}-10^{\circ}}{2}\)) + 2 sin(\(\frac{40^{\circ}+20^{\circ}}{2}\)) . cos(\(\frac{40^{\circ}-20^{\circ}}{2}\))
= 2 . sin (30°) . cos 20° + 2 sin 30° . cos 10°
= 2 sin 30° (cos 20° + cos 10°)
= 2(\(\frac{1}{2}\)) [cos(90° – 70°) + cos(90° – 80°)]
= sin 70° + sin 80°
= RHS
∴ sin 10° + sin 20° + sin 40° + sin 50° = sin 70° + sin 80°

III.

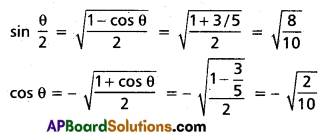
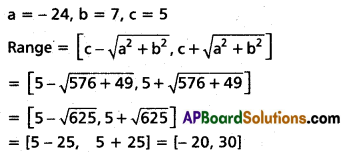
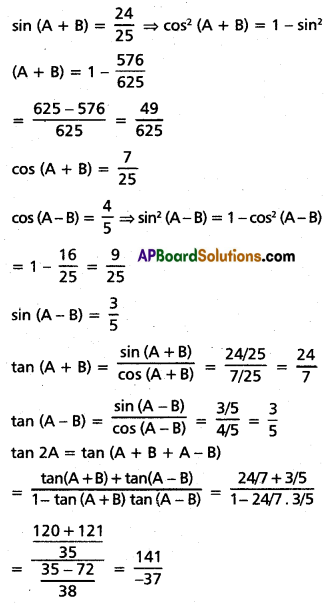
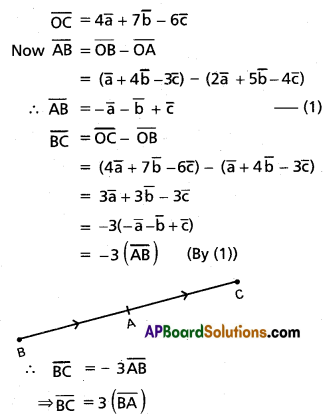
Question 1.
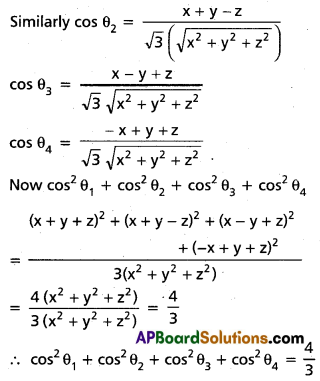
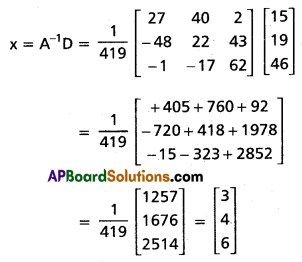
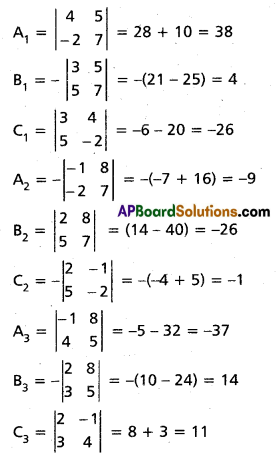
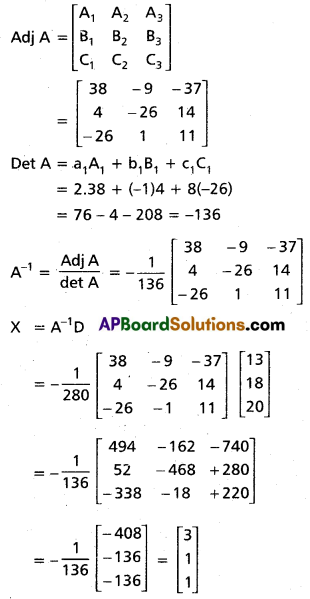
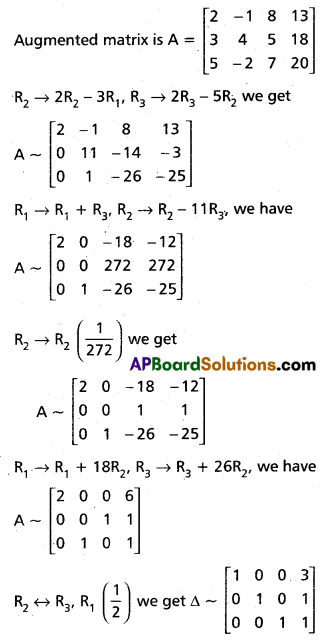
If cos x + cos y = \(\frac{4}{5}\) and cos x – cos y = \(\frac{2}{7}\) find the value of \(14 \tan \left(\frac{x-y}{2}\right)+5 \cot \left(\frac{x+y}{2}\right)\)
Solution:

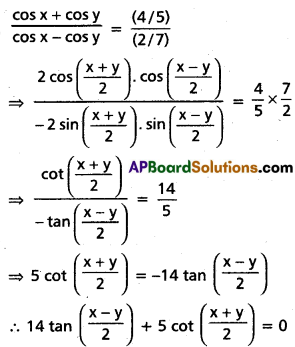
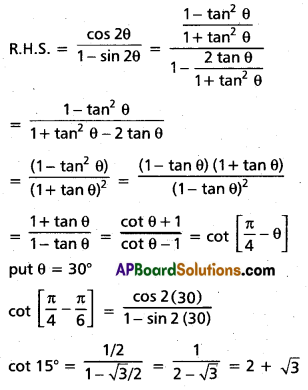
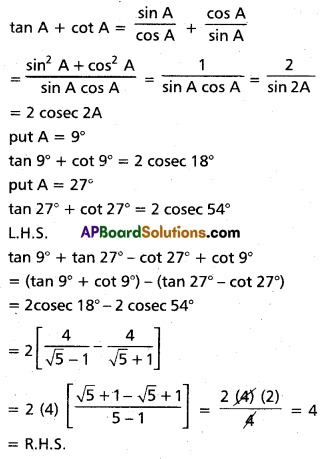
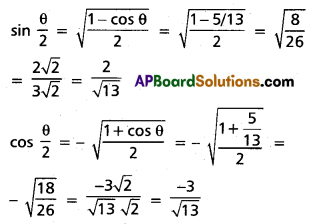
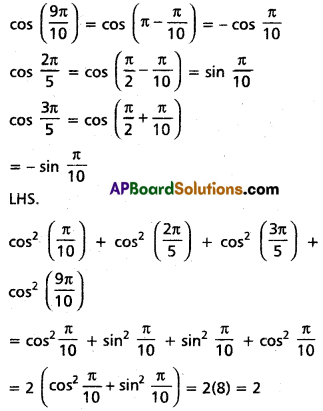
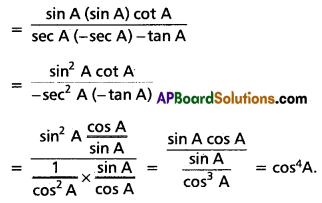
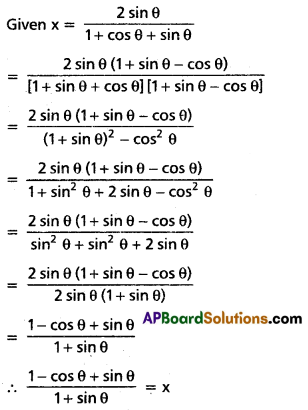
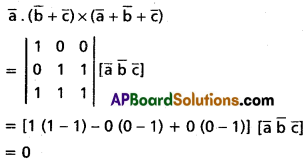
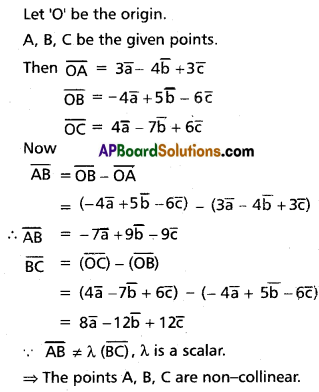
Question 2.
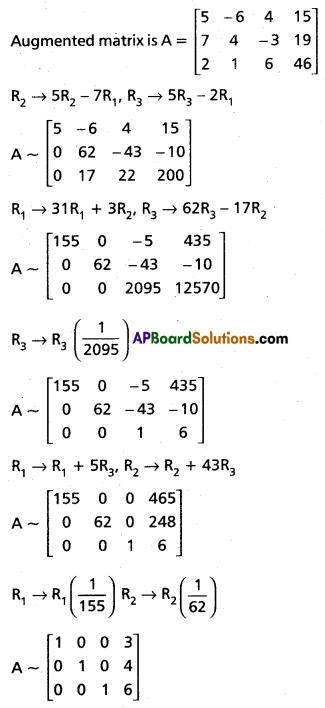
If none of the denominators is zero, prove that
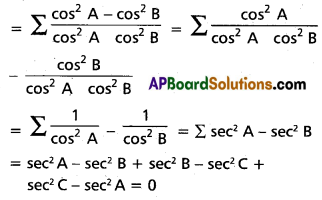
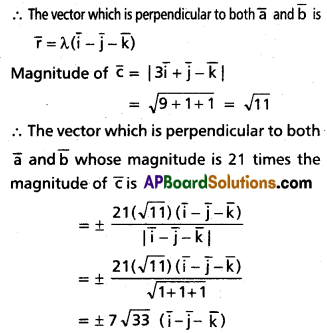
\(\left(\frac{\cos A+\cos B}{\sin A-\sin B}\right)^{n}+\left(\frac{\sin A+\sin B}{\cos A-\cos B}\right)^{n}\) = \(\begin{cases}2 \cot ^{n}\left(\frac{A-B}{2}\right), & \text { if } n \text { is even } \\ 0, & \text { if } n \text { is odd }\end{cases}\)
Solution:

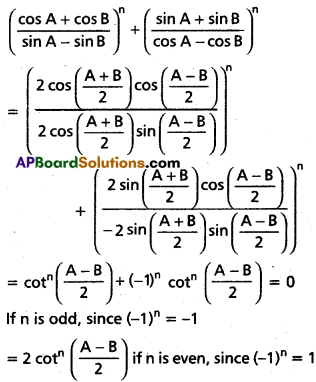
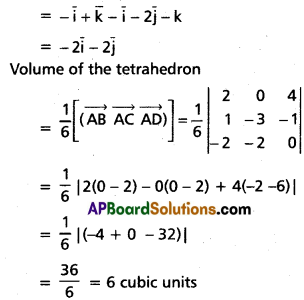
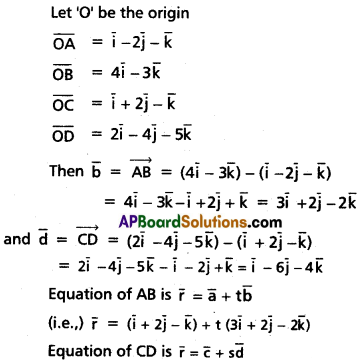
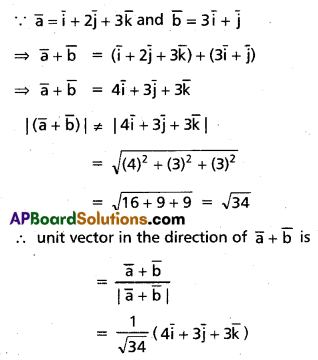
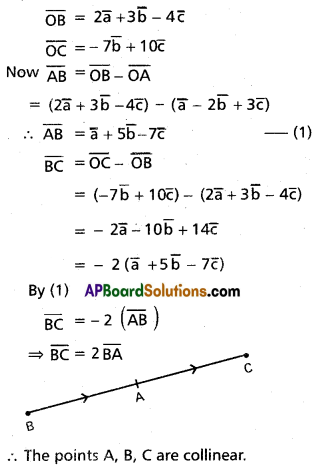
Question 3.
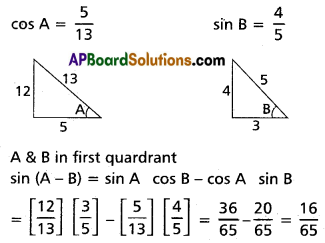
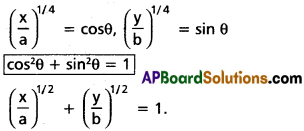
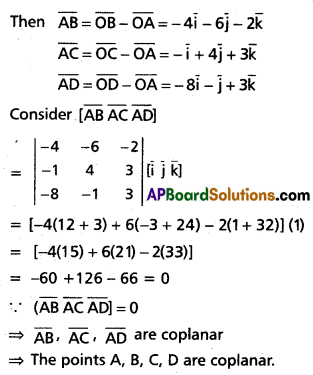
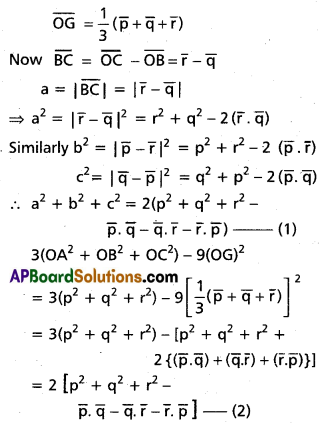
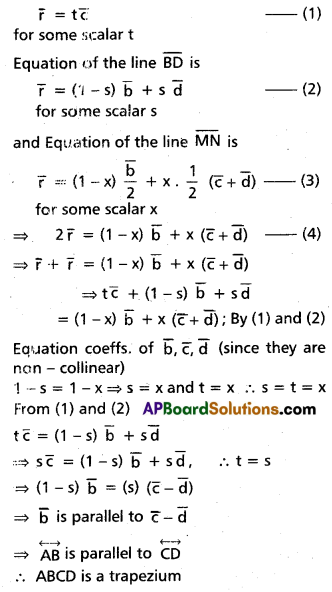
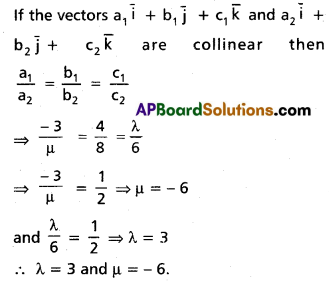
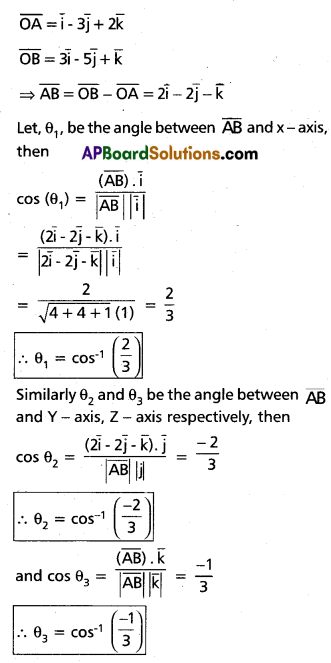
If sin A = sin B and cos A = cos B, then prove that A = 2nπ + B for some integer n.
Solution:

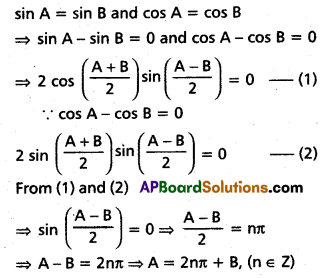
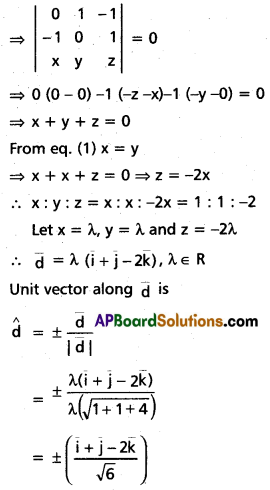
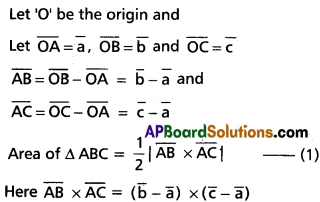
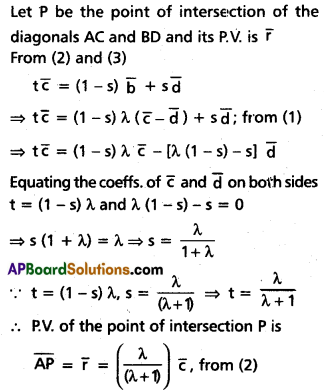
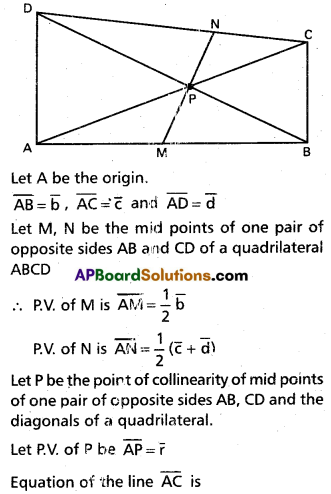
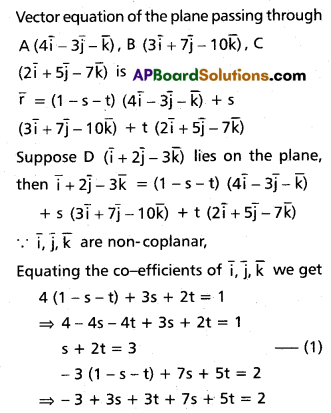
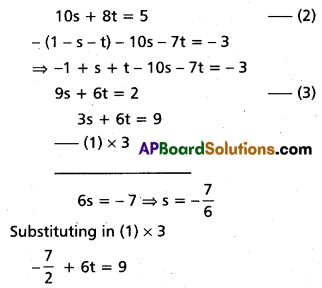
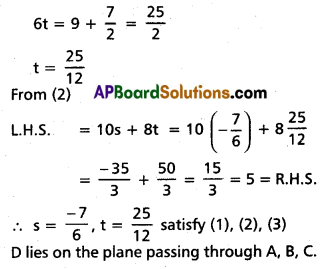
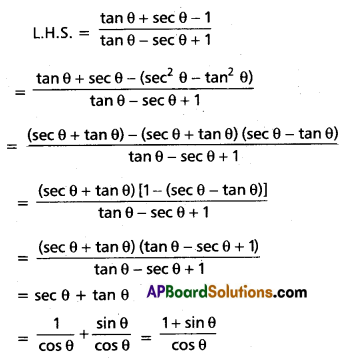
Question 4.
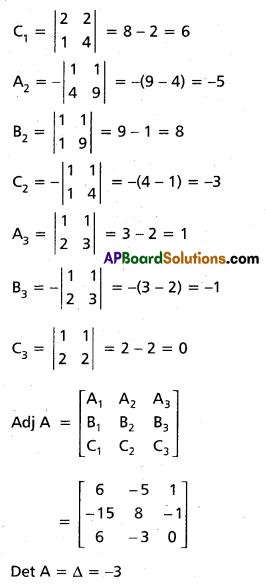
If cos nα ≠ 0 and cos \(\frac{\alpha}{2}\) ≠ 0. then show that \(\frac{\sin (n+1) \alpha-\sin (n-1) \alpha}{\cos (n+1) \alpha+2 \cos n \alpha+\cos (n-1) \alpha}\) = tan \(\frac{\alpha}{2}\)
Solution:

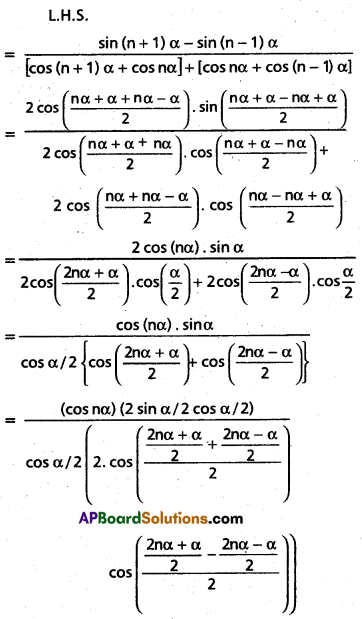
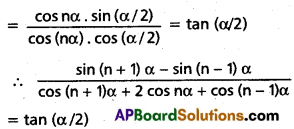
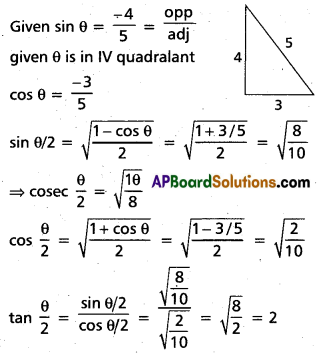
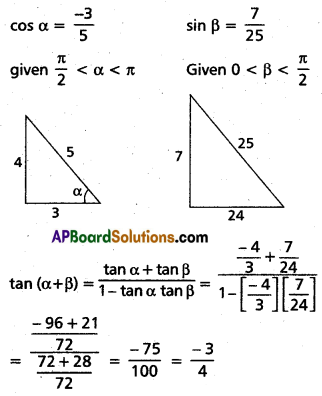
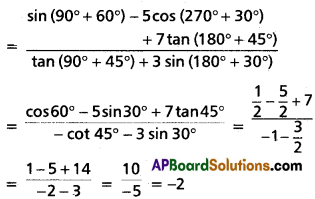
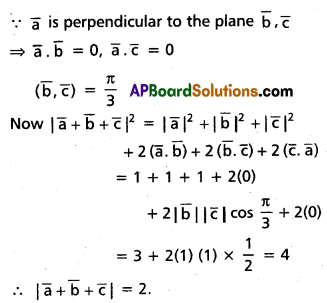
Question 5.
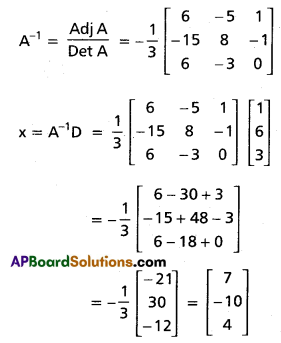
If sec (θ + α) + sec (θ – α) = 2 sec θ and cos α ≠ 1, then show that cos θ = ±√2 cos \(\frac{\alpha}{2}\).
Solution:

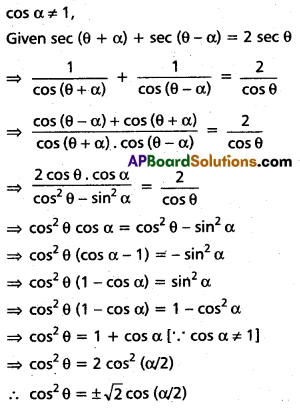
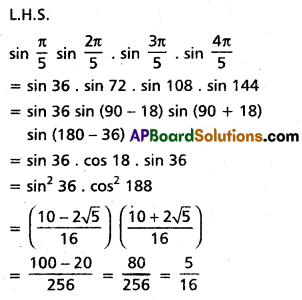
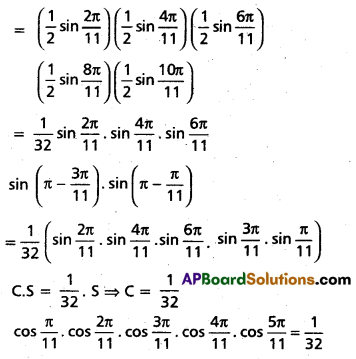
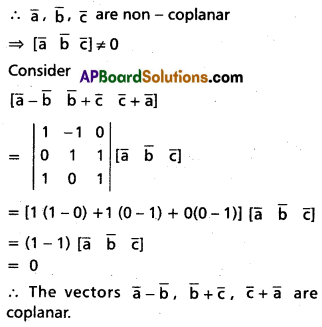
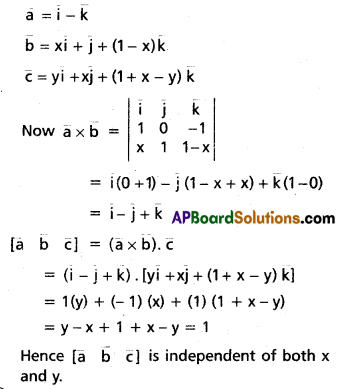
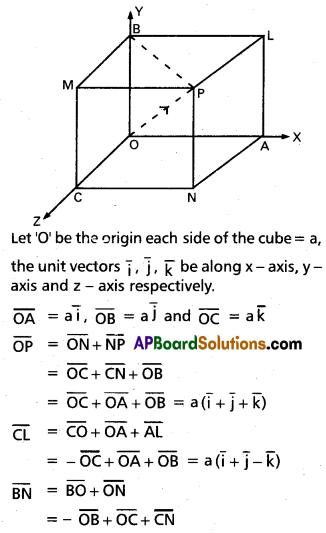
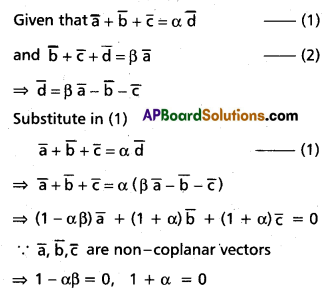
Question 6.
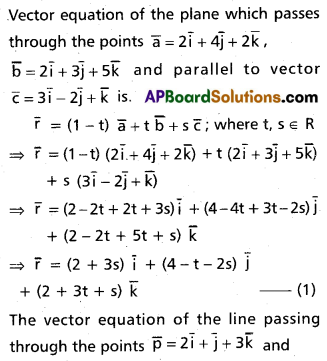
If none of x, y, z is an odd multiple of \(\frac{\pi}{2}\) and if sin (y + z – x), sin (z + x – y), sin (x + y – z) are in A.P., then prove that tan x, tan y, tan z are also in A.P.
Solution:
sin (y + z – x), sin (z + x – y), sin (x + y – z) are in A.P.
⇒ sin (z + x – y) – sin (y + z – x) = sin (x + y – z) – sin (z + x – y)
⇒ 2 cos z sin (x – y) = 2 cos x sin (y – z)
⇒ cos z [sin x cos y – cos x sin y] = cos x [sin y cos z – cos y sin z]
Dividing with cos x cos y cos z, we get
\(\frac{\sin x}{\cos x}-\frac{\sin y}{\cos y}=\frac{\sin y}{\cos y}-\frac{\sin z}{\cos z}\)
⇒ tan x – tan y = tan y – tan z
⇒ tan x + tan z = 2 tan y
∴ tan x, tan y, tan z are in A.P.

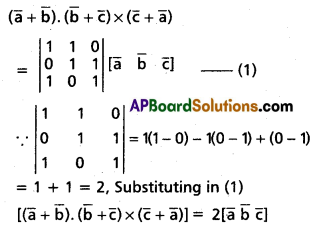
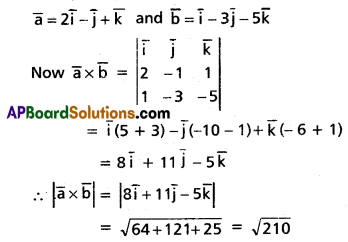
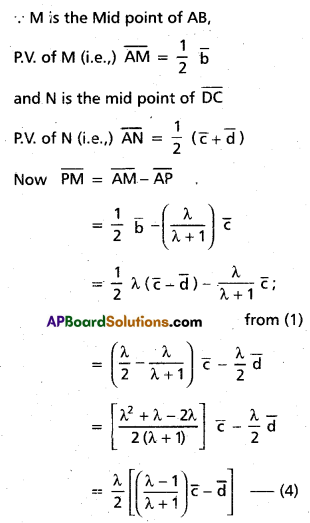
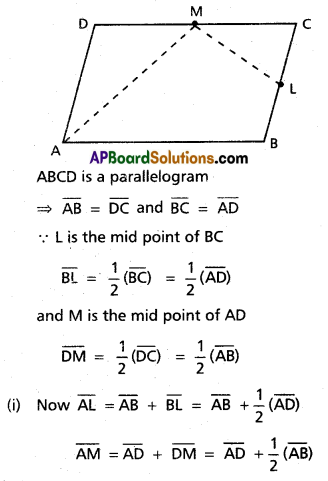
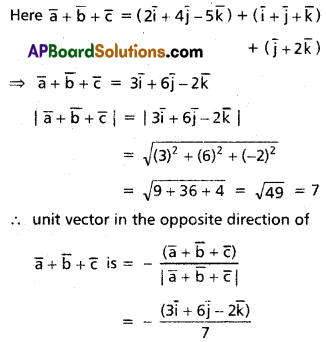
Question 7.
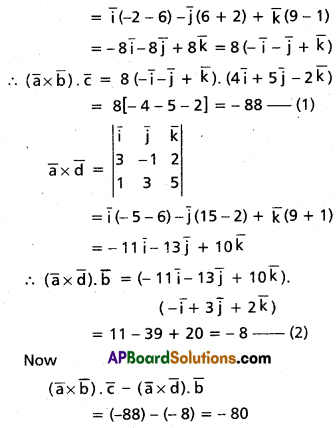
If x, y, z are non zero real numbers and if x cos θ = y cos (θ + \(\frac{2 \pi}{3}\)) = z cos (θ + \(\frac{2 \pi}{3}\)) for some θ ∈ R, then show that xy + yz + zx = 0.
Solution:

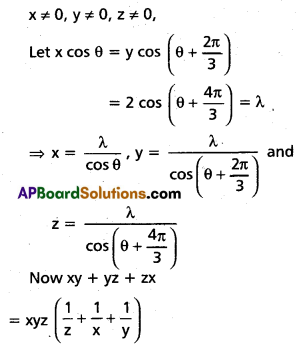
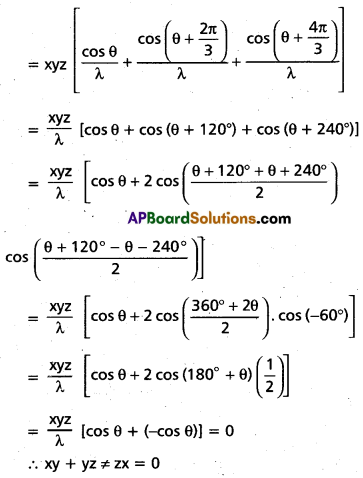
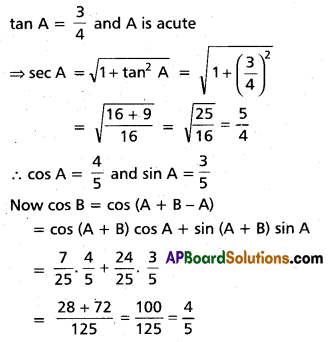
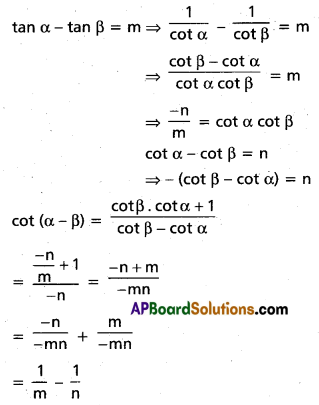
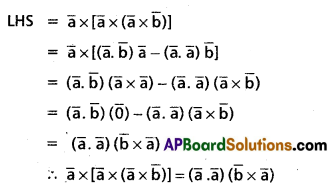
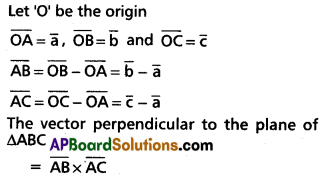
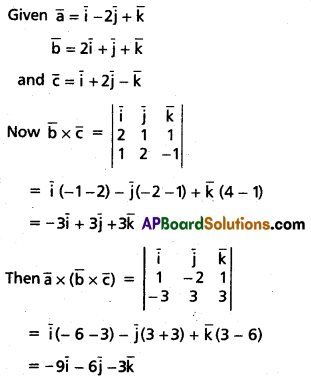
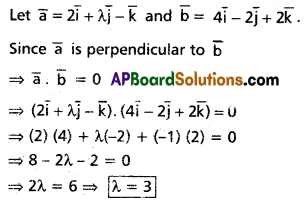
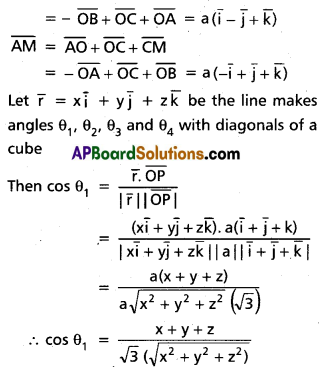
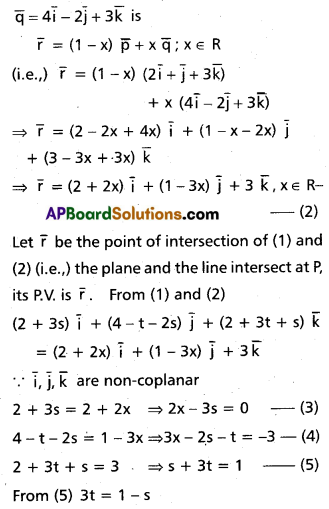
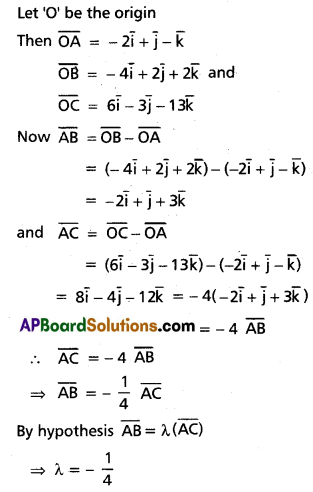
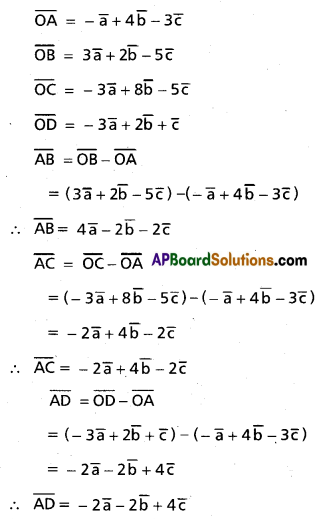
Question 8.
If neither A nor A + B is an odd multiple of \(\frac{\pi}{2}\) and if m sin B = n sin(2A + B), then prove that (m + n) tan A = (m – n) tan (A + B).
Solution:
Neither A nor (A + B) is an odd multiple of \(\frac{\pi}{2}\)
Given that m sin B = n sin (2A + B)

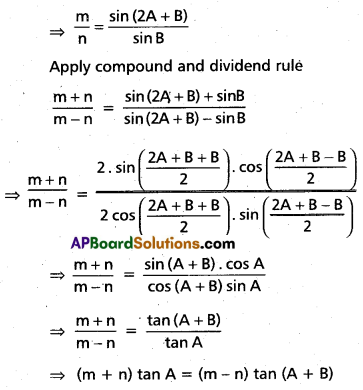
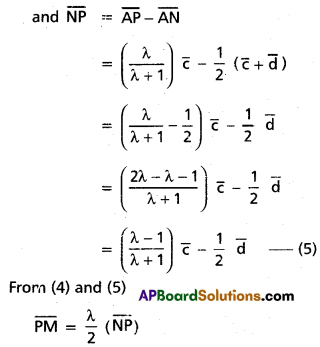
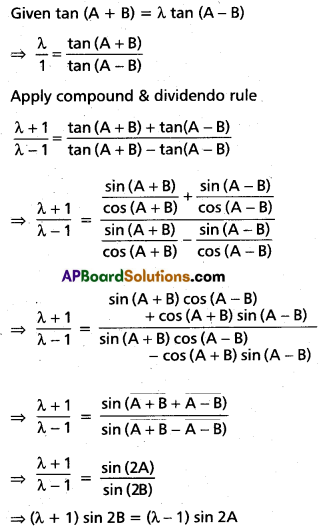
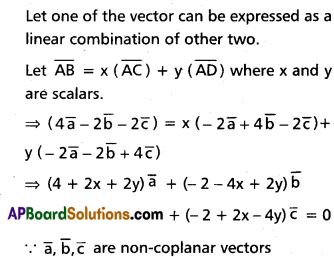
Question 9.
If tan (A + B) = λ tan (A – B), then show that (λ + 1) sin 2B = (λ – 1) sin 2A.
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(d) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(d)

I.

Question 1.
Simplify
(i) \(\frac{\sin 2 \theta}{1+\cos 2 \theta}\)
Solution:
\(\frac{\sin 2 \theta}{1+\cos 2 \theta}\)
= \(\frac{2 \sin \theta \cos \theta}{2 \cos ^{2} \theta}\)
= tan θ

(ii) \(\frac{3 \cos \theta+\cos 3 \theta}{3 \sin \theta-\sin 3 \theta}\)
Solution:
\(\frac{3 \cos \theta+\cos 3 \theta}{3 \sin \theta-\sin 3 \theta}\)
= \(\frac{3 \cos \theta+4 \cos ^{3} \theta-3 \cos \theta}{3 \sin \theta-\left(3 \sin \theta-4 \sin ^{3} \theta\right)}\)
= \(\frac{4 \cos ^{3} \theta}{4 \sin ^{3} \theta}\)
= cot³θ

Question 2.
Evaluate the following.
(i) 6 sin 20° – 8 sin3 20°
Solution:
6 sin 20° – 8 sin³20°
= 2(3 sin 20° – 4 sin³20°)
= 2 sin (3 × 20)
= 2 sin 60°
= 2 \(\left[\frac{\sqrt{3}}{2}\right]\)
= √3

(ii) cos²72° – sin²54°
Solution:

(iii) sin²42° – sin²12°
Solution:
sin (42 + 12) sin (42 – 12)
= sin 54° . sin 30°
= \(\left[\frac{\sqrt{5}+1}{4}\right] \frac{1}{2}\)
= \(\frac{\sqrt{5}+1}{8}\)

Question 3.
(i) Express \(\frac{\sin 4 \theta}{\sin \theta}\) interms of cos³θ and cos θ.
Solution:
consider sin 4θ = sin(3θ + θ)
= sin 3θ cos θ + cos 3θ sin θ
= (3 sin θ – 4 sin³θ) cos θ + (4 cos³θ – 3 cos θ) sin θ
= 3 sin θ cos θ – 4 sin³θ cos θ + 4 cos³θ sin θ – 3 cos θ sin θ
= 4 cos³θ sin θ – 4 sin³θ cos θ
= sin θ (4 cos³θ – 4 sin²θ cos θ)
\(\frac{\sin 4 \theta}{\sin \theta}=\frac{\sin \theta\left(4 \cos ^{3} \theta-4 \sin ^{2} \theta \cos \theta\right)}{\sin \theta}\)
= 4 cos³θ – 4(1 – cos²θ) cos θ
= 4 cos³θ – 4 cos θ + 4 cos³θ
= 8 cos³θ – 4 cos θ

(ii) Express cos⁶A + sin⁶A in terms of sin 2A.
Solution:
cos⁶A + sin⁶A
= (cos²A)³ + (sin²A)³
= (cos²A + sin²A)³ – 3 cos²A sin²A (cos²A + sin²A)
= 1 – 3 cos²A sin²A
= 1 – \(\frac{3}{4}\) (4 cos²A sin²A)
= 1 – \(\frac{3}{4}\) (sin²2A)

(iii) Express \(\frac{1-\cos \theta+\sin \theta}{1+\cos \theta+\sin \theta}\) in terms of tan θ/2
Solution:

Question 4.
(i) If sin α = \(\frac{3}{5}\), where \(\frac{\pi}{2}\) < α < π, evaluate cos 3α and tan 2α.
Solution:

(ii) If cos A = \(\frac{7}{25}\) and \(\frac{3 \pi}{2}\) < A < 2π, then find the value of cot A/2.
Solution:

(iii) If 0 < θ < \(\frac{\pi}{8}\), show that \(\sqrt{2+\sqrt{2+\sqrt{2+2 \cos 4 \theta)}}}=2 \cos (\theta / 2)\)
Solution:

Question 5.
Find the extreme values of
(i) cos 2x + cos2x
Solution:
cos 2x + cos²x
= 2 cos²x – 1 + cos²x
= 3 cos²x – 1
-1 ≤ cos x ≤ 1
0 ≤ cos²x ≤ 1
0 ≤ 3 cos²x ≤ 3
-1 ≤ 3 cos²x – 1 ≤ 2
maximum value = 2
minimum value = -1

(ii) 3 sin²x + 5 cos²x
Solution:
3 sin²x + 5 cos²x
= 3(1 – cos²x) + 5 cos²x
= 3 – 3 cos²x + 5 cos²x
= 3 + 2 cos²x
-1 ≤ cos x ≤ 1
0 ≤ cos²x ≤ 1
0 ≤ 2 cos²x ≤ 2
3 ≤ 3 + 2 cos²x ≤ 5
maximum value = 5
minimum value = 3

Question 6.
If a ≤ cos θ + 3√2 sin[θ + \(\frac{\pi}{4}\)] + 6 ≤ b, then find the largest value of a and smallest value of b.
Solution:
a ≤ cos θ + 3√2 sin[θ + \(\frac{\pi}{4}\)] + 6 ≤ b
consider cos θ + 3√2 sin[θ + \(\frac{\pi}{4}\)] + 6
= cos θ + 3√2[sin θ cos \(\frac{\pi}{4}\) + cos θ sin \(\frac{\pi}{4}\)] + 6
= cos θ + 3√2 sin θ \(\frac{1}{\sqrt{2}}\) + 3√2 cos θ \(\frac{1}{\sqrt{2}}\) + 6
= cos θ + 3 sin θ + 3 cos θ + 6
= 4 cos θ + 3 sin θ + 6
∴ a = 4, b = 3, c = 6
maximum value = c + \(\sqrt{a^{2}+b^{2}}\)
= 6 + \(\sqrt{16+9}\)
= 6 + 5
= 11
minimum value = c – \(\sqrt{a^{2}+b^{2}}\)
= 6 – 5
= 1

Question 7.
Find the periods for the following functions.
(i) cos⁴x
Solution:

(ii) \(2 \sin \left[\frac{\pi x}{4}\right]+3 \cos \left[\frac{\pi x}{3}\right]\)
Solution:

(iii) sin²x + 2 cos²x
Solution:
Let f(x) = sin²x + 2 cos²x
= 1 – cos²x + 2 cos²x
= 1 + cos²x
= 1 + \(\frac{1+\cos 2 x}{2}\)
∴ period of cos 2x = \(\frac{2 \pi}{2}\) = π
∴ period of f(x) = π

(iv) \(2 \sin \left[\frac{\pi}{4}+x\right] \cos x\)
Solution:

(v) \(\frac{5 \sin x+3 \cos x}{4 \sin 2 x+5 \cos x}\)
Solution:
Let f(x) = \(\frac{5 \sin x+3 \cos x}{4 \sin 2 x+5 \cos x}\)
period of sin x = 2π
period of cos x = 2π
period of sin 2x = \(\frac{2 \pi}{2}\) = π
period of cos x = 2π
L.C.M. of (2π, 2π, π, 2π) = 2π
∴ period of f(x) = 2π

II.

Question 1.
(i) If 0 < A < \(\frac{\pi}{4}\) and cos A = \(\frac{4}{5}\), find the values of sin 2A and cos 2A.
Solution:

(ii) For what values of A in the first quadrant, the expression \(\frac{\cot ^{3} A-3 \cot A}{3 \cot ^{2} A-1}\) is positive?
Solution:

(iii) Prove that \(\frac{\cos 3 A+\sin 3 A}{\cos A-\sin A}=1+2 \sin A\)
Solution:

Question 2.
(i) Prove that \(\cot \left[\frac{\pi}{4}-\theta\right]=\frac{\cos 2 \theta}{1-\sin 2 \theta}\) and hence find the value of cot 15°.
Solution:

(ii) If θ lies in third quadrant and sin θ = \(\frac{-4}{5}\), find the values of cosec (θ/2) and tan (θ/2).
Solution:

(iii) If 450° < θ < 540° and sin θ = \(\frac{12}{13}\), then calculate sin (θ/2) and cos (θ/2)
Solution:

(iv) Prove that \(\frac{1}{\cos 290^{\circ}}+\frac{1}{\sqrt{3} \sin 250^{\circ}}=\frac{4}{\sqrt{3}}\)
Solution:

Question 3.
Prove that
(i) \(\frac{\sin 2 A}{(1-\cos 2 A)} \cdot \frac{(1-\cos A)}{\cos A}=\tan \frac{A}{2}\)
Solution:

(ii) \(\frac{\sin 2 x}{(\sec x+1)} \cdot \frac{\sec 2 x}{(\sec 2 x+1)}\) = \(\tan \frac{x}{2}\)
Solution:

(iii) \(\frac{\left(\cos ^{3} \theta-\cos 3 \theta\right)}{\cos \theta}+\frac{\left(\sin ^{3} \theta+\sin 3 \theta\right)}{\sin \theta}=\mathbf{3}\)
Solution:

Question 4.
(i) Show that cos A = \(\frac{\cos 3 A}{(2 \cos 2 A-1)}\). Hence find the value of cos 15°.
Solution:

(ii) Show that sin A = \(\frac{\sin 3 A}{1+2 \cos 2 A}\). Hence find the value of sin 15°.
Solution:

(iii) Prove that tan α = \(\frac{\sin 2 \alpha}{1+\cos 2 \alpha}\) and hence deduce the values tan 15° and tan 22\(\frac{1^{\circ}}{2}\).
Solution:

Question 5.
Prove that
(i) \(\frac{1}{\sin 10^{\circ}}-\frac{\sqrt{3}}{\cos 10^{\circ}}=4\)
Solution:

(ii) √3 cosec 20° – sec 20° = 4
Solution:

(iii) tan 9° – tan 27° – cot 27° + cot 9° = 4
Solution:

(iv) If \(\frac{\sin \alpha}{a}=\frac{\cos \alpha}{b}\), then prove that a sin 2α + b cos 2α = b
Solution:
Given \(\frac{\sin \alpha}{a}=\frac{\cos \alpha}{b}\)
b sin α = a cos α
L.H.S. = a sin 2α + b cos 2α
= a 2 sin α cos α + b (1 – 2 sin²α)
= 2 sin α (a cos α) + b – 2b sin²α
= 2 sin α (b sin α) + b – 2b sin²α
= 2b sin²α + b – 2b sin²α
= b

Question 6.
(i) In a ∆ABC; if \(\tan \frac{A}{2}=\frac{5}{6}\) and tan\(\tan \frac{B}{2}=\frac{20}{37}\), then show that \(\tan \frac{C}{2}=\frac{2}{5}\)
Solution:

(ii) If cos θ = \(\frac{5}{13}\) and 270° < θ < 360°, evaluate sin (θ/2) and cos (θ/2).
Solution:
cos θ = \(\frac{5}{13}\)
given 270 < θ < 360°
⇒ 135 < \(\frac{\theta}{2}\) < 180°
∴ θ lies in the fourth quadrant
θ/2 lies is second quadrant

(iii) If 180° < θ < 270° and sin θ = \(\frac{-4}{5}\) calculate sin[θ/2] cos[θ/2]
Solution:
Given sin θ = \(\frac{-4}{5}\)
⇒ cos θ = \(\frac{-3}{5}\)
given 180 < θ < 270
∴ θ in the III quadrant
Now 90 < \(\frac{\theta}{2}\) < 135
∴ \(\frac{\theta}{2}\) is in II quadrant

Question 7.
(i) Prove that \(\cos ^{2} \frac{\pi}{8}+\cos ^{2} \frac{3 \pi}{8}+\cos ^{2} \frac{5 \pi}{8}+\cos ^{2} \frac{7 \pi}{8}\) = 2
Solution:

(ii) Show that \(\cos ^{4}\left(\frac{\pi}{8}\right)+\cos ^{4}\left(\frac{3 \pi}{8}\right)+\cos ^{4}\left(\frac{5 \pi}{8}\right)+\cos ^{4}\left(\frac{7 \pi}{8}\right)\) = \(\frac{3}{2}\)
Solution:

III.

Question 1.
(i) If tan x + tan(x + \(\frac{\pi}{3}\)) + tan(x + \(\frac{2 \pi}{3}\)) = 3, show that tan 3x = 1
Solution:

(ii) Prove that \(\sin \frac{\pi}{5} \sin \frac{2 \pi}{5} \cdot \sin \frac{3 \pi}{5} \cdot \sin \frac{4 \pi}{5}\) = \(\frac{5}{16}\)
Solution:

(iii) Show that \(\cos ^{2}\left(\frac{\pi}{10}\right)+\cos ^{2}\left(\frac{2 \pi}{5}\right)+\cos ^{2}\left(\frac{3 \pi}{5}\right)\) + \(\cos ^{2}\left(\frac{9 \pi}{10}\right)\) = 2
Solution:

Question 2.
(i) \(\frac{1-\sec 8 \alpha}{1-\sec 4 \alpha}=\frac{\tan 8 \alpha}{\tan 2 \alpha}\)
Solution:

(ii) \(\left[1+\cos \frac{\pi}{10}\right]\left[1+\cos \frac{3 \pi}{10}\right]\left[1+\cos \frac{7 \pi}{10}\right]\) \(\left[1+\cos \frac{9 \pi}{10}\right]=\frac{1}{16}\)
Solution:

Question 3.
(i) Prove that \(\cos \frac{2 \pi}{7} \cdot \cos \frac{4 \pi}{7} \cdot \cos \frac{8 \pi}{7}=\frac{1}{8}\)
Solution:

(ii) \(\cos \frac{\pi}{11} \cdot \cos \frac{2 \pi}{11} \cdot \cos \frac{3 \pi}{11} \cdot \cos \frac{4 \pi}{11}\) \(\cos \frac{5 \pi}{11}=\frac{1}{32}\)
Solution:

Question 4.
(i) If cos α = \(\frac{3}{5}\) and cos β = \(\frac{5}{13}\) and α, β are acute angles, then prove that
(a) \(\sin ^{2}\left[\frac{\alpha-\beta}{2}\right]=\frac{1}{65}\)
(b) \(\cos ^{2}\left[\frac{\alpha+\beta}{2}\right]=\frac{16}{65}\)
Solution:

(ii) If A is not an integral multiple of π, prove that cos A . cos 2A . cos 4A . cos 8A = \(\frac{\sin 16 A}{16 \sin A}\) and hence deduce that \(\cos \frac{2 \pi}{15} \cdot \cos \frac{4 \pi}{15} \cdot \cos \frac{8 \pi}{15} \cdot \cos \frac{16 \pi}{15}=\frac{1}{16}\)
Solution:
Take 16 sin A {cos A . cos 2A . cos 4A . cos 8A}
= 8(2 sin A . cos A) cos 2A . cos 4A . cos 8A
= 8 sin 2A . cos 2A . cos 4A . cos 8A
= 4(2 sin 2A . cos 2A) . cos 4A . cos 8A
= 4 sin 4A . cos 4A . cos 8A
= 2 (2 sin 4A . cos 4A) . cos 8A
= 2 sin 8A . cos 8A
= sin (16A)
∴ 16 sin A {cos A . cos 2A . cos 4A . cos 8A} = sin (16A)
∴ cos A . cos 2A . cos 4A . cos 8A = \(\frac{\sin (16 A)}{16 \sin A}\)

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(c) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(c)

I.

Question 1.
Simplify the following.
(i) cos 100° cos 40° + sin 100° sin 40°
Solution:
cos 100° cos 40° + sin 100° sin 40° = cos(100° – 40°)
= cos 60°
= \(\frac{1}{2}\)

(ii) \(\frac{\cot 55 \cot 35}{\cot 55+\cot 35}\)
Solution:
\(\frac{\cot 55 \cot 35}{\cot 55+\cot 35}\) = cot(55 + 35)
= cot 90
= 0

(iii) \(\tan \left[\frac{\pi}{4}+\theta\right] \cdot \tan \left[\frac{\pi}{4}-\theta\right]\)
Solution:
\(\tan \left[\frac{\pi}{4}+\theta\right] \cdot \tan \left[\frac{\pi}{4}-\theta\right]\)
\(\left[\frac{1+\tan A}{1-\tan A}\right]\left[\frac{1-\tan A}{1+\tan A}\right]\) = 1

(iv) tan 75° + cot 75°
Solution:
tan 75° + cot 75°
= 2 + √3 + 2 – √3
= 4

(v) sin 1140° cos 390° – cos 780° sin 750°
Solution:
sin 1140° cos 390° – cos 780° sin 750°
= sin(3 × 360° + 60°) cos(360°+ 30°) – cos(2 × 360° + 60°) sin(2 × 360° + 30)
= sin 60° cos 30° – cos 60° sin 30°
= sin(60° – 30°)
= sin 30°
= \(\frac{1}{2}\)

Question 2.
(i) Express \(\frac{\sqrt{3} \cos 25+\sin 25}{2}\) as a sine of an angle.
Solution:
\(\frac{\sqrt{3} \cos 25+\sin 25}{2}\)
= \(\frac{\sqrt{3}}{2}\) cos 25° + \(\frac{1}{2}\) sin 25°
= sin 60° cos 25° + cos 60° sin 25°
= sin (60° + 25°)
= sin 85°

(ii) Express (cos θ – sin θ) as a cosine of an angle.
Solution:
cos θ – sin θ
Divide & multiply with √2
\(\frac{1}{\sqrt{2}}\) √2 (cos θ – sin θ)
= √2 \(\frac{1}{\sqrt{2}}\) cos θ – sin θ \(\frac{1}{\sqrt{2}}\)
= √2 [cos \(\frac{\pi}{4}\) cos θ – sin \(\frac{\pi}{4}\) sin θ]
= √2 \(\cos \left[\frac{\pi}{4}+\theta\right]\)

(iii) Express tan θ in terms of tan α, If sin (θ + α) = cos (θ + α).
Solution:
tan θ in term of tan α, if sin(θ + α) = cos (θ + α)
given sin(θ + α) = cos(θ + α)
sin θ cos α + cos θ sin α = cos θ cos α – sin θ sin α and cos θ cos α
\(\frac{\sin \theta \cos \alpha}{\cos \theta \cos \alpha}+\frac{\cos \theta \sin \alpha}{\cos \theta \cos \alpha}=\frac{\cos \theta \cos \alpha}{\cos \theta \cos \alpha}\) – \(\frac{\sin \theta \sin \alpha}{\cos \theta \cos \alpha}\)
tan θ + tan α = 1 – tan θ tan α
tan θ + tan θ tan α = 1 – tan α
tan θ (1 + tan α) = 1 – tan α
tan θ = \(\frac{1-\tan \alpha}{1+\tan \alpha}\)

Question 3.
(i) If tan θ = \(\frac{\cos 11^{\circ}+\sin 11^{\circ}}{\cos 11^{\circ}-\sin 11^{\circ}}\) and θ is the third quadrant find θ.
Solution:
Given tan θ = \(\frac{\cos 11^{\circ}+\sin 11^{\circ}}{\cos 11^{\circ}-\sin 11^{\circ}}\)
= \(\frac{1+\tan 11^{\circ}}{1-\tan 11^{\circ}}\)
= tan (45° + 11°)
= tan (56°)
tan θ = tan 56° = tan (180° + 50°) = tan 236°
∴ θ = 236°

(ii) If 0° < A, B < 90°, such that cos A = \(\frac{5}{13}\) and sin B = \(\frac{4}{5}\), find the value of sin(A – B).
Solution:

(iii) What is the value of tan 20° + tan 40° + √3 tan 20° tan 40°?
Solution:
consider 20° + 40° = 60°
tan (20° + 40°) = tan 60°
\(\frac{\tan 20^{\circ}+\tan 40^{\circ}}{1-\tan 20^{\circ} \tan 40^{\circ}}\) = √3
tan 20° + tan 40° = √3 – √3 tan 20° tan 40°
tan 20° + tan 40° + √3 tan 20° tan 40° = √3

(iv) Find the value of tan 56° – tan 11° – tan 56° tan 11°.
Solution:
consider 56° – 11° = 45°
tan (56° – 11) = tan 45°
\(\frac{\tan 56^{\circ}-\tan 11^{\circ}}{1+\tan 56^{\circ} \tan 11^{\circ}}\) = 1
tan 56° – tan 11 ° = 1 + tan 56° tan 11°
tan 56° – tan 11° – tan 56° tan 11° = 1

(v) Evaluate \(\sum \frac{\sin (A+B) \sin (A-B)}{\cos ^{2} A \cos ^{2} B}\); if none of cos A, cos B, cos C is zero.
Solution:

(vi) Evaluate \(\sum \frac{\sin (C-A)}{\sin C \sin A}\) if none of sin A, sin B, sin C is zero.
Solution:

Question 4.
Prove that
(i) cos 35° + cos 85° + cos 155° = 0
Solution:
cos 35° + cos 85° + cos 155°
= -cos 85° + 2 cos\(\left(\frac{35+155}{2}\right)\) cos\(\left(\frac{35-155}{2}\right)\)
= -cos 85° + 2 cos 85° \(\left(\frac{1}{2}\right)\)
= -cos 85° + cos 85°
= 0

(ii) tan 72° = tan 18° + 2 tan 54°
Solution:
cos A – tan A = \(\frac{1}{\tan A}\) – tan A
= \(\frac{1-\tan ^{2} A}{\tan A}\)
= \(\frac{2\left(1-\tan ^{2} A\right)}{2 \tan A}\)
= \(\frac{2}{\tan 2 A}\)
= 2 cot 2A
cot A = tan A + 2 cot 2A
put A = 18
cot 18° = tan 18° + 2 cot 36°
cot (90° – 72°) = tan 18° + 2 cot (90° – 54°)
tan 72° = tan 18° + 2 tan 54°

(iii) sin 750° cos 480° + cos 120° cos 60° = \(\frac{-1}{2}\)
Solution:
sin 750° = sin (2 × 360° + 30°)
= sin 30°
= \(\frac{1}{2}\)
cos 480° = cos (360° + 120°)
= cos 120°
= \(\frac{-1}{2}\)
L.H.S. = sin 750° cos 480° + cos 120° cos 60°
= \(\frac{1}{2}\left(\frac{-1}{2}\right)+\left(\frac{-1}{2}\right)\left(\frac{1}{2}\right)\)
= \(\frac{-1}{4}-\frac{1}{4}\)
= \(\frac{-1}{2}\)

(iv) cos A + cos(\(\frac{4 \pi}{3}\) – A) + cos(\(\frac{4 \pi}{3}\) + A) = o
Solution:
cos A + cos(\(\frac{4 \pi}{3}\) – A) + cos(\(\frac{4 \pi}{3}\) + A)
= cos A + 2 cos \(\frac{4 \pi}{3}\) cos A (∵ cos(A + B) + cos(A – B) = 2 cos A cos B)
= cos A + 2\(\left(\frac{-1}{2}\right)\) cos A
= cos A – cos A
= 0

(v) cos²θ + cos²(\(\frac{2 \pi}{3}\) + θ) + cos²(\(\frac{2 \pi}{3}\) – θ)
Solution:

Question 5.
Evaluate
(i) \(\sin ^{2} 82 \frac{1}{2}^{\circ}-\sin ^{2} 22 \frac{1^{\circ}}{2}\)
Solution:

(ii) \(\cos ^{2} 112 \frac{1}{2}^{\circ}-\sin ^{2} 52 \frac{1}{2}^{\circ}\)
Solution:

(iii) \(\sin ^{2}\left[\frac{\pi}{8}+\frac{A}{2}\right]-\sin ^{2}\left[\frac{\pi}{8}-\frac{A}{2}\right]\)
Solution:

(iv) \(\cos ^{2} 52 \frac{1}{2}^{\circ}-\sin ^{2} 22 \frac{1}{2}^{\circ}\)
Solution:

Question 6.
Find the minimum and maximum values of
(i) 3 cos x + 4 sin x
Solution:
a = 4, b = 3, c = 0
Minimum value = \(c-\sqrt{a^{2}+b^{2}}=\sqrt{16+9}=-5\)
Maximum value = \(c+\sqrt{a^{2}+b^{2}}=\sqrt{16+9}=5\)

(ii) sin 2x – cos 2x
Solution:
a = 1, b = -1, c = 0
minimum value = \(c-\sqrt{a^{2}+b^{2}}=-\sqrt{1+1}\) = -√2
maximum value = \(c+\sqrt{a^{2}+b^{2}}=\sqrt{1+1}=\sqrt{2}\)

Question 7.
Find the range of
(i) 7 cos x – 24 sin x + 5
Solution:

(ii) 13 cos x + 3√3 sin x – 4
Solution:

II.

Question 1.
(i) If cos α = \(\frac{-3}{5}\) and sin β = \(\frac{7}{25}\), where \(\frac{\pi}{2}\) < α < π and 0 < β < \(\frac{\pi}{2}\), then find the values of tan(α + β) and sin(α + β).
Solution:

(ii) If 0 < A < B < \(\frac{\pi}{4}\) and sin (A + B) = \(\frac{24}{25}\) and cos (A – B) = \(\frac{4}{5}\), then find the value of tan 2A.
Solution:

(iii) If A + B, A are acute angles such that sin (A + B) = \(\frac{24}{25}\) and tan A = \(\frac{3}{4}\), then find the value of cos B.
Solution:
sin (A + B) = \(\frac{24}{25}\) and (A + B) is acute angle

(iv) If tan α – tan β = m and cot α – cot β = n, then prove that cot (α – β) = \(\frac{1}{m}-\frac{1}{n}\)
Solution:

(v) If tan (α – β) = \(\frac{7}{24}\) and tan α = \(\frac{4}{3}\), where α and β are in the first quadrant prove that α – β = \(\frac{\pi}{2}\).
Solution:

Question 2.
(i) Find the expansion of sin (A + B – C).
Solution:
sin (A + B – C) = sin [(A + B) – C]
= sin (A + B). cos C – cos (A + B) sin C
= (sin A cos B + cos A sin B) cos C – (cos A cos B – sin A sin B) sin C
= sin A cos B cos C + cos A sin B cos C – cos A cos B sin C + sin A sin B sin C

(ii) Find the expansion of cos (A – B – C).
Solution:
cos (A – B – C) = cos {(A – B) – C}
= cos (A – B) cos C + sin (A – B) sin C
= (cos A cos B + sin A sin B) cos C + (sin A cos B – cos A sin B) sin C
= cos A cos B cos C + sin A sin B cos C + sin A cos B sin C – cos A sin B sin C

(iii) In a ΔABC, A is obtuse. If sin A = \(\frac{3}{5}\) and sin B = \(\frac{5}{13}\), then show that sin C = \(\frac{16}{65}\)
Solution:
Given sin A = \(\frac{3}{5}\)
cos²A = 1 – sin²A
= 1 – \(\frac{9}{25}\)
= \(\frac{16}{25}\)
cos A = ±\(\frac{4}{5}\)
A is obtuse ⇒ 90° < A < 180°
A tan in II quadrant ⇒ cos A is negative
∴ cos A = \(\frac{-4}{5}\),
Given sin β = \(\frac{5}{13}\)
cos²β = 1 – sin²β
= 1 – \(\frac{25}{169}\)
= \(\frac{144}{169}\)
cos β = ±\(\frac{5}{13}\)
β is acute ⇒ cos β is possible
sin β = \(\frac{12}{13}\)
A + B + C = 180°
C = 180° – (A + B)
sin C = sin (180° – (A + B))
= sin (A + B)
= sin A cos B + cos A sin B
= \(\left(\frac{3}{5}\right)\left(\frac{12}{13}\right)+\left(\frac{-4}{5}\right)\left(\frac{5}{13}\right)\)
= \(\frac{36-20}{65}\)
= \(\frac{16}{65}\)
∴ sin C = \(\frac{16}{65}\)

(iv) If \(\frac{\sin (\alpha+\beta)}{\sin (\alpha-\beta)}=\frac{a+b}{a-b}\), then prove that tan β = ab tan α
Solution:

III.

Question 1.
(i) If A – B = \(\frac{3 \pi}{4}\), then show that (1 – tan A) (1 + tan B) = 2.
Solution:
A – B = \(\frac{3 \pi}{4}\)
tan (A – B) = tan \(\frac{3 \pi}{4}\)
\(\frac{\tan A-\tan B}{1+\tan A \tan B}\) = -1
tan A – tan B = -1 – tan A tan B
1 = -tan A + tan B – tan A tan B
2 = 1 – tan A + tan B – tan A tan B
2 = (1 – tan A) – tan B (1 – tan A)
(1 – tan A) (1 – tan B) = 2

(ii) If A + B + C = \(\frac{\pi}{2}\) and none of A, B, C is an odd multiple of \(\frac{\pi}{2}\), then prove that
(a) cot A + cot B + cot C = cot A cot B cot C
(b) tan A tan B + tan B tan C + tan C tan A = 1 and hence, show that \(\sum \frac{\cos (B+C)}{\cos B \cos C}\) = 2
Solution:

Question 2.
(i) Prove that sin²α + cos²(α + β) + 2 sin α sin β cos(α + β) is independent of α.
Solution:
sin²α + cos²(α + β) + 2 sin α cos (α + β)
= sin²α + cos(α + β) (cos(α + β) + 2 sin α sin β)
= sin²α + cos(α + β) (cos α cos β – sin α sin β + 2 sin α sin β)
= sin²α + cos(α + β) (cos α cos β + sin α sin β)
= sin²α + cos(α + β) cos(α – β)
= sin²α + cos²β – sin²α
= cos²β

(ii) Prove that cos²(α – β) + cos²β – 2 cos(α – β) cos α cos β is independent of β.
Solution:
cos²(α – β) + cos²β – 2 cos (α – β) cos α cos β
= cos²(α – β) + cos²β – cos (α – β) [cos (α + β) + cos (α – β)]
= cos²(α – β) + cos²β – cos (α – β) cos (α + β) – cos²(α – β)
= cos²β – [cos²β – sin²α]
= cos²β – cos²β + sin²α
= sin²α

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(b)

I. Find the periods for the given 1 – 5 functions.

Question 1.
cos(3x + 5) + 7
Solution:
f(x) = cos(3x + 5) + 7
We know that the function g(x) = cos x for all x ∈ R has the period 2π.
Now f(x) = cos(3x + 5) + 7
We get that f(x) is periodic and the period of f is \(\frac{2 \pi}{|3|}=\frac{2 \pi}{3}\)

Question 2.
tan 5x
Solution:
The function g(x) = tan x periodic and π is the period.
∴ f(x) = tan 5x periodic and its period is \(\frac{\pi}{|5|}=\frac{\pi}{5}\)

Question 3.
\(\cos \left(\frac{4 x+9}{5}\right)\)
Solution:
The function h(x) = cos x for all x ∈ R has the period 2π.
Now f(x) = \(\cos \left(\frac{4 x}{5}+\frac{9}{5}\right)\) is periodic and period of f is \(\frac{2 \pi}{\left(\frac{4}{5}\right)}=\frac{5 \pi}{2}\)

Question 4.
|sin x|
Solution:
The function h(x) = sin x for all x ∈ R has the period 2π.
But f(x) = |sin x| is periodic and its period is π.
∵ f(x + π) = |sin(x + π)|
= |-sin x|
= sin x

Question 5.
tan(x + 4x + 9x + …… + n²x) (n any positive integer)
Solution:
tan(1² + 2² + 3² + …… + n²) x = \(\tan \left[\frac{n(n+1)(2 n+1)}{6}\right] x\)
period = \(\frac{6 \pi}{n(n+1)(2 n+1)}\)

Question 6.
Find a sine function whose period is \(\frac{2}{3}\)
Solution:
\(\frac{2 \pi}{|k|}=\frac{2}{3}\)
3π = |k|
∴ sin kx = sin 3πx

Question 7.
Find a cosine function whose period is 7.
Solution:
\(\frac{2 \pi}{|k|}\) = 7
\(\frac{2 \pi}{7}\) = |k|
∴ cos kx = cos \(\frac{2 \pi}{7}\) x

II. Sketch the graph of the following functions.

Question 1.
tan x between 0 and \(\frac{\pi}{4}\)
Solution:

Question 2.
cos 2x in [0, π]
Solution:

Question 3.
sin 2x in the interval (0, π)
Solution:

Question 4.
sin x in the interval [-π, +π]
Solution:

Question 5.
cos²x in [0, π]
Solution:

III.

Question 1.
Sketch the region enclosed by y = sin x, y = cos x and X-axis in the interval [0, π].
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(a)

I. Convert the following into the simplest form.

Question 1.
(i) tan(θ – 14π)
Solution:
= tan(14π – θ)
= tan(2 . (7π) – θ)
= tan θ

(ii) \(\cot \left(\frac{21 \pi}{2}-\theta\right)\)
Solution:
\(\cot \left(\frac{21 \pi}{2}-\theta\right)\)
= \(\cot \left(10 \pi+\left(\frac{\pi}{2}-\theta\right)\right)\)
= \(\cot \left(\frac{\pi}{2}-\theta\right)\)
= tan θ

(iii) cosec(5π + θ)
Solution:
cosec(5π + θ) = cosec(2π + (3π + θ))
= cosec(3π + θ)
= cosec(2π + (π + θ))
= cosec(π + θ)
= -cosec θ

(iv) sec(4π – θ?)
Solution:
sec(4π – θ)
= sec(2π + (2π – θ))
= sec(2π – θ)
= sec θ

Question 2.
Find the value of each of the following.
(i) sin(-405°)
Solution:
sin(-405°) = -sin(360° + 45)
= -sin 45°
= \(-\frac{1}{\sqrt{2}}\)

(ii) \(\cos \left(-\frac{7 \pi}{2}\right)\)
Solution:
\(\cos \left(-\frac{7 \pi}{2}\right)\)
= -cos 630°
= -cos(360° + 270°)
= -cos 270°
= -cos(180° + 90°)
= -cos 90°
= 0

(iii) sec(2100°)
Solution:
sec(2100°) = sec (5 × 360° + 300°)
= sec 300°
= sec(360 – 60°)
= sec 60°
= 2

(iv) cot(-315°)
Solution:
cot(-315°) = -cot 315°
= -cot(360° – 45°)
= -cot 45°
= 1

Question 3.
Evaluate.
(i) cos² 45° + cos² 135° + cos² 225° + cos² 315°
Solution:

(ii) \(\sin ^{2} \frac{2 \pi}{3}+\cos ^{2} \frac{5 \pi}{6}-\tan ^{2} \frac{3 \pi}{4}\)
Solution:

(iii) cos 225° – sin 225° + tan 495° – cot 495°
Solution:
cos (180° + 45°) – sin(180° + 45°) + tan(360° + 135°) – cot(360° + 135°)
= -cos 45° + sin 45° – tan 135° + cot 135°
= \(-\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}+1-1\)
= 0

(iv) (cos θ – sin θ) if (a) θ = \(\frac{7 \pi}{4}\) (b) θ = \(\frac{11 \pi}{3}\)
Solution:

Question 4.
(i) If sin θ = \(\frac{-1}{3}\) and θ does not lie in the third quadrant, find the values of (a) cos θ (b) cot θ
Solution:
∵ sin θ = \(\frac{-1}{3}\) and sin θ is negative and θ does not lie in the IIIrd quadrant.
⇒ θ lies in IV quadrant.
∴ In the IV quadrant, cos θ is positive and cot θ is negative.

(ii) If cos θ = t (0 < t < 1) and θ does not lie in the first quadrant, find the values of (a) sin θ (b) tan θ.
Solution:
cos θ = t, (0 < t < 1)
⇒ cos θ is positive and θ does not lie in the first quadrant.
⇒ θ lies in IV quadrant.
(a) sin θ = \(-\sqrt{1-\cos ^{2} \theta}=-\sqrt{1-t^{2}}\)
(b) tan θ = \(\frac{\sin \theta}{\cos \theta}=\frac{-\sqrt{1-t^{2}}}{t}\)

(iii) Find the value of sin 330°. cos 120° + cos 210°. sin 300°.
Solution:
sin 330°. cos 120° + cos 210°. sin 300°
= sin(360° – 30°) . cos(180° – 60°) + cos(180° + 30°) . sin(360° – 60°)
= (-sin 30°) (-cos 60°) + (-cos 30°) (-sin 60°)
= sin 30° cos 60° + cos 30° sin 60°
= sin(30° + 60°) [∵ sin A cos B + cos A sin B = sin(A + B)]
= sin (90°)
= 1

(iv) If cosec θ + cot θ = \(\frac{1}{3}\), find cos θ and determine the quadrant in which θ lies.
Solution:
cosec θ + cot θ = \(\frac{1}{3}\)
⇒ cosec θ – cot θ = 3 (∵ cosec²θ – cot²θ = 1)
∴ 2 cosec θ = \(\frac{1}{3}\) + 3 = \(\frac{10}{3}\)
∴ cosec θ = \(\frac{5}{3}\) and sin θ = \(\frac{3}{5}\)
2 cot θ = \(\frac{1}{3}\) – 3 = \(\frac{-8}{3}\)
∴ cot θ = \(\frac{-4}{3}\), tan θ = \(\frac{-3}{4}\)
cos θ = (cot θ) . sin θ = \(\left(\frac{-4}{3}\right)\left(\frac{3}{5}\right)=\frac{-4}{5}\)
∵ sin θ is +ve and cos θ is -ve
⇒ θ lies in II quadrant.

Question 5.
(i) If sin α + cosec α = 2. Find the value of sinⁿα + cosecⁿα, n ∈ z.
Solution:
Given sin α + cosec α = 2
Squaring on both sides
sin²α + cosec²α + 2 = 4
sin²α + cosec²α = 2
sin α + cosec α = 2
Cubing on both sides
sin³α + cosec³α + 3 sin α . cosec α (sin α + cosec α) = 8
sin³α + cosec³α + 3(2) = 8
sin³α + cosec³α = 8 – 6
sin³α + cosec³α = 2
similarly sinⁿα + cosecⁿα = -2

(ii) If sec θ + tan θ = 5. Find the quadrant in which θ lies and find the value of sin θ.
Solution:
sec θ + tan θ = 5
⇒ sec θ – tan θ = \(\frac{1}{5}\) (∵ sec²θ – tan²θ = 1)
2 sec θ = 5 + \(\frac{1}{5}\) = \(\frac{26}{5}\)
sec θ = \(\frac{26}{10}=\frac{13}{5}\)
Aquir 2 tan θ = 5 – \(\frac{1}{5}\) = \(\frac{24}{5}\)
tan θ = \(\frac{24}{10}=\frac{12}{5}\)
Now sin θ = \(\frac{\tan \theta}{\sec \theta}=\frac{\frac{12}{5}}{\frac{13}{5}}=\frac{12}{13}\)
tan θ is +ve, sec θ is +ve
⇒ θ lies in the first quadrant.

II.

Question 1.
Prove that
(i) \(\frac{\cos (\pi-A) \cdot \cot \left(\frac{\pi}{2}+A\right) \cos (-A)}{\tan (\pi+A) \tan \left[\frac{3 \pi}{2}+A\right] \sin (2 \pi-A)}\) = cos A
Solution:
\(\frac{\cos (\pi-A) \cdot \cot \left(\frac{\pi}{2}+A\right) \cos (-A)}{\tan (\pi+A) \tan \left[\frac{3 \pi}{2}+A\right] \sin (2 \pi-A)}\)
= \(\frac{-\cos A(-\tan A) \cos A}{\tan A(-\cot A)(-\sin A)}\)
= cos A

(ii) \(\frac{\sin (3 \pi-A) \cos \left(A-\frac{\pi}{2}\right) \tan \left(\frac{3 \pi}{2}-A\right)}{{cosec}\left(\frac{13 \pi}{2}+A\right) \sec (3 \pi+A) \cot \left(A-\frac{\pi}{2}\right)}\) = cos⁴A
Solution:

(iii) sin 780° sin 480° + cos 240°. cos 300° = \(\frac{1}{2}\)
Solution:
sin(2(360°) + 60°) . sin(360° + 120°) + cos(270° – 30°) . cos(360° – 60°)
= sin 60° . sin 120° – sin 30° . cos 60°
= \(\frac{\sqrt{3}}{2} \cdot \frac{\sqrt{3}}{2}-\frac{1}{2} \cdot \frac{1}{2}\)
= \(\frac{3}{4}-\frac{1}{4}\)
= \(\frac{1}{2}\)

(iv) \(\frac{\sin 150^{\circ}-5 \cos 300^{\circ}+7 \tan 225^{\circ}}{\tan 135^{\circ}+3 \sin 210^{\circ}}\) = -2
Solution:

(v) cot(\(\frac{\pi}{20}\)) . cot(\(\frac{3\pi}{20}\)) . cot(\(\frac{5\pi}{20}\)) . cot(\(\frac{7\pi}{20}\)) . cot(\(\frac{9\pi}{20}\)) = 1
Solution:
L.H.S. = cot(\(\frac{\pi}{20}\)) . cot(\(\frac{3\pi}{20}\)) . cot(\(\frac{5\pi}{20}\)) . cot(\(\frac{7\pi}{20}\)) . cot(\(\frac{9\pi}{20}\))
= cot (9°) cot (27°) cot (45°) cot (63°) cot (81°)
= cot (9°) cot (27°) (1) cot (90° – 27°) cot (90° – 9°)
= cot (9°) cot (27°) tan 27° tan 9°
= (tan 9° cot 9°) (tan 27° cot 27°)
= (1) (1)
= 1

Question 2.
Simplify.
(i) \(\frac{\sin \left(-\frac{11 \pi}{3}\right) \tan \left(\frac{35 \pi}{6}\right) \sec \left(-\frac{7 \pi}{3}\right)}{\cot \left(\frac{5 \pi}{4}\right) {cosec}\left(\frac{7 \pi}{4}\right) \cos \left(\frac{17 \pi}{6}\right)}\)
Solution:

(ii) If tan 20° = p, prove that \(\frac{\tan 610^{\circ}+\tan 700^{\circ}}{\tan 560^{\circ}-\tan 470^{\circ}}=\frac{1-p^{2}}{1+p^{2}}\)
Solution:
Given tan 20° = p
L.H.S. = \(\frac{\tan 610^{\circ}+\tan 700^{\circ}}{\tan 560^{\circ}-\tan 470^{\circ}}\)

(iii) If α, β are complementary angles such that b sin α = a, then find the value of (sin α cos β – cos α sin β).
Solution:
∵ α, β are complementary angles
⇒ α + β = 90°
⇒ β = 90° – α
Now sin α cos β – cos α sin β
= sin (α – β)
= sin [(α – (90° – α)]
= sin [2α – 90°]
= -sin (90° – 2α)
= -cos 2α
= -(1 – 2 sin²α) (∵ cos 2α = 1 – 2 sin²α)
= \(-1+2\left(\frac{a}{b}\right)^{2}\) (∵ sin α = \(\frac{a}{b}\))
= \(\frac{-b^{2}+2 a^{2}}{b^{2}}\)
= \(\frac{2 a^{2}-b^{2}}{b^{2}}\)

Question 3.
(i) If cos A = cos B = \(-\frac{1}{2}\), A does not lie in the second quadrant and B does not lie in the third quadrant, then find the value of \(\frac{4 \sin B-3 \tan A}{\tan B+\sin A}\)
Solution:
∵ cos A = \(-\frac{1}{2}\) and A does not lie in second quadrant.
⇒ A lies in the third quadrant, (∵ cos A is -ve)
and cos B = \(-\frac{1}{2}\) and B does not lie in third quadrant.
⇒ B lies in second quadrant.
∵ cos A = \(-\frac{1}{2}\) and A lies in third quadrant.
⇒ A = 240°
∵ cos B = \(-\frac{1}{2}\) and B lies in second quadrant.
⇒ B = 120°

(ii) If 8 tan A = -15 and 25 sin B = -7 and neither A nor B is in the fourth quadrant, then show that sin A cos B + cos A sin B = \(\frac{-304}{425}\)
Solution:
8 tan A = -15 ⇒ tan A = \(\frac{-15}{8}\)
25 sin B = -7 ⇒ sin B = \(-\frac{7}{25}\)
Given neither A nor B is in the fourth quadrant.
Clearly, A is the second quadrant B is the third quadrant
sin A cos B + cos A sin B = \(\left(\frac{15}{17}\right)\left(\frac{-24}{25}\right)+\left(\frac{-8}{17}\right)\left(\frac{-7}{25}\right)\)
= \(\frac{-360}{425}+\frac{56}{425}\)
= \(\frac{-304}{425}\)

(iii) If A, B, C, D are angles of a cyclic quadrilateral, then prove that
(a) sin A – sin C = sin D – sin B
(b) cos A + cos B + cos C + cos D = 0
Solution:
∵ A, B, C, D are angles of a cyclic quadrilateral.
⇒ A + C = 180° and B + D = 180° ……..(1)
C = 180° – A and D = 180° – B
(a) L.H.S. = sin A – sin C
= sin A – sin(180° – A)
= sin A – sin A
= 0
R.H.S. = sin D – sin B
= sin(180°- B) – sin B
= sin B – sin B
= 0
∴ L.H.S. = R.H.S.
i.e., sin A – sin C = sin D – sin B
(b) L.H.S. = cos A + cos B + cos C + cos D
= cos A + cos B + cos(180° – A) + cos(180° – B)
= cos A + cos B – cos A – cos B
= 0
∴ cos A + cos B + cos C + cos D = 0

Question 4.
(i) If a cos θ – b sin θ = c, then show that a sin θ + b sin θ = \(\pm \sqrt{a^{2}+b^{2}-c^{2}}\).
Solution:
a cos θ – b sin θ = c
let a sin θ + b cos θ = x
by squaring and adding
(a cos θ – b sin θ)² + (a sin θ + b cos θ)² = c² + x²
a² cos²θ + b² sin²θ – 2ab sin θ cos θ + a² sin²θ + b² cos²θ + 2ab sin θ cos θ = c² + x²
a² + b² = c² + x²
a² + b² – c² = x²
x = \(\pm \sqrt{a^{2}+b^{2}-c^{2}}\)
∴ a sin θ + b cos θ = \(\pm \sqrt{a^{2}+b^{2}-c^{2}}\)

(ii) If 3 sin A + 5 cos A = 5, then show that 5 sin A – 3 cos A = ±3.
Solution:
3 sin A + 5 cos A = 5
Let 5 sec A – 3 cos A = x
by squaring and adding
(3 sin A + 5 cos A)² + (5 sin A – 3 cos A)² = 5² + x²
9 sin² A + 25 cos² A + 30 sin A cos A + 25 sin² A + 9 cos² A – 30 sin A cos A = 25
9 + 25 = 25 + x²
x² = 9
x = ±3
∴ 5 sin A – 3 cos A = ±3

(iii) If tan²θ = (1 – e²), show that sec θ + tan³θ . cosec θ = (2 – e²)^3/2.
Solution:
tan²θ = 1 – e²
sec²θ = 1 + tan²θ = 2 – e²

III. Prove the following.

Question 1.
(i) \(\frac{(\tan \theta+\sec \theta-1)}{(\tan \theta-\sec \theta+1)}=\frac{1+\sin \theta}{\cos \theta}\)
Solution:

(ii) (1 + cot θ – cosec θ) (1 + tan θ + sec θ) = 2.
Solution:

(iii) 3(sin θ – cos θ)⁴ + 6(sin θ + cos θ)² + 4(sin⁶θ + cos⁶θ) = 13.
Solution:
(sin θ – cos θ)² = sin²θ + cos²θ – 2 sin θ . cos θ
= 1 – 2 sin θ cos θ
(sin θ – cos θ)⁴ = (1 – 2 sin θ cos θ)²
= 1 + 4 sin²θ cos²θ – 4 sin θ cos θ ……(1)
(sin θ + cos θ)² = sin²θ + cos²θ + 2 sin θ cos θ
= 1 + 2 sin θ cos θ …….(2)
sin⁶θ + cos⁶θ = (sin²θ +cos²θ)³ – 3 sin²θ cos²θ (sin²θ + cos²θ)
= 1 – 3 sin²θ cos²θ ……..(3)
L.H.S. = 3(1 + 4 sin²θ cos²θ – 4 sin θ cos θ) + 6(1 + 2 sin θ cos θ) + 4(1 – 3 sin²θ cos²θ)
= 3 + 12 sin²θ cos²θ – 12 sin θ cos θ + 6 + 12 sin θ cos θ + 4 – 12 sin²θ cos²θ
= 3 + 6 + 4
= 13
= R.H.S.

Question 2.
(i) Prove that (sin θ + cosec θ)² + (cos θ + sec θ)² – (tan²θ + cot²θ) = 7.
Solution:
L.H.S. = (sin θ + cosec θ)² + (cos θ + sec θ)² – (tan²θ + cot²θ)
= (sin²θ + cosec²θ + 2 sin θ cosec θ) + (cos²θ + sec²θ + 2 cos θ sec θ) – (tan²θ + cot²θ)
= (sin²θ + cos²θ) + (1 + cot²θ) + (1 + tan²θ) + 4 – tan²θ – cot²θ
= 1 + 1 + 1 + 4
= 7

(ii) cos⁴α + 2 cos²α \(\left(1-\frac{1}{\sec ^{2} \alpha}\right)\) = (1 – sin⁴α)
Solution:
L.H.S. = cos⁴α + 2 cos²α \(\left(1-\frac{1}{\sec ^{2} \alpha}\right)\)
= cos⁴α + 2 cos²α (1 – cos²α)
= cos²α [cos²α + 2 sin²α]
= (1 – sin²α) [cos²α + sin²α + sin²α]
= (1 – sin²α) (1 + sin²α)
= 1 – sin⁴α

(iii) \(\frac{(1+\sin \theta-\cos \theta)^{2}}{(1+\sin \theta+\cos \theta)^{2}}=\frac{1-\cos \theta}{1+\cos \theta}\)
Solution:

(iv) If \(\frac{2 \sin \theta}{(1+\cos \theta+\sin \theta)}\) = x, then find the value of \(\frac{(1-\cos \theta+\sin \theta)}{(1+\sin \theta)}\)
Solution:

Question 3.
Eliminate θ from the following.
(i) x = a cos³θ; y = b sin³θ
Solution:

(ii) x = a cos⁴θ; y = b sin⁴θ
Solution:

(iii) x = a(sec θ + tan θ); y = b(sec θ – tan θ)
Solution:
\(\frac{x}{a}\) = sec θ + tan θ; \(\frac{y}{b}\) = (sec θ – tan θ)
\(\frac{x}{a} \times \frac{y}{b}\) = (sec θ + tan θ) (sec θ – tan θ)
\(\frac{x y}{a b}\) = sec²θ – tan²θ
\(\frac{x y}{a b}\) = 1
xy = ab

(iv) x = cot θ + tan θ; y = sec θ – cos θ
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Products of Vectors Solutions Exercise 5(c) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Products of Vectors Solutions Exercise 5(c)

I.

Question 1.
Compute \([\overline{\mathbf{i}}-\overline{\mathbf{j}} \overline{\mathbf{j}}-\overline{\mathbf{k}} \overline{\mathbf{k}}-\overline{\mathbf{i}}]\)
Solution:

Question 2.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-2 \overline{\mathbf{j}}-3 \overline{\mathbf{k}}, \overline{\mathbf{b}}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}}\), \(\bar{c}=\bar{i}+3 \bar{j}-2 \bar{k}\), then compute \(\overline{\mathbf{a}} \cdot(\overline{\mathbf{b}} \times \overline{\mathbf{c}})\).
Solution:

Question 3.
If \(\bar{a}\) = (1, -1, -6), \(\bar{b}\) = (1, -3, 4) and \(\bar{c}\) = (2, -5, 3), then compute the following
(i) \(\overline{\mathbf{a}} \cdot(\bar{b} \times \bar{c})\)
(ii) \(\overline{\mathbf{a}} \times(\overline{\mathbf{b}} \times \overline{\mathbf{c}})\)
(iii) \((\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}\)
Solution:

Question 4.
Simplify the following.
(i) \((\bar{i}-2 \bar{j}+3 \bar{k}) \times(2 i+j-\bar{k}) \cdot(\bar{j}+\bar{k})\)
(ii) \((2 \bar{i}-3 \bar{j}+\bar{k}) \cdot(\bar{i}-\bar{j}+2 \bar{k}) \cdot(2 \bar{i}+\bar{j}+\bar{k})\)
Solution:

Question 5.
Find the volume of the parallelopiped having coterminous edges.
\(\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}, \overline{\mathbf{i}}-\overline{\mathbf{j}}\) and \(\overline{\mathbf{i}}+\mathbf{2} \overline{\mathbf{j}}-\overline{\mathbf{k}}\)
Solution:
Let \(\overline{\mathrm{a}}=\overline{\mathrm{i}}+\overline{\mathrm{j}}+\overline{\mathrm{k}}, \overline{\mathrm{b}}=\overline{\mathrm{i}}-\overline{\mathrm{j}}\) and \(\overline{\mathrm{c}}=\overline{\mathrm{i}}+2 \overline{\mathrm{j}}-\overline{\mathrm{k}}\)
Volume of the parallelopiped = \([(\bar{a} \bar{b} \bar{c})]\)
= \(\left|\begin{array}{ccc}
1 & 1 & 1 \\
1 & -1 & 0 \\
1 & 2 & -1
\end{array}\right|\)
= 1(1 – 0) – 1(-1 – 0) + 1(2 + 1)
= 1 + 1 + 3
= 5 cubic units.

Question 6.
Find t for which the vectors \(\mathbf{2} \overline{\mathbf{i}}-\mathbf{3} \overline{\mathbf{j}}+\overline{\mathbf{k}}\), \(\bar{i}+2 \mathbf{j}-3 \bar{k}\) and \(\bar{j}-t \bar{k}\) are coplanar.
Solution:

Question 7.
For non-coplanar vectors, \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) determine p for which the vector \(\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}, \overline{\mathbf{a}}+\mathbf{p} \overline{\mathbf{b}}+\mathbf{2} \overline{\mathbf{c}}\) and \(-\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}\) are coplanar.
Solution:

Question 8.
Determine λ, for which the volume of the parallelopiped having coterminous edges \(\bar{i}+\bar{j}\), \(3 \overline{\mathbf{i}}-\overline{\mathbf{j}}\) and \(3 \bar{j}+\lambda \bar{k}\) is 16 cubic units.
Solution:

Question 9.
Find the volume of the tetrahedron having the edges \(\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}, \quad \mathbf{i}-\overline{\mathbf{j}}\) and \(\bar{i}+2 \bar{j}+\bar{k}\).
Solution:

Question 10.
Let \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) be non-coplanar vectors and \(\bar{\alpha}=\bar{a}+2 \bar{b}+3 c, \quad \bar{\beta}=2 \bar{a}+\bar{b}-2 c\) and \(\bar{\gamma}=3 \bar{a}-7 \bar{c}\), then find \(\left[\begin{array}{lll}
\bar{\alpha} & \bar{\beta} & \bar{\gamma}
\end{array}\right]\).
Solution:

Question 11.
Let \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) be non-coplanar vectors. If \(|2 \bar{a}-\bar{b}+3 \bar{c}|, \bar{a}+\bar{b}-2 \bar{c},|\bar{a}+\bar{b}-3 \bar{c}|\) = \(\lambda[\overline{\mathbf{a}} \overline{\mathbf{b}} \overline{\mathbf{c}}]\), then find the value of λ.
Solution:

Question 12.
Let \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) be non-coplanar vectors, if \(\left[\begin{array}{lll}
\bar{a}+2 \bar{b} & 2 \bar{b}+\bar{c} & 5 \bar{c}+\bar{a}
\end{array}\right]\) = \(\lambda\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]\), then find λ.
Solution:

Question 13.
If \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) are non-coplanar vectors, then find the value of \(\frac{(\bar{a}+2 \bar{b}-\bar{c}) \cdot[(\bar{a}-\bar{b}) \times(\bar{a}-\bar{b}-\bar{c})]}{[\bar{a} \bar{b} \bar{c}]}\)
Solution:

Question 14.
If \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) are mutually perpendicular unit vectors, then find the value of \(\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]^{2}\).
Solution:

Question 15.
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are non-zero vectors and \(\overline{\mathbf{a}}\) is perpendicular to both \(\overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\). If \(|\overline{\mathbf{a}}|\) = 2, \(|\overline{\mathbf{b}}|\) = 3, \(|\overline{\mathbf{c}}|\) = 4 and \((\bar{b}, \bar{c})=\frac{2 \pi}{3}\), then find \(|[\bar{a} \bar{b} \bar{c}]|\).
Solution:

Question 16.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are unit coplanar vectors, then find \(\left[\begin{array}{lll}
2 \bar{a}-\bar{b} & 2 \bar{b}-\bar{c} & 2 \bar{c}-\bar{a}
\end{array}\right]\)
Solution:

II.

Question 1.
If \(\left[\begin{array}{lll}
\bar{b} & \bar{c} & \bar{d}
\end{array}\right]+\left[\begin{array}{lll}
\bar{c} & \bar{a} & \bar{d}
\end{array}\right]+\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{d}
\end{array}\right]\) = \(\left[\begin{array}{lll}
\overline{\mathbf{a}} & \overline{\mathbf{b}} & \overline{\mathbf{c}}
\end{array}\right]\) then show that the points with position vectors \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) and \(\bar{d}\) are coplanar.
Solution:

Question 2.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\) non-coplanar vectors, then prove that the four points with position vectors \(2 \bar{a}+3 \bar{b}-\bar{c}\), \(\overline{\mathrm{a}}-2 \overline{\mathrm{b}}+3 \overline{\mathrm{c}}, 3 \overline{\mathrm{a}}+4 \overline{\mathrm{b}}-2 \overline{\mathrm{c}}\) and \(\bar{a}-6 \bar{b}+6 \bar{c}\) are coplanar.
Solution:
Suppose A, B, C, D are the given points.

The vectors \(\overrightarrow{\mathrm{AB}}, \overrightarrow{\mathrm{AC}}, \overrightarrow{\mathrm{AD}}\) are coplanar.
The given points A, B, C, D are coplanar.

Question 3.
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\) are non-zero and non- collinear vectors and θ ≠ 0, is the angle between \(\overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\). If \((\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}\) = \(\frac{1}{3}|\bar{b}||\bar{c}|\bar{a}|\), then find sin θ.
Solution:

Question 4.
Find the volume of the tetrahedron whose vertices are (1, 2, 1), (3, 2, 5), (2, -1, 0) and (-1, 0, 1).
Solution:
Let ‘O’ be the given A, B, C, D be the vertices of the ten tetrahedrons. Then

Question 5.
Show that \((\bar{a}+\bar{b}) \cdot(\bar{b}+\bar{c}) \times(\bar{c}+\bar{a})\) = \(2\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]\)
Solution:

Question 6.
Show that equation of the plane passing through the points with position vectors. \(3 \bar{i}-5 \bar{j}-\overline{\mathbf{k}},-\overline{\mathbf{i}}+5 \bar{j}+7 \overline{\mathbf{k}}\) and parallel to the vector \(3 \overline{\mathbf{i}}-\overline{\mathbf{j}}+7 \overline{\mathbf{k}}\) is 3x + 2y – z = 0.
Solution:
The given plane passes through the points A, B (i.e.,) \(3 \bar{i}-5 \bar{j}-\overline{\mathbf{k}},-\overline{\mathbf{i}}+5 \bar{j}+7 \overline{\mathbf{k}}\) and parallel to the vector \(3 \overline{\mathbf{i}}-\overline{\mathbf{j}}+7 \overline{\mathbf{k}}\)

= x(70 + 8) – y(-28 – 24) + z(4 – 30)
= 78x + 52y – 26z
= 26(3x + 2y – z)
\(\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]\) = \(\left|\begin{array}{rrr}
3 & -5 & -1 \\
-4 & 10 & 8 \\
3 & -1 & 7
\end{array}\right|\)
= 3(70 + 8) + 5(-28 – 24) – 1(4 – 30)
= 234 – 260 + 26
= 0
Equation of the required plane is 26(3x + 2y – z) = 0
i.e., 3x + 2y – z = 0

Question 7.
Prove that \(\overline{\mathbf{a}} \times[\overline{\mathbf{a}} \times(\overline{\mathbf{a}} \times \overline{\mathbf{b}})]\) = \((\overline{\mathbf{a}} \cdot \overline{\mathbf{a}})(\overline{\mathbf{b}} \times \overline{\mathbf{a}})\)
Solution:

Question 8.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) and \(\bar{d}\) are coplanar vectors, then show that \((\bar{a} \times \bar{b}) \times(\bar{c} \times \bar{d})=0\).
Solution:
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) and \(\bar{d}\) are coplanar
⇒ \(\overline{\mathbf{a}} \times \overline{\mathbf{b}}\) is perpendicular to the plane π.
similarly \(\bar{c} \times \bar{d}\) is perpendicular to the plane π.
\(\bar{a} \times \bar{b}\) and \(\bar{c} \times \bar{d}\) are parallel vectors.
⇒ \((\bar{a} \times \bar{b}) \times(\bar{c} \times \bar{d})\) = 0.

Question 9.
Show that \((\overline{\mathrm{a}} \times \overline{\mathrm{b}}) \times(\overline{\mathrm{a}} \times \overline{\mathrm{c}}) \cdot \overline{\mathrm{d}}=[\overline{\mathrm{a}} \overline{\mathrm{b}} \overline{\mathrm{c}}](\overline{\mathrm{a}} \cdot \overline{\mathrm{d}})\).
Solution:

Question 10.
Show that \(\bar{a} \cdot[(\bar{b}+\bar{c}) \times(\bar{a}+\bar{b}+\bar{c})]=0\)
Solution:

Question 11.
Find λ in order that the four points A(3, 2, 1), B(4, λ, 5), C(4, 2, -2) and D(6, 5, -1) be coplanar.
Solution:

Question 12.
Find the vector equation of the plane passing through the intersection of planes \(\bar{r} \cdot(2 \bar{i}+2 \bar{j}-3 \bar{k})=7, \bar{r} \cdot(2 \bar{i}+5 \bar{j}+3 \bar{k})=9\) and through the point (2, 1, 3)
Solution:

Question 13.
Find the equation of the plane passing through (a, b, c) and parallel to the plane \(\bar{r} \cdot(\bar{i}+\bar{i}+\bar{k})=2\).
Solution:
Given equation plane is \(\bar{r} \cdot(\bar{i}+\bar{i}+\bar{k})=2\)
Let \(\bar{r}=x \bar{i}+y \bar{j}+z \bar{k}\)
∴ \(\bar{r} \cdot(\bar{i}+\bar{i}+\bar{k})=2\)
\((x \bar{i}+y \bar{j}+z \bar{k}) \cdot(i+j+k)=2\)
x + y + z = 2
Required plane equation is x + y + z = k …….(1)
Equation (1) passes through (a, b, c)
∴ a + b + c = k
Substitute ‘k’ in equation (1)
∴ x + y + z = a + b + c

Question 14.
Find the shortest distance between the lines \(\bar{r}=6 \bar{i}+2 \bar{j}+2 \bar{k}+\lambda, \bar{i}-2 \bar{j}+2 \bar{k}\) and \(\bar{r}=-4 \bar{j}-\bar{k}+\mu=3 \bar{j}-2 \bar{j}-2 \bar{k}\).
Solution:
The first line passes through point A(6, 2, 2) and is parallel to the vector b = i – 2j + 2k.
Second line passes through the point C(-4, 0, -1) and is parallel to the vector d = 3i – 2j – 2k

Question 15.
Find the equation of the plane passing through the line of intersection of the planes \(\bar{r} \cdot(\bar{i}+\bar{j}+\bar{k})=1\) and \(\bar{r} \cdot(2 \bar{i}+3 \bar{i}-\bar{k})+4=0\) and parallel to X-axis.
Solution:
Given the equation of planes are

Since it is parallel to X-axis.

Question 16.
Prove that the four points \(4 \bar{i}+5 \bar{j}+\bar{k}\), \(-(\overline{\mathbf{j}}+\overline{\mathbf{k}}), 3 \overline{\mathbf{i}}+9 \overline{\mathbf{j}}+4 \overline{\mathbf{k}}\) and \(-4 \bar{i}+4 \bar{j}+4 \bar{k}\) are coplanar.
Solution:
Let ‘O’ be the origin. A, B, C, D be the given points. Then

Question 17.
If \(\bar{a}, \bar{b}, \bar{c}\) are non – copianar, then show that the vectors \(\overline{\mathbf{a}}-\overline{\mathbf{b}}, \overline{\mathbf{b}}+\overline{\mathbf{c}}\), \(\overline{\mathbf{c}}+\overline{\mathbf{a}}\) are coplanar.
Solution:

Question 18.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are the position vectors of the points A, B and C respectively, then prove that the vector \(\overline{\mathbf{a}} \times \overline{\mathbf{b}}+\overline{\mathbf{b}} \times \overline{\mathbf{c}}+\overline{\mathbf{c}} \times \overline{\mathbf{a}}\) is perpendicular to the plane of ∆ABC.
Solution:

III.

Question 1.
Show that \((\bar{a} \times(\bar{b} \times \bar{c}) \times \bar{c})=(\bar{a} \cdot \bar{c})(\bar{b} \times \bar{c})\) and \((\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \cdot(\overline{\mathbf{a}} \times \overline{\mathbf{c}})+(\overline{\mathbf{a}} \cdot \overline{\mathbf{b}})(\overline{\mathbf{a}} \cdot \overline{\mathbf{c}})\) = \((\overline{\mathbf{a}} \cdot \overline{\mathbf{a}})(\overline{\mathbf{b}} \cdot \overline{\mathbf{c}})\)
Solution:

Question 2.
If A = (1, -2, -1), B = (4, 0, -3), C = (1, 2, -1) and D = (2, -4, -5), find the distance between AB and CD.
Solution:

Question 3.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-2 \overline{\mathbf{j}}+\overline{\mathbf{k}}, \overline{\mathbf{b}}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\) and \(\overline{\mathbf{c}}=\overline{\mathbf{i}}+2 \overline{\mathbf{j}}-\overline{\mathbf{k}}\), find \(\bar{a} \times(\bar{b} \times \bar{c})\) and \(|(\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}|\).
Solution:

Question 4.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-2 \overline{\mathbf{j}}-3 \overline{\mathbf{k}}, \overline{\mathbf{b}}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}}\) and \(\bar{c}=\bar{i}+3 \bar{j}-2 \bar{k}\), verift that \(\overline{\mathbf{a}} \times(\overline{\mathbf{b}} \times \overline{\mathbf{c}}) \neq(\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}\)
Solution:

From (1) and (2), we get
\(\overline{\mathbf{a}} \times(\overline{\mathbf{b}} \times \overline{\mathbf{c}}) \neq(\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}\)
i.e., vector multiplication is not associative.

Question 5.
If \(\overline{\mathbf{a}}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}-3 \overline{\mathbf{k}}, \overline{\mathbf{b}}=\overline{\mathbf{i}}-\mathbf{2} \mathbf{j}+\overline{\mathbf{k}}\), \(\bar{c}=-\bar{i}+\bar{j}-4 \bar{k}\) and \(\overline{\mathbf{d}}=\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\), then compute \(|(\overline{\mathrm{a}} \times \overline{\mathrm{b}}) \times(\overline{\mathrm{c}} \times \overline{\mathrm{d}})|\)
Solution:

Question 6.
If A = (1, a, a²), B = (1, b, b²) and C = (1, c, c²) are non-coplanar vectors and \(\left|\begin{array}{lll}
a & a^{2} & 1+a^{3} \\
b & b^{2} & 1+b^{3} \\
c & c^{2} & 1+c^{3}
\end{array}\right|\) = 0, then show that abc + 1 = 0
Solution:
\(\bar{A}, \bar{B}, \bar{C}\) are non-coplanar vectors.
∆ = \(\left|\begin{array}{lll}
1 & a & a^{2} \\
1 & b & b^{2} \\
1 & c & c^{2}
\end{array}\right|\) ≠ 0 ………..(1)

∆ + (abc) ∆ = 0; ∆(1 + abc) = 0
∆ ≠ 0 ⇒ 1 + abc = 0

Question 7.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are non-zero vectors, then \(|(\overline{\mathbf{a}} \times \mathbf{b} \cdot \overline{\mathbf{c}})|=|\overline{\mathbf{a}}||\mathbf{b}||\overline{\mathbf{c}}|\) \(\Leftrightarrow \overline{\mathbf{a}} \cdot \overline{\mathbf{b}}=\overline{\mathbf{b}} \cdot \overline{\mathbf{c}}=\overline{\mathbf{c}} \cdot \overline{\mathbf{a}}=\mathbf{0}\)
Solution:

Question 8.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-\mathbf{2} \overline{\mathbf{j}}+3 \overline{\mathbf{k}}, \quad \mathbf{b}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\), \(\bar{c}=\overline{\mathbf{i}}+\overline{\mathbf{j}}+2 \overline{\mathbf{k}}\) then find \(|(\bar{a} \times \bar{b}) \times \bar{c}|\) and \(|\overline{\mathbf{a}} \times(\mathbf{b} \times \mathbf{c})|\).
Solution:

Question 9.
If \(|\bar{a}|=1,|\bar{b}|=1,|\bar{c}|=2\) and \(\bar{a} \times(a \times \bar{c})+\bar{b}=0\) then find the angle between \(\bar{a}\) and \(\bar{c}\).
Solution:

Question 10.
Let \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-\overline{\mathbf{k}}, \quad \overline{\mathbf{b}}=\mathbf{x} \overline{\mathbf{i}}+\overline{\mathbf{j}}+(\mathbf{1}-\mathbf{x}) \overline{\mathbf{k}}\) and \(\bar{c}=y \bar{i}+x \bar{j}+(1+x-y) \bar{k}\), prove that the scalar triple product \(\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]\) is independent of both x and y.
Solution:

Question 11.
Let \(\overline{\mathbf{b}}=\mathbf{2} \overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}}, \overline{\mathbf{c}}=\overline{\mathbf{i}}+\mathbf{3} \overline{\mathbf{k}}\). If \(\overline{\mathrm{a}}\) is a unit vector then find the maximum value of \(\left[\begin{array}{lll}
\overline{\mathbf{a}} & \overline{\mathbf{b}} & \bar{c}
\end{array}\right]\).
Solution:
Let \(\bar{a}=x \bar{i}+y \bar{j}+z \bar{k}\) and x² + y² + z² = 1
∵ \(\overline{\mathrm{a}}\) unit vector

Question 12.
Let \(\overline{\mathrm{a}}=\overline{\mathrm{i}}-\overline{\mathrm{i}}, \overline{\mathrm{b}}=\overline{\mathrm{i}}-\overline{\mathrm{k}}, \overline{\mathrm{c}}=\overline{\mathrm{k}}-\overline{\mathrm{i}}\) Find unit vector \(\bar{d}\) such that \(\overline{\mathrm{a}} \cdot \overline{\mathrm{d}}=0=[\bar{b} \overline{\mathrm{c}} \overline{\mathrm{d}}]\)
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Products of Vectors Solutions Exercise 5(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Products of Vectors Solutions Exercise 5(b)

I.

Question 1.
If \(|\overline{\mathbf{p}}|=2,|\overline{\mathbf{q}}|=3\) and \((\bar{p}, \bar{q})=\frac{\pi}{6}\), then find \(|\bar{p} \times \bar{q}|^{2}\).
Solution:

Question 2.
If \(\overline{\mathbf{a}}=2 \overline{\mathbf{i}}-\overline{\mathbf{j}}+\overline{\mathbf{k}}\) and \(\overline{\mathbf{b}}=\overline{\mathbf{i}}-3 \overline{\mathbf{j}}-5 \overline{\mathbf{k}}\), then find \(|\overline{\mathbf{a}} \times \overline{\mathbf{b}}|\).
Solution:

Question 3.
If \(\bar{a}=2 \bar{i}-3 \bar{j}+\overline{\mathbf{k}}\) and \(\bar{b}=\bar{i}+4 \bar{j}-2 \bar{k}\), then find \((\overline{\mathbf{a}}+\overline{\mathbf{b}}) \times(\overline{\mathbf{a}}-\overline{\mathbf{b}})\).
Solution:

Question 4.
If \(4 \bar{i}+\frac{2 p}{3} \bar{j}+p \bar{k}\) is parallel to the vector \(\overline{\mathbf{i}}+2 \overline{\mathbf{j}}+3 \overline{\mathbf{k}}\), find p.
Solution:

Question 5.
Compute \(\overline{\mathbf{a}} \times(\overline{\mathbf{b}}+\overline{\mathbf{c}})+\overline{\mathbf{b}} \times(\overline{\mathbf{c}}+\overline{\mathbf{a}})+\overline{\mathbf{c}} \times(\overline{\mathbf{a}}+\overline{\mathbf{b}})\)
Solution:

Question 6.
If \(\overline{\mathbf{p}}=\mathbf{x} \overline{\mathbf{i}}+\mathbf{y} \overline{\mathbf{j}}+\mathbf{z} \overline{\mathbf{k}}\), find the value of \(|\overline{\boldsymbol{p}} \times \overline{\mathbf{k}}|^{2}\)
Solution:

Question 7.
Compute \(2 \bar{j} \times(3 \bar{i}-4 \bar{k})+(\bar{i}+2 \hat{j}) \times \bar{k}\).
Solution:

Question 8.
Find a unit vector perpendicular to both \(\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\) and \(2 \bar{i}+\bar{j}+3 \bar{k}\).
Solution:

Question 9.
If θ is the angle between the vectors \(\overline{\mathbf{i}}+\overline{\mathbf{j}}\) and \(\overline{\mathbf{j}}+\overline{\mathbf{k}}\), then find sin ?.
Solution:

Question 10.
Find the area of the parallelogram having \(\bar{a}=2 \bar{j}-\bar{k}\) and \(\overline{\mathbf{b}}=-\overline{\mathbf{i}}+\overline{\mathbf{k}}\) as adjacent sides.
Solution:
Vector area of the parallelogram having \(\bar{a}=2 \bar{j}-\bar{k}\) and \(\overline{\mathbf{b}}=-\overline{\mathbf{i}}+\overline{\mathbf{k}}\) as adjacent sides.

Question 11.
Find the area of the parallelogram, whose diagonals are \(3 \overline{\mathbf{i}}+\overline{\mathbf{j}}-2 \overline{\mathbf{k}}\) and \(\bar{i}-3 \bar{j}+4 \bar{k}\).
Solution:

Question 12.
Find the area of the triangle having \(3 \bar{i}+4 \bar{j}\) and \(-5 \bar{i}+7 \bar{j}\) as two of its sides.
Solution:

Question 13.
Find unit vector perpendicular to the plane determined by the vectors \(\bar{a}=4 \bar{i}+3 \bar{j}-\bar{k}\) and \(\overline{\mathbf{b}}=2 \tilde{i}-6 \overline{\mathbf{j}}-3 \overline{\mathbf{k}}\).
Solution:

Question 14.
Find the area of the triangle whose vertices are A(1, 2, 3), B(2, 3, 1) and C(3, 1, 2).
Solution:
Suppose \(\bar{i}, \bar{j}, \bar{k}\) are unit vectors along the co-ordinate axes.
Position vectors of A, B, C are

II.

Question 1.
If \(\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}=\overline{\mathbf{0}}\), then prove that \(\overline{\mathbf{a}} \times \overline{\mathbf{b}}=\overline{\mathbf{b}} \times \overline{\mathbf{c}}=\overline{\mathbf{c}} \times \overline{\mathbf{a}}\).
Solution:

Question 2.
If \(\overline{\mathbf{a}}=2 \bar{i}+\bar{j}-\bar{k}, \quad \bar{b}=-\bar{i}+2 \bar{j}-4 \bar{k}\) and \(\overline{\mathbf{c}}=\overline{\mathbf{i}}+\mathbf{j}+\overline{\mathbf{k}}\), then find \((\bar{a} \times \bar{b}) \cdot(\bar{b} \times \bar{c})\).
Solution:

Question 3.
Find the vector area and the area of the parallelogram having \(\overline{\mathbf{a}}=\overline{\mathbf{i}}+2 \overline{\mathbf{j}}-\overline{\mathbf{k}}\) and \(\overline{\mathbf{b}}=2 \overline{\mathbf{i}}-\overline{\mathbf{j}}+\mathbf{2} \overline{\mathbf{k}}\) as adjacent sides.
Solution:

Question 4.
If \(\overline{\mathbf{a}} \times \overline{\mathbf{b}}=\overline{\mathbf{b}} \times \overline{\mathbf{c}} \neq \overline{\mathbf{0}}\), show that, \(\overline{\mathbf{a}}+\overline{\mathbf{c}}=\mathbf{p} \overline{\mathbf{b}}\), where p is some scalar.
Solution:

Question 5.
Let \(\overline{\mathbf{a}}\) and \(\overline{\mathbf{b}}\) be vectors, satisfying \(|\overline{\mathbf{a}}|=|\overline{\mathbf{b}}|=5\) and \((\bar{a}, \bar{b})=45^{\circ}\). Find the area of the triangle having \(\overline{\mathbf{a}}-\mathbf{2} \overline{\mathbf{b}}\) and \(3 \bar{a}+2 \bar{b}\) as two of its sides.
Solution:

Question 6.
Find the vector having magnitude ?6 units and perpendicular to both \(\mathbf{2} \overline{\mathbf{i}}-\overline{\mathbf{k}}\) and \(\mathbf{3} \overline{\mathbf{i}}-\overline{\mathbf{j}}-\overline{\mathbf{k}}\).
Solution:

Question 7.
Find a unit vector perpendicular to the plane determined by the points P(1, -1, 2), Q(2, 0, -1) and R(0, 2, 1).
Solution:

Question 8.
If \(\overline{\mathbf{a}} \cdot \overline{\mathbf{b}}=\overline{\mathbf{a}} \cdot \overline{\mathbf{c}}\) and \(\overline{\mathbf{a}} \times \overline{\mathrm{b}}=\overline{\mathrm{a}} \times \overline{\mathrm{c}}, \overline{\mathbf{a}} \neq 0\), then show that \(\overline{\mathbf{b}}=\overline{\mathbf{c}}\).
Solution:

Question 9.
Find a vector of magnitude 3 and perpendicular to both the vector \(\overline{\mathbf{b}}=2 \bar{i}-2 \bar{j}+\bar{k}\) and \(\bar{c}=2 \bar{i}+2 \bar{j}+3 \bar{k}\).
Solution:

Question 10.
If \(|\overline{\mathbf{a}}|\) = 13, \(|\overline{\mathbf{b}}|\) = 5 and \(\overline{\mathbf{a}} \cdot \overline{\mathbf{b}}=\mathbf{6 0}\), then find \(|\overline{\mathbf{a}} \times \overline{\mathbf{b}}|\).
Solution:

Question 11.
Find a unit vector perpendicular to the plane passing through the points (1, 2, 3), (2, -1, 1) and (1, 2, -4).
Solution:
Let ‘O’ be the origin and let A, B, C be the given points.

III.

Question 1.
If \(\overline{\mathbf{a}}\), \(\overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\) represent the vertices A, B and C respectively of ∆ABC, then prove that \(|(\overline{\mathbf{a}} \times \overline{\mathbf{b}})+(\overline{\mathbf{b}} \times \overline{\mathbf{c}})+(\overline{\mathbf{c}} \times \overline{\mathbf{a}})|\) is twice the area of ∆ABC.
Solution:

Question 2.
If \(\overline{\mathbf{a}}=2 \overline{\mathbf{i}}+3 \overline{\mathbf{j}}+4 \overline{\mathbf{k}}, \overline{\mathbf{b}}=\overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}}\) and \(\overline{\mathbf{c}}=\overline{\mathbf{i}}-\overline{\mathbf{j}}+\overline{\mathbf{k}}\), then compute \(\overline{\mathbf{a}} \times(\overline{\mathbf{b}} \times \overline{\mathbf{c}})\) and verify that it is perpendicular to \(\bar{a}\).
Solution:

Question 3.
If \(\overline{\mathbf{a}}=7 \overline{\mathbf{i}}-2 \overline{\mathbf{j}}+3 \overline{\mathbf{k}}, \overline{\mathbf{b}}=2 \overline{\mathbf{i}}+8 \overline{\mathbf{k}}\) and \(\overline{\mathbf{c}}=\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\) then compute \(\overline{\mathbf{a}} \times \mathbf{b}, \overline{\mathbf{a}} \times \overline{\mathbf{c}}\) and \(\bar{a} \times(\bar{b}+\bar{c})\). Verify whether cross product is distributive over vector addition.
Solution:

Question 4.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}, \overline{\mathbf{c}}=\overline{\mathbf{j}}-\overline{\mathbf{k}}\), then find vector \(\overline{\mathbf{b}}\) such that \(\overline{\mathbf{a}} \times \overline{\mathbf{b}}=\overline{\mathbf{c}}\) and \(\bar{a} \cdot \bar{b}=3\).
Solution:

Question 5.
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are three vectors of equal magnitudes and each of them is inclined at an angle of 60° to the others. If \(|\bar{a}+\bar{b}+\bar{c}|=\sqrt{6}\), then find \(|\overline{\mathbf{a}}|\).
Solution:

Question 6.
For any two vectors \(\bar{a}\) and \(\bar{b}\), show that \(\left(1+|\bar{a}|^{2}\right)\left(1+|\bar{b}|^{2}\right)\) = \(|\mathbf{1}-\overline{\mathbf{a}} \cdot \overline{\mathbf{b}}|^{2}+|\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{a}} \times \overline{\mathbf{b}}|^{2}\)
Solution:

Question 7.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are unit vectors such that \(\overline{\mathbf{a}}\) is perpendicular to the plane of \(\overline{\mathbf{b}}, \overline{\mathbf{c}}\) and the angle between \(\overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\) is \(\frac{\pi}{3}\), then find \(|\bar{a}+\bar{b}+\bar{c}|\).
Solution:
Given that \(|\bar{a}|=|\bar{b}|=|\bar{c}|=1\)

Question 8.
\(\overline{\mathbf{a}}=3 \overline{\mathbf{i}}-\overline{\mathbf{j}}+2 \overline{\mathbf{k}}, \overline{\mathbf{b}}=-\overline{\mathbf{i}}+3 \overline{\mathbf{j}}+2 \overline{\mathbf{k}}\), \(\bar{c}=4 \bar{i}+5 \bar{j}-2 \bar{k}\) and \(\bar{d}=\bar{i}+3 \bar{j}+5 \bar{k}\) then compute the following.
(i) \((\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times(\bar{c} \times \bar{d})\) and
(ii) \((\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \cdot \overline{\mathbf{c}}-(\overline{\mathbf{a}} \times \overline{\mathbf{d}}) \cdot \overline{\mathbf{b}}\)
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Products of Vectors Solutions Exercise 5(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Products of Vectors Solutions Exercise 5(a)

I.

Question 1.
Find the angle between the vectors \(\bar{i}+2 \bar{j}+3 \bar{k}\) and \(3 \bar{i}-\bar{j}+2 \bar{k}\).
Solution:
Let \(\overline{\mathrm{a}}=\overline{\mathrm{i}}+2 \overline{\mathrm{j}}+3 \overline{\mathrm{k}}\) and \(\overline{\mathrm{b}}=3 \overline{\mathrm{i}}-\overline{\mathrm{j}}+2 \overline{\mathrm{k}}\) and ‘θ’ be the angle between them (i.e.,) \((\bar{a}, \bar{b})\) = θ

Question 2.
If the vectors \(\mathbf{2} \overline{\mathbf{i}}+\lambda \overline{\mathbf{j}}-\overline{\mathbf{k}}\) and \(4 \bar{i}-2 \bar{j}+2 \bar{k}\) are perpendicular to each other, then find λ.
Solution:

Question 3.
For what values of λ, the vectors \(\overline{\mathbf{i}}-\lambda \overline{\mathbf{j}}+2 \overline{\mathbf{k}}\) and \(8 \overline{\mathbf{i}}+6 \overline{\mathbf{j}}-\overline{\mathbf{k}}\) are at right angles?
Solution:

Question 4.
\(\overline{\mathbf{a}}=2 \overline{\mathbf{i}}-\overline{\mathbf{j}}+\overline{\mathbf{k}}, \overline{\mathbf{b}}=\overline{\mathbf{i}}-3 \overline{\mathbf{j}}-5 \overline{\mathbf{k}}\). Find the vector C such that \(\overline{\mathbf{a}}\), \(\overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\) form the sides of a triangle.
Solution:

Question 5.
Find the angle between the planes \(\bar{r} \cdot(2 \bar{i}-\bar{j}+2 \bar{k})=3\) and \(\overline{\mathrm{r}} \cdot(3 \overline{\mathrm{i}}+6 \bar{j}+\bar{k})=4\).
Solution:

Question 6.
Let \(\overline{\mathbf{e}}_{1}\) and \(\overline{\mathbf{e}}_{2}\) be unit vectors makingangle θ. If \(\frac{1}{2}\left|\bar{e}_{1}-\bar{e}_{2}\right|=\sin \lambda \theta\), then find λ.
Solution:

Question 7.
Let \(\overline{\mathbf{a}}=\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\) and \(\overline{\mathbf{b}}=\mathbf{2} \overline{\mathbf{i}}+3 \overline{\mathbf{j}}+\overline{\mathbf{k}}\). Find
(i) The projection vector of \(\overline{\mathbf{b}}\) on \(\overline{\mathbf{a}}\) and its magnitude.
(ii) The vector components of \(\overline{\mathbf{b}}\) in the direction of a and perpendicular to \(\overline{\mathbf{a}}\).
Solution:

Question 8.
Find the equation of the plane through the point (3, -2, 1) and perpendicular to the vector (4, 7, -4).
Solution:

Question 9.
If \(\overline{\mathbf{a}}=2 \bar{i}+2 \bar{j}-3 \bar{k}\); \(\overline{\mathbf{b}}=3 \overline{\mathbf{i}}-\overline{\mathbf{j}}+2 \overline{\mathbf{k}}\), then find the angle between \(2 \overline{\mathbf{a}}+\overline{\mathbf{b}}\) and \(\bar{a}+2 \bar{b}\).
Solution:

II.

Question 1.
Find unit vector parallel to the XOY- plane and perpendicular to the vector \(4 \bar{i}-3 \bar{j}+\bar{k}\).
Solution:
Any vector parallel to XOY-plane will be of the form \(p \bar{i}+q \bar{j}\)
∴ The vector parallel to the XOY-plane and perpendicular to the vector \(4 \bar{i}-3 \bar{j}+\bar{k}\) is \(3 \bar{i}+4 \bar{j}\)
Its magnitude = \(|3 \bar{i}+\overline{4 j}|=\sqrt{9+16}=5\)
∴ Unit vector parallel to the XOY-plane and perpendicular to the vector \(4 \bar{i}-3 \bar{j}+\bar{k}\) is \(\pm \frac{(3 \overline{\mathrm{i}}+4 \overline{\mathrm{j}})}{5}\)

Question 2.
If \(\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathrm{c}}=0,|\overline{\mathbf{a}}|=3,|\overline{\mathbf{b}}|=5\) and \(|\bar{c}|=7\), then find the angle between \(\overline{\mathbf{a}}\) and \(\overline{\mathbf{b}}\).
Solution:

Question 3.
If \(|\overline{\mathbf{a}}|\) = 2, \(|\overline{\mathbf{b}}|\) = 3 and \(|\overline{\mathbf{c}}|\) = 4 and each of \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) is perpendicular to the sum of the other two vectors, then find the magnitude of \(\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}\).
Solution:

Question 4.
Find the equation of the plane passing through the point \(\overline{\mathbf{a}}=\mathbf{2} \overline{\mathbf{i}}+3 \bar{j}-\overline{\mathbf{k}}\) and perpendicular to the vector \(3 \bar{i}-2 \bar{j}-2 \bar{k}\) and the distance of this plane from the origin.
Solution:
Equation of the plane passing through the point \(\overline{\mathbf{a}}=\mathbf{2} \overline{\mathbf{i}}+3 \bar{j}-\overline{\mathbf{k}}\) and perpendicular to the vector \(\bar{n}=3 \bar{i}-2 \bar{j}-2 \bar{k}\) is

Question 5.
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) and \(\overline{\mathbf{d}}\) are the position vectors of four coplanar points such that \((\mathbf{a}-\overline{\mathbf{d}}) \cdot(\bar{b}-\bar{c})=(\bar{b}-\bar{d}) \cdot(\bar{c}-\bar{a})=0\). Show that the point \(\bar{d}\) represents the orthocentre of the triangle with \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) as its vertices.
Solution:
Position vectors of A, B, C, D are \(\bar{a}\), \(\bar{b}\), \(\bar{c}\) and \(\bar{d}\) respectively.


⇒ BD is perpendicular to AC
∴ BD is another altitude of ∆ABC.
Altitudes AD and BD intersect at D.
∴ D is the orthocentre of ∆ABC.

III.

Question 1.
Show that the points (5, -1, 1), (7, -4, 7), (1, -6, 10) and (-1, -3, 4) are the vertices of a rhombus.
Solution:
Let A(5, -1, 1), B(7, -4, 7), C(1, -6, 10) and D(-1, -3, 4) are the given points.

∵ AB = BC = CD = DA = 7 units
AC ≠ BD
∴ A, B, C, D points are the vertices of a rhombus.

Question 2.
Let \(\bar{a}=4 \bar{i}+5 \bar{j}-\bar{k}, \quad \bar{b}=\bar{i}-4 \bar{j}+5 \bar{k}\) and \(\overline{\mathbf{c}}=\mathbf{3} \overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}}\). Find the vector which is perpendicular to both \(\overline{\mathbf{a}}\) and \(\overline{\mathbf{b}}\) and whose magnitude is twenty one times the magnitude of \(\overline{\mathbf{c}}\).
Solution:

Question 3.
G is the centroid of ΔABC and a, b, c are the lengths of the sides BC, CA and AB respectively prove that a² + b² + c² = 3 (OA² + OB² + OC²) – 9(OG)² where O is any point.
Solution:
Given that \(\overline{\mathrm{BC}}=\overline{\mathrm{a}}, \overline{\mathrm{CA}}=\overline{\mathrm{b}}, \overline{\mathrm{AB}}=\overline{\mathrm{c}}\)
Let ‘O’ be the origin and let \(\overline{\mathrm{OA}}=\overline{\mathrm{p}}, \overline{\mathrm{OB}}=\overline{\mathrm{q}} \text { and } \overline{\mathrm{OC}}=\overline{\mathrm{r}}\)
Then P.V. of the centroid of ΔABC is

From (1) and (2)
∴ a² + b² + c² = 3(OA² + OB² + OC²) – 9(OG)².

Question 4.
A line makes angles θ₁, θ₂, θ₃, and θ₄ with the diagonals of a cube. Show that cos²θ₁ + cos²θ₂ + cos²θ₃ + cos²θ₄ = \(\frac{4}{3}\).
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Addition of Vectors Solutions Exercise 4(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Addition of Vectors Solutions Exercise 4(b)

I.

Question 1.
Find the vector equation of the line passing through the point \(2 \bar{i}+3 \bar{j}+\bar{k}\) and parallel to the vector \(4 \bar{i}-2 \bar{j}+3 \bar{k}\).
Solution:

Question 2.
OABC is a parallelogram. If \(\overline{O A}=\bar{a}\) and \(\overline{O C}=\bar{c}\), find the vector equation of the side BC.
Solution:

Question 3.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are the position vectors of the vertices A, B and C respectively of ∆ABC, theind the vector equation of the median through the vertex A.
Solution:

Question 4.
Find the vertor equation of the line joining the points \(2 \bar{i}+\bar{j}+3 \bar{k}\) and \(-4 \bar{i}+3 \bar{j}-\bar{k}\).
Solution:

Question 5.
Find the vector equation of the plane passing through the points \(\overline{\mathbf{i}}-2 \overline{\mathbf{j}}+5 \overline{\mathbf{k}},-5 \overline{\mathbf{j}}-\overline{\mathbf{k}} \text { and }-3 \overline{\mathbf{i}}+5 \overline{\mathbf{j}}\).
Solution:

Question 6.
Find the vector equation of the plane through the points (0, 0, 0), (0, 5, 0) and (2, 0, 1).
Solution:

II.

Question 1.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are noncoplanar find the point of intersection of the line passing through the points \(2 \bar{a}+3 \bar{b}-\bar{c}\), \(3 \bar{a}+4 \bar{b}-2 \bar{c}\) with the line joining the points \(\bar{a}-2 \bar{b}+3 \bar{c}, \bar{a}-6 \bar{b}+6 \bar{c}\).
Solution:

Question 2.
ABCD is a trapezium in which AB and CD are parallel. Prove by vector methods, that the mid points of the sides AB, CD and the intersection of the diagonals are collinear.
Solution:




⇒ M, P, N are collinear
Hence the midpoints of parallel sides of a trapezium and the point of intersection of the diagonals are collinear.

Question 3.
In a quadrilateral ABCD, if the midpoints of one pair of opposite sides and the point of intersection of the diagonals are collinear, using vector methods, prove that the quadrilateral ABCD is a trapezium.
Solution:

III.

Question 1.
Find the vector equation of the plane which passes through the points \(2 \bar{i}+4 \bar{j}+2 \bar{k}, 2 \bar{i}+3 \bar{j}+5 \bar{k}\) and parallel to the vector \(3 \overline{\mathbf{i}}-2 \overline{\mathbf{j}}+\overline{\mathbf{k}}\). Also find the point where this plane meets the line joining the points \(2 \overline{\mathbf{i}}+\overline{\mathbf{j}}+3 \overline{\mathbf{k}}\) and \(4 \bar{i}-2 \bar{j}+3 \bar{k}\).
Solution:

Question 2.
Find the vector equation of the plane passing through points \(4 \overline{\mathbf{i}}-3 \overline{\mathbf{j}}-\overline{\mathbf{k}}\), \(3 \overline{\mathbf{i}}+7 \overline{\mathbf{j}}-10 \overline{\mathbf{k}}\) and \(2 \bar{i}+5 \bar{j}-7 \bar{k}\), and show that the point \(\overline{\mathbf{i}}+2 \bar{j}-3 \overline{\mathbf{k}}\) lies in the plane.
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Addition of Vectors Solutions Exercise 4(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Addition of Vectors Solutions Exercise 4(a)

I.

Question 1.
ABCD is a Parallelogram. If L and M are the middle points of BC and CD, respectively, then find (i) AL and AM in terms of AB and AD (ii) λ, if AM = λ AD – LM.
Solution:

Question 2.
In ∆ABC, P, Q, and R are the midpoints of the sides AB, BC, and CA respectively. If D is any point.
(i) then express \(\overline{\mathrm{DA}}+\overline{\mathrm{DB}}+\overline{\mathrm{DC}}\) interms of \(\overline{D P}\), \(\overline{D Q}\) and \(\overline{D R}\).
(ii) If \(\overline{\mathbf{P A}}+\overline{\mathbf{Q B}}+\overline{\mathbf{R C}}=\bar{\alpha}\) then find \(\bar{\alpha}\)
Solution:

Question 3.
Let \(\overline{\mathbf{a}}=\overline{\mathbf{i}}+2 \overline{\mathbf{j}}+3 \overline{\mathbf{k}}\) and \(\overline{\mathbf{b}}=\mathbf{3} \overline{\mathbf{i}}+\overline{\mathbf{j}}\). Find the unit vector in the direction of \(\overline{\mathbf{a}}+\overline{\mathbf{b}}\).
Solution:

Question 4.
If the vectors \(-3 \overline{\mathbf{i}}+4 \bar{j}+\lambda \overline{\mathbf{k}}\) and \(\mu \bar{i}+8 \bar{i}+6 \bar{k}\) are coilinear vectors , then find λ and µ.
Solution:

Question 5.
ABCDE is a pentagon. If the sum of the vectors \(\overline{\mathrm{AB}}, \overline{\mathrm{AE}}, \overline{\mathrm{BC}}, \overline{\mathrm{DC}}, \overline{\mathrm{ED}}\) and \(\overline{\mathbf{A C}}\) is λ \(\overline{\mathbf{A C}}\), then find the value of λ.
Solution:

Question 6.
If the position vectors of the points A, B and C are \(-2 \overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}},-4 \overline{\mathbf{i}}+2 \overline{\mathbf{j}}+2 \overline{\mathbf{k}}\) and \(6 \bar{i}-3 \bar{j}-13 \bar{k}\) respectively and \(\overline{\mathbf{A B}}=\lambda \overline{\mathrm{AC}}\), then find the value of λ.
Solution:

Question 7.
If \(\overline{\mathrm{OA}}=\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}, \overline{\mathrm{AB}}=3 \bar{i}-2 \overline{\mathbf{j}}+\overline{\mathbf{k}}\), \(\overline{B C}=\bar{i}+2 \bar{j}-2 \bar{k}\) and \(\overline{C D}=2 \bar{i}+\bar{j}+3 \bar{k}\), then find the vector \(\overline{O D}\).
Solution:

Question 8.
\(\overline{\mathbf{a}}=2 \overline{\mathbf{i}}+5 \overline{\mathbf{j}}+\overline{\mathbf{k}}\) and \(\bar{b}=4 \bar{i}+m \bar{j}+n \bar{k}\) are collinear vectors, then find m and n.
Solution:

Question 9.
Let \(\bar{a}=2 \bar{i}+4 \overline{\mathbf{j}}-5 \overline{\mathbf{k}}, \bar{b}=\hat{i}+\bar{j}+\bar{k}\) and \(\bar{c}=\bar{j}+2 \bar{k}\). Find the unit vector in the opposite direction of \(\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}\).
Solution:

Question 10.
Is the triangle formed by the vectors \(3 \bar{i}+5 \bar{j}+2 \bar{k}, 2 \bar{i}-3 \bar{j}-5 \bar{k}\) and \(-5 \bar{i}-2 \bar{j}+3 \bar{k}\) equilateral?
Solution:

Question 11.
If α, β and γ be the angles made by the vector \(3 \bar{i}-6 \bar{i}+2 \bar{k}\) with the positive directions of the co-ordinate axes, then find cos α, cos β, cos γ.
Solution:
Unit vectors along the co-ordinate axes are respectively \(\bar{i}, \bar{j}, \bar{k}\).

Question 12.
Find the angles made by the straight line passing through the points (1, -3, 2) and (3, -5, 1) with the co-ordinate axes.
Solution:
Unit vectors along the co-ordinate axes are respectively \(\bar{i}, \bar{j}, \bar{k}\).
Let A(1, -3, 2) and B(3, -5, 1) be two given points.
Let ‘O’ be the origin. Then

II.

Question 1.
If \(\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}=\alpha \overline{\mathbf{d}}, \overline{\mathbf{b}}+\overline{\mathbf{c}}+\overline{\mathbf{d}}=\beta \overline{\mathbf{a}}\) and \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathrm{c}}\) are non-coplanar vectors, then show that \(\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}+\overline{\mathbf{d}}=\mathbf{0}\).
Solution:

Question 2.
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are non-coplanar vectors. Prove that the following four points are coplanar.
(i) \(-\overline{\mathbf{a}}+4 \overline{\mathbf{b}}-3 \bar{c}, \quad 3 \bar{a}+2 \bar{b}-5 \bar{c}\), \(-3 \overline{\mathbf{a}}+8 \overline{\mathbf{b}}-5 \overline{\mathbf{c}},-3 \overline{\mathbf{a}}+2 \overline{\mathbf{b}}+\overline{\mathbf{c}}\)
Solution:
Let ‘O’ be the origin and A, B, C, D be the four points.


4 + 2x + 2y = 0 ……..(1)
-2 – 4x + 2y = 0 ……..(2)
-2 + 2x – 4y = 0 …….(3)
Solve (1) and (3)
6y + 6 = 0 ⇒ y = -1
Substitute in (1)
2x + 2 (-1) + 4 = 0
⇒ 2x + 2 = 0
⇒ x = -1
Substitute x = -1, y = -1 in (2)
-2 – 4(-1) + 2(-1) = -4 + 4 = 0
∴ The vectors \(\overline{\mathrm{AB}}, \overline{\mathrm{AC}}, \overline{\mathrm{AD}}\) are coplanar
⇒ A, B, C, D are coplanar
Hence the given points are coplanar.

(ii) \(6 \bar{a}+2 \bar{b}-\bar{c}, 2 \bar{a}-\bar{b}+3 \bar{c},-\bar{a}+2 \bar{b}-4 \bar{c},\)\(-12 \bar{a}-\bar{b}-3 \bar{c}\)
Solution:
Let O be the origin. Let A, B, C, D be the given points.

Let us suppose that one vector can be expressed as a linear combination of the other two.

∵ \(\bar{a}, \bar{b}, \bar{c}\) are non-coplanar vectors.
7x + 18y – 4 = 0 ………(1)
-3 + 3y = 0 ⇒ y = 1 …….(2)
3x + 2y + 4 = 0 ………(3)
Substitute y =1 in (3)
3x + 2 + 4 = 0 ⇒ x = -2
Substitute x = -2 and y = 1 in (1)
7(-2) + 18(1) – 4 = 0 ⇒ 0 = 0
Hence \(\overline{\mathrm{AB}}, \overline{\mathrm{AC}}\) and \(\overline{\mathrm{AD}}\) are coplanar.
⇒ The points A, B, C, D are coplanar.

Question 3.
If \(\overline{\mathbf{i}}, \overline{\mathbf{j}}, \overline{\mathbf{k}}\) are unit vectors along the positive directions of the coordinate axes, then show that the four points \(4 \overline{\mathbf{i}}+5 \overline{\mathbf{j}}+\overline{\mathbf{k}},-\overline{\mathbf{j}}-\overline{\mathbf{k}}, 3 \overline{\mathbf{i}}+9 \overline{\mathbf{j}}+4 \overline{\mathbf{k}}\) and \(-4 \bar{i}+4 \bar{j}+4 \bar{k}\) are coplanar.
Solution:
Let ‘O’ be the origin and let A, B, C, D be the given points.


⇒ The given points A, B, C, D are coplanar.
Second Method:
\(\left[\begin{array}{lll}
\overline{\mathrm{AB}} & \overline{\mathrm{AC}} & \overline{\mathrm{AD}}
\end{array}\right]\) = \(\left|\begin{array}{ccc}
-4 & -6 & -2 \\
-1 & 4 & 3 \\
-8 & -1 & 3
\end{array}\right|\)
= -4(12 + 3) + 6(-3 + 24) – 2(1 + 32)
= -60 + 126 – 66
= 0
Hence the vectors \(\overline{\mathrm{AB}}, \overline{\mathrm{AC}}\) and \(\overline{\mathrm{AD}}\) are coplanar.
⇒ The given points A, B, C, D are coplanar.

Question 4.
If a, b, c are non-coplanar vectors, then test for the collinearity of the following points whose position vectors are given by
(i) \(\bar{a}-2 \bar{b}+3 \bar{c}, 2 \bar{a}+3 \bar{b}-4 \bar{c},-7 \bar{b}+10 \bar{c}\)
Solution:
Let ‘O’ be the origin. A, B, C be the given points.
Then \(\overline{\mathrm{OA}}=\overline{\mathrm{a}}-2 \overline{\mathrm{b}}+3 \overline{\mathrm{c}}\)

(ii) \(3 \bar{a}-4 \bar{b}+3 \bar{c}\), \(-4 \bar{a}+5 \bar{b}-6 \bar{c}\), \(4 \overline{\mathbf{a}}-7 \overline{\mathbf{b}}+6 \overline{\mathbf{c}}\)
Solution:

(iii) \(\begin{aligned}
&2 \bar{a}+5 \bar{b}-4 \bar{c}, \bar{a}+4 \bar{b}-3 \bar{c}, \\
&4 \bar{a}+7 \bar{b}-6 \bar{c}
\end{aligned}\)
Solution:
Let ‘O’ be the origin and A, B, C be the given points.
Then \(\overline{\mathrm{OA}}=2 \overline{\mathrm{a}}+5 \overline{\mathrm{b}}-4 \overline{\mathrm{c}}\), \(\overline{\mathrm{OB}}=\overline{\mathrm{a}}+4 \overline{\mathrm{b}}-3 \overline{\mathrm{c}}\)

∴ The points A, B, C are collinear.

III.

Question 1.
In the Cartesian plane, O is the origin of the coordinate axes. A person starts at O and walks a distance of 3 units in the NORTH-EAST direction and reaches point P. From P he walks 4 units of distance parallel to NORTH-WEST direction and reaches the point Q. Express the vector \(\overline{\mathbf{O Q}}\) in terms of \(\overline{\mathbf{i}}\) and \(\overline{\mathbf{j}}\) (observe that ∠XOP = 45°)
Solution:
‘O’ the origin of co-ordinate axes.

Question 2.
The points O, A, B, X and Y are such that \(\overline{\mathbf{O A}}=\overline{\mathbf{a}}, \overline{\mathbf{O B}}=\overline{\mathbf{b}}, \overline{\mathbf{O X}}=\mathbf{3} \overline{\mathbf{a}}\) and \(\overline{\mathbf{O Y}}=\mathbf{3} \overline{\mathbf{b}}\). Find \(\overline{\mathbf{B X}}\) and \(\overline{\mathbf{A Y}}\) interms of \(\bar{a}\) and \(\bar{b}\). Futher, if the point P divides AY in the ratio 1 : 3, then express \(\overline{\mathrm{BP}}\) interms of \(\bar{a}\) and \(\bar{a}\).
Solution:

Question 3.
If ∆OAB, E is the midpoint of AB and F is a point on OA such that OF = 2(FA). If C is the point of intersection of \(\overline{\mathrm{OE}}\) and \(\overline{\mathrm{BF}}\), then find the ratios OC : CE and BC : CF.
Solution:

Question 4.
Point E divides the segment PQ internally in the ratio 1 : 2 and R is any point not on the line PQ. If F is a point on QR such that QF : FR = 2 : 1, then show that EF is parallel to PR.
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Matrices Solutions Exercise 3(i) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Matrices Solutions Exercise 3(i)

Solve the following systems of homogeneous equations.

Question 1.
2x + 3y – z = 0, x – y – 2z = 0, 3x + y + 3z = 0
Solution:
The Coefficient matrix is \(\left[\begin{array}{ccc}
2 & 3 & -1 \\
1 & -1 & -2 \\
3 & 1 & 3
\end{array}\right]\)
det of \(\left[\begin{array}{ccc}
2 & 3 & -1 \\
1 & -1 & -2 \\
3 & 1 & 3
\end{array}\right]=\left[\begin{array}{ccc}
2 & 3 & -1 \\
1 & -1 & -2 \\
3 & 1 & 3
\end{array}\right]\)
= 2(-3 + 2) – 3(3 + 6) – 1(1 + 3)
= -2 – 27 – 4
= -33 ≠ 0, ρ(A) = 3
Hence the system has the trivial solution x = y = z = 0 only.

Question 2.
3x + y – 2z = 0, x + y + z = 0, x – 2y + z = 0
Hint: If the determinant of the coefficient matrix ≠ 0 then the system has a trivial solution (i.e.) ρ(A) = 3.
Solution:
The coefficient matrix is \(\left[\begin{array}{ccc}
3 & 1 & -2 \\
1 & 1 & 1 \\
1 & -2 & 1
\end{array}\right]\)
\(\left|\begin{array}{ccc}
3 & 1 & -2 \\
1 & 1 & 1 \\
1 & -2 & 1
\end{array}\right|\)
= 3(1 + 2) – 1(1 – 1) – 2(-2 – 1)
= 9 + 6
= 15 ≠ 0, ρ(A) = 3
Hence the system has the trivial solutions x = y = z = 0 only.

Question 3.
x + y – 2z = 0, 2x + y – 3z = 0, 5x + 4y – 9z = 0
Solution:
The coefficient matrix is \(\left[\begin{array}{rrr}
1 & 1 & -2 \\
2 & 1 & -3 \\
5 & 4 & -9
\end{array}\right]\) = A (say)
|A| = \(\left|\begin{array}{rrr}
1 & 1 & -2 \\
2 & 1 & -3 \\
5 & 4 & -9
\end{array}\right|\)
= 1(-9 + 12) – 1(-18 + 15) – 2(8 – 5)
= 3 + 3 – 6
= 0
∴ Rank of A = 2 as the submatrix \(\left[\begin{array}{ll}
1 & 1 \\
2 & 1
\end{array}\right]\) is non-singular, ρ(A) < 3
Hence the system has a non-trivial solution.
A = \(\left[\begin{array}{lll}
1 & 1 & -2 \\
2 & 1 & -3 \\
5 & 4 & -9
\end{array}\right]\)
R₂ → R₂ – 2R₁, R₃ → R₃ – 3R₁
A ~ \(\left[\begin{array}{ccc}
1 & 1 & -2 \\
0 & -1 & 1 \\
0 & -1 & -1
\end{array}\right]\)
The system of equation is equivalent to the given system of equations are x + y – 2z = 0, -y + z = 0
Let z = k
⇒ y = k, x = k
∴ x = y = z = k for real number k.

Question 4.
x + y – z = 0, x – 2y + z = 0, 3x + 6y – 5z = 0
Solution:
Coefficient matrix A = \(\left[\begin{array}{ccc}
1 & 1 & -1 \\
1 & -2 & 1 \\
3 & 6 & -5
\end{array}\right]\)
R₂ → R₂ – R₁, R₃ → R₃ – 3R₁
A ~ \(\left[\begin{array}{ccc}
1 & 1 & -1 \\
0 & -3 & 2 \\
0 & 3 & -2
\end{array}\right]\)
⇒ det A = 0 as R₂, R₃ are identical.
and rank (A) = 2 as the submatrix \(\left[\begin{array}{cc}
1 & 1 \\
0 & -3
\end{array}\right]\) is non-singular.
Hence the system has a non-trivial solution, ∵ ρ(A) < 3
The system of equations equivalent to the given system of equations are
x + y – z = 0
3y – 2z = 0
Let z = k
⇒ y = \(\frac{2 k}{3}\)
x = \(\frac{k}{3}\)
∴ x = \(\frac{k}{3}\), y = \(\frac{2 k}{3}\), z = k for any real number of k.

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Matrices Solutions Exercise 3(h) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Matrices Solutions Exercise 3(h)

Solve the following systems of equations.
(i) by using Cramer’s rule and matrix inversion method, when the coefficient matrix is non-singular.
(ii) by using the Gauss-Jordan method. Also, determine whether the system has a unique solution or an infinite number of solutions, or no solution, and find the solutions if exist.

Question 1.
5x – 6y + 4z = 15
7x + 4y – 3z = 19
2x + y + 6z = 46
Solution:
(i) Cramer’s rule:
Δ = \(\left|\begin{array}{ccc}
5 & -6 & 4 \\
7 & 4 & -3 \\
2 & 1 & 6
\end{array}\right|\)
= 5(24 + 3) + 6(42 + 6) + 4(7 – 8)
= 135 + 288 – 4
= 419
Δ₁ = \(\left|\begin{array}{ccc}
15 & -6 & 4 \\
19 & 4 & -3 \\
46 & 1 & 6
\end{array}\right|\)
= 15(24 + 3) + 6(114 + 138) + 4(19 – 184)
= 405 + 1512 – 660
= 1917 – 660
= 1257
Δ₂ = \(\left|\begin{array}{ccc}
5 & 15 & 4 \\
7 & 19 & -3 \\
2 & 46 & 6
\end{array}\right|\)
= 5(114 + 138) – 15(42 + 6) + 4(322 – 38)
= 1260 – 720 + 1136
= 1676
Δ₃ = \(\left|\begin{array}{ccc}
5 & -6 & 15 \\
7 & 4 & 19 \\
2 & 1 & 46
\end{array}\right|\)
= 5(184 – 19) + 6(322 – 38) + 15(7 – 8)
= 825 + 1704 – 15
= 2529 – 15
= 2514

Solution is x = 3, y = 4, z = 6.

(ii) Matrix inversion method:



Solution is x = 3, y = 4, z = 6

(iii) Gauss-Jordan method:

∴ Unique solution exists.
Solution is x = 3, y = 4, z = 6.

Question 2.
x + y + z = 1
2x + 2y + 3z = 6
x + 4y + 9z = 3
Solution:
(i) Cramer’s rule:
Δ = \(\left|\begin{array}{lll}
1 & 1 & 1 \\
2 & 2 & 3 \\
1 & 4 & 9
\end{array}\right|\)
= 1(18 – 12) – 1(18 – 3) + 1(8 – 2)
= 6 – 15 + 6
= -3
Δ₁ = \(\left|\begin{array}{lll}
1 & 1 & 1 \\
6 & 2 & 3 \\
3 & 4 & 9
\end{array}\right|\)
= 1(18 – 12) – 1(54 – 9) + 1(24 – 6)
= 6 – 45 + 18
= -21
Δ₂ = \(\left|\begin{array}{lll}
1 & 1 & 1 \\
2 & 6 & 3 \\
1 & 3 & 9
\end{array}\right|\)
= 1(54 – 9) – 1(18 – 3) + 1(6 – 6)
= 45 – 15
= 30
Δ₃ = \(\left|\begin{array}{lll}
1 & 1 & 1 \\
2 & 2 & 6 \\
1 & 4 & 3
\end{array}\right|\)
= 1(6 – 24) – 1(6 – 6) + 1(8 – 2)
= -18 – 0 + 6
= -12

Solution is x = 7, y = -10, z = 4

(ii) Matrix inversion method:



∴ Solution is x = 7, y = -10, z = 4

(iii) Gauss-Jordan method:
Augmented matrix is A = \(\left[\begin{array}{llll}
1 & 1 & 1 & 1 \\
2 & 2 & 3 & 6 \\
1 & 4 & 9 & 3
\end{array}\right]\)
R₂ → R₂ – 2R₁, R₃ → R₃ – R₁

Unique solution exists.
∴ Solution is x = 7, y = -10, z = 4

Question 3.
x – y + 3z = 5
4x + 2y – z = 0
-x + 3y + z = 5
Solution:
(i) Cramer’s rule:
Δ = \(\left|\begin{array}{ccc}
1 & -1 & 3 \\
4 & 2 & -1 \\
-1 & 3 & 1
\end{array}\right|\)
= 1(2 + 3) + 1(4 – 1) + 3(12 + 2)
= 5 + 3 + 42
= 50
Δ₁ = \(\left|\begin{array}{ccc}
5 & -1 & 3 \\
0 & 2 & -1 \\
5 & 3 & 1
\end{array}\right|\)
= 5(2 + 3) + 1(0 + 5) + 3(0 – 10)
= 25 + 5 – 30
= 0
Δ₂ = \(\left|\begin{array}{ccc}
1 & 5 & 3 \\
4 & 0 & -1 \\
-1 & 5 & 1
\end{array}\right|\)
= 1(0 + 5) – 5(4 – 1) + 3(20 – 0)
= 5 – 15 + 60
= 50
Δ₃ = \(\left|\begin{array}{ccc}
1 & -1 & 5 \\
4 & 2 & 0 \\
-1 & 3 & 5
\end{array}\right|\)
= 1(10 – 0) + 1(20 – 0) + 5(12 + 2)
= 10 + 20 + 70
= 100

∴ Solution is x = 0, y = 1, z = 2.

(ii) Matrix inversion method:



Solution is x = 0, y = 1, z = 2

(iii) Gauss Jordan method:

Unique solution exists.
∴ Solution is x = 0, y = 1, z = 2

Question 4.
2x + 6y + 11 = 0
6x + 20y – 6z + 3 = 0
6y – 18z + 1 = 0
Solution:
(i) Cramer’s rule:
Δ = \(\left|\begin{array}{ccc}
2 & 6 & 0 \\
6 & 20 & -6 \\
0 & 6 & -18
\end{array}\right|\)
= 2(-360 + 36) – 6(-108 – 0)
= -648 + 648
= 0
∴ Cramer’s rule and matrix inversion method cannot be used.
∵ Δ = 0

(ii) Gauss Jordan method:

ρ(A) = 2, ρ(AB) = 3
ρ(A) ≠ ρ(AB)
∴ The given system of equations does not have a solution.

Question 5.
2x – y + 3z = 9
x + y + z = 6
x – y + z = 2
Solution:
(i) Cramer’s rule:
Δ = \(\left|\begin{array}{ccc}
2 & -1 & 3 \\
1 & 1 & 1 \\
1 & -1 & 1
\end{array}\right|\)
= 2(1 + 1) + 1(1 – 1) + 3(-1 – 1)
= 4 + 0 – 6
= -2
Δ₁ = \(\left|\begin{array}{ccc}
9 & -1 & 3 \\
6 & 1 & 1 \\
2 & -1 & 1
\end{array}\right|\)
= 9(1 + 1) + 1(6 – 2) + 3(-6 – 2)
= 18 + 4 – 24
= -2
Δ₂ = \(\left|\begin{array}{lll}
2 & 9 & 3 \\
1 & 6 & 1 \\
1 & 2 & 1
\end{array}\right|\)
= 2(6 – 2) – 9(1 – 1) + 3(2 – 6)
= 8 – 0 – 12
= -4
Δ₃ = \(\left|\begin{array}{ccc}
2 & -1 & 9 \\
1 & 1 & 6 \\
1 & -1 & 2
\end{array}\right|\)
= 2(2 + 6) + 1(2 – 6) + 9(-1 – 1)
= 16 – 4 – 18
= -6

Solution is x = 1, y = 2, z = 3.

(ii) Matrix inversion method:


Solution is x = 1, y = 2, z = 3.

(iii) Gauss-Jordan method:

∴ The given equations have a unique solution.
Solution is x = 1, y = 2, z = 3

Question 6.
2x – y + 8z = 13
3x + 4y + 5z = 18
5x – 2y + 7z = 20
Solution:
(i) Cramer’s rule:
Δ = \(\left|\begin{array}{ccc}
2 & -1 & 8 \\
3 & 4 & 5 \\
5 & -2 & 7
\end{array}\right|\)
= 2(28 + 10) + 1(21 – 25) + 8(-6 – 20)
= 76 – 4 – 208
= -136
Δ₁ = \(\left|\begin{array}{ccc}
13 & -1 & 8 \\
18 & 4 & 5 \\
20 & -2 & 7
\end{array}\right|\)
= 13(28 + 10) + 1(126 – 100) + 8(-36 – 80)
= 494 + 26 – 928
= -408
Δ₂ = \(\left|\begin{array}{lll}
2 & 13 & 8 \\
3 & 18 & 5 \\
5 & 20 & 7
\end{array}\right|\)
= 2(126 – 100) – 13(21 – 25) + 8(60 – 90)
= 52 + 52 – 240
= -136
Δ₃ = \(\left|\begin{array}{ccc}
2 & -1 & 13 \\
3 & 4 & 18 \\
5 & -2 & 20
\end{array}\right|\)
= 2(80 + 36) + 1(60 – 90) + 13(-6 – 20)
= 232 – 30 – 338
= -136

∴ Solution is x = 3, y = 1, z = 1

(ii) Matrix inversion method:



∴ Solution is x = 3, y = 1, z = 1

(iii) Gauss Jordan method:

∴ The given equations have a unique solution and Solution is x = 3, y = 1, z = 1.

Question 7.
2x – y + 3z = 8
-x + 2y + z = 4
3x + y – 4z = 0
Solution:
(i) Cramer’s rule:
Δ = \(\left|\begin{array}{ccc}
2 & -1 & 3 \\
-1 & 2 & 1 \\
3 & 1 & -4
\end{array}\right|\)
= 2(-8 – 1) + 1(4 – 3) + 3(-1 – 6)
= -18 + 1 – 21
= -38
Δ₁ = \(\left|\begin{array}{ccc}
8 & -1 & 3 \\
4 & 2 & 1 \\
0 & 1 & -4
\end{array}\right|\)
= 8(-8 – 1) + 1(-16 – 0) + 3(4 – 0)
= -72 – 16 + 12
= -76
Δ₂ = \(\left|\begin{array}{ccc}
2 & 8 & 3 \\
-1 & 4 & 1 \\
3 & 0 & -4
\end{array}\right|\)
= 2(-16 – 0) – 8(4 – 3) + 3(-0 – 12)
= -32 – 8 – 36
= -76
Δ₃ = \(\left|\begin{array}{ccc}
2 & -1 & 8 \\
-1 & 2 & 4 \\
3 & 1 & 0
\end{array}\right|\)
= 2(0 – 4) + 1(0 – 12) + 8(-1 – 6)
= -8 – 12 – 56
= -76

∴ Solution is x = 2, y = 2, z = 2.

(ii) Matrix inversion method:


Solution is x = 2, y = 2, z = 2

(iii) Gauss Jordan method:


∴ The given equations have a unique solution and solution is x = 2, y = 2, z = 2.

Question 8.
x + y + z = 9
2x + 5y + 7z = 52
2x + y – z = 0
Solution:
(i) Cramer’s rule:
Δ = \(\left|\begin{array}{ccc}
1 & 1 & 1 \\
2 & 5 & 7 \\
2 & 1 & -1
\end{array}\right|\)
= 1(-5 – 7) – 1(-2 – 14) + 1(2 – 10)
= -12 + 16 – 8
= -4
Δ₁ = \(\left|\begin{array}{ccc}
9 & 1 & 1 \\
52 & 5 & 7 \\
0 & 1 & -1
\end{array}\right|\)
= 9(-5 – 7) – 1(-52 – 0) + 1(52 – 0)
= -108 + 52 + 52
= -4
Δ₂ = \(\left|\begin{array}{ccc}
1 & 9 & 1 \\
2 & 52 & 7 \\
2 & 0 & -1
\end{array}\right|\)
= 1(-52 – 0) – 9(-2 – 14) + 1(0 – 104)
= -52 + 144 – 104
= -12
Δ₃ = \(\left|\begin{array}{ccc}
1 & 1 & 9 \\
2 & 5 & 52 \\
2 & 1 & 0
\end{array}\right|\)
= 1(0 – 52) – 1(0 – 104) + 9(2 – 10)
= -52 + 104 – 72
= -20

(ii) Matrix inversion method:


Solution is x = 1, y = 3, z = 5

(iii) Gauss Jordan method:

∴ The given equations have a unique solution and solution is x = 1, y = 3, z = 5.