Mahesh

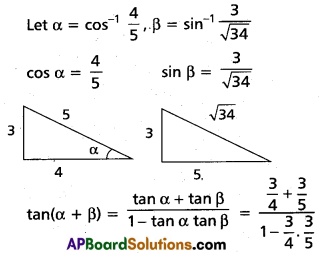
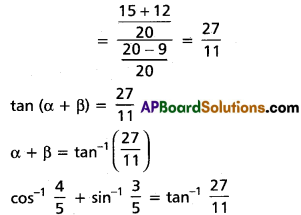
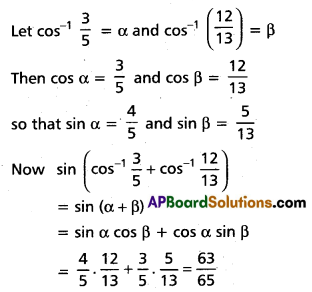
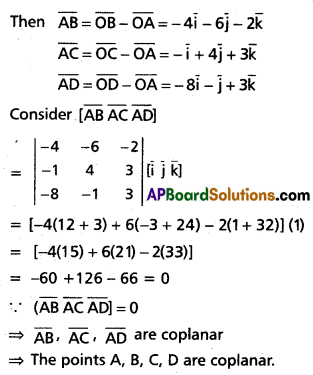
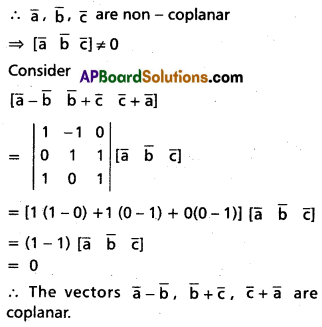
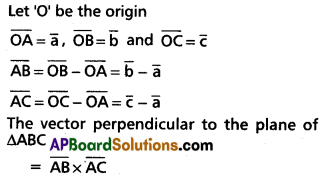
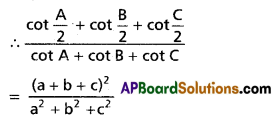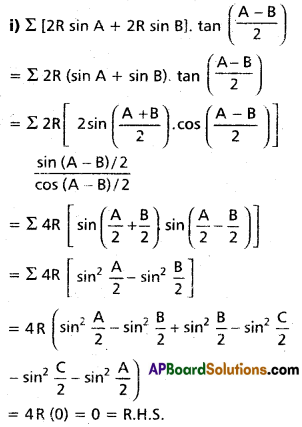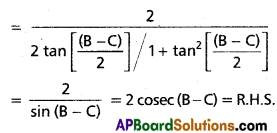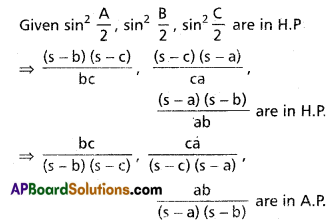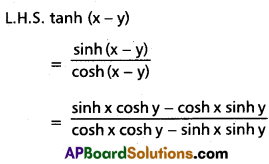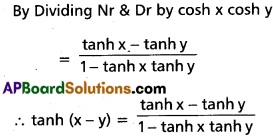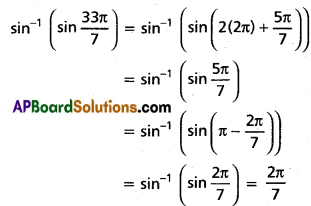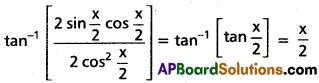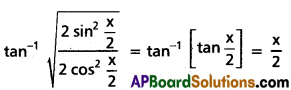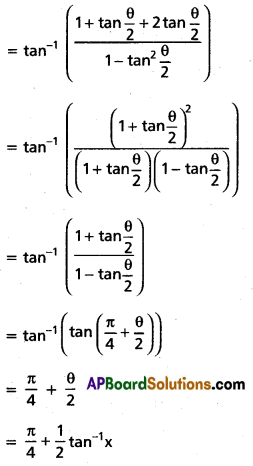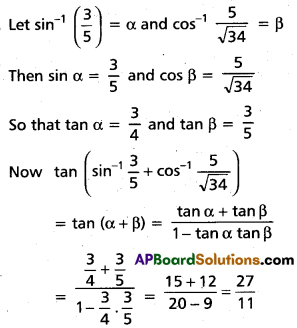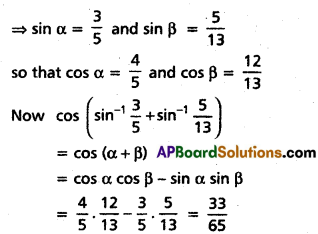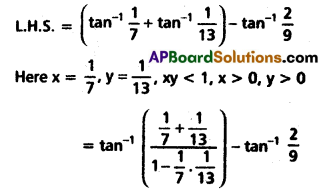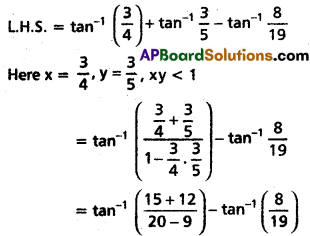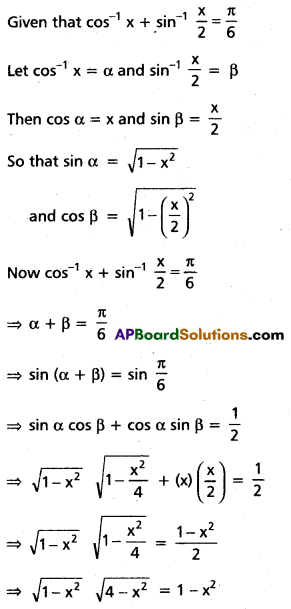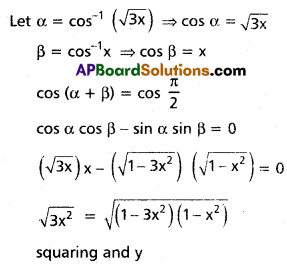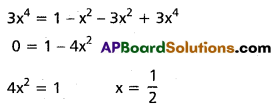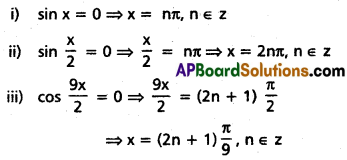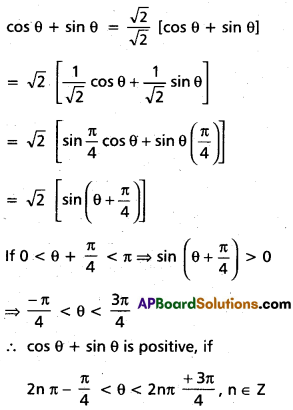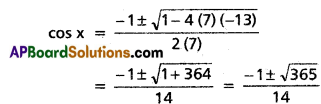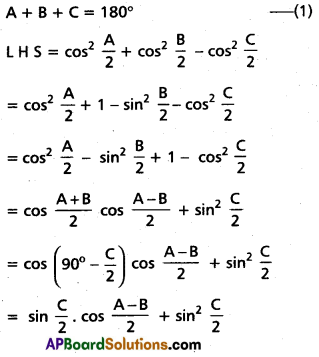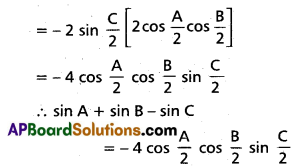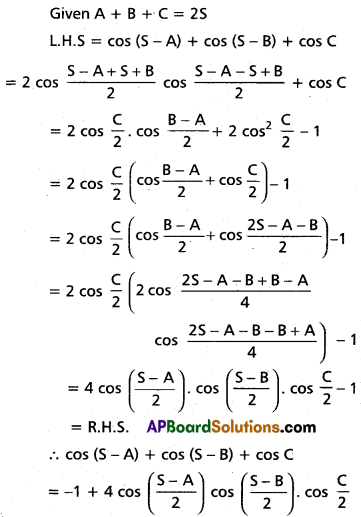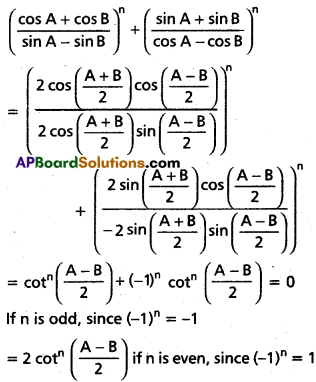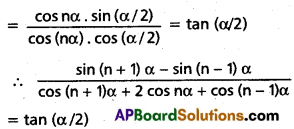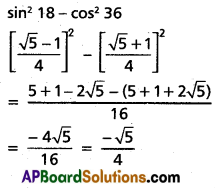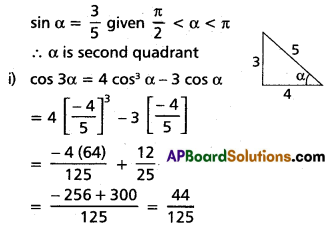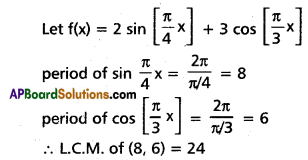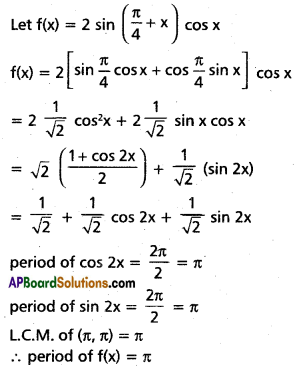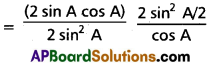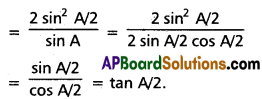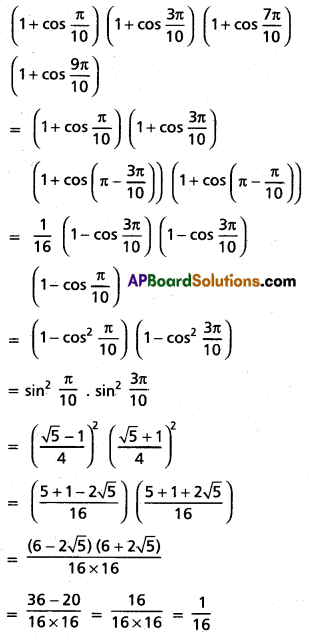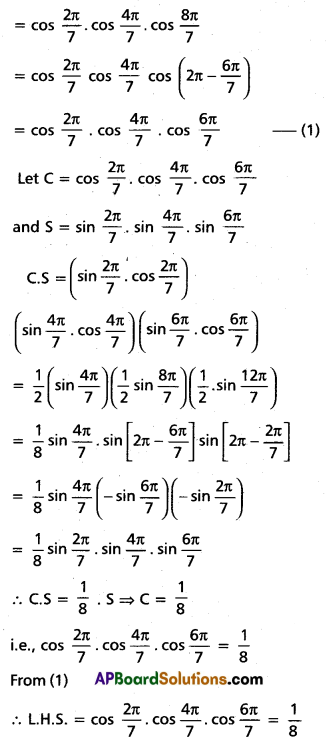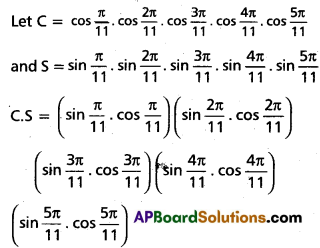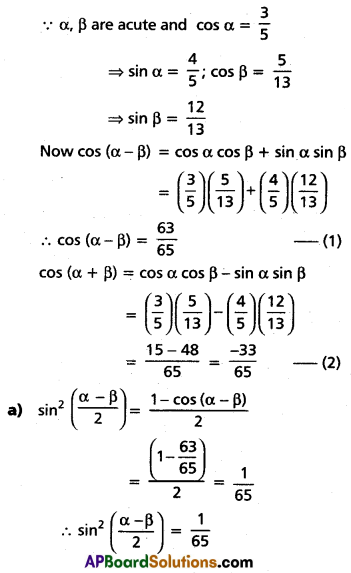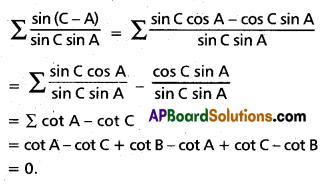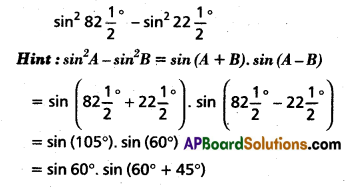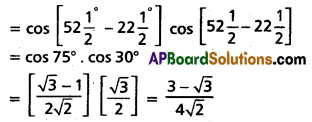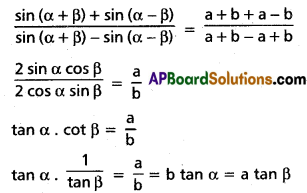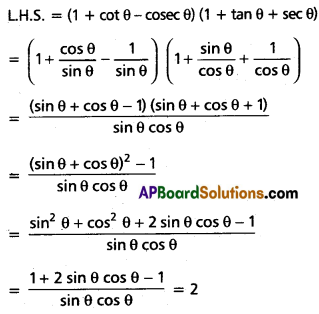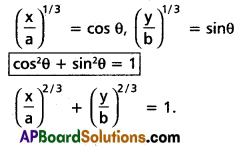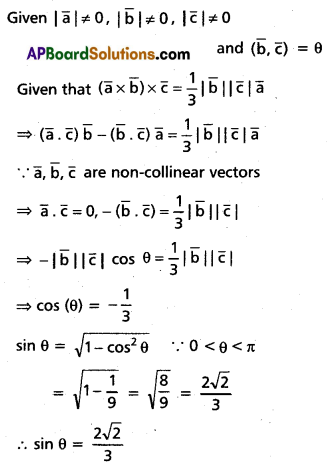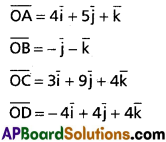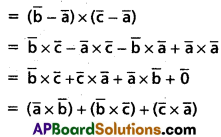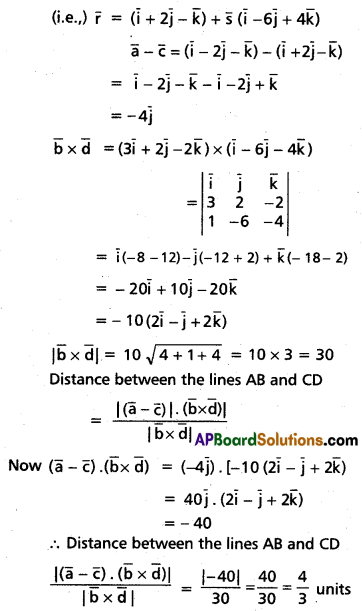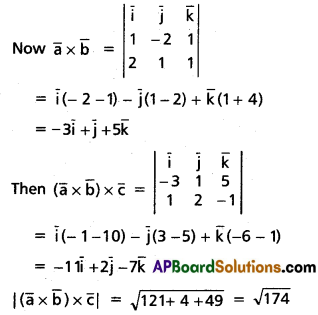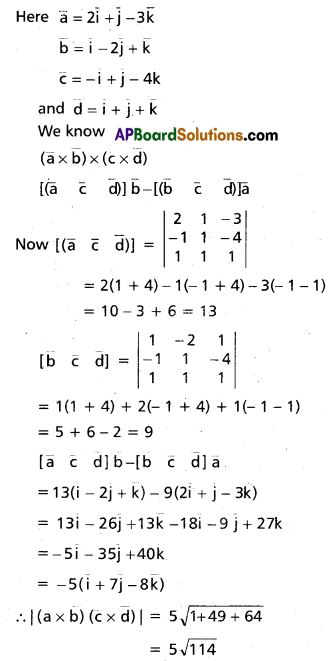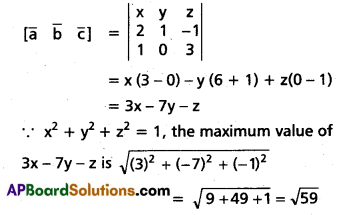Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Properties of Triangles Solutions Exercise 10(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Properties of Triangles Solutions Exercise 10(b)

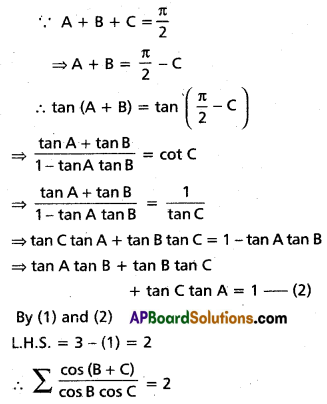
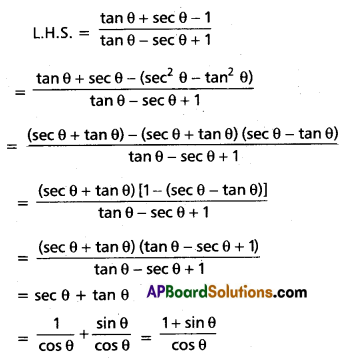
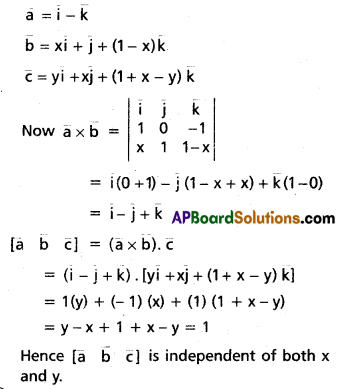
All problems in this exercise have reference to ΔABC.

I.

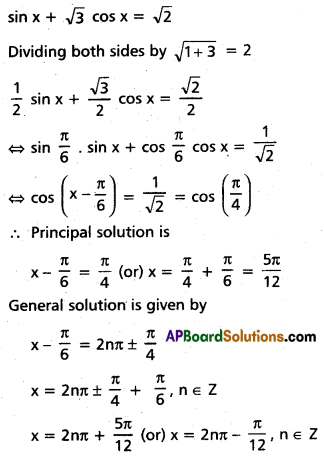
Question 1.
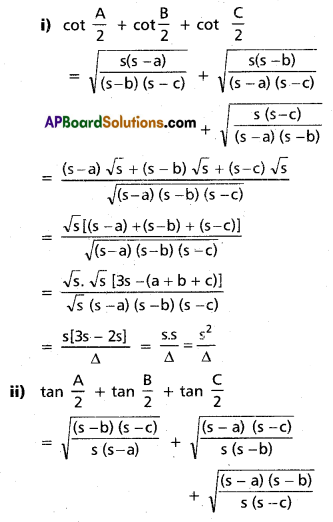
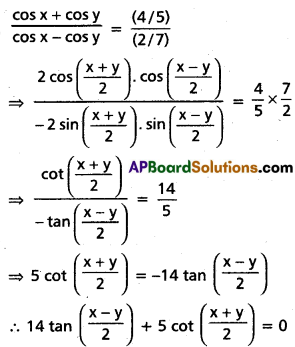
Express \(\Sigma r_{1} \cot \frac{A}{2}\) in terms of s.
Solution:
\(\Sigma r_{1} \cot \frac{A}{2}\) = \(\Sigma\left(s \tan \frac{A}{2}\right) \cot \frac{A}{2}\)
= Σs
= s + s + s
= 3s

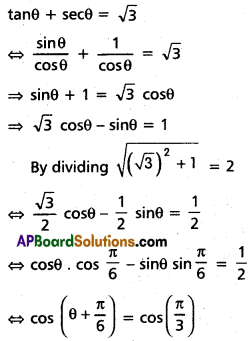
Question 2.
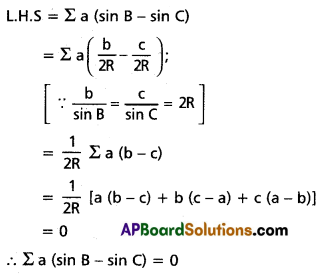
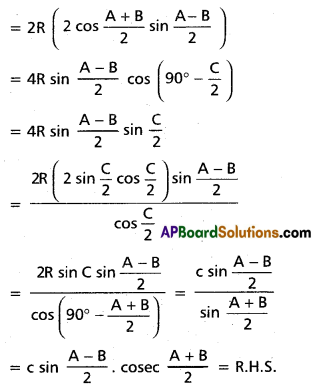
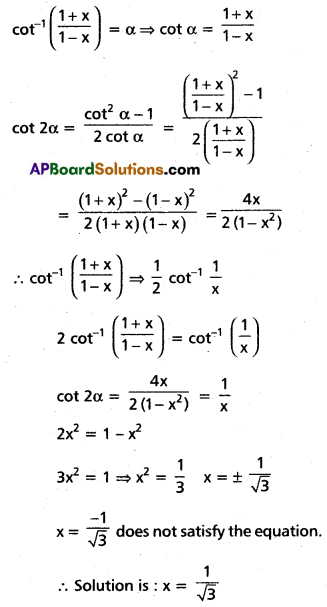
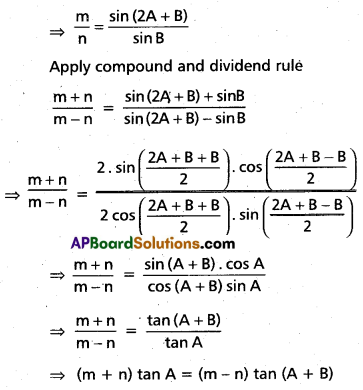
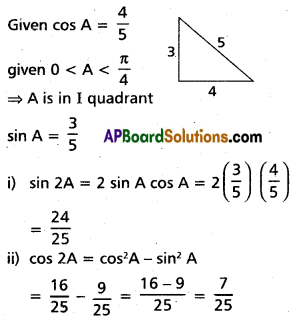
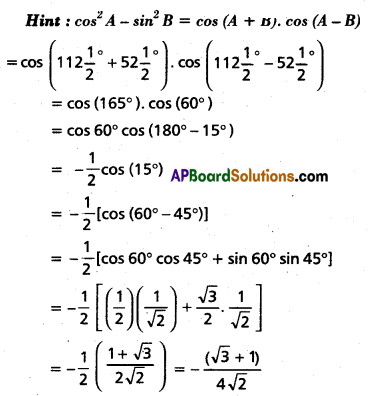
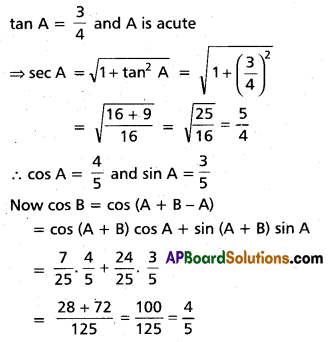
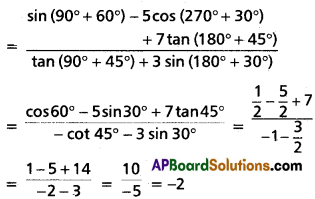
Show that Σa cot A = 2(R + r).
Solution:
L.H.S = Σa . cot A
= Σ2R sin A \(\frac{\cos A}{\sin A}\)
= 2R Σ cos A
= \(2 R\left(1+4 \sin \frac{A}{2} \sin \frac{B}{2} \sin \frac{C}{2}\right)\) (From transformants)
= \(2\left(R+4 R \sin \frac{A}{2} \cdot \sin \frac{B}{2} \cdot \sin \frac{C}{2}\right)\)
= 2(R + r)
= R.H.S

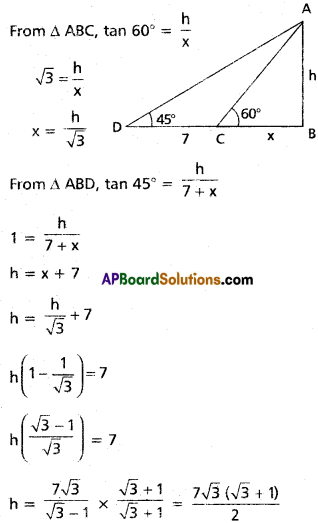
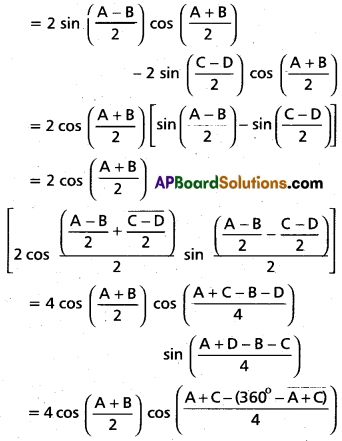
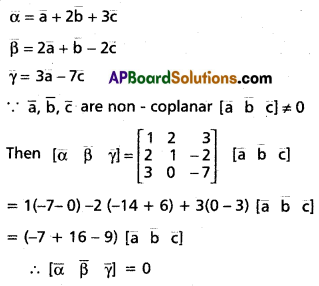
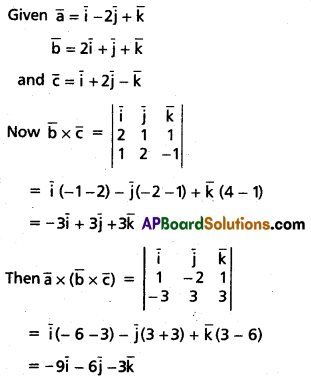
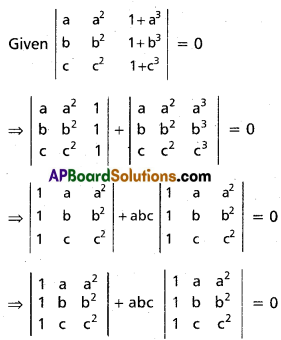
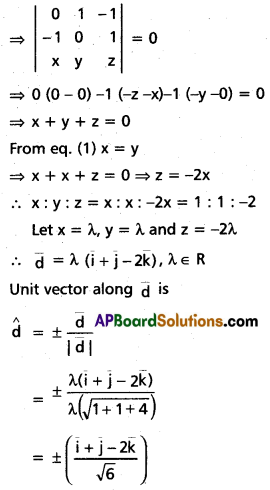
Question 3.
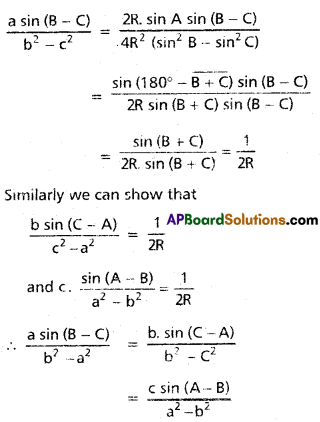
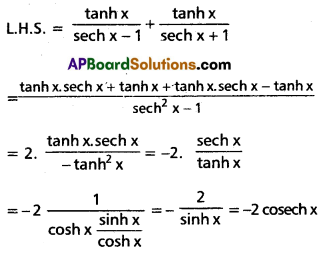
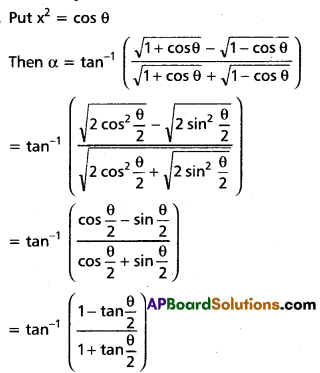
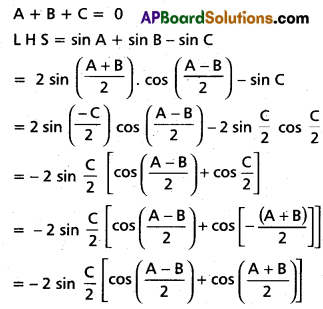
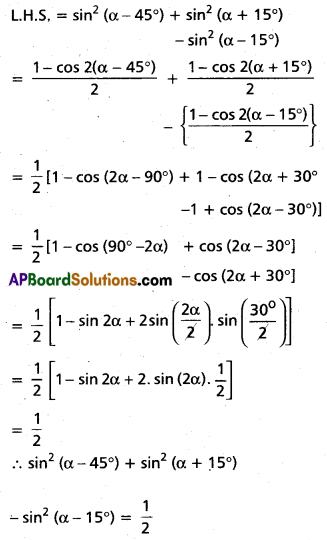
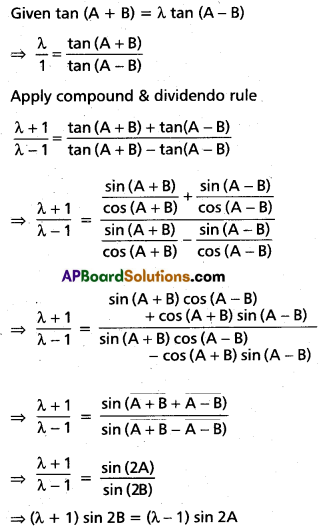
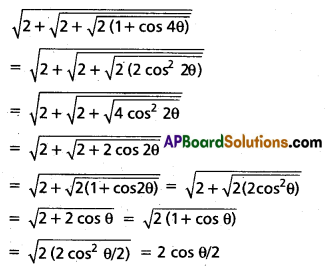
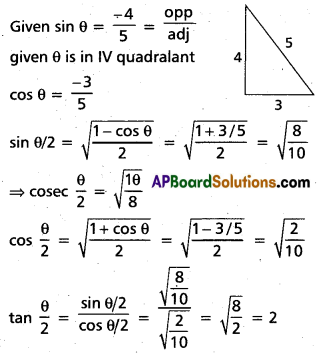
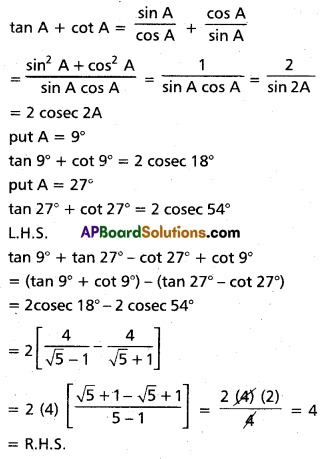
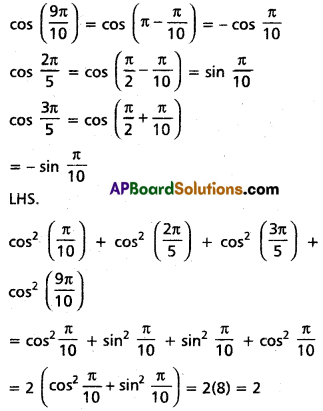
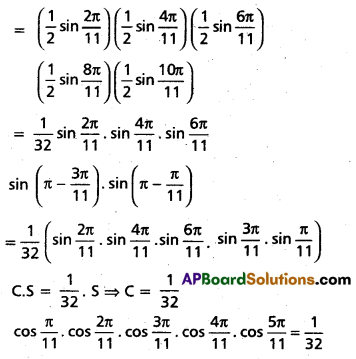
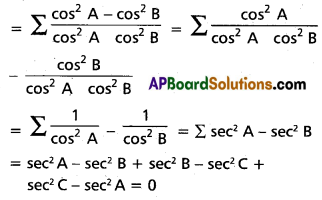
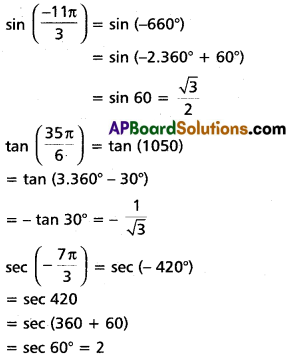
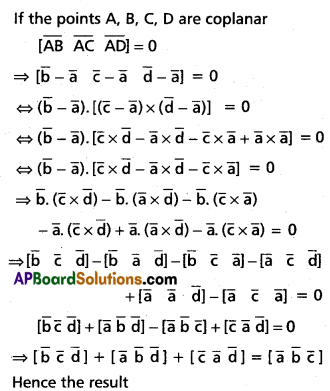
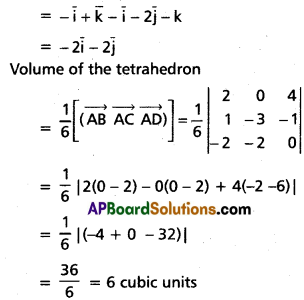
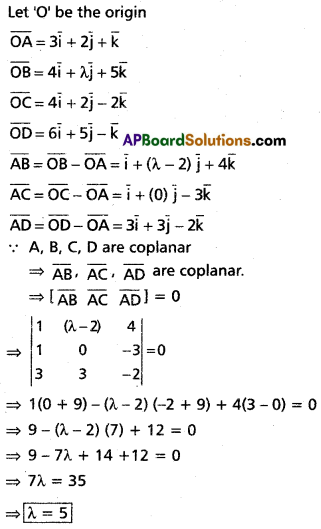
In ∆ABC, prove that r₁ + r₂ + r₃ – r = 4R.
Solution:

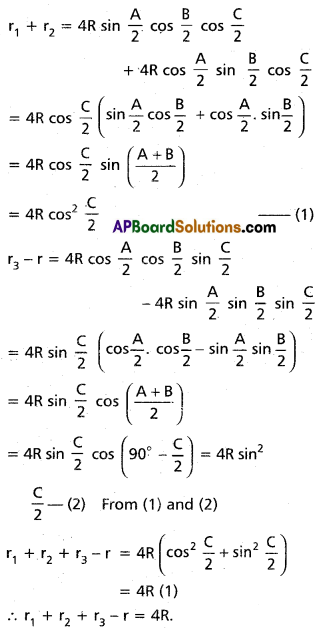
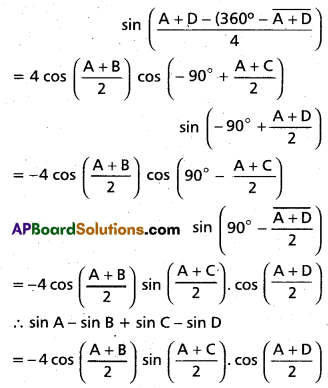
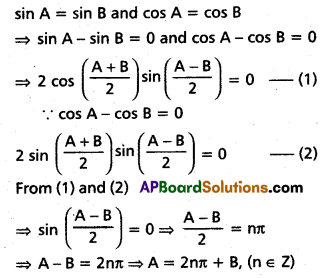
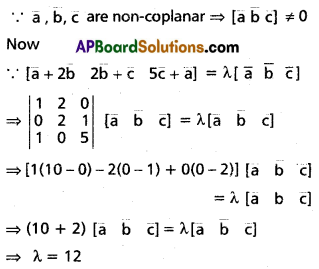
Question 4.
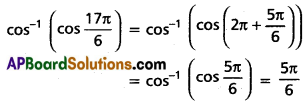
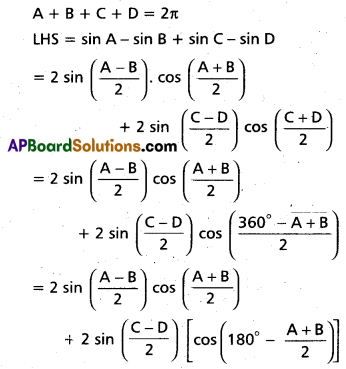
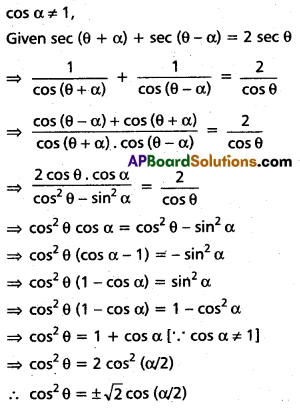
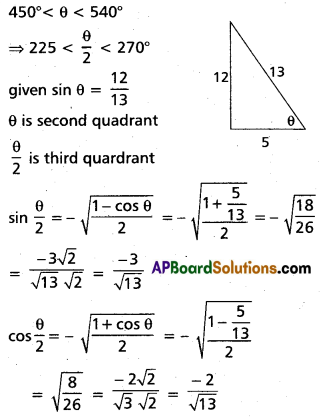
In ∆ABC, prove that r + r₁ + r₂ – r₃ = 4R cos C.
Solution:

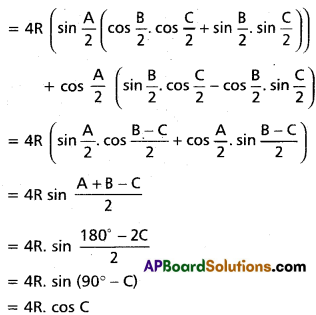
Question 5.
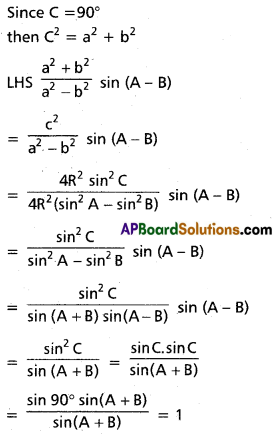
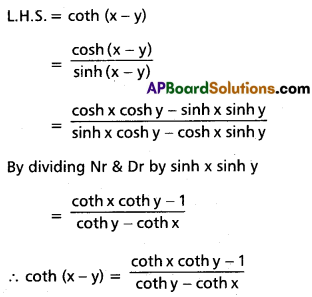
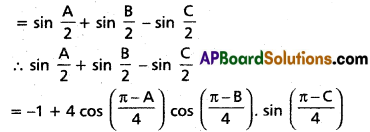
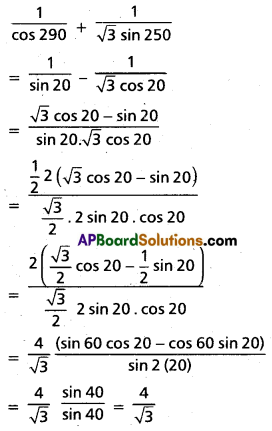
If r + r₁ + r₂ + r₃ then show that C = 90°.
Solution:

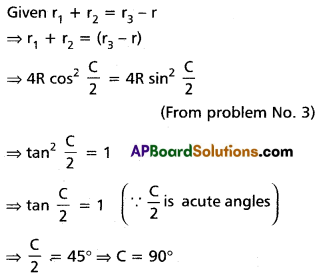
II.

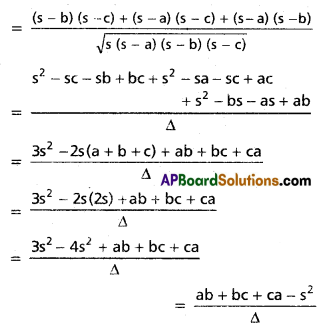
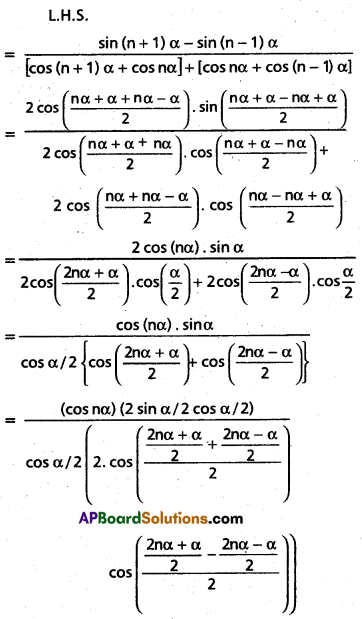
Question 1.
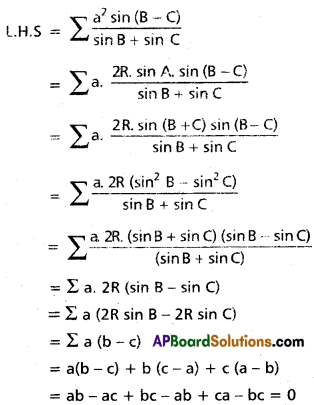
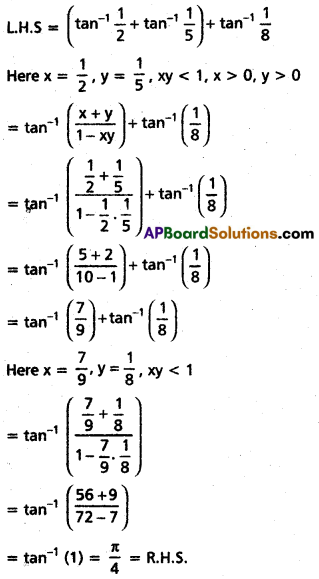
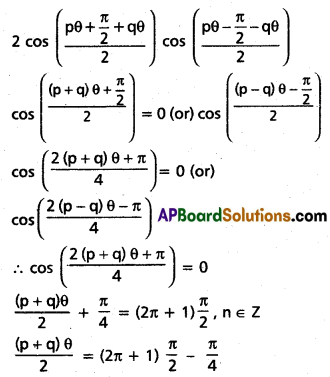
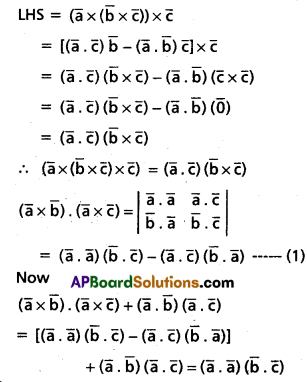
Prove that 4(r₁r₂ + r₂r₃ + r₃r₁) = (a + b + c)²
Solution:

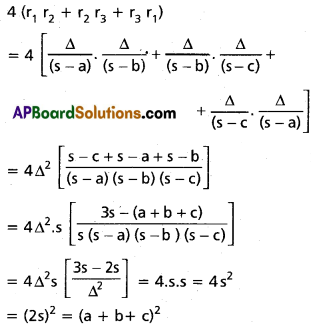
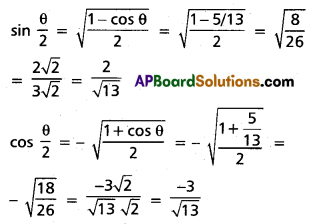
Question 2.
Prove that \(\left(\frac{1}{r}-\frac{1}{r_{1}}\right)\left(\frac{1}{r}-\frac{1}{r_{2}}\right)\left(\frac{1}{r}-\frac{1}{r_{3}}\right)=\frac{a b c}{\Delta^{3}}=\frac{4 R}{r^{2} s^{2}}\)
Solution:

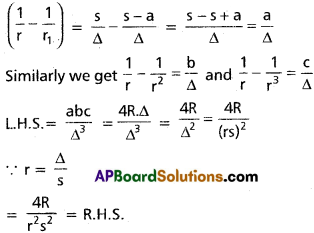
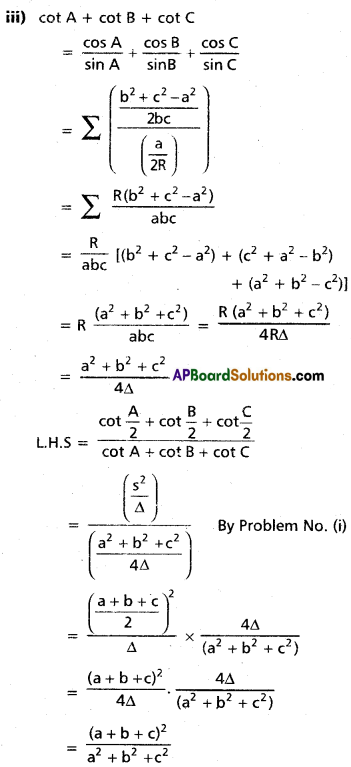
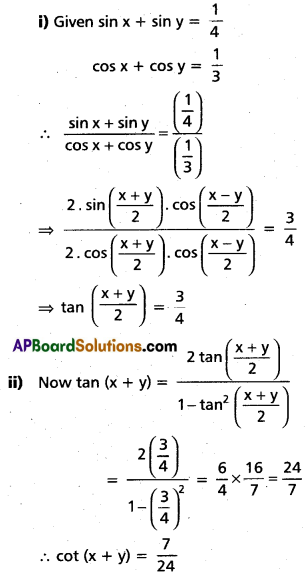
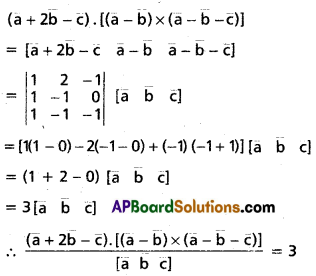
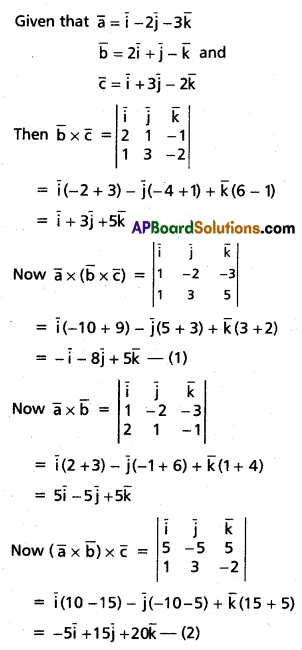
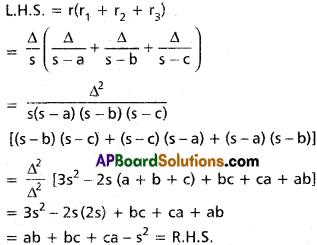
Question 3.
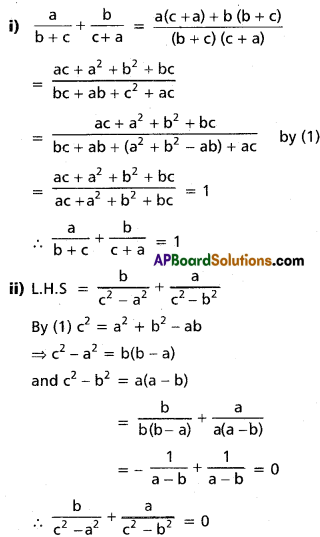
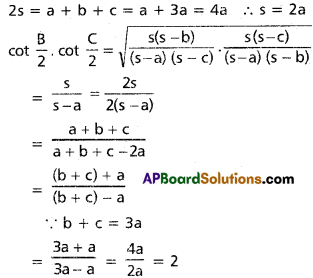
Prove that r(r₁ + r₂ + r₃) = ab + bc + ca – s².
Solution:

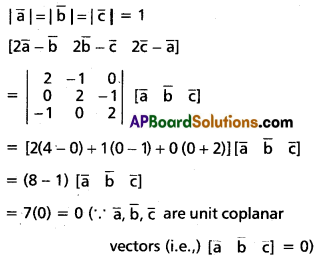
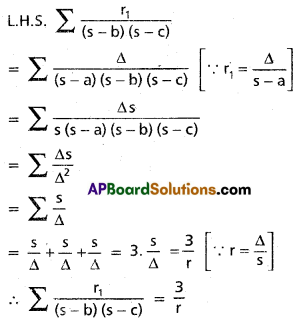
Question 4.
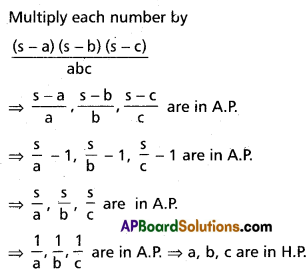
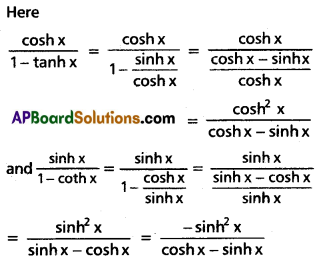
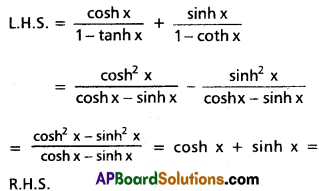
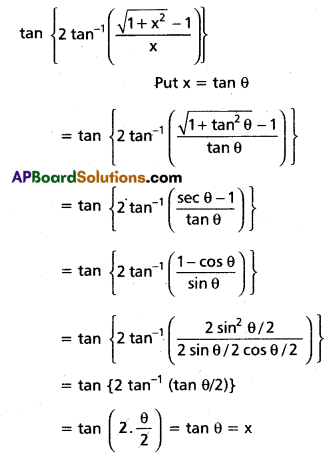
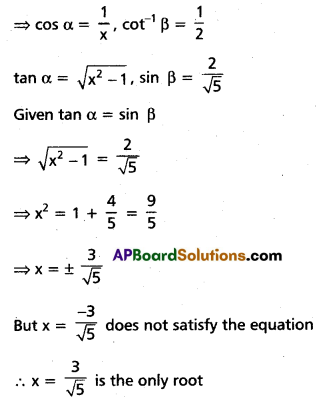
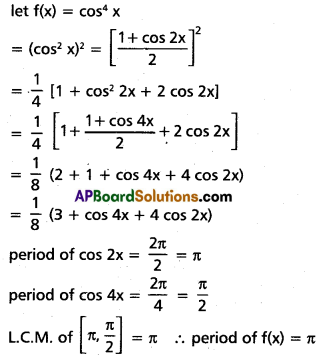
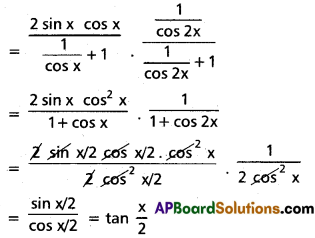
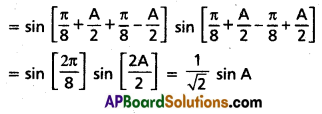
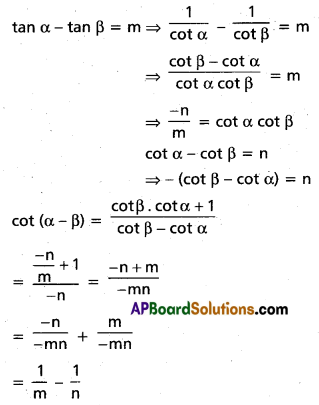
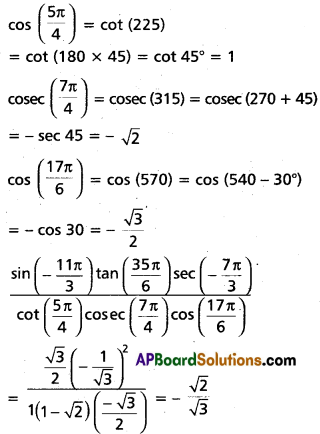
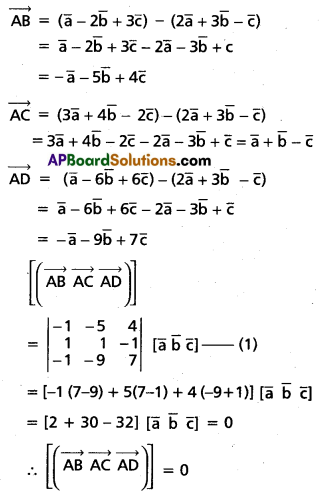
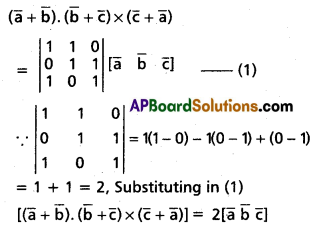
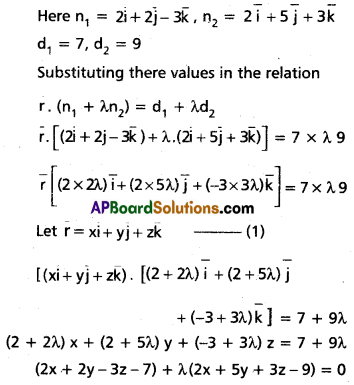
Show that \(\sum \frac{r_{1}}{(s-b)(s-c)}=\frac{3}{r}\)
Solution:

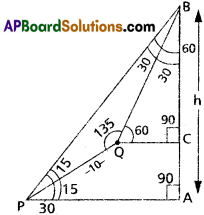
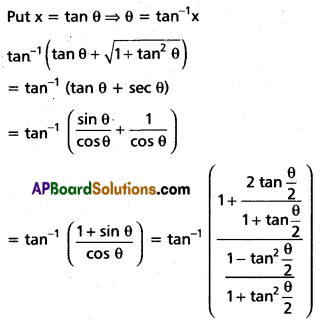
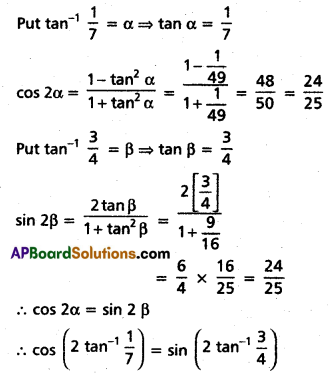
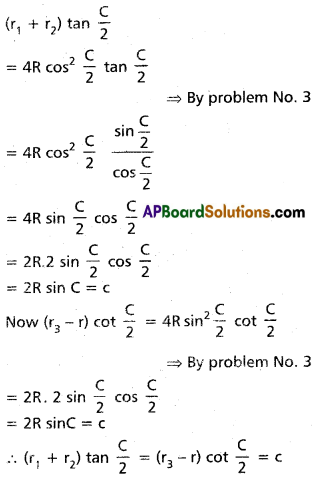
Question 5.
Show that \(\left(r_{1}+r_{2}\right) \tan \frac{C}{2}=\left(r_{3}-r\right) \cot \frac{C}{2}=c\)
Solution:

Question 6.
Show that r₁r₂r₃ = \(r^{3} \cot ^{2} \frac{A}{2} \cdot \cot ^{2} \frac{B}{2} \cdot \cot ^{2} \frac{C}{2}\)
Solution:

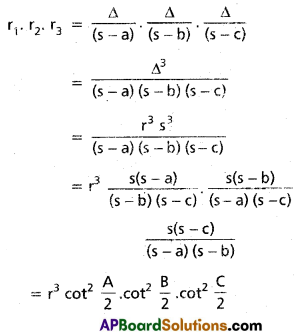
III.

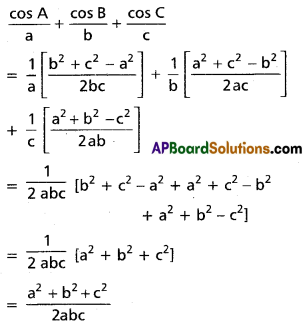
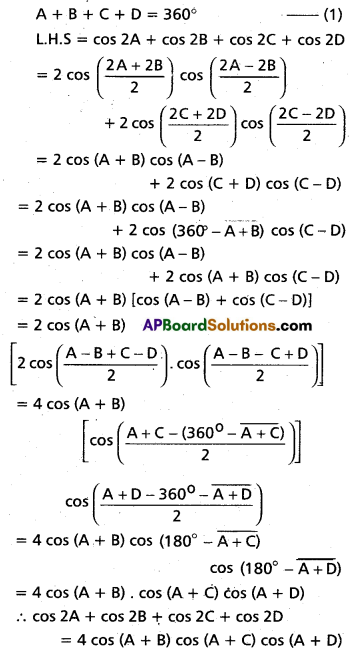
Question 1.
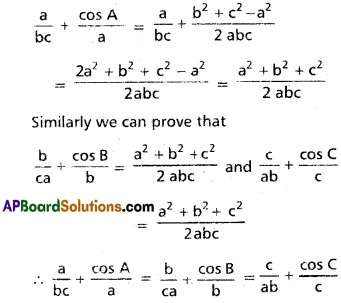
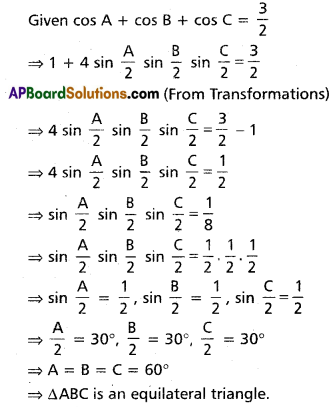
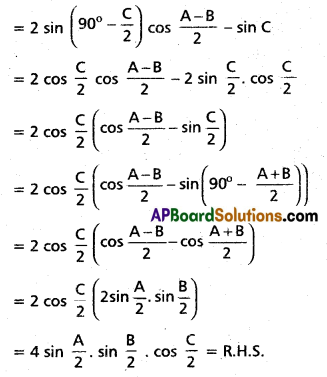
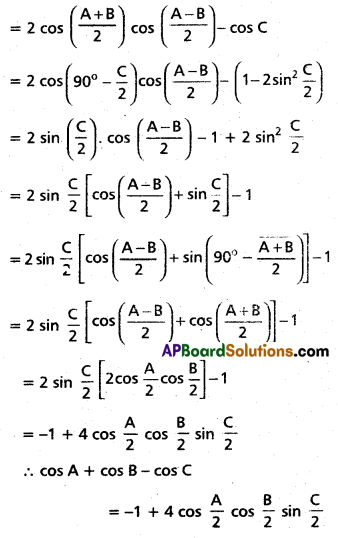
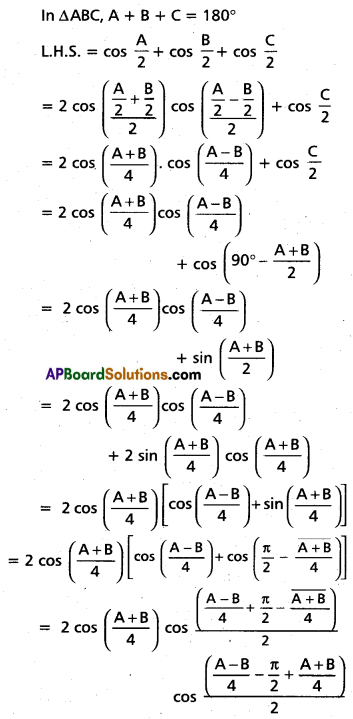
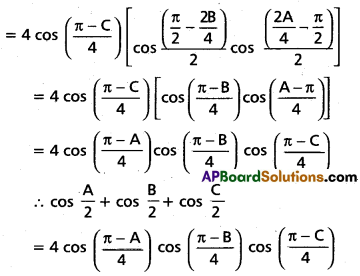
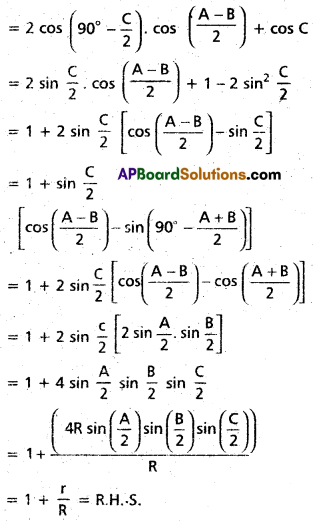
Show that cos A + cos B + cos C = 1 + \(\frac{r}{R}\)
Solution:
L.H.S = cos A + cos B + cos C
= 2 cos(\(\frac{A+B}{2}\)) cos(\(\frac{A-B}{2}\)) + cos C

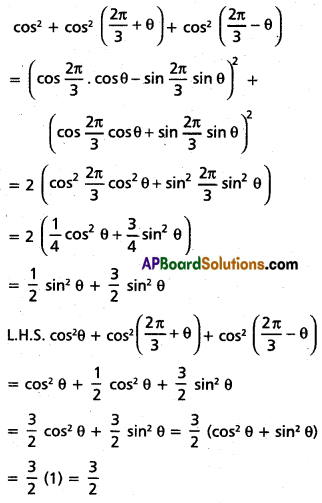
Question 2.
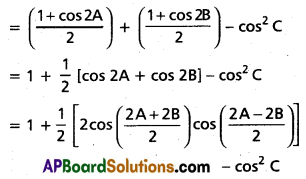
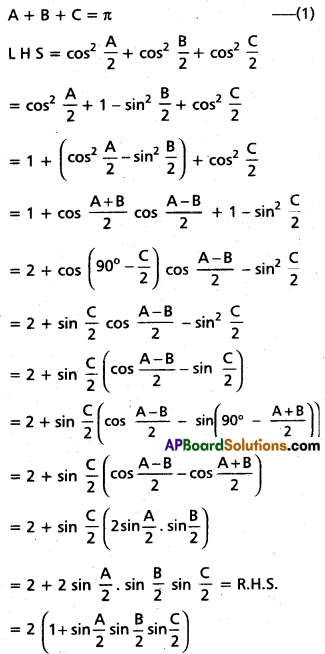
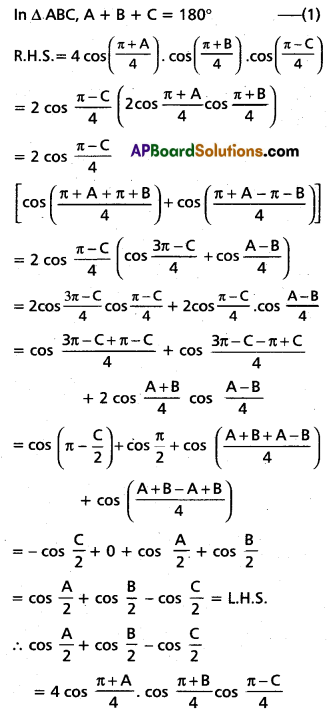
Show that \(\cos ^{2} \frac{A}{2}+\cos ^{2} \frac{B}{2}+\cos ^{2} \frac{C}{2}=2+\frac{r}{2 R}\)
Solution:

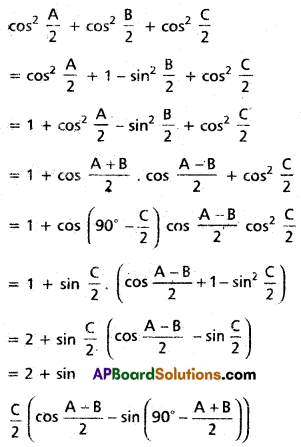
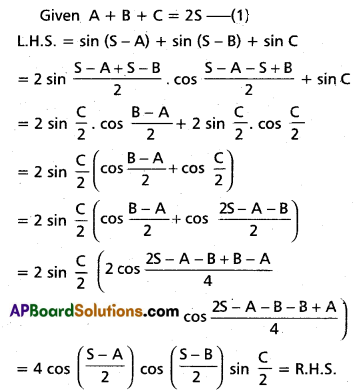
Question 3.
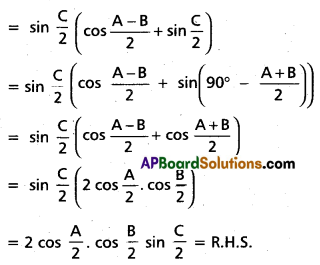
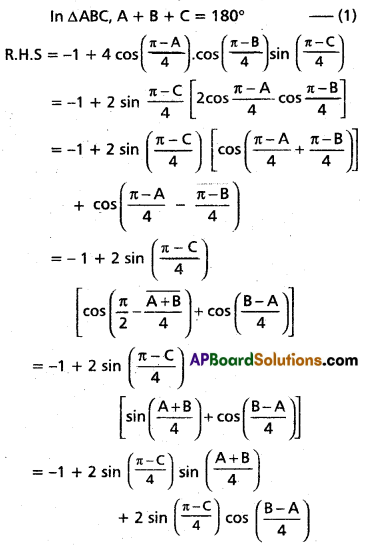
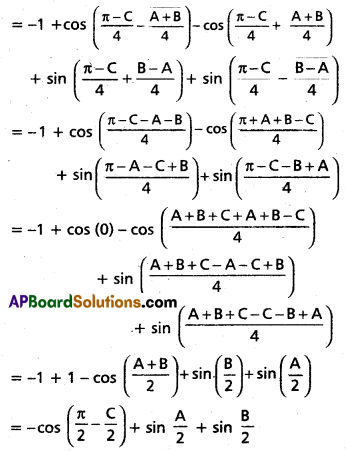
Show that \(\sin ^{2} \frac{A}{2}+\sin ^{2} \frac{B}{2}+\sin ^{2} \frac{C}{2}=1-\frac{r}{2 R}\)
Solution:

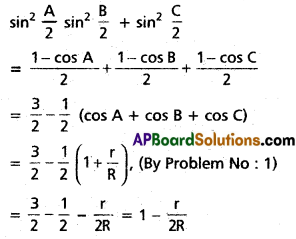
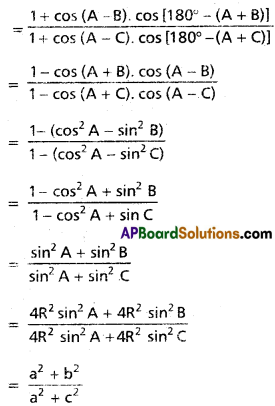
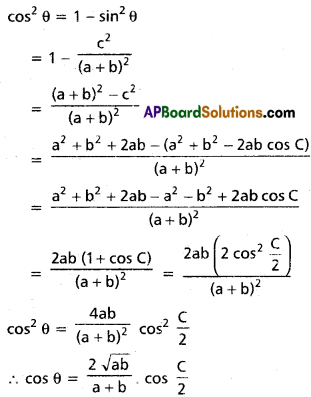
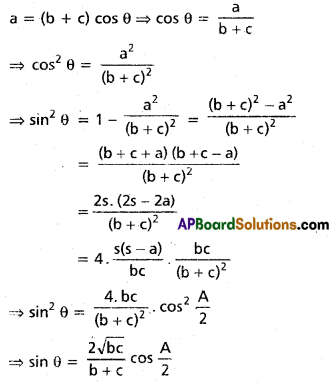
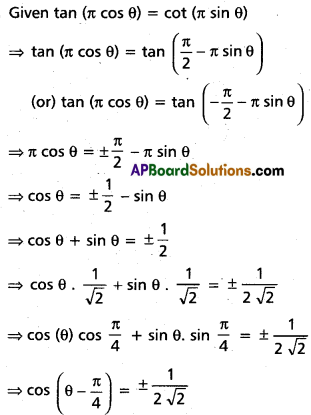
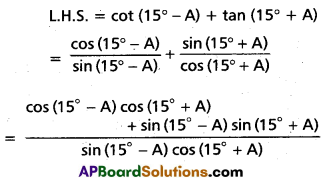
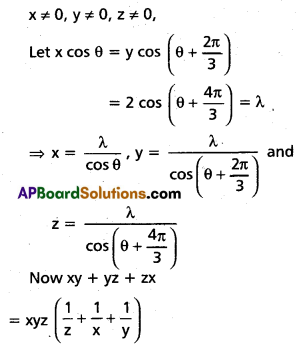
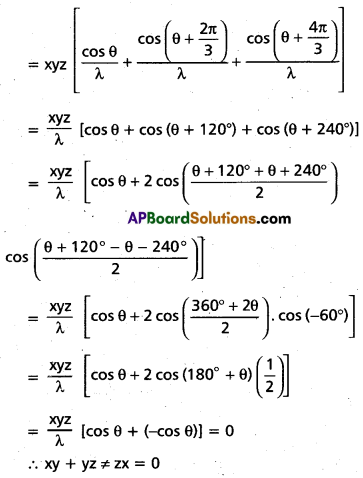
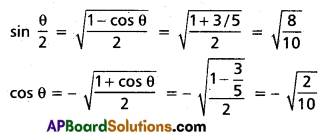
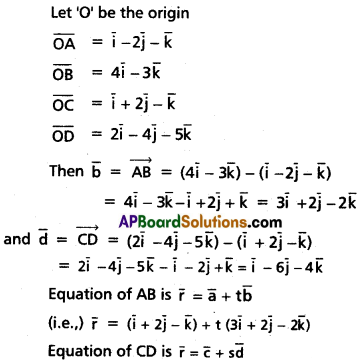
Question 4.
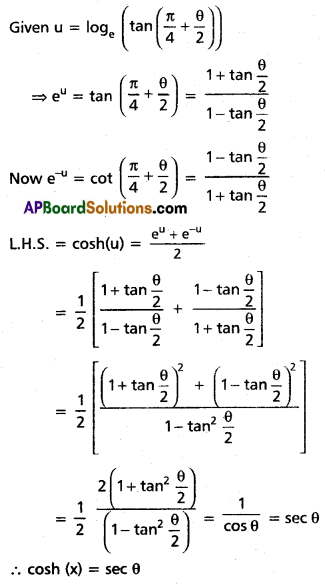
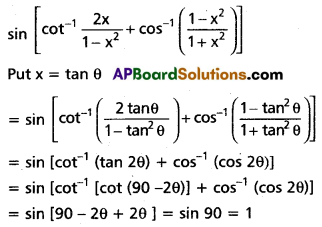
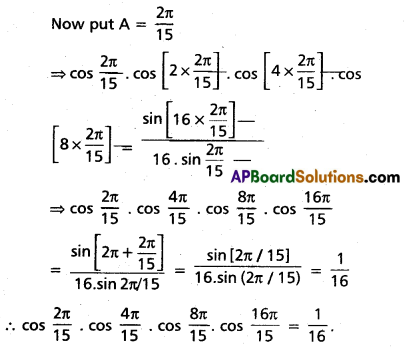
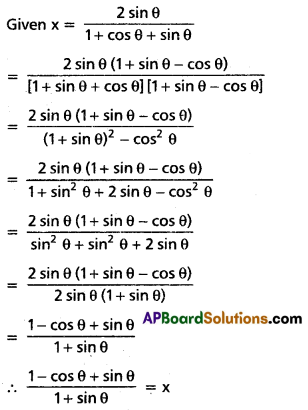
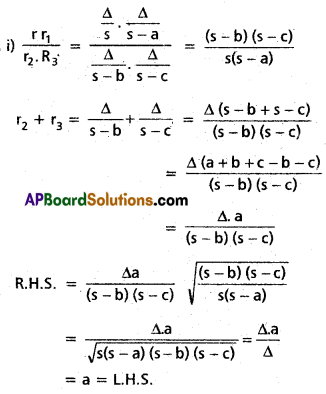
Show that
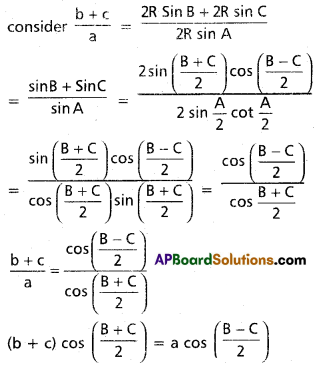
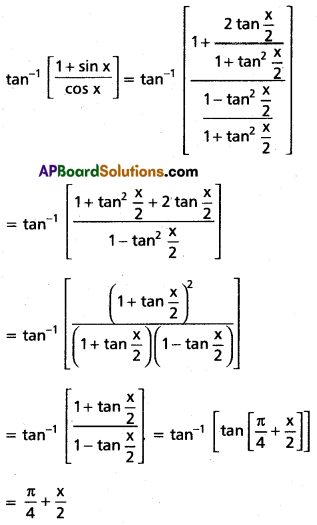
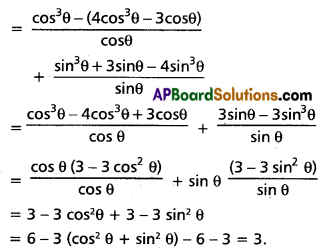
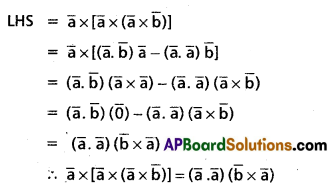
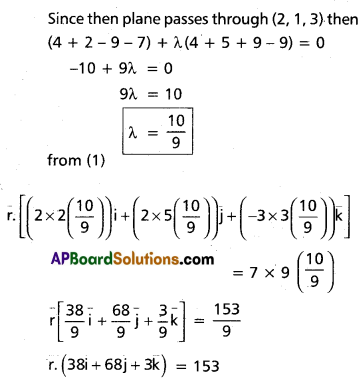
(i) a = (r₂ + r₃) \(\sqrt{\frac{r r_{1}}{r_{2} r_{3}}}\)
(ii) ∆ = r₁r₂ \(\sqrt{\frac{4 R-r_{1}-r_{2}}{r_{1}+r_{2}}}\)
Solution:

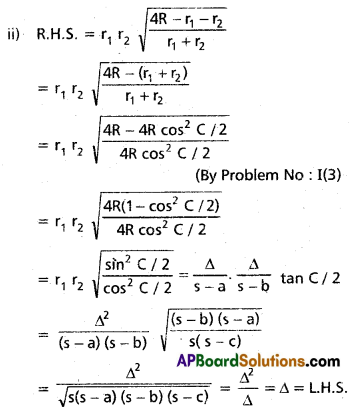
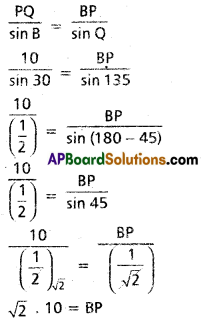
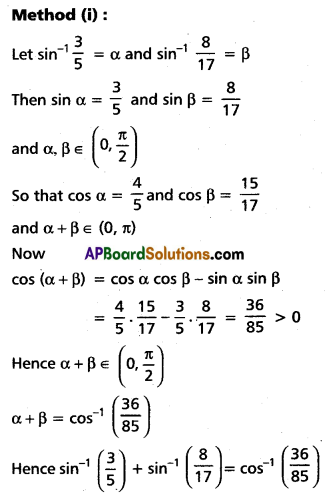
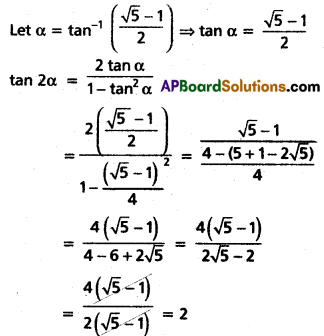
Question 5.
Prove that \(r_{1}^{2}+r_{2}^{2}+r_{3}^{2}+r^{2}\) = 16R² – (a² + b² + c²).
Solution:

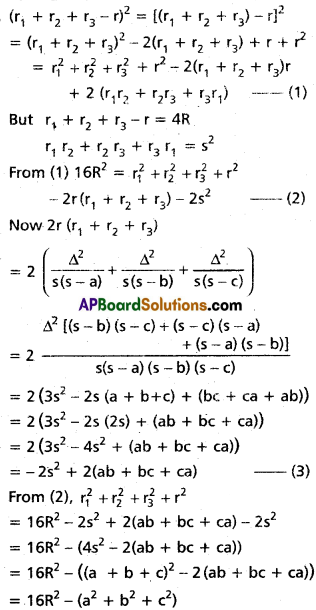
Question 6.
If p₁, p₂, p₃ are altitudes drawn from vertices A, B, C to the opposite sides of a triangle respectively, then show that
(i) \(\frac{1}{p_{1}}+\frac{1}{p_{2}}+\frac{1}{p_{3}}=\frac{1}{r}\)
(ii) \(\frac{1}{p_{1}}+\frac{1}{p_{2}}-\frac{1}{p_{3}}=\frac{1}{r_{3}}\)
(iii) p₁ . p₂ . p₃ = \(\frac{(a b c)^{2}}{8 R^{3}}=\frac{8 \Delta^{3}}{a b c}\)
Solution:

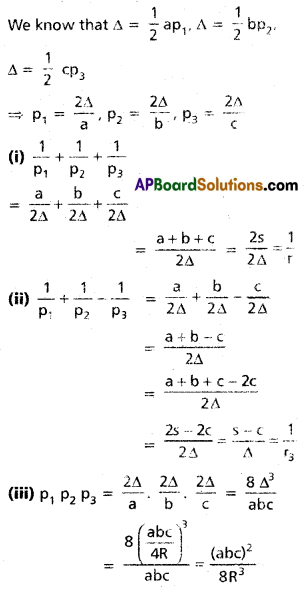
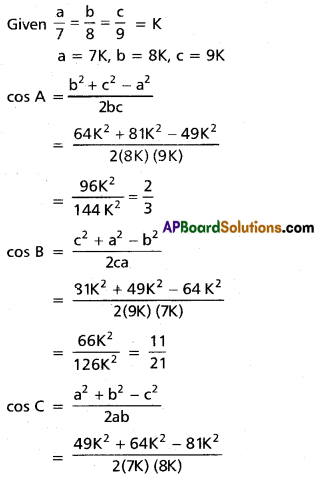
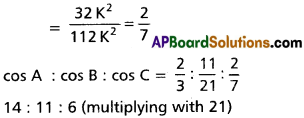
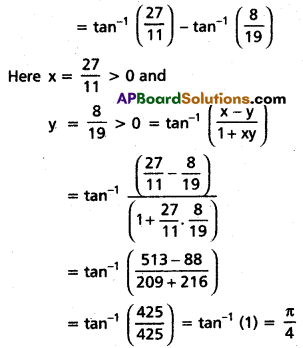
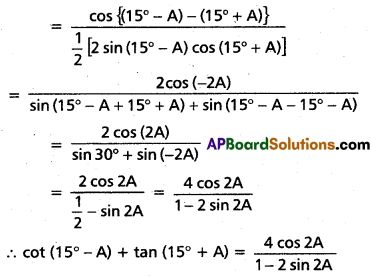
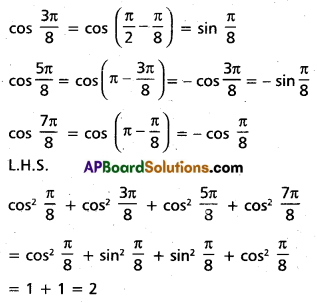
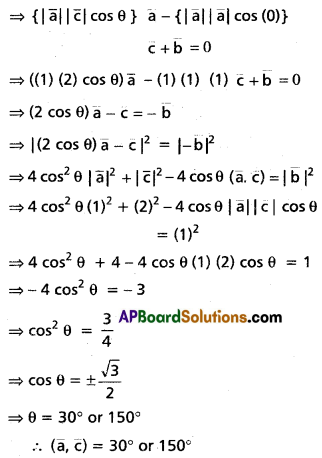
Question 7.
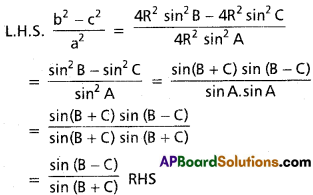
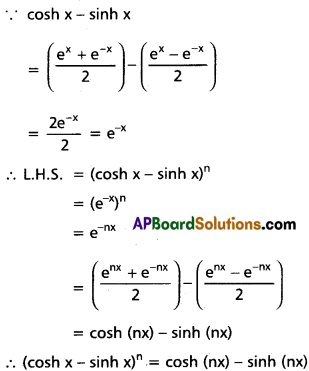
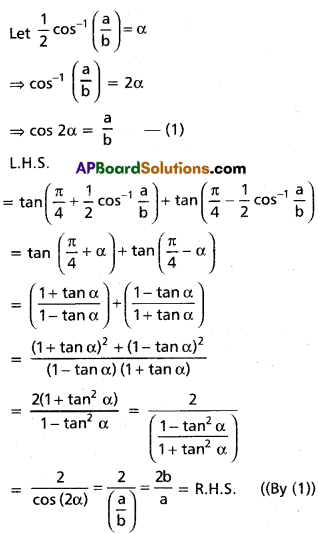
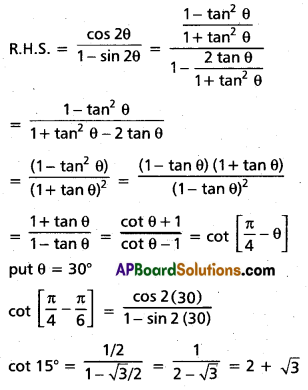
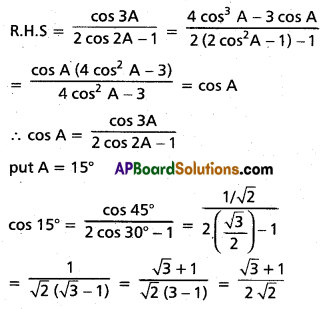
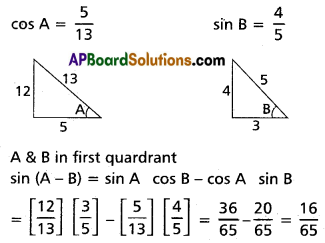
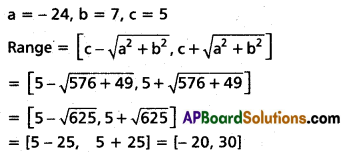
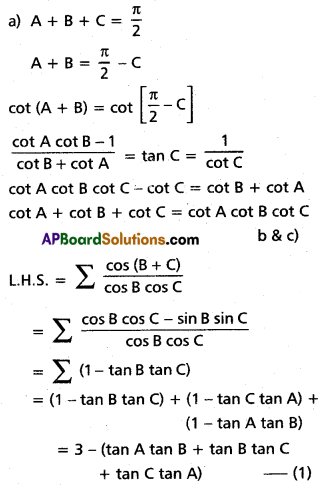
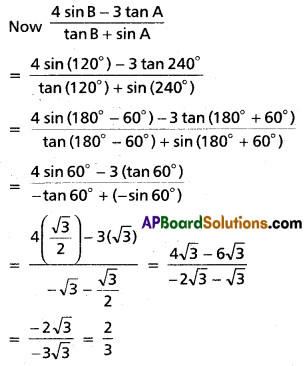
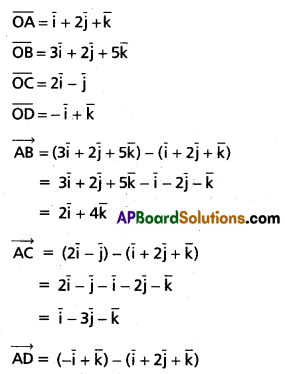
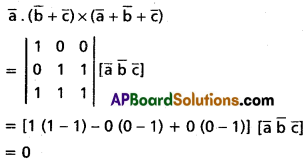
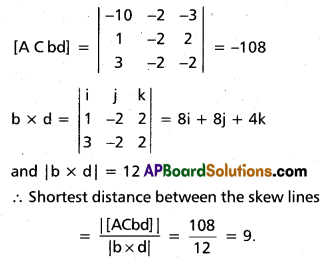
If a = 13, b = 14, c = 15, show that R = \(\frac{65}{8}\), r = 4, r₁ = \(\frac{21}{2}\), r₂ = 12 and r₃ = 14.
Solution:
a = 13, b = 14, c = 15
s = \(\frac{a+b+c}{2}\)
= \(\frac{13+14+15}{2}\)
= 21
s – a = 21 – 13 = 8
s – b = 21 – 14 = 7
s – c = 21 – 15 = 6

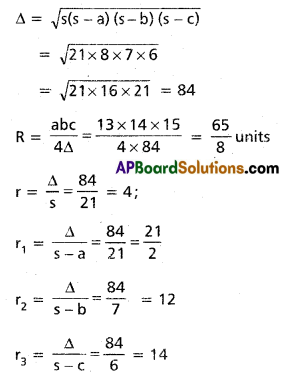
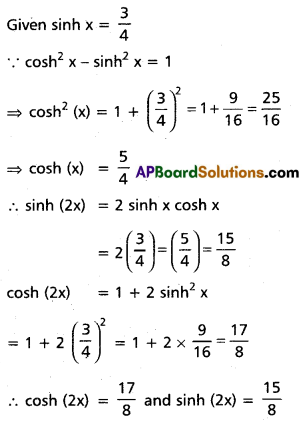
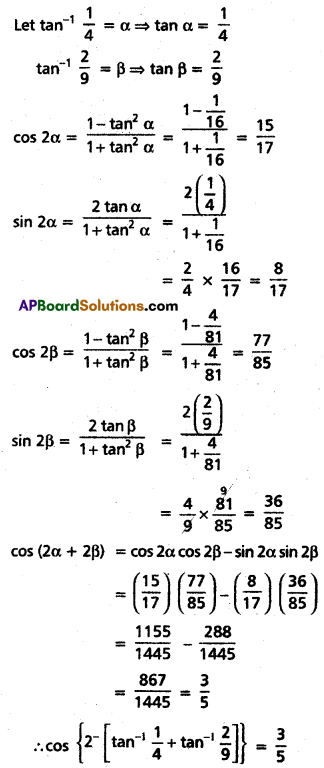
Question 8.
If r₁ = 2, r₂ = 3, r₃ = 6 and r = 1, prove that a = 3, b = 4 and c = 5.
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Properties of Triangles Solutions Exercise 10(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Properties of Triangles Solutions Exercise 10(a)

All problems in this exercise refer to ΔABC

I.

Question 1.
Show that Σa(sin B – sin C) = 0
Solution:

Question 2.
If a = √3 + 1 cms, ∠B = 30°, ∠C = 45°, then find c.
Solution:
∠B = 30°, ∠C = 45° and a = (√3 + 1) cms
A = 180° – (B + C)
= 180° – (30° + 45°)
= 180° – 75°
= 105°

Question 3.
If a = 2 cms, b = 3 cms, c = 4 cms, then find cos A.
Solution:
a = 2 cms, b = 3 cms and c = 4 cms

Question 4.
If a = 26 cms, b = 30 cms and cos C = \(\frac{63}{65}\), then find c.
Solution:
a = 26 cms, b = 30 cms and cos C = \(\frac{63}{65}\)
c² = a² + b² – 2ab cos C
⇒ c² = 676 + 900 – 2 × 26 × 30 × \(\frac{63}{65}\)
⇒ c² = 1576 – 1512
⇒ c² = 64
⇒ c = 8

Question 5.
If the angles are in the ratio 1 : 5 : 6, then find the ratio of its sides.
Solution:
Given \(\frac{A}{1}=\frac{B}{5}=\frac{C}{6}\), B = 5A, C = 6A
A + B + C = 180°
⇒ A + 5A + 6A = 180°
⇒ 12A = 180°
⇒ A = 15°
∴ B = 5A = 75°
∴ C = 6A = 90°
a : b : c = sin A : sin B : sin C
= sin 15° : sin 75° : sin 90°
= \(\frac{\sqrt{3}-1}{2 \sqrt{2}}: \frac{\sqrt{3}+1}{2 \sqrt{2}}: 1\)
= (√3 – 1) : (√3 + 1) : 2√2

Question 6.
Prove that 2(bc cos A + ca cos B + ab cos C) = a² + b² + c².
Solution:
L.H.S = Σ2bc cos A
= Σ2bc \(\frac{\left(b^{2}+c^{2}-a^{2}\right)}{2 b c}\)
= Σ(b² + c² – a²)
= b² + c² – a² + c² + a² – b² + a² + b² – c²
= a² + b² + c²
= R.H.S

Question 7.
Prove that \(\frac{a^{2}+b^{2}-c^{2}}{c^{2}+a^{2}-b^{2}}=\frac{\tan B}{\tan C}\)
Solution:
L.H.S = \(\frac{a^{2}+b^{2}-c^{2}}{c^{2}+a^{2}-b^{2}}=\frac{\tan B}{\tan C}\)
[∵ c² = a² + b² – 2ab cos C and b² = a² + c² – 2ac cos B]

Question 8.
Prove that (b + c) cos A + (c + a) cos B + (a + b) cos C = a + b + c
Solution:
L.H.S = (b + c) cos A + (c + a) cos B + (a + b) cos C
= (b cos A + c cos A) + (c cos B + a cos B) + (a cos C + b cos C)
= (b cos C + c cos B) + (a cos C + c cos A) + (a cos B + b cos A)
= a + b + c
= R.H.S

Question 9.
Prove that (b – a cos C) sin A = a cos A sin C
Solution:
LHS = (b – a cos C) sin A
= (a cos C + c cos A – a cos C) sin A
= c cos A sin A [∵ b = a cos C + c cos A]
= (2R sin C) cos A sin A
= a cos A sin C (∵ 2R sin A = a)

Question 10.
If 4, 5 are two sides of a triangle and the included angle is 60°, find its area.
Solution:
Let a = 4, b = 5 are two sides and included angle is C = 60° then
Area of ∆ = \(\frac{1}{2}\) ab sin C
= \(\frac{1}{2}\) × 4 × 5 × sin 60°
= 2 × 5 × \(\frac{\sqrt{3}}{2}\)
= 5√3 sq.cm

Question 11.
Show that \(b \cos ^{2} \frac{C}{2}+c \cos ^{2} \frac{B}{2}=s\)
Solution:

Question 12.
If \(\frac{a}{\cos A}=\frac{b}{\cos B}=\frac{c}{\cos C}\), then show that ∆ABC is equilateral.
Solution:
Given that \(\frac{a}{\cos A}=\frac{b}{\cos B}=\frac{c}{\cos C}\)
⇒ \(\frac{2 R \sin A}{\cos A}=\frac{2 R \sin B}{\cos B}=\frac{2 R \sin C}{\cos C}\)
⇒ \(\frac{\sin A}{\cos A}=\frac{\sin B}{\cos B}=\frac{\sin C}{\cos C}\)
⇒ tan A = tan B = tan C
⇒ A = B = C
⇒ ∆ABC is an equilateral triangle.

II.

Question 1.
Prove that a cos A + b cos B + c cos C = 4R sin A sin B sin C.
Solution:
L.H.S = (2R sin A) cos A + (2R sin B) cos B + (2R sin C) cos C
= R (sin 2A + sin 2B + sin 2C)
= R (2 sin (A + B) cos (A – B) + sin 2C)
= R [2 sin (180° – C) cos (A – B) + sin 2C]
= R (2 sin C . cos (A – B) + 2 sin C . cos C)
= 2R sin C (cos (A – B)) + cos C)
= 2R sin C (cos (A – B) + cos (180° – \(\overline{\mathrm{A}+\mathrm{B}}\))
= 2R sin C [cos (A – B) – cos (A + B)]
= 2R sin C (2 sin A sin B)
= 4R sin A sin B sin C
= R.H.S.

Question 2.
Prove that Σa³ sin(B – C) = 0.
Solution:
L.H.S = Σa² [a sin (B – C)]
= Σa² [2R . sin A sin (B – C)]
= R Σ a² (2 sin (180° – \(\overline{\mathrm{B}+\mathrm{C}}\)) sin (B – C))
= R Σ a² [2 sin (B + C) . sin (B – C)]
= R Σ a² (sin² B – sin² C)
= R Σ a² \(\left(\frac{b^{2}}{4 R^{2}}-\frac{c^{2}}{4 R^{2}}\right)\)
= \(\frac{1}{2 R}\) Σ[a² (b² – c²)]
= \(\frac{1}{2 R}\) a² (b² – c²) + b² (c² – a²) + c² (a² – b²)]
= \(\frac{1}{2 R}\) (a²b² – a²c² + b²c² – a²b² + a²c² – b²c²)
= \(\frac{1}{2 R}\) × 0
= 0
= R.H.S

Question 3.
Prove that \(\frac{a \sin (B-C)}{b^{2}-c^{2}}=\frac{b \sin (C-A)}{c^{2}-a^{2}}=\frac{c \sin (A-B)}{a^{2}-b^{2}}\)
Solution:

Question 4.
Prove that \(\sum a^{2} \frac{a^{2} \sin (B-C)}{\sin B+\sin C}=0\)
Solution:

Question 5.
Prove that \(\frac{a}{b c}+\frac{\cos A}{a}=\frac{b}{c a}+\frac{\cos B}{b}=\frac{c}{a b}+\frac{\cos C}{c}\)
Solution:

Question 6.
Prove that \(\frac{1+\cos (A-B) \cos C}{1+\cos (A-C) \cos B}=\frac{a^{2}+b^{2}}{a^{2}+c^{2}}\)
Solution:

Question 7.
If C = 60°, then show that
(i) \(\frac{\mathbf{a}}{\mathbf{b}+\mathbf{c}}+\frac{\mathbf{b}}{\mathbf{c}+\mathbf{a}}=1\)
(ii) \(\frac{b}{c^{2}-a^{2}}+\frac{a}{c^{2}-b^{2}}=0\)
Solution:
∠C = 60°
⇒ c² = a² + b² – 2ab cos C
⇒ c² = a² + b² – 2ab cos 60°
⇒ c² = a² + b² – 2ab (\(\frac{1}{2}\))
⇒ c² = a² + b² – ab

Question 8.
If a : b : c = 7 : 8 : 9, find cos A : cos B : cos C.
Solution:

Question 9.
Show that \(\frac{\cos A}{a}+\frac{\cos B}{b}+\frac{\cos C}{c}=\frac{a^{2}+b^{2}+c^{2}}{2 a b c}\)
Solution:

Question 10.
Prove that (b – a) cos C + c (cos B – cos A) = c . sin(\(\frac{A-B}{2}\)) cosec(\(\frac{A+B}{2}\))
Solution:
L.H.S = b cos C – a cos C + c cos B – c cos A
= (b cos C + c cos B) – (a cos C + c cos A)
= a – b
= 2R (sin A – sin B)

Question 11.
Express \(a \sin ^{2} \frac{C}{2}+c \cdot \sin ^{2} \frac{A}{2}\) interms of s, a, b, c.
Solution:

Question 12.
If b + c = 3a, then rind the value of \(\cot \frac{\mathrm{B}}{2} \cot \frac{\mathrm{C}}{2}\)
Solution:

Question 13.
Prove that (b + c) cos (\(\frac{B+C}{2}\)) = a cos (\(\frac{B-C}{2}\))
Solution:

Question 14.
In ΔABC, show that \(\frac{b^{2}-c^{2}}{a^{2}}=\sin \frac{(B-C)}{(B+C)}\)
Solution:

III.

Question 1.
Prove that
(i) \(\cot \frac{A}{2}+\cot \frac{B}{2}+\cot \frac{C}{2}=\frac{s^{2}}{\Delta}\)
(ii) \(\tan \frac{A}{2}+\tan \frac{B}{2}+\tan \frac{C}{2}\) = \(\frac{b c+c a+a b-s^{2}}{\Delta}\)
(iii) \(\frac{\cot \frac{A}{2}+\cot \frac{B}{2}+\cot \frac{C}{2}}{\cot A+\cot B+\cot C}\) = \(\frac{(a+b+c)^{2}}{a^{2}+b^{2}+c^{2}}\)
Solution:

Question 2.
Show that
(i) Σ(a + b) tan(\(\frac{A-B}{2}\)) = 0
(ii) \(\frac{\mathbf{b}-\boldsymbol{c}}{\mathbf{b}+\mathbf{c}} \cot \frac{A}{2}+\frac{b+c}{b-c} \tan \frac{A}{2}\) = 2 cosec(B – C)
Solution:

Question 3.
(i) If sin θ = \(\frac{a}{b+c}\), then show that cos θ = \(\frac{2 \sqrt{b c}}{b+c} \cdot \cos \frac{A}{2}\)
Solution:

(ii) If a = (b + c) cos θ, then prove that sin θ = \(\frac{2 \sqrt{b c}}{b+c} \cos \left(\frac{A}{2}\right)\)
Solution:

(iii) For any anlge θ show that a cos θ = b cos (C + θ) + c cos (B – θ).
Solution:
b cos (C + θ) + c cos (B – θ)
= b (cos C . cos θ – sin C sin θ) + c (cos B cos θ + sin B sin θ)
= (b cos C + c cos B) cos θ + (-b sin C + C sin B) sin θ
= a cos θ + (-2R sin B sin C + 2R sin B sin C) sin θ
= a cos θ

Question 4.
If the angles of ∆ABC are in A.P and b : c = √3 : √2 , then show that A = 75°.
Solution:
∵ The angles A, B, C of a triangle are in A.P.
⇒ 2B = A + C
⇒ 3B = A + B + C
⇒ 3B = 180°
⇒ B = 60°

Question 5.
If \(\frac{a^{2}+b^{2}}{a^{2}-b^{2}}=\frac{\sin C}{\sin (A-B)}\), prove that ∆ABC is either isosceles or right-angled.
Solution:

⇒ sin 2A = sin 2B
⇒ A = B
⇒ ∆ABC is isosceles
or 2A = 180° – 2B
or A = 90° – B
or A + B = 90°
so A ≠ B ⇒ ∆ABC is a right-angled triangle
∴ ∆ABC is either isosceles (or) right-angled.

Question 6.
If cos A + cos B + cos C = \(\frac{3}{2}\), then show that the triangle is equilateral.
Solution:

Question 7.
If cos² A + cos² B + cos² C = 1, then show that ∆ABC is right-angled.
Solution:
Given cos² A + cos² B + cos² C = 1 …….(1)
Now cos² A + cos² B + cos² C
= cos² A + 1 – sin² B + cos² C
= 1 + (cos² A – sin² B) + cos² C
= 1 + cos (A + B) cos (A – B) + cos² C
= 1 + cos (180° – C) cos (A – B) + cos² C
= 1 – cos C . cos (A – B) + cos² C
= 1 – cos C (cos (A – B) – cos C)
= 1 – cos C (cos (A – B) – cos (180° – \(\overline{A+B}\)))
= 1 – cos C (cos (A – B) + cos (A + B))
= 1 – cos C (2 cos A cos B)
= 1 – 2 cos A cos B cos C
Substituting in (1) we get
1 – 2 cos A cos B cos C = 1
∴ 2 cos A cos B cos C = 0
∴ cos A = 0 or cos B = 0 or cos C = 0
i.e., A = 90° or B = 90° or C = 90°
∴ ∆ABC is right-angled.

Question 8.
If a² + b² + c² = 8R², then prove that the triangle is right angled.
Solution:
Given a² + b² + c² = 8R²
⇒ 4R² (sin² A + sin² B + sin² C) = 8R²
⇒ sin² A + sin² B + sin² C = 2 ……(1)
Now sin² A + sin² B + sin² C
= 1 – cos² A + sin² B + sin² C
= 1 – (cos² A – sin² B) + sin² C
= 1 – cos (A + B) . cos (A – B) + sin² C
= 1 – cos (180° – C) cos (A – B) + sin² C
= 1 + cos C cos (A – B) + 1 – cos² C
= 2 + cos C (cos (A – B) – cos C)
= 2 + cos C (cos (A – B) – cos (180° – \(\overline{A+B}\)))
= 2 + cos C (cos (A – B) + cos (A + B))
= 2 + cos C (2 cos A cos B)
= 2 + 2 cos A cos B cos C
Substituting in (1), we get
2 + 2 cos A cos B cos C = 2
2 cos A cos B cos C = 0
⇒ cos A = 0 or cos B = 0 or cos C = 0
∴ A = 90° or B = 90° or C = 90°
∴ ΔABC is right-angled.

Question 9.
If cot \(\frac{A}{2}\), cot \(\frac{B}{2}\), cot \(\frac{C}{2}\) are in A.P., then prove that a, b, c are in A.P.
Solution:
∵ cot \(\frac{A}{2}\), cot \(\frac{B}{2}\), cot \(\frac{C}{2}\) are in A.P
⇒ \(\frac{(s)(s-a)}{\Delta}, \frac{(s)(s-b)}{\Delta}, \frac{(s)(s-c)}{\Delta}\) are in A.P.
⇒ s – a, s – b, s – c are in A.P
⇒ -a, -b, -c are in A.P
⇒ a, b, c are in A.P

Question 10.
If \(\sin ^{2} \frac{A}{2}, \sin ^{2} \frac{B}{2}, \sin ^{2} \frac{C}{2}\) are in H.P., show that a, b, c are in H.P.
Solution:

Question 11.
If C = 90° then prove that \(\frac{a^{2}+b^{2}}{a^{2}-b^{2}}\) sin (A – B) = 1
Solution:

Question 12.
Show that \(\frac{a^{2}}{4} \sin 2 C+\frac{c^{2}}{4} \sin 2 A\) = ∆
Solution:
LHS = \(\frac{a^{2}}{4} \sin 2 C+\frac{c^{2}}{4} \sin 2 A\)
= \(\frac{4 R^{2} \sin ^{2} A}{4}\) 2 sin C cos C + \(\frac{4 R^{2} \sin ^{2} C}{4}\) 2 sin A cos A
= 2R² sin²A sin C cos C + 2R² sin²C sin A cos A
= 2R² sin A sin C (sin A cos C + cos A sin C)
= 2R² sin A sin C sin(A + C)
= 2R² sin A sin C sin(180 – B)
= 2R² sin A sin B sin C
= ∆
= RHS

Question 13.
A lamp post is situated at the middle point I the side AC of a triangular plot A with BC = 7 meters, CA = 8 meters, and AB = 9 meters, lamp post subtends an angle of 15° at point B. find H height of the lamp post.
Solution:
Let MP be the height of the lamp post

Question 14.
Two ships leave a port at the same time. One goes 24 km. per hour in the direction N45°E and other travels 32 km per hour in the direction S75°E. Find the distance between the ships at the end of 3 hours.
Solution:
Given the first ship goes 24 km per hour After 3 hours the first ship goes 72 km.

Given the second ship goes 32 km per hour After 3 hours, the second ship goes 96 km.
Let AB = x
∠AOB = 180° – (75 + 45) = 60°
Apply cosine rule for ∆AOB,
cos 60° = \(\frac{(72)^{2}+(96)^{2}-x^{2}}{2(72)(96)}\)
⇒ \(\frac{1}{2}=\frac{5184+9216-x^{2}}{13824}\)
⇒ 13824 = 28800 – 2x²
⇒ 2x² = 14976
⇒ x² = 7488
⇒ x = 86.4 (Appx)
At the end of 3 hours the difference between ships was 86.4 km.

Question 15.
A tree stands vertically on the slant of the hill. From A point on the ground 35 meters down the hill from the base of the tree, the angle, the elevation of the top of the tree is 60° if the angle of elevation of the foot of the tree from A is 15°, then find the height of the tree.
Solution:
Let BC be the height of the tree
BC = h
Let BD = x, AD = y
Given AB = 35 m

Question 16.
The upper \(\frac{3}{4}\)th portion of a vertical pole subtends an angle tan-1(\(\frac{3}{5}\)) at a point in the horizontal plane through its foot and at a distance 40 m from the foot. Given that the vertical pole is at a height less than 100 m from the ground, find its height.
Solution:

⇒ 6400 + h² = 200h
⇒ h² – 200h + 6400 = 0
⇒ h² – 160h – 40h + 6400 = 0
⇒ h(h – 160) – 40(h – 160) = 0
⇒ (h – 160) (h – 40) = 0
⇒ h = 40 or 160
but the height of the pole should be less than 100m
∴ h = 40 m

Question 17.
AB is a vertical pole with B at the ground level and A at the top. A man finds that the angle of elevation of point A from a certain point C on the ground is 60°. He moves away from the pole along the line BC to a point D such that CD = 7 m. From D, the angle of elevation of point A is 45°. Find the height of the pole.
Solution:
Let ‘h’ be the height of the pole
AB = h
given CD = 7 m
∠ACB = 60°, ∠ADB = 45°, Let BC = x

Question 18.
Let an object be placed at some height h cm and let P and Q two points of observation which are at a distance of 10 cm apart on a line inclined at an angle of 15° to the horizontal. If the angles of elevation of the object from P and Q are 30° and 60° respectively then find h.
Solution:
Let AB = Height of the object from ‘A’ = h m
Given that P & Q are two observations,
PQ = 10 cms

From ∆APB,
∠P = 30; ∠A = 90; ∠B = ?
A + P + B = 180°
⇒ 30° + 30° + B = 180°
⇒ B = 180° – 120°
⇒ B = 60°
From ∆BQC,
∠Q = 60°; ∠C = 90°; ∠B = ?
Q + C + B = 180°
⇒ 60° + 90° + B = 180°
⇒ B = 180° – 150°
⇒ B = 30°
From ∆BQP,
∠P = 15; ∠B = 30; ∠Q = ?
P + B + Q = 180°
⇒ 15° + 30° + Q = 180°
⇒ Q = 180° – 45°
⇒ Q = 135°
Applying the ‘sin’ rule for ∆BQP

From ∆PAB,
sin 30° = \(\frac{B A}{B P}\)
BP . sin 30° = AB = h
√2 × 10 × \(\frac{1}{2}\) = AB = h
5√2 = AB = h
∴ h = 5√2

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Hyperbolic Functions Solutions Exercise 9(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Hyperbolic Functions Solutions Exercise 9(a)

Question 1.
If sinh x = \(\frac{3}{4}\), find cosh (2x) and sinh (2x).
Solution:

Question 2.
If sinh x = 3, then show that x = log_e(3 + √10).
Solution:

Question 3.
Prove that
(i) tanh (x – y) = \(\frac{\tanh x-\tanh y}{1-\tanh x \tanh y}\)
Solution:

(ii) coth (x – y) = \(\frac{{coth} x \cdot {coth} y-1}{{coth} y-{coth} x}\)
Solution:

Question 4.
Prove that
(i) (cosh x – sinh x)ⁿ = cosh (nx) – sinh (nx), for any n ∈ R.
Solution:

(ii) (cosh x + sinh x)ⁿ = cosh (nx) + sinh (nx), for any n ∈ R.
Solution:

Question 5.
Prove that \(\frac{\tanh x}{{sech} x-1}+\frac{\tanh x}{{sech} x+1}\) = -2 cosech x, for x ≠ 0
Solution:

Question 6.
Prove that \(\frac{\cosh x}{1-\tanh x}+\frac{\sinh x}{1-{coth} x}\) = sinh x + cosh x, for x ≠ 0
Solution:

Question 7.
For any x ∈ R, prove that cosh⁴x – sinh⁴x = cosh (2x)
Solution:
L.H.S = cosh⁴x – sinh⁴x
= (cosh²x)² – (sinh²x)²
= [cosh²x – sinh²x] [cosh²x + sinh²x]
= (1) cosh (2x)
= cosh (2x)
∴ cosh⁴x – sinh⁴x = cosh (2x)

Question 8.
If u = \(\log _{e}\left(\tan \left(\frac{\pi}{4}+\frac{\theta}{2}\right)\right)\) and if cos θ > 0,then prove that cosh u = sec θ.
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Inverse Trigonometric Functions Solutions Exercise 8(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Inverse Trigonometric Functions Solutions Exercise 8(a)

I.

Question 1.
Evaluate the following.
(i) \(\sin ^{-1}\left(\frac{-\sqrt{3}}{2}\right)\)
Solution:

(ii) \(\cos ^{-1}\left(\frac{1}{\sqrt{2}}\right)\)
Solution:
\(\cos ^{-1}\left(\frac{1}{\sqrt{2}}\right)=\cos ^{-1}\left(\cos \frac{\pi}{4}\right)=\frac{\pi}{4}\)

(iii) sec^-1(-√2)
Solution:

(iv) cot^-1(-√3)
Solution:

(v) \(\sin \left(\frac{\pi}{3}-\sin ^{-1}\left(\frac{-1}{2}\right)\right)\)
Solution:

(vi) \(\sin ^{-1}\left(\sin \frac{5 \pi}{6}\right)\)
Solution:

(vii) \(\cos ^{-1}\left(\cos \frac{5 \pi}{4}\right)\)
Solution:

Question 2.
Find the values of
(i) \(\sin \left(\cos ^{-1} \frac{3}{5}\right)\)
Solution:
\(\sin \left(\cos ^{-1} \frac{3}{5}\right)=\sin \left(\sin ^{-1} \frac{4}{5}\right)=\frac{4}{5}\)

(ii) \(\tan \left({cosec}^{-1} \frac{65}{63}\right)\)
Solution:
\(\tan \left({cosec}^{-1} \frac{65}{63}\right)=\tan \left(\tan ^{-1} \frac{63}{16}\right)\) = \(\frac{63}{16}\)

(iii) \(\sin \left(2 \sin ^{-1} \frac{4}{5}\right)\)
Solution:

(iv) \(\sin ^{-1}\left(\sin \frac{33 \pi}{7}\right)\)
Solution:

(v) \(\cos ^{-1}\left(\cos \frac{17 \pi}{6}\right)\)
Solution:

Question 3.
Simplify each of the following.
(i) \(\tan ^{-1}\left[\frac{\sin x}{1+\cos x}\right]\)
Solution:

(ii) tan^-1(sec x + tan x)
Solution:

(iii) \(\tan ^{-1} \sqrt{\frac{1-\cos x}{1+\cos x}}\)
Solution:

(iv) sin^-1(2 cos²θ – 1) + cos-1(1 – 2 sin²θ)
Solution:
sin^-1(cos 2θ) + cos^-1(cos 2θ)
= sin^-1[sin (90° – 2θ)] + cos^-1(cos 2θ)
= 90° – 2θ + 2θ
= 90°

(v) \(\tan ^{-1}\left(x+\sqrt{1+x^{2}}\right)\); x ∈ R
Solution:

II.

Question 1.
Prove that
(i) \(\sin ^{-1} \frac{3}{5}+\sin ^{-1} \frac{8}{17}=\cos ^{-1} \frac{36}{85}\)
Solution:

(ii) \(\sin ^{-1} \frac{3}{5}+\cos ^{-1} \frac{12}{13}=\cos ^{-1} \frac{33}{65}\)
Solution:

(iii) \(\tan \left[\cot ^{-1} 9+{cosec}^{-1} \frac{\sqrt{41}}{4}\right]=1\)
Solution:

(iv) \(\cos ^{-1} \frac{4}{5}+\sin ^{-1} \frac{3}{\sqrt{34}}=\tan ^{-1} \frac{27}{11}\)
Solution:

Question 2.
Find the values of
(i) \(\sin \left(\cos ^{-1} \frac{3}{5}+\cos ^{-1} \frac{12}{13}\right)\)
Solution:

(ii) \(\tan \left(\sin ^{-1} \frac{3}{5}+\cos ^{-1} \frac{5}{\sqrt{34}}\right)\)
Solution:

(iii) \(\cos \left(\sin ^{-1} \frac{3}{5}+\sin ^{-1} \frac{5}{13}\right)\)
Solution:
Let \(\sin ^{-1} \frac{3}{5}\) = α and \(\sin ^{-1} \frac{5}{13}\) = β

Question 3.
Prove that
(i) \(\cos \left[2 \tan ^{-1} \frac{1}{7}\right]=\sin \left[2 \tan ^{-1} \frac{3}{4}\right]\)
Solution:

(ii) \(\tan \left[2 \tan ^{-1}\left(\frac{\sqrt{5}-1}{2}\right)\right]=2\)
Solution:

(iii) \(\cos \left\{2\left[\tan ^{-1}\left(\frac{1}{4}\right)+\tan ^{-1}\left(\frac{2}{9}\right)\right]\right\}=\frac{3}{5}\)
Solution:

Question 4.
Prove that
(i) \(\tan ^{-1} \frac{1}{7}+\tan ^{-1} \frac{1}{13}-\tan ^{-1} \frac{2}{9}=0\)
Solution:

(ii) \(\tan ^{-1} \frac{1}{2}+\tan ^{-1} \frac{1}{5}+\tan ^{-1} \frac{1}{8}=\frac{\pi}{4}\)
Solution:

(iii) \(\tan ^{-1} \frac{3}{4}+\tan ^{-1} \frac{3}{5}-\tan ^{-1} \frac{8}{19}=\frac{\pi}{4}\)
Solution:

(iv) \(\tan ^{-1} \frac{1}{7}+\tan ^{-1} \frac{1}{8}\) = \(\cot ^{-1} \frac{201}{43}+\cot ^{-1} 18\)
Solution:

Question 5.
Show that
(i) sec² (tan^-1 2) + cosec² (cot^-1 2) = 10
Solution:
Let a = tan^-1 2 ⇒ tan α = 2
sec² α = 1 + tan^-1 α = 1 + 4 = 5
Let β = cot^-1 2 ⇒ cot β = 2
cosec² β = 1 + cot² β = 1 + 4 = 5
LHS = sec² (tan^-1 2) + cosec² (cot^-1 2)
= 5 + 5
= 10
= RHS

(ii) Find the value of \(\left(\cos ^{-1} \frac{4}{5}+\tan ^{-1} \frac{2}{3}\right)\)
Solution:

(iii) If sin^-1 x – cos^-1 x = \(\frac{\pi}{6}\) then find x.
Solution:

III.

Question 1.
Prove that
(i) \(2 \sin ^{-1} \frac{3}{5}-\cos ^{-1} \frac{5}{13}=\cos ^{-1} \frac{323}{325}\)
Solution:

(ii) \(\sin ^{-1} \frac{4}{5}+2 \tan ^{-1} \frac{1}{3}=\frac{\pi}{2}\)
Solution:

(iii) \(4 \tan ^{-1} \frac{1}{5}+\tan ^{-1} \frac{1}{99}-\tan ^{-1} \frac{1}{70}=\frac{\pi}{4}\)
Solution:

Question 2.
(i) If α = \({tan}^{-1}\left(\frac{\sqrt{1+x^{2}}-\sqrt{1-x^{2}}}{\sqrt{1+x^{2}}+\sqrt{1-x^{2}}}\right)\) then prove that x² = sin 2α.
Solution:

(ii) Prove that tan\(\left\{2-\tan ^{-1}\left(\frac{\sqrt{1+x^{2}}-1}{x}\right)\right\}=\mathbf{x}\)
Solution:

(iii) Prove that \(\sin \left[\cot ^{-1} \frac{2 x}{1-x^{2}}+\cos ^{-1}\left(\frac{1-x^{2}}{1+x^{2}}\right)\right]\) = 1
Solution:

(iv) Prove that \(\left\{\frac{\pi}{4}+\frac{1}{2} \cos ^{-1}\left(\frac{a}{b}\right)\right\}\)
Solution:

Question 3.
(i) If cos^-1 p + cos^-1 q + cos^-1 r = π, then prove that p² + q² + r² + 2pqr = 1
Solution:
Let cos^-1 p = A, cos^-1 q = B and cos^-1 r = C
then A + B + C = π ………(1)
and p = cos A, q = cos B and r cos C
Now p² + q² + r² = cos² A + cos² B + cos² C
= cos² A + (1 – sin² B + cos² C)
= 1 + (cos² A – sin² B) + cos² C
= 1 + cos (A + B) . cos (A – B) + cos² C
= 1 + cos (π – C) cos (A – B) + cos² C (By (1))
= 1 – cos C cos (A – B) + cos² C
= 1 – cos C [cos (A – B) – cos C]
= 1 – cos C [cos (A – B) – cos(180° – \(\overline{A+B}\)]
= 1 – cos C [cos (A – B) + cos (A + B)]
= 1 – cos C [2 cos A cos B]
= 1 – 2 pqr
∴ p² + q² + r² + 2pqr = 1

(ii) If \(\sin ^{-1}\left(\frac{2 p}{1+p^{2}}\right)-\cos ^{-1}\left(\frac{1-q^{2}}{1+q^{2}}\right)\) = \(\tan ^{-1}\left(\frac{2 x}{1-x^{2}}\right)\), then prove that x = \(\frac{p-q}{1+p q}\)
Solution:

(iii) If a, b, c are distinct non-zero real numbers having the same sign, prove that \(\cot ^{-1}\left(\frac{a b+1}{a-b}\right)+\cot ^{-1}\left(\frac{b c+1}{b-c}\right)\) + \(\cot ^{-1}\left(\frac{c a+1}{c-a}\right)\) = π or 2π
Solution:
Since (a – b) + (b – c) + (c – a) = 0.
(a – b), (b – c), (c – a) all cannot have the same sign.
Now two cases arise, namely, either two of these numbers are positive and one negative (or) two of these numbers are negative and one is positive.
Case (i): Without loss of generality, we assume that (a – b), (b – c) are both positive and (c – a) is negative

Case (ii): Without loss of generality, we assume that (a – b) and (b – c) are both negative and (c – a) is positive.

(iv) If sin^-1 (x) + sin^-1 (y) + sin^-1 (z) = π, then prove that \(x \sqrt{1-x^{2}}+y \sqrt{1-y^{2}}+z \sqrt{1-z^{2}}=2 x y z\)
Solution:
Let sin^-1 (x) = A, sin^-1 (y) = B and sin^-1 (z) = C
Then A + B + C = π …………(1)
and x = sin A, y = sin B and z = sin C
Now LHS = \(x \sqrt{1-x^{2}}+y \sqrt{1-y^{2}}+z \sqrt{1-z^{2}}\)
= sin A \(\sqrt{1-\sin ^{2} A}\) + sin B \(\sqrt{1-\sin ^{2} B}\) + sin C \(\sqrt{1-\sin ^{2} C}\)
= sin A cos A + sin B cos B + sin C cos C
= \(\frac{1}{2}\) [sin 2A + sin 2B + sin 2C]
= \(\frac{1}{2}\) [2 . sin (A + B) cos (A – B) + sin 2C]
= \(\frac{1}{2}\) [2 sin (π – c). cos (A – B) + sin 2C]
= \(\frac{1}{2}\) [2 sin C cos (A – B) + 2 sin C cos C]
= \(\frac{1}{2}\) (2 sin C) [cos (A – B) + cos C]
= sin C [cos (A – B) + cos (180° – \(\overline{A+B}\)]
= sin C [cos (A – B) – cos (A + B)]
= sin C [2 sin A sin B]
= 2 xyz
∴ \(x \sqrt{1-x^{2}}+y \sqrt{1-y^{2}}+z \sqrt{1-z^{2}}=2 x y z\)

(v) (a) If tan^-1 x + tan^-1 y + tan^-1 z = π, then prove that x + y + z = xyz.
Solution:
Let A = tan^-1 x, B = tan^-1 y, C = tan^-1 z
tan A = x, tan B = y, tan C = z
Given A + B + C = π ……….(1)
A + B = π – C
tan (A + B) = tan (π – C)
\(\frac{\tan A+\tan B}{1-\tan A \tan B}\) = -tan C
\(\frac{x+y}{1-x y}\) = -z
x + y = -z + xyz
∴ x + y + z = xyz

(b) If tan^-1 x + tan^-1 y + tan^-1 z = \(\frac{\pi}{2}\), then prove that xy + yz + zx = 1.
Solution:
Let tan^-1 x = A, tan^-1 y = B and tan^-1 z = C
Then A + B + C = \(\frac{\pi}{2}\) …….(1)
and x = tan A, y = tan B and z = tan C
∵ A + B + C = \(\frac{\pi}{2}\)
A + B = \(\frac{\pi}{2}\) – C
⇒ tan (A + B) = tan(\(\frac{\pi}{2}\) – C)
⇒ \(\frac{\tan A+\tan B}{1-\tan A \cdot \tan B}\) = cot C
⇒ \(\frac{x+y}{1-x y}=\frac{1}{z}\)
⇒ zx + yz = 1 – xy (or) xy + yz + zx = 1

Question 4.
Solve the following equations for x:
(i) \({Tan}^{-1}\left(\frac{x-1}{x-2}\right)+{Tan}^{-1}\left(\frac{x+1}{x+2}\right)=\frac{\pi}{4}\)
Solution:

(ii) \(\tan ^{-1}\left(\frac{1}{2 x+1}\right)+\tan ^{-1}\left(\frac{1}{4 x+1}\right)\) = \(\tan ^{-1} \frac{2}{x^{2}}\)
Solution:
Given that

⇒ x²(3x + 1) = 2x(4x + 3)
⇒ x [x(3x + 1) – 2(4x + 3)] = 0
⇒ x = 0 (or) 3x² – 7x – 6 = 0
⇒ x = 0 (or) 3x² – 9x + 2x – 6 = 0
⇒ x = 0 (or) 3x(x – 3) + 2(x – 3) = 0
⇒ x = 0 (or) (3x + 2) (x – 3) = 0
⇒ x = 0 (or) 3 (or) \(\frac{-2}{3}\)

(iii) \(3 \sin ^{-1}\left(\frac{2 x}{1+x^{2}}\right)-4 \cos ^{-1}\left(\frac{1-x^{2}}{1+x^{2}}\right)\) + \(2 \tan ^{-1}\left(\frac{2 x}{1-x^{2}}\right)=\frac{\pi}{3}\)
Solution:

(iv) sin^-1(1 – x) – 2 sin^-1 x = \(\frac{\pi}{2}\)
Solution:
Given sin^-1(1 – x) – 2 sin^-1x = \(\frac{\pi}{2}\)
Let sin^-1(1 – x) = α and sin^-1(x) = β
Then sin α = 1 – x and sin β = x
cos α = \(\sqrt{1-(1-x)^{2}}\) and cos β = \(\sqrt{1-x^{2}}\)
Now sin^-1(1 – x) – 2 sin^-1(x) = \(\frac{\pi}{2}\)
⇒ α – 2β = \(\frac{\pi}{2}\)
⇒ α = \(\frac{\pi}{2}\) + 2β
⇒ sin α = sin (\(\frac{\pi}{2}\) + 2β)
⇒ sin α = cos 2β
⇒ 1 – x = 1 – 2 sin2β
⇒ 1 – x = 1 – 2x²
⇒ 2x² – x = 0
⇒ x(2x – 1) = 0
⇒ x = 0 (or) x = \(\frac{1}{2}\)
But when x = \(\frac{1}{2}\)

Hence x = 0 is the only solution for the given equation.

Question 5.
Solve the following equations.
(i) \(\cot ^{-1}\left(\frac{1+x}{1-x}\right)=\frac{1}{2} \cot ^{-1}\left(\frac{1}{x}\right)\), x > 0 and x ≠ 1
Solution:

(ii) \(\tan \left[\cos ^{-1} \frac{1}{x}\right]=\sin \left[\cot ^{-1} \frac{1}{2}\right]\); x ≠ 0
Solution:
Let \(\cos ^{-1}\left(\frac{1}{x}\right)=\alpha, \cot ^{-1} \frac{1}{2}=\beta\)

(iii) \(\cos ^{-1} x+\sin ^{-1} \frac{x}{2}=\frac{\pi}{6}\)
Solution:

(iv) \(\cos ^{-1}(\sqrt{3} \cdot x)+\cos ^{-1} x=\frac{\pi}{2}\)
Solution:

(v) \(\sin \left[\sin ^{-1}\left(\frac{1}{5}\right)+\cos ^{-1} x\right]=1\)
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Equations Solutions Exercise 7(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Equations Solutions Exercise 7(a)

I.

Question 1.
Find the principal solutions of the angles in the equations
(i) 2 cos²θ = 1
Solution:
cos²θ = \(\frac{1}{2}\)
θ = 45°, 135°

(ii) √3 sec θ + 2 = 0
Solution:
sec θ = \(\frac{-2}{\sqrt{3}}\)
⇒ cos θ = \(\frac{-\sqrt{3}}{2}\)
⇒ θ = 150°

(iii) 3 tan²θ = 1
Solution:
tan²θ = \(\frac{1}{3}\)
θ = ±\(\frac{\pi}{6}\)

Question 2.
Solve the following equations.
(i) cos 2θ = \(\frac{\sqrt{5}+1}{4}\), θ ∈ [0, 2π]
Solution:
cos 2θ = \(\frac{\sqrt{5}+1}{4}\)
⇒ cos 2θ = cos 36° = cos (\(\frac{\pi}{5}\))
and \(\frac{\pi}{5}\) ∈ [0, 2π]
2θ = \(\frac{\pi}{5}\) ⇒ θ = \(\frac{\pi}{10}\) is the principal solution
and 2θ = 2nπ ± \(\frac{\pi}{5}\) where n ∈ Z is the general solution
⇒ θ = nπ ± \(\frac{\pi}{10}\)
The values of θ in [0, 2π] are \(\left\{\frac{\pi}{10}, \frac{9 \pi}{10}, \frac{11 \pi}{10}, \frac{19 \pi}{10}\right\}\)

(ii) tan²θ = 1, θ ∈ [-π, π]
Solution:
tan²θ = 1 ⇒ tan θ = ±1
tan θ = ±1 = tan (\(\pm \frac{\pi}{4}\))
The principal solutions are θ = \(\pm \frac{\pi}{4}\)
and the general solution is given by nπ ± \(\frac{\pi}{4}\), n ∈ Z
Put n = -1, 0, 1
\(\left\{\frac{-3 \pi}{4}, \frac{-\pi}{4}, \frac{\pi}{4}, \frac{3 \pi}{4}\right\}\) is the solution set for the given equation in [-π, π]

(iii) sin 3θ = \(\frac{\sqrt{3}}{2}\), θ ∈ [-π, π]
Solution:
sin 3θ = \(\frac{\sqrt{3}}{2}\) = sin \(\frac{\pi}{3}\)
and \(\frac{\pi}{3}\) ∈ [-π, π]
∴ 3θ = \(\frac{\pi}{3}\) is the principal solution
and 3θ = \(n \pi+(-1)^{n} \frac{\pi}{3}\), n ∈ Z
⇒ θ = \(\frac{n \pi}{3}+(-1)^{n} \cdot \frac{\pi}{9}\), n ∈ Z is the general solution.
The solution of θ in [-π, π] are \(\left\{\frac{-5 \pi}{9}, \frac{-4 \pi}{9}, \frac{\pi}{9}, \frac{2 \pi}{9}, \frac{7 \pi}{9}, \frac{8 \pi}{9}\right\}\)

(iv) cos²θ = \(\frac{3}{4}\), θ ∈ [0, π]
Solution:
cos²θ = \(\frac{3}{4}\)
⇒ cos θ = ±\(\frac{\sqrt{3}}{4}\)
The general solution is given by
θ = nπ ± \(\frac{\pi}{6}\), n ∈ Z
Put n = 0, 1
The solution set for the given equation in [0, π] is \(\left\{\frac{\pi}{6}, \frac{5 \pi}{6}\right\}\)

(v) 2 sin²θ = sin θ, θ ∈ (0, π)
Solution:
2 sin²θ – sin θ = 0
sin θ (2 sin θ – 1) = 0
sin θ = 0 or sin θ = \(\frac{1}{2}\)
since θ ∈ (0, π)
∴ The solution of θ = \(\left\{\frac{\pi}{6}+\frac{5 \pi}{6}\right\}\)

Question 3.
Find general solutions to the following equations.
(i) sin θ = \(\frac{\sqrt{3}}{2}\), cos θ = \(\frac{-1}{2}\)
Solution:
sin θ = \(\frac{\sqrt{3}}{2}\), cos θ = \(\frac{-1}{2}\)
∵ sin θ is +ve and cos θ is -ve
⇒ θ lies in II quadrant
sin θ = \(\frac{\sqrt{3}}{2}=\sin \left(\frac{2 \pi}{3}\right)\)
cos θ = \(\frac{-1}{2}=\cos \left(\frac{2 \pi}{3}\right)\)
⇒ θ = 2nπ ± \(\frac{2 \pi}{3}\), n ∈ Z is the general solution.

(ii) tan x = \(\frac{-1}{\sqrt{3}}\), sec x = \(\frac{2}{\sqrt{3}}\)
Solution:
∵ tan x = \(\frac{-1}{\sqrt{3}}\) and sec x = \(\frac{2}{\sqrt{3}}\)
⇒ x lies in IV quadrant
tan x = \(\frac{-1}{\sqrt{3}}=\tan \left(\frac{-\pi}{6}\right)\)
sec x = \(\frac{2}{\sqrt{3}}=\sec \left(\frac{-\pi}{6}\right)\)
∴ θ = 2nπ + \(\left(\frac{-\pi}{6}\right)\), n ∈ Z is the general solution.

(iii) cosec θ = -2, cot θ = -√3
Solution:
cosec θ = -2, cot θ = -√3
⇒ θ lies in IV quadrant
cosec θ = -2
⇒ sin θ = \(-\frac{1}{2}=\sin \left(-\frac{\pi}{6}\right)\)
cot θ = -√3
⇒ tan θ = \(-\frac{1}{\sqrt{3}}=\tan \left(-\frac{\pi}{6}\right)\)
∴ θ = 2nπ + \(\left(\frac{-\pi}{6}\right)\), n ∈ Z is the general solution.

Question 4.
(i) If sin (270° – x) = cos 292°, then find x in (0, 360°).
Solution:
sin (270° – x) = cos (292°)
⇒ -cos x = cos (180° + 112°)
⇒ -cos x = -cos 112°
⇒ cos x = cos 112°
⇒ x = 112° or x = 360° – 112° = 248°

(ii) If x < 90°and sin (x + 28°) = cos (3x – 78°), then find x.
Solution:
sin (x + 28°) = cos (3x – 78°)
= sin (90° – 3x + 78°)
= sin (168° – 3x)
x + 28° = 168° – 3x + 28° (180°) or
= 180° – (168° – 3x) + 2x (180°)
⇔ there exists n ∈ Z such that
4x = 140° + 2x (180°)
2x = 16° – 2x (180°)
⇔ there exists n ∈ z such that
x = 35° + x(90°) or x = 8° – x (180°)
Hence x = 8° and x = 35° are the only values of x that lie (0, 90°) and satisfy the given equation.

Question 5.
Find general solutions to the following equations.
(i) 2 sin²θ = 3 cos θ
Solution:
2 sin²θ = 3 cos θ
⇒ 2(1 – cos²θ) = 3 cos θ
⇒ 2 cos²θ + 3 cos θ – 2 = 0
⇒ 2 cos²θ + 4 cos θ – cos θ – 2 = 0
⇒ 2 cos θ (cos θ + 2) – 1 (cos θ + 2) = 0
⇒ (2 cos θ – 1) (cos θ + 2) = 0
⇒ cos θ = \(\frac{1}{2}\) (or) cos θ = -2
∴ The range of cos θ is [-1, 1]
cos θ = -2 is not admissible
∴ cos θ = \(\frac{1}{2}=\cos \frac{\pi}{3}\)
⇒ θ = \(\frac{\pi}{3}\) is the principal solution and
θ = 2nπ ± \(\frac{\pi}{3}\), n ∈ Z is the general solution.

(ii) sin²θ – cos θ = \(\frac{1}{4}\)
Solution:
sin²θ – cos θ = \(\frac{1}{4}\)
⇒ 4(1 – cos²θ) – 4 cos θ = 1
⇒ 4 cos²θ + 4 cos θ – 3 = 0
⇒ 4 cos²θ + 6 cos θ – 2 cos θ – 3 = 0
⇒ 2 cos θ (2 cos θ + 3) – (2 cos θ + 3) = 0
⇒ (2 cos θ – 1) (2 cos θ + 3) = 0
∴ cos θ = \(\frac{1}{2}\) (or) cos θ = \(\frac{-3}{2}\)
∵ The range of cos θ is [-1, 1]
cos θ = \(\frac{-3}{2}\) is not admisable
∴ cos θ = \(\frac{1}{2}=\cos \left(\frac{\pi}{3}\right)\)
θ = \(\frac{\pi}{3}\) is the principal solution and
θ = 2nπ ± \(\frac{\pi}{3}\), n ∈ Z is the general solution.

(iii) 5 cos²θ + 7 sin²θ = 6
Solution:
5 cos²θ + 7 sin²θ = 6
Dividing by cos²θ
⇒ 5 + 7 tan²θ = 6 sec²θ
⇒ 5 + 7 tan²θ = 6(1 + tan²θ)
⇒ tan²θ = 1
⇒ tan θ = ±1
∴ θ = nπ ± \(\frac{\pi}{4}\), n ∈ Z is the general solution.

(iv) 3 sin⁴x + cos⁴x = 1
Solution:
3 sin⁴x + cos⁴x = 1
⇒ 3 sin⁴x + (cos²x)² = 1
⇒ 3 sin⁴x + (1 – sin²x)² = 1
⇒ 3 sin⁴x + 1 + sin⁴x – 2 sin²x = 1
⇒ 4 sin⁴x – 2 sin²x = 0
⇒ 2 sin²x (2 sin²x – 1) = 0
⇒ sin x = 0 (or) sin x = ±\(\frac{1}{\sqrt{2}}\)
If sin x = 0
⇔ x = nπ, n ∈ Z is the general solution.
If sin x = ±\(\frac{1}{\sqrt{2}}\)
⇒ x = nπ ± \(\frac{\pi}{4}\), n ∈ Z is the general solution.
∴ General solution is x = nπ (or) nπ ± \(\frac{\pi}{4}\), n ∈ Z.

II.

Question 1.
Solve the following equations and write a general solution.
(i) 2 sin²θ – 4 = 5 cos θ
Solution:
2(1 – cos²θ) – 4 = 5 cos θ
2 – 2cos²θ – 4 = 5 cos θ
2 cos²θ + 5 cos θ + 2 = 0
2 cos²θ + 4 cos θ + cos θ + 2 = 0
2 cos θ (cos θ + 2) + 1 (cos θ + 2) = 0
(cos θ + 2) (2 cos θ + 1) = 0
cos θ = -2 or cos θ = \(-\frac{1}{2}\)
cos θ = -2 is not possible
∴ cos θ = \(-\frac{1}{2}\) = cos \(\frac{2 \pi}{3}\)
∴ General solution is θ = 2nπ ± \(\frac{2 \pi}{3}\), n ∈ Z

(ii) 2 + √3 sec x – 4 cos x = 2√3
Solution:
2 + √3 sec x – 4 cos x = 2√3
\(\frac{2 \cos x+\sqrt{3}-4 \cos ^{2} x}{\cos x}\) = 2√3
2 cos x + √3 – 4 cos²x = 2√3 cos x
4 cos²x + 2√3 cos x – 2 cos x – √3 = 0
2 cos x (2 cos x + √3) – 1 (2 cos x + √3) = 0
(2 cos x – 1) (2 cos x + √3) = 0
cos x = \(\frac{1}{2}\) (or) cos x = \(\frac{-\sqrt{3}}{2}\)
If cos x = \(\frac{1}{2}\) = cos \(\frac{\pi}{3}\), n ∈ Z
General solution x = 2nπ ± \(\frac{\pi}{3}\)
If cos x = \(\frac{-\sqrt{3}}{2}\) = cos \(\frac{5 \pi}{3}\)
General solution x = 2nπ ± \(\frac{5 \pi}{3}\), n ∈ Z

(iii) 2 cos²θ + 11 sin θ = 7
Solution:
2 (1 – sin²θ) + 11 sin θ = 7
2 – 2 sin²θ + 11 sin θ = 7
2 sin²θ – 11 sin θ + 5 = 0
2 sin²θ – 10 sin θ – sin θ + 5 = 0
2 sin θ (sin θ – 5) – 1(sin θ – 5) = 0
(sin θ – 5) (2 sin θ – 1) = 0
sin θ = 5 or sin θ = \(\frac{1}{2}\)
If sin θ = 5 is not possible
∴ sin θ = \(\frac{1}{2}\) = sin \(\frac{\pi}{6}\)
General solultion θ = nπ + (-1)ⁿ \(\frac{\pi}{6}\), n ∈ Z

(iv) 6 tan²x – 2 cos²x = cos 2x
Solution:
6 tan²x – 2 cos²x = cos 2x
⇒ 6(sec²x – 1) – 2 cos²x = 2 cos²x – 1
⇒ 6 sec²x – 6 – 4 cos²x + 1 = 0
⇒ 6 sec²x – 4 cos²x – 5 = 0
⇒ \(\frac{6}{\cos ^{2} x}\) – 4 cos²x – 5 = 0
⇒ 6 – 4 cos⁴x – 5 cos²x = 0
⇒ 4 cos⁴x + 5 cos²x – 6 = 0
⇒ 4 cos⁴x + 8 cos²x – 3 cos²x – 6 = 0
⇒ 4 cos²x (cos²x + 2) – 3(cos²x + 2) = 0
⇒ (4 cos²x – 3) (cos²x + 2) = 0
⇒ 4 cos²x = 3, cos²x ≠ -2
⇒ cos x = ±\(\frac{\sqrt{3}}{2}\)
∴ x = nπ ± \(\frac{\pi}{6}\), n ∈ Z is the general solution.

(v) 4 cos²θ + √3 = 2(√3 + 1) cos θ
Solution:
4 cos²θ – 2(√3 + 1) cos θ + √3 = 0
⇒ 4 cos²θ – 2√3 cos θ – 2 cos θ + √3 = 0
⇒ 2 cos θ (2 cos θ – √3) – 1(2 cos θ – √3) = 0
⇒ (2 cos θ – 1) (2 cos θ – √3) = 0
⇒ cos θ = \(\frac{1}{2}\) (or) cos θ = \(\frac{\sqrt{3}}{2}\)
If cos θ = \(\frac{1}{2}\) = cos \(\left(\frac{\pi}{3}\right)\)
∴ θ = 2nπ ± \(\frac{\pi}{3}\), n ∈ Z is the general solution.
If cos θ = \(\frac{\sqrt{3}}{2}\) = cos \(\left(\frac{\pi}{6}\right)\)
∴ θ = 2nπ ± \(\frac{\pi}{6}\), n ∈ Z is the general solution.

(vi) 1 + sin 2x – (sin 3x – cos 3x)²
Solution:
1 + sin 2x = (sin 3x – cos 3x)²
⇒ 1 + sin 2x = sin²3x + cos²3x – 2 sin 3x cos 3x
⇒ 1 + sin 2x = 1 – sin (2 × 3x)
⇒ sin 6x + sin 2x = 0
⇒ \(2 \sin \left(\frac{6 x+2 x}{2}\right) \cdot \cos \left(\frac{6 x-2 x}{2}\right)=0\)
⇒ sin (4x) . cos (2x) = 0
⇒ cos 2x = 0 (or) sin 4x = 0
If cos 2x = 0 = cos \(\frac{\pi}{2}\)
⇒ 2x = \(\frac{\pi}{2}\) is the principal solution and
2x = (2n + 1) \(\frac{\pi}{2}\), n ∈ Z is the general solution,
so that x = \(\frac{n \pi}{2}+\frac{\pi}{4}\), n ∈ Z
If sin 4x = 0 = sin(nπ), n ∈ Z
4x = nπ, n ∈ Z is the general solution.
So that 4x = nπ
⇒ x = \(\frac{n \pi}{4}\), n ∈ Z
∴ x = \(\frac{n \pi}{4}\); \(\frac{n \pi}{2}+\frac{\pi}{4}\), n ∈ Z is the general solution.

(vii) 2 sin²x + sin²2x = 2
Solution:
2 sin²x + sin²(2x) = 2
⇒ 2 sin2x + (2 sin x cos x)2 – 2 = 0
⇒ sin²x + 2 sin²x cos²x – 1 = 0
⇒ 2 sin²x cos²x – (1 – sin²x) = 0
⇒ 2 sin²x cos²x – cos²x = 0
⇒ cos²x (2 sin²x – 1) = 0
⇒ cos x = 0 (or) sin x = ±\(\frac{1}{\sqrt{2}}\)
If cos x = 0 = cos \(\frac{\pi}{2}\)
⇒ x = \(\frac{\pi}{2}\) is the principal solution
and cos x = 0 ⇔ \(\sin \left(x-\frac{\pi}{2}\right)=0\)
⇔ x – \(\frac{\pi}{2}\) = nπ, n ∈ Z
⇔ x = nπ + \(\frac{\pi}{2}\)
⇔ x = (2n + 1) \(\frac{\pi}{2}\), n ∈ Z
∴ x = (2n + 1) \(\frac{\pi}{2}\), n ∈ Z is the general solution of cos x = 0
If sin x = ±\(\frac{1}{\sqrt{2}}\) = sin(±\(\frac{\pi}{4}\))
x = ±\(\frac{\pi}{4}\) are principal solutions
and x = nπ ± \(\frac{\pi}{4}\), n ∈ Z is the general solution
∴ The general solutions are \(\left[\left\{(2 n+1) \frac{\pi}{2}\right\},\left\{n \pi \pm \frac{\pi}{4}\right\} n \in Z\right]\)

Question 2.
Solve the following equations.
(i) √3 sin θ – cos θ = √2
Solution:

(ii) cot x + cosec x = √3
Solution:

(iii) sin x + √3 cos x = √2
Solution:

Question 3.
Solve the following equations:
(i) tan θ + sec θ = √3, 0 ≤ θ ≤ 2π
Solution:

(ii) cos 3x + cos 2x = sin \(\frac{3 x}{2}\) + sin \(\frac{x}{2}\), 0 ≤ x ≤ 2π
Solution:

(iii) cot²x – (√3 + 1) cot x + √3 = 0, 0 < x < \(\frac{\pi}{2}\)
Solution:
cot²x – (√3 + 1) cot x + √3 = 0
⇔ cot²x – √3 cot x – cot x + √3 = 0
⇔ cot x (cot x – √3) – 1(cot x – √3) = 0
⇔ cot x = √3 (or) cot x = 1
case (i): cot x = 1 ⇒ tan x = 1
∴ x = \(\left\{\frac{\pi}{4}\right\}\)
case (ii): cot x = √3 ⇒ tan x = \(\frac{1}{\sqrt{3}}\)
∴ x = \(\left\{\frac{\pi}{6}\right\}\)
∴ Solutions are \(\left\{\frac{\pi}{6}, \frac{\pi}{4}\right\}\)

(iv) sec x . cos 5x + 1 = 0; 0 < x < 2π
Solution:

III.

Question 1.
(i) Solve sin x + sin 2x + sin 3x = cos x + cos 2x + cos 3x
Solution:
(sin 3x + sin x) + sin 2x = (cos 3x + cos x) + cos 2x
⇔ 2 . sin(\(\frac{3 x+x}{2}\)) . cos (\(\frac{3 x-x}{2}\)) + sin 2x = 2 . cos (\(\frac{3 x+x}{2}\)) . cos (\(\frac{3 x-x}{2}\)) + cos 2x
⇔ 2 sin 2x cos x + sin 2x = 2 cos 2x cos x + cos 2x
⇔ sin 2x (2 cos x + 1) = cos 2x (2 cos x + 1)
⇔ (2 cos x + 1) (sin 2x – cos 2x ) = 0
⇔ cos x = \(-\frac{1}{2}\) (or) sin 2x = cos 2x (i.e.,) tan (2x) = 1
Case (i):
cos x = \(-\frac{1}{2}\) = cos (\(\frac{2 \pi}{3}\))
Principal solution is x = \(\frac{2 \pi}{3}\)
and General solution is x = 2nπ ± \(\frac{2 \pi}{3}\), n ∈ z
Case (ii):
tan 2x = 1 = tan \(\frac{\pi}{4}\)
∴ Principal solution is 2x = \(\frac{\pi}{4}\) (i.e.,) x = \(\frac{\pi}{2}\)
General solution is 2x = nπ + \(\frac{\pi}{4}\), n ∈ z
⇒ x = \(\frac{\mathrm{n} \pi}{2}+\frac{\pi}{8}\), n ∈ Z
∴ General solution is \(\left.\left[\left\{2 n \pi \pm \frac{2 \pi}{3}\right\},\left\{\frac{n \pi}{2}+\frac{\pi}{8}\right\} / n \in Z\right\}\right]\)

(ii) If x + y = \(\frac{2 \pi}{3}\) and sin x + sin y = \(\frac{3}{2}\), find x and y.
Solution:

(iii) If sin 3x + sin x + 2 cos x = sin 2x + 2 cos²x, find the general solution.
Solution:
Given sin 3x + sin x + 2 cos x = sin 2x + 2 cos²x
⇒ 2 . sin (\(\frac{3 x+x}{2}\)) . cos (\(\frac{3 x-x}{2}\)) + 2 cos x = 2 sin x cos x + 2 cos²x
⇒ 2 . sin 2x . cos x + 2 cos x = 2 cos x (sin x + cos x)
⇒ 2 cos x (sin 2x + 1) = 2 cos x (sin x + cos x)
⇒ 2 cos x [sin 2x + 1 – sin x – cos x] = 0
⇒ cos x = 0 (or) sin 2x – sin x + 1 – cos x = 0

(iv) Solve cos 3x – cos 4x = cos 5x – cos 6x
Solution:
-2 sin 5x . sin x = -2 sin 4x . sin x
⇒ 2 sin x [sin 5x – sin 4x] = 0
⇒ 4 sin x . cos \(\frac{9 x}{2}\) . sin \(\frac{x}{2}\) = 0

Question 2.
Solve the following equations.
(i) cos 2θ + cos 8θ = cos 5θ
Solution:
cos 2θ + cos 8θ = cos 5θ
2 cos (\(\frac{2 \theta+8 \theta}{2}\)) cos (\(\frac{2 \theta-8 \theta}{2}\)) – cos 5θ = 0
2 cos 5θ . cos 3θ – cos 5θ = 0
cos 5θ (2 cos 3θ – 1) = 0
If cos 5θ = 0
Solution is 5θ = (2n + 1) \(\frac{\pi}{2}\)
θ = (2n + 1) \(\frac{\pi}{10}\), n ∈ z
If 2 cos 3θ – 1 = 0
cos 3θ = \(\frac{1}{2}\) = cos \(\frac{\pi}{3}\)
Solution is 3θ = 2nπ ± \(\frac{\pi}{3}\)
θ = \(\frac{2 n \pi}{3} \pm \frac{\pi}{9}\), n ∈ Z

(ii) cos θ – cos 7θ = sin 4θ
Solution:
-2 sin (\(\frac{\theta+7 \theta}{2}\)) sin (\(\frac{\theta-7 \theta}{2}\)) – sin 4θ = 0
2 sin 4θ sin 3θ – sin 4θ = 0
sin 4θ (2 sin 3θ – 1) = 0
If sin 4θ = 0
∴ Solution is 4θ = nπ
θ = \(\frac{n \pi}{4}\), n ∈ z
If 2 sin 3θ – 1 = 0
sin 3θ = \(\frac{1}{2}\) = sin \(\frac{\pi}{6}\)
Solution is 3θ = nπ + (-1)ⁿ \(\frac{\pi}{6}\)
θ = \(\frac{n \pi}{3}+(-1)^{n} \frac{\pi}{18}\), n ∈ z

(iii) sin θ + sin 5θ = sin 3θ, 0 < θ < π
Solution:
sin θ + sin 5θ = sin 3θ
sin θ + sin 5θ – sin 3θ = 0
sin θ + 2 cos (\(\frac{5 \theta+3 \theta}{2}\)) sin (\(\frac{5 \theta-3 \theta}{2}\)) = 0
sin θ + 2 cos 4θ . sin θ = 0
sin θ (1 + 2 cos 4θ) = 0
sin θ = 0, cos 4θ = \(\frac{-1}{2}\)
If sin θ = 0, solution is θ = nπ, n ∈ Z
If cos 4θ = \(\frac{-1}{2}\) = cos(\(\frac{2 \pi}{3}\))
Solution is 4θ = 2nπ ± \(\frac{2 \pi}{3}\)
θ = \(\frac{2 n \pi}{4} \pm \frac{2 \pi}{12}\), n ∈ Z
θ = \(\frac{n \pi}{2} \pm \frac{\pi}{6}\), n ∈ Z
Since 0 < θ < π
Then π = \(\frac{\pi}{6}, \frac{\pi}{3}, \frac{2 \pi}{3}, \frac{5 \pi}{6}\)

Question 3.
(i) If tan pθ = cot qθ and p ≠ -q show that the solutions are in A.P. with common difference \(\frac{\pi}{p+q}\)
Solution:
tan pθ = cot qθ = tan \(\frac{\pi}{2}\) – qθ
pθ = nπ + \(\frac{\pi}{2}\) – qθ
(p + q) θ = (2n + 1) \(\frac{\pi}{2}\)
θ = \(\frac{(2 n+1)}{p+q} \frac{\pi}{2}\), n is an integer
The Solutions \(\frac{\pi}{2(p+q)}, \frac{3 \pi}{2(p+q)}, \frac{5 \pi}{2(p+q)}\) + …………
∴ The solution form an Arithematical proportion With common difference \(\frac{2 \pi}{2(p+q)}=\frac{\pi}{p+q}\)

(ii) Show that the solutions of cos pθ = sin qθ form two series each of which is an A.P. Find also the common difference of each A.P. (p ≠ ±q).
Solution:
cos pθ = sin qθ
cos pθ – sin qθ = 0
cos pθ + \(\cos \left[\frac{\pi}{2}+9 \theta\right]\) = 0

(iii) Find the number of solutions of the equation tan x + sec x = 2 cos x; cos x ≠ 0, lying in the interival (0, π).
Solution:
tan x + sec x = 2 cos x
\(\frac{\sin x}{\cos x}+\frac{1}{\cos x}\) = 2 cos x
sin x + 1 = 2 cos²x
sin x + 1 = 2(1 – sin²x)
sin x + 1 = (2 – 2 sin²x)
2 sin²x + sin x – 1 = 0
2 sin²x + 2 sin x – sin x – 1 = 0
2 sin x (sin x + 1) – 1 (sin x + 1) = 0
(sin x + 1)(2 sin x – 1) = 0
sin x = -1 (or) sin x = \(\frac{1}{2}\)
If sin x = -1
x = \(\frac{-\pi}{2} \text { (or) } \frac{3 \pi}{2}\)
If sin x = \(\frac{1}{2}\)
x = \(\frac{\pi}{6} \text { (or) } \frac{5 \pi}{6}\)
In the interval (0, π)
Number of solutions = 2

(iv) Solve sin 3α = 4 sin α sin(x + α) sin(x – α) where α ≠ nπ, n ∈ Z
Solution:
3 sin α – 4 sin³α = 4 sin α (sin²x – sin²α)
Dividing with sin α
3 – 4 sin²α = 4 (sin²x – sin²α)
3 – 4 sin²α = 4 sin²x – 4 sin²α
4 sin²x = 3
2 sin²x = \(\frac{3}{2}\)
1 – cos 2x = \(\frac{3}{2}\)
cos 2x = \(-\frac{1}{2}=\cos \frac{2 \pi}{3}\)
2x = 2nπ ± \(\frac{2 \pi}{3}\), ∀ n ∈ Z
x = nπ ± \(\frac{\pi}{3}\), n ∈ Z

Question 4.
(i) If tan(π cos θ) = cot(π sin θ), then prove that \(\cos \left(\theta-\frac{\pi}{4}\right)=\pm \frac{1}{2 \sqrt{2}}\)
Solution:

(ii) Find the range of θ if cos θ + sin θ is positive.
Solution:

Question 5.
If α, β are the solutions of the equation a cos θ + b sin θ = c, where a, b, c ∈ R and if a² + b² > 0, cos α ≠ cos β and sin α ≠ sin β, then show that
(i) sin α + sin β = \(\frac{2 b c}{a^{2}+b^{2}}\)
(ii) cos α + cos β = \(\frac{2 a c}{a^{2}+b^{2}}\)
(iii) cos α . cos β = \(\frac{c^{2}-b^{2}}{a^{2}+b^{2}}\)
(iv) sin α . sin β = \(\frac{c^{2}-a^{2}}{a^{2}+b^{2}}\)
Solution:
a cos θ = b sin θ = c
First write this as a quadratic equation in sin θ
⇔ a cos θ = c – b sin θ
By squaring on both sides, we get
a² cos²θ = (c – b sin θ)²
⇔ a² (1 – sin²θ) = c² + b² sin²θ – 2 bc sin θ
⇔ (a² + b²) sin²θ – 2bc sin θ + (c² – a²) = 0
It is a quadratic equation in sin θ,
It has sin α and sin β as roots since α and β are solutions for the given equation
(i) Sum of the roots = sin α + sin β = \(\frac{2 b c}{a^{2}+b^{2}}\)
Again a cos θ + b sin θ = c
Write this as a quadratic equation in cos θ
⇔ b sin θ = c – a cos θ
By squaring on both sides
⇔ b² sin²θ = (c – a cos θ)²
⇔ b²(1 – cos²θ) = c² + a² cos²θ – 2 ca cos θ
⇔ (a² + b²) cos²θ – 2 ca cos θ + (c² – b²) = 0
It is a quadratic equation in cos θ. It has cos α, cos β be its roots.
(ii) Sum of the roots = cos α + cos β = \(\frac{2 c a}{a^{2}+b^{2}}\)
(iii) Product of the roots = cos α . cos β = \(\frac{c^{2}-b^{2}}{a^{2}+b^{2}}\)
(iv) Product of the roots = sin α . sin β = \(\frac{c^{2}-a^{2}}{a^{2}+b^{2}}\)

Question 6.
(i) Find the common roots of the equations cos 2x + sin 2x = cot x and 2 cos²x + cos²2x = 1.
Solution:
Let tan x = A
Given that cos 2x + sin 2x = cot x
⇔ \(\frac{1-\tan ^{2} x}{1+\tan ^{2} x}+\frac{2 \tan x}{1+\tan ^{2} x}=\frac{1}{\tan x}\)
⇔ \(\frac{1-A^{2}}{1+A^{2}}+\frac{2 A}{1+A^{2}}=\frac{1}{A}\)
⇔ (1 – A² + 2A) A = (1 + A2)
⇔ A – A³ + 2A² = 1 + A²
⇔ A³ – A² – A + 1 = 0
⇔ A = 1
A = 1 satisfy this equation

∴ A³ – A² – A + 1 = 0
⇔ (A – 1) (A² – 1) = 0
⇔ (A – 1) (A – 1) (A + 1) = 0
⇔ A = 1, A = -1
∴ tan x = ±1
⇒ x = (2n + 1) \(\frac{\pi}{4}\), n ∈ z
Given 2 cos²x + cos² 2x = 1
⇔ (2 cos²x – 1) + cos²(2x) = 0
⇔ cos 2x + cos²(2x) = 0
⇔ cos 2x (1 + cos 2x) = 0
⇔ cos 2x = 0 (or) cos 2x = -1
Case (i): cos 2x = 0
⇔ 2x = (2n + 1) \(\frac{\pi}{2}\)
∴ x = (2n + 1) \(\frac{\pi}{4}\), n ∈ z
∴ (2n + 1) \(\frac{\pi}{4}\), n ∈ z is the common root for the given two trigonometric equations.

(ii) Solve the equation \(\sqrt{6-\cos x+7 \sin ^{2} x}+\cos x=0\)
Solution:
\(\sqrt{6-\cos x+7 \sin ^{2} x}+\cos x=0\)
6 – cos x + 7 sin²x ≥ 0
⇒ 7(1 – cos²x) – cos x + 6 ≥ 0
⇒ 7 – 7 cos²x – cos x + 6 ≥ 0
⇒ 7 cos²x + cos x – 13 ≤ 0
Consider 7 cos²x + cos x – 13 = 0

cos x values do not lie in [-1, 1]
Hence the given equation has no solution.

(iii) If |tan x| = tan x + \(\frac{1}{\cos x}\) and x ∈ [0, 2π], find the value of x.
Solution:
Case (i):
|tan x| = tan x, if x lies either in I (or) in III quadrant
Then |tan x| = tan x + \(\frac{1}{\cos x}\)
⇒ tan x = tan x + sin x
⇒ sec x = 0 which is impossible, since sec x ∉ (-1, 1)
Case (ii):
|tan x| = -tan x, if x lies in II & IV quadrants
Then |tan x| = tan x + \(\frac{1}{\cos x}\)
⇒ -tan x = tan x + sec x
⇒ -2 tan x = sec x
⇒ \(-2 \frac{\sin x}{\cos x}-\frac{1}{\cos x}\) = 0
⇒ -2 sin x – 1 = 0
⇒ sin x = \(\frac{-1}{2}=\sin \left(\frac{-\pi}{6}\right)=\sin \left(2 \pi-\frac{\pi}{6}\right)\)
∴ x = \(\frac{11 \pi}{6}\)

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(f) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(f)

Question 1.
If A, B, C are angles in a triangle, then prove that
(i) sin 2A – sin 2B + sin 2C = 4 cos A sin B cos C
Solution:
∵ A, B, C are angles in a triangle
⇒ A + B + C = 180° ……….(1)
LHS = sin 2A – sin 2B + sin 2C
= sin 2A + sin 2C – sin 2B
= 2 sin (\(\frac{2 A+2 C}{2}\)) . cos(\(\frac{2 A-2 C}{2}\)) – sin 2B
= 2 sin (A + C) cos (A – C) – sin B
= 2 sin (180° – B) cos (A – C) – 2 sin B cos B
= 2 sin B cos (A – C) – 2 sin B cos B
= 2 sin B [cos (A – C) – cos B]
= 2 sin B [cos (A – C) – cos (180° – (A + C)]
= 2 sin B [cos (A – C) + cos (A + C)]
= 2 sin B (2 cos A cos C)
= 4 cos A sin B cos C
∴ sin 2A – sin 2B + sin 2C = 4 cos A sin B cos C

(ii) cos 2A – cos 2B + cos 2C = 1 – 4 sin A cos B Sin C
Solution:
L.H.S. = -(cos 2B – cos 2A) + cos 2C
= -2 sin (A + B) sin (A – B) + cos 2C
= -2 sin (180° – C) sin (A – B) + cos 2C
= -2 sin C sin (A – B) + 1 – 2 sin2C
= 1 – 2 sin C (sin (A – B) + sin C)
= 1 – 2 sin C sin (A – B) + sin (180° – \(\overline{\mathrm{A}+\mathrm{B}}\))
= 1 – 2 sin C (sin (A – B) + sin (A + B))
= 1 – 2 sin C (2 sin A cos B)
= 1 – 4 sin A cos B sin C
= R.H.S.

Question 2.
If A, B, C are angles in a triangle, then prove that
(i) sin A + sin B – sin C = 4 sin \(\frac{A}{2}\) sin \(\frac{B}{2}\) cos \(\frac{C}{2}\)
Solution:
L.H.S. = (sin A + sin B) – sin C
= 2 sin (\(\frac{A+B}{2}\)) cos (\(\frac{A-B}{2}\)) – sin C

(ii) cos A + cos B – cos C = -1 + 4 cos \(\frac{A}{2}\) cos \(\frac{B}{2}\) sin \(\frac{C}{2}\)
Solution:
A, B, C are angles in a triangle
A + B + C = 180° ………(1)
LHS = cos A + cos B – cos C

Question 3.
If A, B, C are angles in a triangle, then prove that
(i) sin²A + sin²B – sin²C = 2 sin A sin B cos C
Solution:
Given A + B + C = 180°
L.H.S. = sin²A + [sin²B – sin²C]
= sin²A + sin (B + C) sin (B – C)
= sin²A + sin (180° – A) . sin (B – C)
= sin²A + sin A . sin (B – C)
= sin A (sin A + sin (B – C))
= sin A [sin (180° – \(\overline{\mathrm{B}+\mathrm{C}}\)) + sin (B – C)]
= sin A [sin (B + C) + sin (B – C)]
= sin A [2 sin B cos C]
= 2 sin A sin B cos C
= R.H.S

(ii) cos²A + cos²B – cos²C = 1 – 2 sin A sin B cos C
Solution:
A, B, C are angles in a triangle
⇒ A + B + C = 180° ……..(1)
L.H.S = cos²A + cos²B – cos²C
= cos²A + cos²B – cos²C

= 1 + cos (A + B) cos (A – B) – cos²C
= 1 + cos (180° – C) cos (A – B) – cos²C [By (1)]
= 1 – cos C cos (A – B) – cos²C
= 1 – cos C [cos (A – B) + cos C]
= 1 – cos C [cos (A – B) + cos(180° – \(\overline{A+B}\)] [By eq. (1)]
= 1 – cos C [cos (A – B) – cos (A + B)]
= 1 – cos C [2 sin A sin B]
= 1 – 2 sin A sin B cos C
∴ cos²A + cos²B – cos²C = 1 – 2 sin A sin B cos C

Question 4.
If A + B + C = π, then prove that
(i) \(\cos ^{2} \frac{A}{2}+\cos ^{2} \frac{B}{2}+\cos ^{2} \frac{C}{2}\) = \(2\left[1+\sin \frac{A}{2}+\sin \frac{B}{2} \sin \frac{C}{2}\right]\)
Solution:

(ii) \(\cos ^{2} \frac{A}{2}+\cos ^{2} \frac{B}{2}-\cos ^{2} \frac{C}{2}=2 \cos \frac{A}{2}\) \(\cos \frac{B}{2} \sin \frac{C}{2}\)
Solution:

Question 5.
In triangle ABC, prove that
(i) \(\cos \frac{A}{2}+\cos \frac{B}{2}+\cos \frac{C}{2}\) = \(4 \cos \frac{\pi-A}{4} \cos \frac{\pi-B}{4} \cos \frac{\pi-C}{4}\)
Solution:

(ii) \(\cos \frac{A}{2}+\cos \frac{B}{2}-\cos \frac{C}{2}\) = \(4 \cos \frac{\pi+A}{4} \cdot \cos \frac{\pi+B}{4} \cos \frac{\pi-C}{4}\)
Solution:

(iii) \(\sin \frac{A}{2}+\sin \frac{B}{2}-\sin \frac{C}{2}\) = \(-1+4 \cos \frac{\pi-A}{4} \cos \frac{\pi-B}{4} \sin \frac{\pi-C}{4}\)
Solution:

Question 6.
If A + B + C = π/2, then prove that cos 2A + cos 2B + cos 2C = 1 + 4 sin A sin B sin C
Solution:
A + B + C = π/2 ………(1)
LHS = cos 2A + cos 2B + cos 2C
= 2 cos (\(\frac{2 \mathrm{~A}+2 \mathrm{~B}}{2}\)) cos (\(\frac{2 \mathrm{~A}-2 \mathrm{~B}}{2}\)) + cos 2C
= 2 cos (A + B) . cos (A – B) + cos 2C
= 2 cos (90° – C) cos (A – B) + cos 2C
= 2 sin C cos (A – B) + (1 – 2 sin²C)
= 1 + 2 sin C [cos (A – B) – sin C]
= 1 + 2 sin C [cos (A – B)- sin (90° – \(\overline{A+B}\))]
= 1 + 2 sin C [cos (A – B) – cos (A +B)]
= 1 + 2 sin C [2 sin A sin B]
= 1 + 4 sin A sin B sin C
= RHS
∴ cos 2A + cos 2B + cos 2C = 1 + 4 sin A sin B sin C

Question 7.
If A + B + C = 3π/2, then prove that
(i) cos²A + cos²B – cos²C = -2 cos A cos B sin C
Solution:
A + B + C = 3π/2 ……..(1)
L.H.S. = cos²A + cos²B – cos²C
= cos²A + (1 – sin²B) – cos²C
= (cos²A – sin²B) + (1 – cos²C)
= cos (A + B) cos (A – B) + sin²C
= cos (270° – C) cos(A – B) + sin²C
= -sin C cos (A – B) + sin²C
= sin C [sin C – cos (A – B)]
= sin C [sin (270°- \(\overline{A+B}\)) – cos (A – B)]
= sin C [-cos (A + B) – cos (A – B)]
= -sin C [cos (A + B) + cos (A – B)]
= -sin C [2 cos A cos B]
= -2 cos A cos B sin C
= RHS
∴ cos²A + cos²B – cos²C = -2 cos A cos B sin C

(ii) sin 2A + sin 2B – sin 2C = -4 sin A sin B cos C
Solution:
Here A + B + C = 270° ………(1)
LHS = sin 2A + sin 2B – sin 2C
= 2 sin (\(\frac{2 A+2 B}{2}\)) cos (\(\frac{2 A-2 B}{2}\)) – sin 2C
= 2 sin (A + B) . cos (A – B) – 2 sin C cos 2C
= 2 sin (270° – C) cos (A – B) – 2 sin C cos C
= -2 cos C cos (A – B) – 2 sin C cos C
= -2 cos C [cos (A – B) + sin C]
= -2 cos C [cos (A – B) + sin (270° – \(\overline{A+B}\))]
= -2 cos C [cos (A – B) – cos (A + B)]
= -2 cos C [2 sin A sin B]
= -4 sin A sin B cos C
= RHS
∴ sin 2A + sin 2B – sin 2C = -4 sin A sin B cos C

Question 8.
If A + B + C = 0°, then prove that
(i) sin 2A + sin 2B + sin 2C = -4 sin A sin B sin C
Solution:
Here A + B + C = 0 ………(1)
LHS = sin 2A + sin 2B + sin 2C
= 2 sin (\(\frac{2 A+2 B}{2}\)) cos (\(\frac{2 A-2 B}{2}\)) + sin 2C
= 2 sin (A + B) . cos (A – B) + 2 sin C cos 2C
= 2 sin (-C) cos (A – B) + 2 sin C cos C
= -2 sin C cos (A – B) + 2 sin C cos C
= -2 sin C [cos (A – B) – cos C]
= -2 sin C [cos (A – B) – cos (-A – B)]
= -2 sin C [cos (A – B) – cos (A + B)]
= -2 sin C [2 sin A sin B]
= -4 sin A sin B sin C
= RHS
∴ sin 2A + sin 2B + sin 2C = -4 sin A sin B sin C

(ii) sin A + sin B – sin C = – 4 cos \(\frac{A}{2}\) cos \(\frac{B}{2}\) sin \(\frac{C}{2}\)
Solution:

Question 9.
If A + B + C + D = 2π then prove that
(i) sin A – sin B + sin C – sin D = \(-4 \cos \left(\frac{A+B}{2}\right) \sin \left(\frac{A+C}{2}\right) \cos \left(\frac{A+D}{2}\right)\)
Solution:

(ii) cos 2A + cos 2B + cos 2C + cos 2D = 4 cos (A + B) cos (A + C) cos (A + D)
Solution:

Question 10.
If A + B + C = 2S, then prove that
(i) sin (S – A) + sin (S – B) + sin C = \(4 \cos \left(\frac{S-A}{2}\right) \cos \left(\frac{S-B}{2}\right) \sin \frac{C}{2}\)
Solution:

(ii) cos (S – A) + cos (S – B) + cos C = \(-1+4 \cos \left(\frac{S-A}{2}\right) \cos \left(\frac{S-B}{2}\right) \cdot \cos \frac{C}{2}\)
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(e) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(e)

I.

Question 1.
Prove that sin 50° – sin70° + sin 10° = 0
Solution:
LHS = sin 50° – sin 70° + sin 10°
= 2 cos(\(\frac{50^{\circ}+70^{\circ}}{2}\)) . sin(\(\frac{50^{\circ}-70^{\circ}}{2}\)) + sin 10°
= 2 cos 60° . sin (-10°) + sin 10°
= 2(\(\frac{1}{2}\)) (-sin 10°) + sin 10°
= -sin 10° + sin 10°
= 0
= RHS
∴ sin 50° – sin 70° + sin 10° = 0

Question 2.
Prove that \(\frac{\sin 70^{\circ}-\cos 40^{\circ}}{\cos 50^{\circ}-\sin 20^{\circ}}=\frac{1}{\sqrt{3}}\)
Solution:

Question 3.
Prove that cos 55° +cos 65° + cos 175° = 0
Solution:
LHS = cos 55° + cos 65° + cos 175°
= cos 65° + cos 55° + cos (180° – 5°)
= 2 cos(\(\frac{65^{\circ}+55^{\circ}}{2}\)) . cos(\(\frac{65^{\circ}-55^{\circ}}{2}\)) – cos 5°
= 2 cos (60°) . cos (5°) – cos 5°
= 2(\(\frac{1}{2}\)) cos 5° – cos 5°
= cos 5° – cos 5°
= 0
= RHS
∴ cos 55° + cos 65° + cos 175° = 0

Question 4.
Prove that 4(cos 66° + sin 84°) = √3 + √15
Solution:

Question 5.
Prove that cos 20° cos 40° – sin 5° sin 25° = \(\frac{\sqrt{3}+1}{4}\)
Solution:
cos 20° cos40° – sin 5° sin 25°
= \(\frac{1}{2}\) [2 cos 20° cos 40° – 2 sin 5° sin 25°]
= \(\frac{1}{2}\) [cos (20° + 40°) + cos (20° – 40°) – {cos (5° – 25°) – cos (5° + 25°)}]
= \(\frac{1}{2}\) [cos 60° + cos 20° – cos 20° + cos 30°]
= \(\frac{1}{2}\left[\frac{1}{2}+\frac{\sqrt{3}}{2}\right]\)
= \(\frac{\sqrt{3}+1}{4}\)
= RHS
∴ cos 20° cos 40° – sin 5° sin 25° = \(\frac{\sqrt{3}+1}{4}\)

Question 6.
Prove that cos 48° . cos 12° = \(\frac{3+\sqrt{5}}{8}\)
Solution:
LHS = cos 48° . cos 12°
= \(\frac{1}{2}\) (2 cos 48°. cos 12°)
= \(\frac{1}{2}\) [cos (48° + 12°) + cos (48° – 12°)]
= \(\frac{1}{2}\) [cos 60° + cos 36°]
= \(\frac{1}{2}\left[\frac{1}{2}+\frac{\sqrt{5}+1}{4}\right]\)
= \(\frac{1}{2}\left[\frac{2+\sqrt{5}+1}{4}\right]\)
= \(\frac{3+\sqrt{5}}{8}\)
= RHS
∴ cos 48° . cos 12° = \(\frac{3+\sqrt{5}}{8}\)

II.

Question 1.
Prove that cos θ + cos[\(\frac{2 \pi}{3}\) + θ] + cos[\(\frac{4 \pi}{3}\) + θ] = 0
Solution:

Question 2.
Prove that sin²(α – π/4) + sin²(α + π/2) – sin²(α – π/2) = \(\frac{1}{2}\)
Solution:

Question 3.
If sin x + sin y = \(\frac{1}{4}\) and cos x + cos y = \(\frac{1}{3}\), then show that
(i) \(\tan \left(\frac{x+y}{2}\right)=\frac{3}{4}\)
(ii) cot (x + y) = \(\frac{7}{24}\)
Solution:

Question 4.
If neither [A – \(\frac{\pi}{12}\)] nor [A – \(\frac{5 \pi}{12}\)] is an integral multiple of π. Prove that cot(π/2 – A) + tan(π/12 + A) = \(\frac{4 \cos 2 A}{1-2 \sin 2 A}\)
Solution:

Question 5.
Prove that 4 cos 12° cos 48° cos 72° = cos 36°.
Solution:
LHS = 4 cos 12° cas 48° cos 72°
= 2 cos 12° {2 cos 72° cos 48°}
= 2 cos 12° {cos (72° + 48°) + cos (72° – 48°)}
= 2 cos 12° {cos (120°) + cos 24°)
= 2 cos 12° {\(\frac{1}{2}\) + cos 24°}
= 2 cos 12° \(\left\{\frac{-1+2 \cos 24^{\circ}}{2}\right\}\)
= -cos 12° + 2 cos 24° cos 12°
= -cos 12° + {cos(24° + 12°) + cos (24° – 12°))
= -cos 12° + cos 36° + cos 12°
= cos 36°
= RHS
∴ 4 cos 12° cos 48° cos 72° = cos 36°

Question 6.
Prove that sin 10° + sin 20° + sin 40° + sin 50° = sin 70° + sin 80°
Solution:
LH.S. = sin 10° + sin 20° + sin 40° + sin 50°
= (sin 50°+ sin 10°) + (sin 40° + sin 20°)
= 2 sin(\(\frac{50^{\circ}+10^{\circ}}{2}\)) . cos(\(\frac{50^{\circ}-10^{\circ}}{2}\)) + 2 sin(\(\frac{40^{\circ}+20^{\circ}}{2}\)) . cos(\(\frac{40^{\circ}-20^{\circ}}{2}\))
= 2 . sin (30°) . cos 20° + 2 sin 30° . cos 10°
= 2 sin 30° (cos 20° + cos 10°)
= 2(\(\frac{1}{2}\)) [cos(90° – 70°) + cos(90° – 80°)]
= sin 70° + sin 80°
= RHS
∴ sin 10° + sin 20° + sin 40° + sin 50° = sin 70° + sin 80°

III.

Question 1.
If cos x + cos y = \(\frac{4}{5}\) and cos x – cos y = \(\frac{2}{7}\) find the value of \(14 \tan \left(\frac{x-y}{2}\right)+5 \cot \left(\frac{x+y}{2}\right)\)
Solution:

Question 2.
If none of the denominators is zero, prove that
\(\left(\frac{\cos A+\cos B}{\sin A-\sin B}\right)^{n}+\left(\frac{\sin A+\sin B}{\cos A-\cos B}\right)^{n}\) = \(\begin{cases}2 \cot ^{n}\left(\frac{A-B}{2}\right), & \text { if } n \text { is even } \\ 0, & \text { if } n \text { is odd }\end{cases}\)
Solution:

Question 3.
If sin A = sin B and cos A = cos B, then prove that A = 2nπ + B for some integer n.
Solution:

Question 4.
If cos nα ≠ 0 and cos \(\frac{\alpha}{2}\) ≠ 0. then show that \(\frac{\sin (n+1) \alpha-\sin (n-1) \alpha}{\cos (n+1) \alpha+2 \cos n \alpha+\cos (n-1) \alpha}\) = tan \(\frac{\alpha}{2}\)
Solution:

Question 5.
If sec (θ + α) + sec (θ – α) = 2 sec θ and cos α ≠ 1, then show that cos θ = ±√2 cos \(\frac{\alpha}{2}\).
Solution:

Question 6.
If none of x, y, z is an odd multiple of \(\frac{\pi}{2}\) and if sin (y + z – x), sin (z + x – y), sin (x + y – z) are in A.P., then prove that tan x, tan y, tan z are also in A.P.
Solution:
sin (y + z – x), sin (z + x – y), sin (x + y – z) are in A.P.
⇒ sin (z + x – y) – sin (y + z – x) = sin (x + y – z) – sin (z + x – y)
⇒ 2 cos z sin (x – y) = 2 cos x sin (y – z)
⇒ cos z [sin x cos y – cos x sin y] = cos x [sin y cos z – cos y sin z]
Dividing with cos x cos y cos z, we get
\(\frac{\sin x}{\cos x}-\frac{\sin y}{\cos y}=\frac{\sin y}{\cos y}-\frac{\sin z}{\cos z}\)
⇒ tan x – tan y = tan y – tan z
⇒ tan x + tan z = 2 tan y
∴ tan x, tan y, tan z are in A.P.

Question 7.
If x, y, z are non zero real numbers and if x cos θ = y cos (θ + \(\frac{2 \pi}{3}\)) = z cos (θ + \(\frac{2 \pi}{3}\)) for some θ ∈ R, then show that xy + yz + zx = 0.
Solution:

Question 8.
If neither A nor A + B is an odd multiple of \(\frac{\pi}{2}\) and if m sin B = n sin(2A + B), then prove that (m + n) tan A = (m – n) tan (A + B).
Solution:
Neither A nor (A + B) is an odd multiple of \(\frac{\pi}{2}\)
Given that m sin B = n sin (2A + B)

Question 9.
If tan (A + B) = λ tan (A – B), then show that (λ + 1) sin 2B = (λ – 1) sin 2A.
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(d) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(d)

I.

Question 1.
Simplify
(i) \(\frac{\sin 2 \theta}{1+\cos 2 \theta}\)
Solution:
\(\frac{\sin 2 \theta}{1+\cos 2 \theta}\)
= \(\frac{2 \sin \theta \cos \theta}{2 \cos ^{2} \theta}\)
= tan θ

(ii) \(\frac{3 \cos \theta+\cos 3 \theta}{3 \sin \theta-\sin 3 \theta}\)
Solution:
\(\frac{3 \cos \theta+\cos 3 \theta}{3 \sin \theta-\sin 3 \theta}\)
= \(\frac{3 \cos \theta+4 \cos ^{3} \theta-3 \cos \theta}{3 \sin \theta-\left(3 \sin \theta-4 \sin ^{3} \theta\right)}\)
= \(\frac{4 \cos ^{3} \theta}{4 \sin ^{3} \theta}\)
= cot³θ

Question 2.
Evaluate the following.
(i) 6 sin 20° – 8 sin3 20°
Solution:
6 sin 20° – 8 sin³20°
= 2(3 sin 20° – 4 sin³20°)
= 2 sin (3 × 20)
= 2 sin 60°
= 2 \(\left[\frac{\sqrt{3}}{2}\right]\)
= √3

(ii) cos²72° – sin²54°
Solution:

(iii) sin²42° – sin²12°
Solution:
sin (42 + 12) sin (42 – 12)
= sin 54° . sin 30°
= \(\left[\frac{\sqrt{5}+1}{4}\right] \frac{1}{2}\)
= \(\frac{\sqrt{5}+1}{8}\)

Question 3.
(i) Express \(\frac{\sin 4 \theta}{\sin \theta}\) interms of cos³θ and cos θ.
Solution:
consider sin 4θ = sin(3θ + θ)
= sin 3θ cos θ + cos 3θ sin θ
= (3 sin θ – 4 sin³θ) cos θ + (4 cos³θ – 3 cos θ) sin θ
= 3 sin θ cos θ – 4 sin³θ cos θ + 4 cos³θ sin θ – 3 cos θ sin θ
= 4 cos³θ sin θ – 4 sin³θ cos θ
= sin θ (4 cos³θ – 4 sin²θ cos θ)
\(\frac{\sin 4 \theta}{\sin \theta}=\frac{\sin \theta\left(4 \cos ^{3} \theta-4 \sin ^{2} \theta \cos \theta\right)}{\sin \theta}\)
= 4 cos³θ – 4(1 – cos²θ) cos θ
= 4 cos³θ – 4 cos θ + 4 cos³θ
= 8 cos³θ – 4 cos θ

(ii) Express cos⁶A + sin⁶A in terms of sin 2A.
Solution:
cos⁶A + sin⁶A
= (cos²A)³ + (sin²A)³
= (cos²A + sin²A)³ – 3 cos²A sin²A (cos²A + sin²A)
= 1 – 3 cos²A sin²A
= 1 – \(\frac{3}{4}\) (4 cos²A sin²A)
= 1 – \(\frac{3}{4}\) (sin²2A)

(iii) Express \(\frac{1-\cos \theta+\sin \theta}{1+\cos \theta+\sin \theta}\) in terms of tan θ/2
Solution:

Question 4.
(i) If sin α = \(\frac{3}{5}\), where \(\frac{\pi}{2}\) < α < π, evaluate cos 3α and tan 2α.
Solution:

(ii) If cos A = \(\frac{7}{25}\) and \(\frac{3 \pi}{2}\) < A < 2π, then find the value of cot A/2.
Solution:

(iii) If 0 < θ < \(\frac{\pi}{8}\), show that \(\sqrt{2+\sqrt{2+\sqrt{2+2 \cos 4 \theta)}}}=2 \cos (\theta / 2)\)
Solution:

Question 5.
Find the extreme values of
(i) cos 2x + cos2x
Solution:
cos 2x + cos²x
= 2 cos²x – 1 + cos²x
= 3 cos²x – 1
-1 ≤ cos x ≤ 1
0 ≤ cos²x ≤ 1
0 ≤ 3 cos²x ≤ 3
-1 ≤ 3 cos²x – 1 ≤ 2
maximum value = 2
minimum value = -1

(ii) 3 sin²x + 5 cos²x
Solution:
3 sin²x + 5 cos²x
= 3(1 – cos²x) + 5 cos²x
= 3 – 3 cos²x + 5 cos²x
= 3 + 2 cos²x
-1 ≤ cos x ≤ 1
0 ≤ cos²x ≤ 1
0 ≤ 2 cos²x ≤ 2
3 ≤ 3 + 2 cos²x ≤ 5
maximum value = 5
minimum value = 3

Question 6.
If a ≤ cos θ + 3√2 sin[θ + \(\frac{\pi}{4}\)] + 6 ≤ b, then find the largest value of a and smallest value of b.
Solution:
a ≤ cos θ + 3√2 sin[θ + \(\frac{\pi}{4}\)] + 6 ≤ b
consider cos θ + 3√2 sin[θ + \(\frac{\pi}{4}\)] + 6
= cos θ + 3√2[sin θ cos \(\frac{\pi}{4}\) + cos θ sin \(\frac{\pi}{4}\)] + 6
= cos θ + 3√2 sin θ \(\frac{1}{\sqrt{2}}\) + 3√2 cos θ \(\frac{1}{\sqrt{2}}\) + 6
= cos θ + 3 sin θ + 3 cos θ + 6
= 4 cos θ + 3 sin θ + 6
∴ a = 4, b = 3, c = 6
maximum value = c + \(\sqrt{a^{2}+b^{2}}\)
= 6 + \(\sqrt{16+9}\)
= 6 + 5
= 11
minimum value = c – \(\sqrt{a^{2}+b^{2}}\)
= 6 – 5
= 1

Question 7.
Find the periods for the following functions.
(i) cos⁴x
Solution:

(ii) \(2 \sin \left[\frac{\pi x}{4}\right]+3 \cos \left[\frac{\pi x}{3}\right]\)
Solution:

(iii) sin²x + 2 cos²x
Solution:
Let f(x) = sin²x + 2 cos²x
= 1 – cos²x + 2 cos²x
= 1 + cos²x
= 1 + \(\frac{1+\cos 2 x}{2}\)
∴ period of cos 2x = \(\frac{2 \pi}{2}\) = π
∴ period of f(x) = π

(iv) \(2 \sin \left[\frac{\pi}{4}+x\right] \cos x\)
Solution:

(v) \(\frac{5 \sin x+3 \cos x}{4 \sin 2 x+5 \cos x}\)
Solution:
Let f(x) = \(\frac{5 \sin x+3 \cos x}{4 \sin 2 x+5 \cos x}\)
period of sin x = 2π
period of cos x = 2π
period of sin 2x = \(\frac{2 \pi}{2}\) = π
period of cos x = 2π
L.C.M. of (2π, 2π, π, 2π) = 2π
∴ period of f(x) = 2π

II.

Question 1.
(i) If 0 < A < \(\frac{\pi}{4}\) and cos A = \(\frac{4}{5}\), find the values of sin 2A and cos 2A.
Solution:

(ii) For what values of A in the first quadrant, the expression \(\frac{\cot ^{3} A-3 \cot A}{3 \cot ^{2} A-1}\) is positive?
Solution:

(iii) Prove that \(\frac{\cos 3 A+\sin 3 A}{\cos A-\sin A}=1+2 \sin A\)
Solution:

Question 2.
(i) Prove that \(\cot \left[\frac{\pi}{4}-\theta\right]=\frac{\cos 2 \theta}{1-\sin 2 \theta}\) and hence find the value of cot 15°.
Solution:

(ii) If θ lies in third quadrant and sin θ = \(\frac{-4}{5}\), find the values of cosec (θ/2) and tan (θ/2).
Solution:

(iii) If 450° < θ < 540° and sin θ = \(\frac{12}{13}\), then calculate sin (θ/2) and cos (θ/2)
Solution:

(iv) Prove that \(\frac{1}{\cos 290^{\circ}}+\frac{1}{\sqrt{3} \sin 250^{\circ}}=\frac{4}{\sqrt{3}}\)
Solution:

Question 3.
Prove that
(i) \(\frac{\sin 2 A}{(1-\cos 2 A)} \cdot \frac{(1-\cos A)}{\cos A}=\tan \frac{A}{2}\)
Solution:

(ii) \(\frac{\sin 2 x}{(\sec x+1)} \cdot \frac{\sec 2 x}{(\sec 2 x+1)}\) = \(\tan \frac{x}{2}\)
Solution:

(iii) \(\frac{\left(\cos ^{3} \theta-\cos 3 \theta\right)}{\cos \theta}+\frac{\left(\sin ^{3} \theta+\sin 3 \theta\right)}{\sin \theta}=\mathbf{3}\)
Solution:

Question 4.
(i) Show that cos A = \(\frac{\cos 3 A}{(2 \cos 2 A-1)}\). Hence find the value of cos 15°.
Solution:

(ii) Show that sin A = \(\frac{\sin 3 A}{1+2 \cos 2 A}\). Hence find the value of sin 15°.
Solution:

(iii) Prove that tan α = \(\frac{\sin 2 \alpha}{1+\cos 2 \alpha}\) and hence deduce the values tan 15° and tan 22\(\frac{1^{\circ}}{2}\).
Solution:

Question 5.
Prove that
(i) \(\frac{1}{\sin 10^{\circ}}-\frac{\sqrt{3}}{\cos 10^{\circ}}=4\)
Solution:

(ii) √3 cosec 20° – sec 20° = 4
Solution:

(iii) tan 9° – tan 27° – cot 27° + cot 9° = 4
Solution:

(iv) If \(\frac{\sin \alpha}{a}=\frac{\cos \alpha}{b}\), then prove that a sin 2α + b cos 2α = b
Solution:
Given \(\frac{\sin \alpha}{a}=\frac{\cos \alpha}{b}\)
b sin α = a cos α
L.H.S. = a sin 2α + b cos 2α
= a 2 sin α cos α + b (1 – 2 sin²α)
= 2 sin α (a cos α) + b – 2b sin²α
= 2 sin α (b sin α) + b – 2b sin²α
= 2b sin²α + b – 2b sin²α
= b

Question 6.
(i) In a ∆ABC; if \(\tan \frac{A}{2}=\frac{5}{6}\) and tan\(\tan \frac{B}{2}=\frac{20}{37}\), then show that \(\tan \frac{C}{2}=\frac{2}{5}\)
Solution:

(ii) If cos θ = \(\frac{5}{13}\) and 270° < θ < 360°, evaluate sin (θ/2) and cos (θ/2).
Solution:
cos θ = \(\frac{5}{13}\)
given 270 < θ < 360°
⇒ 135 < \(\frac{\theta}{2}\) < 180°
∴ θ lies in the fourth quadrant
θ/2 lies is second quadrant

(iii) If 180° < θ < 270° and sin θ = \(\frac{-4}{5}\) calculate sin[θ/2] cos[θ/2]
Solution:
Given sin θ = \(\frac{-4}{5}\)
⇒ cos θ = \(\frac{-3}{5}\)
given 180 < θ < 270
∴ θ in the III quadrant
Now 90 < \(\frac{\theta}{2}\) < 135
∴ \(\frac{\theta}{2}\) is in II quadrant

Question 7.
(i) Prove that \(\cos ^{2} \frac{\pi}{8}+\cos ^{2} \frac{3 \pi}{8}+\cos ^{2} \frac{5 \pi}{8}+\cos ^{2} \frac{7 \pi}{8}\) = 2
Solution:

(ii) Show that \(\cos ^{4}\left(\frac{\pi}{8}\right)+\cos ^{4}\left(\frac{3 \pi}{8}\right)+\cos ^{4}\left(\frac{5 \pi}{8}\right)+\cos ^{4}\left(\frac{7 \pi}{8}\right)\) = \(\frac{3}{2}\)
Solution:

III.

Question 1.
(i) If tan x + tan(x + \(\frac{\pi}{3}\)) + tan(x + \(\frac{2 \pi}{3}\)) = 3, show that tan 3x = 1
Solution:

(ii) Prove that \(\sin \frac{\pi}{5} \sin \frac{2 \pi}{5} \cdot \sin \frac{3 \pi}{5} \cdot \sin \frac{4 \pi}{5}\) = \(\frac{5}{16}\)
Solution:

(iii) Show that \(\cos ^{2}\left(\frac{\pi}{10}\right)+\cos ^{2}\left(\frac{2 \pi}{5}\right)+\cos ^{2}\left(\frac{3 \pi}{5}\right)\) + \(\cos ^{2}\left(\frac{9 \pi}{10}\right)\) = 2
Solution:

Question 2.
(i) \(\frac{1-\sec 8 \alpha}{1-\sec 4 \alpha}=\frac{\tan 8 \alpha}{\tan 2 \alpha}\)
Solution:

(ii) \(\left[1+\cos \frac{\pi}{10}\right]\left[1+\cos \frac{3 \pi}{10}\right]\left[1+\cos \frac{7 \pi}{10}\right]\) \(\left[1+\cos \frac{9 \pi}{10}\right]=\frac{1}{16}\)
Solution:

Question 3.
(i) Prove that \(\cos \frac{2 \pi}{7} \cdot \cos \frac{4 \pi}{7} \cdot \cos \frac{8 \pi}{7}=\frac{1}{8}\)
Solution:

(ii) \(\cos \frac{\pi}{11} \cdot \cos \frac{2 \pi}{11} \cdot \cos \frac{3 \pi}{11} \cdot \cos \frac{4 \pi}{11}\) \(\cos \frac{5 \pi}{11}=\frac{1}{32}\)
Solution:

Question 4.
(i) If cos α = \(\frac{3}{5}\) and cos β = \(\frac{5}{13}\) and α, β are acute angles, then prove that
(a) \(\sin ^{2}\left[\frac{\alpha-\beta}{2}\right]=\frac{1}{65}\)
(b) \(\cos ^{2}\left[\frac{\alpha+\beta}{2}\right]=\frac{16}{65}\)
Solution:

(ii) If A is not an integral multiple of π, prove that cos A . cos 2A . cos 4A . cos 8A = \(\frac{\sin 16 A}{16 \sin A}\) and hence deduce that \(\cos \frac{2 \pi}{15} \cdot \cos \frac{4 \pi}{15} \cdot \cos \frac{8 \pi}{15} \cdot \cos \frac{16 \pi}{15}=\frac{1}{16}\)
Solution:
Take 16 sin A {cos A . cos 2A . cos 4A . cos 8A}
= 8(2 sin A . cos A) cos 2A . cos 4A . cos 8A
= 8 sin 2A . cos 2A . cos 4A . cos 8A
= 4(2 sin 2A . cos 2A) . cos 4A . cos 8A
= 4 sin 4A . cos 4A . cos 8A
= 2 (2 sin 4A . cos 4A) . cos 8A
= 2 sin 8A . cos 8A
= sin (16A)
∴ 16 sin A {cos A . cos 2A . cos 4A . cos 8A} = sin (16A)
∴ cos A . cos 2A . cos 4A . cos 8A = \(\frac{\sin (16 A)}{16 \sin A}\)

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(c) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(c)

I.

Question 1.
Simplify the following.
(i) cos 100° cos 40° + sin 100° sin 40°
Solution:
cos 100° cos 40° + sin 100° sin 40° = cos(100° – 40°)
= cos 60°
= \(\frac{1}{2}\)

(ii) \(\frac{\cot 55 \cot 35}{\cot 55+\cot 35}\)
Solution:
\(\frac{\cot 55 \cot 35}{\cot 55+\cot 35}\) = cot(55 + 35)
= cot 90
= 0

(iii) \(\tan \left[\frac{\pi}{4}+\theta\right] \cdot \tan \left[\frac{\pi}{4}-\theta\right]\)
Solution:
\(\tan \left[\frac{\pi}{4}+\theta\right] \cdot \tan \left[\frac{\pi}{4}-\theta\right]\)
\(\left[\frac{1+\tan A}{1-\tan A}\right]\left[\frac{1-\tan A}{1+\tan A}\right]\) = 1

(iv) tan 75° + cot 75°
Solution:
tan 75° + cot 75°
= 2 + √3 + 2 – √3
= 4

(v) sin 1140° cos 390° – cos 780° sin 750°
Solution:
sin 1140° cos 390° – cos 780° sin 750°
= sin(3 × 360° + 60°) cos(360°+ 30°) – cos(2 × 360° + 60°) sin(2 × 360° + 30)
= sin 60° cos 30° – cos 60° sin 30°
= sin(60° – 30°)
= sin 30°
= \(\frac{1}{2}\)

Question 2.
(i) Express \(\frac{\sqrt{3} \cos 25+\sin 25}{2}\) as a sine of an angle.
Solution:
\(\frac{\sqrt{3} \cos 25+\sin 25}{2}\)
= \(\frac{\sqrt{3}}{2}\) cos 25° + \(\frac{1}{2}\) sin 25°
= sin 60° cos 25° + cos 60° sin 25°
= sin (60° + 25°)
= sin 85°

(ii) Express (cos θ – sin θ) as a cosine of an angle.
Solution:
cos θ – sin θ
Divide & multiply with √2
\(\frac{1}{\sqrt{2}}\) √2 (cos θ – sin θ)
= √2 \(\frac{1}{\sqrt{2}}\) cos θ – sin θ \(\frac{1}{\sqrt{2}}\)
= √2 [cos \(\frac{\pi}{4}\) cos θ – sin \(\frac{\pi}{4}\) sin θ]
= √2 \(\cos \left[\frac{\pi}{4}+\theta\right]\)

(iii) Express tan θ in terms of tan α, If sin (θ + α) = cos (θ + α).
Solution:
tan θ in term of tan α, if sin(θ + α) = cos (θ + α)
given sin(θ + α) = cos(θ + α)
sin θ cos α + cos θ sin α = cos θ cos α – sin θ sin α and cos θ cos α
\(\frac{\sin \theta \cos \alpha}{\cos \theta \cos \alpha}+\frac{\cos \theta \sin \alpha}{\cos \theta \cos \alpha}=\frac{\cos \theta \cos \alpha}{\cos \theta \cos \alpha}\) – \(\frac{\sin \theta \sin \alpha}{\cos \theta \cos \alpha}\)
tan θ + tan α = 1 – tan θ tan α
tan θ + tan θ tan α = 1 – tan α
tan θ (1 + tan α) = 1 – tan α
tan θ = \(\frac{1-\tan \alpha}{1+\tan \alpha}\)

Question 3.
(i) If tan θ = \(\frac{\cos 11^{\circ}+\sin 11^{\circ}}{\cos 11^{\circ}-\sin 11^{\circ}}\) and θ is the third quadrant find θ.
Solution:
Given tan θ = \(\frac{\cos 11^{\circ}+\sin 11^{\circ}}{\cos 11^{\circ}-\sin 11^{\circ}}\)
= \(\frac{1+\tan 11^{\circ}}{1-\tan 11^{\circ}}\)
= tan (45° + 11°)
= tan (56°)
tan θ = tan 56° = tan (180° + 50°) = tan 236°
∴ θ = 236°

(ii) If 0° < A, B < 90°, such that cos A = \(\frac{5}{13}\) and sin B = \(\frac{4}{5}\), find the value of sin(A – B).
Solution:

(iii) What is the value of tan 20° + tan 40° + √3 tan 20° tan 40°?
Solution:
consider 20° + 40° = 60°
tan (20° + 40°) = tan 60°
\(\frac{\tan 20^{\circ}+\tan 40^{\circ}}{1-\tan 20^{\circ} \tan 40^{\circ}}\) = √3
tan 20° + tan 40° = √3 – √3 tan 20° tan 40°
tan 20° + tan 40° + √3 tan 20° tan 40° = √3

(iv) Find the value of tan 56° – tan 11° – tan 56° tan 11°.
Solution:
consider 56° – 11° = 45°
tan (56° – 11) = tan 45°
\(\frac{\tan 56^{\circ}-\tan 11^{\circ}}{1+\tan 56^{\circ} \tan 11^{\circ}}\) = 1
tan 56° – tan 11 ° = 1 + tan 56° tan 11°
tan 56° – tan 11° – tan 56° tan 11° = 1

(v) Evaluate \(\sum \frac{\sin (A+B) \sin (A-B)}{\cos ^{2} A \cos ^{2} B}\); if none of cos A, cos B, cos C is zero.
Solution:

(vi) Evaluate \(\sum \frac{\sin (C-A)}{\sin C \sin A}\) if none of sin A, sin B, sin C is zero.
Solution:

Question 4.
Prove that
(i) cos 35° + cos 85° + cos 155° = 0
Solution:
cos 35° + cos 85° + cos 155°
= -cos 85° + 2 cos\(\left(\frac{35+155}{2}\right)\) cos\(\left(\frac{35-155}{2}\right)\)
= -cos 85° + 2 cos 85° \(\left(\frac{1}{2}\right)\)
= -cos 85° + cos 85°
= 0

(ii) tan 72° = tan 18° + 2 tan 54°
Solution:
cos A – tan A = \(\frac{1}{\tan A}\) – tan A
= \(\frac{1-\tan ^{2} A}{\tan A}\)
= \(\frac{2\left(1-\tan ^{2} A\right)}{2 \tan A}\)
= \(\frac{2}{\tan 2 A}\)
= 2 cot 2A
cot A = tan A + 2 cot 2A
put A = 18
cot 18° = tan 18° + 2 cot 36°
cot (90° – 72°) = tan 18° + 2 cot (90° – 54°)
tan 72° = tan 18° + 2 tan 54°

(iii) sin 750° cos 480° + cos 120° cos 60° = \(\frac{-1}{2}\)
Solution:
sin 750° = sin (2 × 360° + 30°)
= sin 30°
= \(\frac{1}{2}\)
cos 480° = cos (360° + 120°)
= cos 120°
= \(\frac{-1}{2}\)
L.H.S. = sin 750° cos 480° + cos 120° cos 60°
= \(\frac{1}{2}\left(\frac{-1}{2}\right)+\left(\frac{-1}{2}\right)\left(\frac{1}{2}\right)\)
= \(\frac{-1}{4}-\frac{1}{4}\)
= \(\frac{-1}{2}\)

(iv) cos A + cos(\(\frac{4 \pi}{3}\) – A) + cos(\(\frac{4 \pi}{3}\) + A) = o
Solution:
cos A + cos(\(\frac{4 \pi}{3}\) – A) + cos(\(\frac{4 \pi}{3}\) + A)
= cos A + 2 cos \(\frac{4 \pi}{3}\) cos A (∵ cos(A + B) + cos(A – B) = 2 cos A cos B)
= cos A + 2\(\left(\frac{-1}{2}\right)\) cos A
= cos A – cos A
= 0

(v) cos²θ + cos²(\(\frac{2 \pi}{3}\) + θ) + cos²(\(\frac{2 \pi}{3}\) – θ)
Solution:

Question 5.
Evaluate
(i) \(\sin ^{2} 82 \frac{1}{2}^{\circ}-\sin ^{2} 22 \frac{1^{\circ}}{2}\)
Solution:

(ii) \(\cos ^{2} 112 \frac{1}{2}^{\circ}-\sin ^{2} 52 \frac{1}{2}^{\circ}\)
Solution:

(iii) \(\sin ^{2}\left[\frac{\pi}{8}+\frac{A}{2}\right]-\sin ^{2}\left[\frac{\pi}{8}-\frac{A}{2}\right]\)
Solution:

(iv) \(\cos ^{2} 52 \frac{1}{2}^{\circ}-\sin ^{2} 22 \frac{1}{2}^{\circ}\)
Solution:

Question 6.
Find the minimum and maximum values of
(i) 3 cos x + 4 sin x
Solution:
a = 4, b = 3, c = 0
Minimum value = \(c-\sqrt{a^{2}+b^{2}}=\sqrt{16+9}=-5\)
Maximum value = \(c+\sqrt{a^{2}+b^{2}}=\sqrt{16+9}=5\)

(ii) sin 2x – cos 2x
Solution:
a = 1, b = -1, c = 0
minimum value = \(c-\sqrt{a^{2}+b^{2}}=-\sqrt{1+1}\) = -√2
maximum value = \(c+\sqrt{a^{2}+b^{2}}=\sqrt{1+1}=\sqrt{2}\)

Question 7.
Find the range of
(i) 7 cos x – 24 sin x + 5
Solution:

(ii) 13 cos x + 3√3 sin x – 4
Solution:

II.

Question 1.
(i) If cos α = \(\frac{-3}{5}\) and sin β = \(\frac{7}{25}\), where \(\frac{\pi}{2}\) < α < π and 0 < β < \(\frac{\pi}{2}\), then find the values of tan(α + β) and sin(α + β).
Solution:

(ii) If 0 < A < B < \(\frac{\pi}{4}\) and sin (A + B) = \(\frac{24}{25}\) and cos (A – B) = \(\frac{4}{5}\), then find the value of tan 2A.
Solution:

(iii) If A + B, A are acute angles such that sin (A + B) = \(\frac{24}{25}\) and tan A = \(\frac{3}{4}\), then find the value of cos B.
Solution:
sin (A + B) = \(\frac{24}{25}\) and (A + B) is acute angle

(iv) If tan α – tan β = m and cot α – cot β = n, then prove that cot (α – β) = \(\frac{1}{m}-\frac{1}{n}\)
Solution:

(v) If tan (α – β) = \(\frac{7}{24}\) and tan α = \(\frac{4}{3}\), where α and β are in the first quadrant prove that α – β = \(\frac{\pi}{2}\).
Solution:

Question 2.
(i) Find the expansion of sin (A + B – C).
Solution:
sin (A + B – C) = sin [(A + B) – C]
= sin (A + B). cos C – cos (A + B) sin C
= (sin A cos B + cos A sin B) cos C – (cos A cos B – sin A sin B) sin C
= sin A cos B cos C + cos A sin B cos C – cos A cos B sin C + sin A sin B sin C

(ii) Find the expansion of cos (A – B – C).
Solution:
cos (A – B – C) = cos {(A – B) – C}
= cos (A – B) cos C + sin (A – B) sin C
= (cos A cos B + sin A sin B) cos C + (sin A cos B – cos A sin B) sin C
= cos A cos B cos C + sin A sin B cos C + sin A cos B sin C – cos A sin B sin C

(iii) In a ΔABC, A is obtuse. If sin A = \(\frac{3}{5}\) and sin B = \(\frac{5}{13}\), then show that sin C = \(\frac{16}{65}\)
Solution:
Given sin A = \(\frac{3}{5}\)
cos²A = 1 – sin²A
= 1 – \(\frac{9}{25}\)
= \(\frac{16}{25}\)
cos A = ±\(\frac{4}{5}\)
A is obtuse ⇒ 90° < A < 180°
A tan in II quadrant ⇒ cos A is negative
∴ cos A = \(\frac{-4}{5}\),
Given sin β = \(\frac{5}{13}\)
cos²β = 1 – sin²β
= 1 – \(\frac{25}{169}\)
= \(\frac{144}{169}\)
cos β = ±\(\frac{5}{13}\)
β is acute ⇒ cos β is possible
sin β = \(\frac{12}{13}\)
A + B + C = 180°
C = 180° – (A + B)
sin C = sin (180° – (A + B))
= sin (A + B)
= sin A cos B + cos A sin B
= \(\left(\frac{3}{5}\right)\left(\frac{12}{13}\right)+\left(\frac{-4}{5}\right)\left(\frac{5}{13}\right)\)
= \(\frac{36-20}{65}\)
= \(\frac{16}{65}\)
∴ sin C = \(\frac{16}{65}\)

(iv) If \(\frac{\sin (\alpha+\beta)}{\sin (\alpha-\beta)}=\frac{a+b}{a-b}\), then prove that tan β = ab tan α
Solution:

III.

Question 1.
(i) If A – B = \(\frac{3 \pi}{4}\), then show that (1 – tan A) (1 + tan B) = 2.
Solution:
A – B = \(\frac{3 \pi}{4}\)
tan (A – B) = tan \(\frac{3 \pi}{4}\)
\(\frac{\tan A-\tan B}{1+\tan A \tan B}\) = -1
tan A – tan B = -1 – tan A tan B
1 = -tan A + tan B – tan A tan B
2 = 1 – tan A + tan B – tan A tan B
2 = (1 – tan A) – tan B (1 – tan A)
(1 – tan A) (1 – tan B) = 2

(ii) If A + B + C = \(\frac{\pi}{2}\) and none of A, B, C is an odd multiple of \(\frac{\pi}{2}\), then prove that
(a) cot A + cot B + cot C = cot A cot B cot C
(b) tan A tan B + tan B tan C + tan C tan A = 1 and hence, show that \(\sum \frac{\cos (B+C)}{\cos B \cos C}\) = 2
Solution:

Question 2.
(i) Prove that sin²α + cos²(α + β) + 2 sin α sin β cos(α + β) is independent of α.
Solution:
sin²α + cos²(α + β) + 2 sin α cos (α + β)
= sin²α + cos(α + β) (cos(α + β) + 2 sin α sin β)
= sin²α + cos(α + β) (cos α cos β – sin α sin β + 2 sin α sin β)
= sin²α + cos(α + β) (cos α cos β + sin α sin β)
= sin²α + cos(α + β) cos(α – β)
= sin²α + cos²β – sin²α
= cos²β

(ii) Prove that cos²(α – β) + cos²β – 2 cos(α – β) cos α cos β is independent of β.
Solution:
cos²(α – β) + cos²β – 2 cos (α – β) cos α cos β
= cos²(α – β) + cos²β – cos (α – β) [cos (α + β) + cos (α – β)]
= cos²(α – β) + cos²β – cos (α – β) cos (α + β) – cos²(α – β)
= cos²β – [cos²β – sin²α]
= cos²β – cos²β + sin²α
= sin²α

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(b) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(b)

I. Find the periods for the given 1 – 5 functions.

Question 1.
cos(3x + 5) + 7
Solution:
f(x) = cos(3x + 5) + 7
We know that the function g(x) = cos x for all x ∈ R has the period 2π.
Now f(x) = cos(3x + 5) + 7
We get that f(x) is periodic and the period of f is \(\frac{2 \pi}{|3|}=\frac{2 \pi}{3}\)

Question 2.
tan 5x
Solution:
The function g(x) = tan x periodic and π is the period.
∴ f(x) = tan 5x periodic and its period is \(\frac{\pi}{|5|}=\frac{\pi}{5}\)

Question 3.
\(\cos \left(\frac{4 x+9}{5}\right)\)
Solution:
The function h(x) = cos x for all x ∈ R has the period 2π.
Now f(x) = \(\cos \left(\frac{4 x}{5}+\frac{9}{5}\right)\) is periodic and period of f is \(\frac{2 \pi}{\left(\frac{4}{5}\right)}=\frac{5 \pi}{2}\)

Question 4.
|sin x|
Solution:
The function h(x) = sin x for all x ∈ R has the period 2π.
But f(x) = |sin x| is periodic and its period is π.
∵ f(x + π) = |sin(x + π)|
= |-sin x|
= sin x

Question 5.
tan(x + 4x + 9x + …… + n²x) (n any positive integer)
Solution:
tan(1² + 2² + 3² + …… + n²) x = \(\tan \left[\frac{n(n+1)(2 n+1)}{6}\right] x\)
period = \(\frac{6 \pi}{n(n+1)(2 n+1)}\)

Question 6.
Find a sine function whose period is \(\frac{2}{3}\)
Solution:
\(\frac{2 \pi}{|k|}=\frac{2}{3}\)
3π = |k|
∴ sin kx = sin 3πx

Question 7.
Find a cosine function whose period is 7.
Solution:
\(\frac{2 \pi}{|k|}\) = 7
\(\frac{2 \pi}{7}\) = |k|
∴ cos kx = cos \(\frac{2 \pi}{7}\) x

II. Sketch the graph of the following functions.

Question 1.
tan x between 0 and \(\frac{\pi}{4}\)
Solution:

Question 2.
cos 2x in [0, π]
Solution:

Question 3.
sin 2x in the interval (0, π)
Solution:

Question 4.
sin x in the interval [-π, +π]
Solution:

Question 5.
cos²x in [0, π]
Solution:

III.

Question 1.
Sketch the region enclosed by y = sin x, y = cos x and X-axis in the interval [0, π].
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(a) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Trigonometric Ratios up to Transformations Solutions Exercise 6(a)

I. Convert the following into the simplest form.

Question 1.
(i) tan(θ – 14π)
Solution:
= tan(14π – θ)
= tan(2 . (7π) – θ)
= tan θ

(ii) \(\cot \left(\frac{21 \pi}{2}-\theta\right)\)
Solution:
\(\cot \left(\frac{21 \pi}{2}-\theta\right)\)
= \(\cot \left(10 \pi+\left(\frac{\pi}{2}-\theta\right)\right)\)
= \(\cot \left(\frac{\pi}{2}-\theta\right)\)
= tan θ

(iii) cosec(5π + θ)
Solution:
cosec(5π + θ) = cosec(2π + (3π + θ))
= cosec(3π + θ)
= cosec(2π + (π + θ))
= cosec(π + θ)
= -cosec θ

(iv) sec(4π – θ?)
Solution:
sec(4π – θ)
= sec(2π + (2π – θ))
= sec(2π – θ)
= sec θ

Question 2.
Find the value of each of the following.
(i) sin(-405°)
Solution:
sin(-405°) = -sin(360° + 45)
= -sin 45°
= \(-\frac{1}{\sqrt{2}}\)

(ii) \(\cos \left(-\frac{7 \pi}{2}\right)\)
Solution:
\(\cos \left(-\frac{7 \pi}{2}\right)\)
= -cos 630°
= -cos(360° + 270°)
= -cos 270°
= -cos(180° + 90°)
= -cos 90°
= 0

(iii) sec(2100°)
Solution:
sec(2100°) = sec (5 × 360° + 300°)
= sec 300°
= sec(360 – 60°)
= sec 60°
= 2

(iv) cot(-315°)
Solution:
cot(-315°) = -cot 315°
= -cot(360° – 45°)
= -cot 45°
= 1

Question 3.
Evaluate.
(i) cos² 45° + cos² 135° + cos² 225° + cos² 315°
Solution:

(ii) \(\sin ^{2} \frac{2 \pi}{3}+\cos ^{2} \frac{5 \pi}{6}-\tan ^{2} \frac{3 \pi}{4}\)
Solution:

(iii) cos 225° – sin 225° + tan 495° – cot 495°
Solution:
cos (180° + 45°) – sin(180° + 45°) + tan(360° + 135°) – cot(360° + 135°)
= -cos 45° + sin 45° – tan 135° + cot 135°
= \(-\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}+1-1\)
= 0

(iv) (cos θ – sin θ) if (a) θ = \(\frac{7 \pi}{4}\) (b) θ = \(\frac{11 \pi}{3}\)
Solution:

Question 4.
(i) If sin θ = \(\frac{-1}{3}\) and θ does not lie in the third quadrant, find the values of (a) cos θ (b) cot θ
Solution:
∵ sin θ = \(\frac{-1}{3}\) and sin θ is negative and θ does not lie in the IIIrd quadrant.
⇒ θ lies in IV quadrant.
∴ In the IV quadrant, cos θ is positive and cot θ is negative.

(ii) If cos θ = t (0 < t < 1) and θ does not lie in the first quadrant, find the values of (a) sin θ (b) tan θ.
Solution:
cos θ = t, (0 < t < 1)
⇒ cos θ is positive and θ does not lie in the first quadrant.
⇒ θ lies in IV quadrant.
(a) sin θ = \(-\sqrt{1-\cos ^{2} \theta}=-\sqrt{1-t^{2}}\)
(b) tan θ = \(\frac{\sin \theta}{\cos \theta}=\frac{-\sqrt{1-t^{2}}}{t}\)

(iii) Find the value of sin 330°. cos 120° + cos 210°. sin 300°.
Solution:
sin 330°. cos 120° + cos 210°. sin 300°
= sin(360° – 30°) . cos(180° – 60°) + cos(180° + 30°) . sin(360° – 60°)
= (-sin 30°) (-cos 60°) + (-cos 30°) (-sin 60°)
= sin 30° cos 60° + cos 30° sin 60°
= sin(30° + 60°) [∵ sin A cos B + cos A sin B = sin(A + B)]
= sin (90°)
= 1

(iv) If cosec θ + cot θ = \(\frac{1}{3}\), find cos θ and determine the quadrant in which θ lies.
Solution:
cosec θ + cot θ = \(\frac{1}{3}\)
⇒ cosec θ – cot θ = 3 (∵ cosec²θ – cot²θ = 1)
∴ 2 cosec θ = \(\frac{1}{3}\) + 3 = \(\frac{10}{3}\)
∴ cosec θ = \(\frac{5}{3}\) and sin θ = \(\frac{3}{5}\)
2 cot θ = \(\frac{1}{3}\) – 3 = \(\frac{-8}{3}\)
∴ cot θ = \(\frac{-4}{3}\), tan θ = \(\frac{-3}{4}\)
cos θ = (cot θ) . sin θ = \(\left(\frac{-4}{3}\right)\left(\frac{3}{5}\right)=\frac{-4}{5}\)
∵ sin θ is +ve and cos θ is -ve
⇒ θ lies in II quadrant.

Question 5.
(i) If sin α + cosec α = 2. Find the value of sinⁿα + cosecⁿα, n ∈ z.
Solution:
Given sin α + cosec α = 2
Squaring on both sides
sin²α + cosec²α + 2 = 4
sin²α + cosec²α = 2
sin α + cosec α = 2
Cubing on both sides
sin³α + cosec³α + 3 sin α . cosec α (sin α + cosec α) = 8
sin³α + cosec³α + 3(2) = 8
sin³α + cosec³α = 8 – 6
sin³α + cosec³α = 2
similarly sinⁿα + cosecⁿα = -2

(ii) If sec θ + tan θ = 5. Find the quadrant in which θ lies and find the value of sin θ.
Solution:
sec θ + tan θ = 5
⇒ sec θ – tan θ = \(\frac{1}{5}\) (∵ sec²θ – tan²θ = 1)
2 sec θ = 5 + \(\frac{1}{5}\) = \(\frac{26}{5}\)
sec θ = \(\frac{26}{10}=\frac{13}{5}\)
Aquir 2 tan θ = 5 – \(\frac{1}{5}\) = \(\frac{24}{5}\)
tan θ = \(\frac{24}{10}=\frac{12}{5}\)
Now sin θ = \(\frac{\tan \theta}{\sec \theta}=\frac{\frac{12}{5}}{\frac{13}{5}}=\frac{12}{13}\)
tan θ is +ve, sec θ is +ve
⇒ θ lies in the first quadrant.

II.

Question 1.
Prove that
(i) \(\frac{\cos (\pi-A) \cdot \cot \left(\frac{\pi}{2}+A\right) \cos (-A)}{\tan (\pi+A) \tan \left[\frac{3 \pi}{2}+A\right] \sin (2 \pi-A)}\) = cos A
Solution:
\(\frac{\cos (\pi-A) \cdot \cot \left(\frac{\pi}{2}+A\right) \cos (-A)}{\tan (\pi+A) \tan \left[\frac{3 \pi}{2}+A\right] \sin (2 \pi-A)}\)
= \(\frac{-\cos A(-\tan A) \cos A}{\tan A(-\cot A)(-\sin A)}\)
= cos A

(ii) \(\frac{\sin (3 \pi-A) \cos \left(A-\frac{\pi}{2}\right) \tan \left(\frac{3 \pi}{2}-A\right)}{{cosec}\left(\frac{13 \pi}{2}+A\right) \sec (3 \pi+A) \cot \left(A-\frac{\pi}{2}\right)}\) = cos⁴A
Solution:

(iii) sin 780° sin 480° + cos 240°. cos 300° = \(\frac{1}{2}\)
Solution:
sin(2(360°) + 60°) . sin(360° + 120°) + cos(270° – 30°) . cos(360° – 60°)
= sin 60° . sin 120° – sin 30° . cos 60°
= \(\frac{\sqrt{3}}{2} \cdot \frac{\sqrt{3}}{2}-\frac{1}{2} \cdot \frac{1}{2}\)
= \(\frac{3}{4}-\frac{1}{4}\)
= \(\frac{1}{2}\)

(iv) \(\frac{\sin 150^{\circ}-5 \cos 300^{\circ}+7 \tan 225^{\circ}}{\tan 135^{\circ}+3 \sin 210^{\circ}}\) = -2
Solution:

(v) cot(\(\frac{\pi}{20}\)) . cot(\(\frac{3\pi}{20}\)) . cot(\(\frac{5\pi}{20}\)) . cot(\(\frac{7\pi}{20}\)) . cot(\(\frac{9\pi}{20}\)) = 1
Solution:
L.H.S. = cot(\(\frac{\pi}{20}\)) . cot(\(\frac{3\pi}{20}\)) . cot(\(\frac{5\pi}{20}\)) . cot(\(\frac{7\pi}{20}\)) . cot(\(\frac{9\pi}{20}\))
= cot (9°) cot (27°) cot (45°) cot (63°) cot (81°)
= cot (9°) cot (27°) (1) cot (90° – 27°) cot (90° – 9°)
= cot (9°) cot (27°) tan 27° tan 9°
= (tan 9° cot 9°) (tan 27° cot 27°)
= (1) (1)
= 1

Question 2.
Simplify.
(i) \(\frac{\sin \left(-\frac{11 \pi}{3}\right) \tan \left(\frac{35 \pi}{6}\right) \sec \left(-\frac{7 \pi}{3}\right)}{\cot \left(\frac{5 \pi}{4}\right) {cosec}\left(\frac{7 \pi}{4}\right) \cos \left(\frac{17 \pi}{6}\right)}\)
Solution:

(ii) If tan 20° = p, prove that \(\frac{\tan 610^{\circ}+\tan 700^{\circ}}{\tan 560^{\circ}-\tan 470^{\circ}}=\frac{1-p^{2}}{1+p^{2}}\)
Solution:
Given tan 20° = p
L.H.S. = \(\frac{\tan 610^{\circ}+\tan 700^{\circ}}{\tan 560^{\circ}-\tan 470^{\circ}}\)

(iii) If α, β are complementary angles such that b sin α = a, then find the value of (sin α cos β – cos α sin β).
Solution:
∵ α, β are complementary angles
⇒ α + β = 90°
⇒ β = 90° – α
Now sin α cos β – cos α sin β
= sin (α – β)
= sin [(α – (90° – α)]
= sin [2α – 90°]
= -sin (90° – 2α)
= -cos 2α
= -(1 – 2 sin²α) (∵ cos 2α = 1 – 2 sin²α)
= \(-1+2\left(\frac{a}{b}\right)^{2}\) (∵ sin α = \(\frac{a}{b}\))
= \(\frac{-b^{2}+2 a^{2}}{b^{2}}\)
= \(\frac{2 a^{2}-b^{2}}{b^{2}}\)

Question 3.
(i) If cos A = cos B = \(-\frac{1}{2}\), A does not lie in the second quadrant and B does not lie in the third quadrant, then find the value of \(\frac{4 \sin B-3 \tan A}{\tan B+\sin A}\)
Solution:
∵ cos A = \(-\frac{1}{2}\) and A does not lie in second quadrant.
⇒ A lies in the third quadrant, (∵ cos A is -ve)
and cos B = \(-\frac{1}{2}\) and B does not lie in third quadrant.
⇒ B lies in second quadrant.
∵ cos A = \(-\frac{1}{2}\) and A lies in third quadrant.
⇒ A = 240°
∵ cos B = \(-\frac{1}{2}\) and B lies in second quadrant.
⇒ B = 120°

(ii) If 8 tan A = -15 and 25 sin B = -7 and neither A nor B is in the fourth quadrant, then show that sin A cos B + cos A sin B = \(\frac{-304}{425}\)
Solution:
8 tan A = -15 ⇒ tan A = \(\frac{-15}{8}\)
25 sin B = -7 ⇒ sin B = \(-\frac{7}{25}\)
Given neither A nor B is in the fourth quadrant.
Clearly, A is the second quadrant B is the third quadrant
sin A cos B + cos A sin B = \(\left(\frac{15}{17}\right)\left(\frac{-24}{25}\right)+\left(\frac{-8}{17}\right)\left(\frac{-7}{25}\right)\)
= \(\frac{-360}{425}+\frac{56}{425}\)
= \(\frac{-304}{425}\)

(iii) If A, B, C, D are angles of a cyclic quadrilateral, then prove that
(a) sin A – sin C = sin D – sin B
(b) cos A + cos B + cos C + cos D = 0
Solution:
∵ A, B, C, D are angles of a cyclic quadrilateral.
⇒ A + C = 180° and B + D = 180° ……..(1)
C = 180° – A and D = 180° – B
(a) L.H.S. = sin A – sin C
= sin A – sin(180° – A)
= sin A – sin A
= 0
R.H.S. = sin D – sin B
= sin(180°- B) – sin B
= sin B – sin B
= 0
∴ L.H.S. = R.H.S.
i.e., sin A – sin C = sin D – sin B
(b) L.H.S. = cos A + cos B + cos C + cos D
= cos A + cos B + cos(180° – A) + cos(180° – B)
= cos A + cos B – cos A – cos B
= 0
∴ cos A + cos B + cos C + cos D = 0

Question 4.
(i) If a cos θ – b sin θ = c, then show that a sin θ + b sin θ = \(\pm \sqrt{a^{2}+b^{2}-c^{2}}\).
Solution:
a cos θ – b sin θ = c
let a sin θ + b cos θ = x
by squaring and adding
(a cos θ – b sin θ)² + (a sin θ + b cos θ)² = c² + x²
a² cos²θ + b² sin²θ – 2ab sin θ cos θ + a² sin²θ + b² cos²θ + 2ab sin θ cos θ = c² + x²
a² + b² = c² + x²
a² + b² – c² = x²
x = \(\pm \sqrt{a^{2}+b^{2}-c^{2}}\)
∴ a sin θ + b cos θ = \(\pm \sqrt{a^{2}+b^{2}-c^{2}}\)

(ii) If 3 sin A + 5 cos A = 5, then show that 5 sin A – 3 cos A = ±3.
Solution:
3 sin A + 5 cos A = 5
Let 5 sec A – 3 cos A = x
by squaring and adding
(3 sin A + 5 cos A)² + (5 sin A – 3 cos A)² = 5² + x²
9 sin² A + 25 cos² A + 30 sin A cos A + 25 sin² A + 9 cos² A – 30 sin A cos A = 25
9 + 25 = 25 + x²
x² = 9
x = ±3
∴ 5 sin A – 3 cos A = ±3

(iii) If tan²θ = (1 – e²), show that sec θ + tan³θ . cosec θ = (2 – e²)^3/2.
Solution:
tan²θ = 1 – e²
sec²θ = 1 + tan²θ = 2 – e²

III. Prove the following.

Question 1.
(i) \(\frac{(\tan \theta+\sec \theta-1)}{(\tan \theta-\sec \theta+1)}=\frac{1+\sin \theta}{\cos \theta}\)
Solution:

(ii) (1 + cot θ – cosec θ) (1 + tan θ + sec θ) = 2.
Solution:

(iii) 3(sin θ – cos θ)⁴ + 6(sin θ + cos θ)² + 4(sin⁶θ + cos⁶θ) = 13.
Solution:
(sin θ – cos θ)² = sin²θ + cos²θ – 2 sin θ . cos θ
= 1 – 2 sin θ cos θ
(sin θ – cos θ)⁴ = (1 – 2 sin θ cos θ)²
= 1 + 4 sin²θ cos²θ – 4 sin θ cos θ ……(1)
(sin θ + cos θ)² = sin²θ + cos²θ + 2 sin θ cos θ
= 1 + 2 sin θ cos θ …….(2)
sin⁶θ + cos⁶θ = (sin²θ +cos²θ)³ – 3 sin²θ cos²θ (sin²θ + cos²θ)
= 1 – 3 sin²θ cos²θ ……..(3)
L.H.S. = 3(1 + 4 sin²θ cos²θ – 4 sin θ cos θ) + 6(1 + 2 sin θ cos θ) + 4(1 – 3 sin²θ cos²θ)
= 3 + 12 sin²θ cos²θ – 12 sin θ cos θ + 6 + 12 sin θ cos θ + 4 – 12 sin²θ cos²θ
= 3 + 6 + 4
= 13
= R.H.S.

Question 2.
(i) Prove that (sin θ + cosec θ)² + (cos θ + sec θ)² – (tan²θ + cot²θ) = 7.
Solution:
L.H.S. = (sin θ + cosec θ)² + (cos θ + sec θ)² – (tan²θ + cot²θ)
= (sin²θ + cosec²θ + 2 sin θ cosec θ) + (cos²θ + sec²θ + 2 cos θ sec θ) – (tan²θ + cot²θ)
= (sin²θ + cos²θ) + (1 + cot²θ) + (1 + tan²θ) + 4 – tan²θ – cot²θ
= 1 + 1 + 1 + 4
= 7

(ii) cos⁴α + 2 cos²α \(\left(1-\frac{1}{\sec ^{2} \alpha}\right)\) = (1 – sin⁴α)
Solution:
L.H.S. = cos⁴α + 2 cos²α \(\left(1-\frac{1}{\sec ^{2} \alpha}\right)\)
= cos⁴α + 2 cos²α (1 – cos²α)
= cos²α [cos²α + 2 sin²α]
= (1 – sin²α) [cos²α + sin²α + sin²α]
= (1 – sin²α) (1 + sin²α)
= 1 – sin⁴α

(iii) \(\frac{(1+\sin \theta-\cos \theta)^{2}}{(1+\sin \theta+\cos \theta)^{2}}=\frac{1-\cos \theta}{1+\cos \theta}\)
Solution:

(iv) If \(\frac{2 \sin \theta}{(1+\cos \theta+\sin \theta)}\) = x, then find the value of \(\frac{(1-\cos \theta+\sin \theta)}{(1+\sin \theta)}\)
Solution:

Question 3.
Eliminate θ from the following.
(i) x = a cos³θ; y = b sin³θ
Solution:

(ii) x = a cos⁴θ; y = b sin⁴θ
Solution:

(iii) x = a(sec θ + tan θ); y = b(sec θ – tan θ)
Solution:
\(\frac{x}{a}\) = sec θ + tan θ; \(\frac{y}{b}\) = (sec θ – tan θ)
\(\frac{x}{a} \times \frac{y}{b}\) = (sec θ + tan θ) (sec θ – tan θ)
\(\frac{x y}{a b}\) = sec²θ – tan²θ
\(\frac{x y}{a b}\) = 1
xy = ab

(iv) x = cot θ + tan θ; y = sec θ – cos θ
Solution:

Practicing the Intermediate 1st Year Maths 1A Textbook Solutions Inter 1st Year Maths 1A Products of Vectors Solutions Exercise 5(c) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1A Products of Vectors Solutions Exercise 5(c)

I.

Question 1.
Compute \([\overline{\mathbf{i}}-\overline{\mathbf{j}} \overline{\mathbf{j}}-\overline{\mathbf{k}} \overline{\mathbf{k}}-\overline{\mathbf{i}}]\)
Solution:

Question 2.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-2 \overline{\mathbf{j}}-3 \overline{\mathbf{k}}, \overline{\mathbf{b}}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}}\), \(\bar{c}=\bar{i}+3 \bar{j}-2 \bar{k}\), then compute \(\overline{\mathbf{a}} \cdot(\overline{\mathbf{b}} \times \overline{\mathbf{c}})\).
Solution:

Question 3.
If \(\bar{a}\) = (1, -1, -6), \(\bar{b}\) = (1, -3, 4) and \(\bar{c}\) = (2, -5, 3), then compute the following
(i) \(\overline{\mathbf{a}} \cdot(\bar{b} \times \bar{c})\)
(ii) \(\overline{\mathbf{a}} \times(\overline{\mathbf{b}} \times \overline{\mathbf{c}})\)
(iii) \((\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}\)
Solution:

Question 4.
Simplify the following.
(i) \((\bar{i}-2 \bar{j}+3 \bar{k}) \times(2 i+j-\bar{k}) \cdot(\bar{j}+\bar{k})\)
(ii) \((2 \bar{i}-3 \bar{j}+\bar{k}) \cdot(\bar{i}-\bar{j}+2 \bar{k}) \cdot(2 \bar{i}+\bar{j}+\bar{k})\)
Solution:

Question 5.
Find the volume of the parallelopiped having coterminous edges.
\(\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}, \overline{\mathbf{i}}-\overline{\mathbf{j}}\) and \(\overline{\mathbf{i}}+\mathbf{2} \overline{\mathbf{j}}-\overline{\mathbf{k}}\)
Solution:
Let \(\overline{\mathrm{a}}=\overline{\mathrm{i}}+\overline{\mathrm{j}}+\overline{\mathrm{k}}, \overline{\mathrm{b}}=\overline{\mathrm{i}}-\overline{\mathrm{j}}\) and \(\overline{\mathrm{c}}=\overline{\mathrm{i}}+2 \overline{\mathrm{j}}-\overline{\mathrm{k}}\)
Volume of the parallelopiped = \([(\bar{a} \bar{b} \bar{c})]\)
= \(\left|\begin{array}{ccc}
1 & 1 & 1 \\
1 & -1 & 0 \\
1 & 2 & -1
\end{array}\right|\)
= 1(1 – 0) – 1(-1 – 0) + 1(2 + 1)
= 1 + 1 + 3
= 5 cubic units.

Question 6.
Find t for which the vectors \(\mathbf{2} \overline{\mathbf{i}}-\mathbf{3} \overline{\mathbf{j}}+\overline{\mathbf{k}}\), \(\bar{i}+2 \mathbf{j}-3 \bar{k}\) and \(\bar{j}-t \bar{k}\) are coplanar.
Solution:

Question 7.
For non-coplanar vectors, \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) determine p for which the vector \(\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}, \overline{\mathbf{a}}+\mathbf{p} \overline{\mathbf{b}}+\mathbf{2} \overline{\mathbf{c}}\) and \(-\overline{\mathbf{a}}+\overline{\mathbf{b}}+\overline{\mathbf{c}}\) are coplanar.
Solution:

Question 8.
Determine λ, for which the volume of the parallelopiped having coterminous edges \(\bar{i}+\bar{j}\), \(3 \overline{\mathbf{i}}-\overline{\mathbf{j}}\) and \(3 \bar{j}+\lambda \bar{k}\) is 16 cubic units.
Solution:

Question 9.
Find the volume of the tetrahedron having the edges \(\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}, \quad \mathbf{i}-\overline{\mathbf{j}}\) and \(\bar{i}+2 \bar{j}+\bar{k}\).
Solution:

Question 10.
Let \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) be non-coplanar vectors and \(\bar{\alpha}=\bar{a}+2 \bar{b}+3 c, \quad \bar{\beta}=2 \bar{a}+\bar{b}-2 c\) and \(\bar{\gamma}=3 \bar{a}-7 \bar{c}\), then find \(\left[\begin{array}{lll}
\bar{\alpha} & \bar{\beta} & \bar{\gamma}
\end{array}\right]\).
Solution:

Question 11.
Let \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) be non-coplanar vectors. If \(|2 \bar{a}-\bar{b}+3 \bar{c}|, \bar{a}+\bar{b}-2 \bar{c},|\bar{a}+\bar{b}-3 \bar{c}|\) = \(\lambda[\overline{\mathbf{a}} \overline{\mathbf{b}} \overline{\mathbf{c}}]\), then find the value of λ.
Solution:

Question 12.
Let \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) be non-coplanar vectors, if \(\left[\begin{array}{lll}
\bar{a}+2 \bar{b} & 2 \bar{b}+\bar{c} & 5 \bar{c}+\bar{a}
\end{array}\right]\) = \(\lambda\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]\), then find λ.
Solution:

Question 13.
If \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) are non-coplanar vectors, then find the value of \(\frac{(\bar{a}+2 \bar{b}-\bar{c}) \cdot[(\bar{a}-\bar{b}) \times(\bar{a}-\bar{b}-\bar{c})]}{[\bar{a} \bar{b} \bar{c}]}\)
Solution:

Question 14.
If \(\bar{a}\), \(\bar{b}\) and \(\bar{c}\) are mutually perpendicular unit vectors, then find the value of \(\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]^{2}\).
Solution:

Question 15.
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are non-zero vectors and \(\overline{\mathbf{a}}\) is perpendicular to both \(\overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\). If \(|\overline{\mathbf{a}}|\) = 2, \(|\overline{\mathbf{b}}|\) = 3, \(|\overline{\mathbf{c}}|\) = 4 and \((\bar{b}, \bar{c})=\frac{2 \pi}{3}\), then find \(|[\bar{a} \bar{b} \bar{c}]|\).
Solution:

Question 16.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are unit coplanar vectors, then find \(\left[\begin{array}{lll}
2 \bar{a}-\bar{b} & 2 \bar{b}-\bar{c} & 2 \bar{c}-\bar{a}
\end{array}\right]\)
Solution:

II.

Question 1.
If \(\left[\begin{array}{lll}
\bar{b} & \bar{c} & \bar{d}
\end{array}\right]+\left[\begin{array}{lll}
\bar{c} & \bar{a} & \bar{d}
\end{array}\right]+\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{d}
\end{array}\right]\) = \(\left[\begin{array}{lll}
\overline{\mathbf{a}} & \overline{\mathbf{b}} & \overline{\mathbf{c}}
\end{array}\right]\) then show that the points with position vectors \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) and \(\bar{d}\) are coplanar.
Solution:

Question 2.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\) non-coplanar vectors, then prove that the four points with position vectors \(2 \bar{a}+3 \bar{b}-\bar{c}\), \(\overline{\mathrm{a}}-2 \overline{\mathrm{b}}+3 \overline{\mathrm{c}}, 3 \overline{\mathrm{a}}+4 \overline{\mathrm{b}}-2 \overline{\mathrm{c}}\) and \(\bar{a}-6 \bar{b}+6 \bar{c}\) are coplanar.
Solution:
Suppose A, B, C, D are the given points.

The vectors \(\overrightarrow{\mathrm{AB}}, \overrightarrow{\mathrm{AC}}, \overrightarrow{\mathrm{AD}}\) are coplanar.
The given points A, B, C, D are coplanar.

Question 3.
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\) are non-zero and non- collinear vectors and θ ≠ 0, is the angle between \(\overline{\mathbf{b}}\) and \(\overline{\mathbf{c}}\). If \((\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}\) = \(\frac{1}{3}|\bar{b}||\bar{c}|\bar{a}|\), then find sin θ.
Solution:

Question 4.
Find the volume of the tetrahedron whose vertices are (1, 2, 1), (3, 2, 5), (2, -1, 0) and (-1, 0, 1).
Solution:
Let ‘O’ be the given A, B, C, D be the vertices of the ten tetrahedrons. Then

Question 5.
Show that \((\bar{a}+\bar{b}) \cdot(\bar{b}+\bar{c}) \times(\bar{c}+\bar{a})\) = \(2\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]\)
Solution:

Question 6.
Show that equation of the plane passing through the points with position vectors. \(3 \bar{i}-5 \bar{j}-\overline{\mathbf{k}},-\overline{\mathbf{i}}+5 \bar{j}+7 \overline{\mathbf{k}}\) and parallel to the vector \(3 \overline{\mathbf{i}}-\overline{\mathbf{j}}+7 \overline{\mathbf{k}}\) is 3x + 2y – z = 0.
Solution:
The given plane passes through the points A, B (i.e.,) \(3 \bar{i}-5 \bar{j}-\overline{\mathbf{k}},-\overline{\mathbf{i}}+5 \bar{j}+7 \overline{\mathbf{k}}\) and parallel to the vector \(3 \overline{\mathbf{i}}-\overline{\mathbf{j}}+7 \overline{\mathbf{k}}\)

= x(70 + 8) – y(-28 – 24) + z(4 – 30)
= 78x + 52y – 26z
= 26(3x + 2y – z)
\(\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]\) = \(\left|\begin{array}{rrr}
3 & -5 & -1 \\
-4 & 10 & 8 \\
3 & -1 & 7
\end{array}\right|\)
= 3(70 + 8) + 5(-28 – 24) – 1(4 – 30)
= 234 – 260 + 26
= 0
Equation of the required plane is 26(3x + 2y – z) = 0
i.e., 3x + 2y – z = 0

Question 7.
Prove that \(\overline{\mathbf{a}} \times[\overline{\mathbf{a}} \times(\overline{\mathbf{a}} \times \overline{\mathbf{b}})]\) = \((\overline{\mathbf{a}} \cdot \overline{\mathbf{a}})(\overline{\mathbf{b}} \times \overline{\mathbf{a}})\)
Solution:

Question 8.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) and \(\bar{d}\) are coplanar vectors, then show that \((\bar{a} \times \bar{b}) \times(\bar{c} \times \bar{d})=0\).
Solution:
\(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) and \(\bar{d}\) are coplanar
⇒ \(\overline{\mathbf{a}} \times \overline{\mathbf{b}}\) is perpendicular to the plane π.
similarly \(\bar{c} \times \bar{d}\) is perpendicular to the plane π.
\(\bar{a} \times \bar{b}\) and \(\bar{c} \times \bar{d}\) are parallel vectors.
⇒ \((\bar{a} \times \bar{b}) \times(\bar{c} \times \bar{d})\) = 0.

Question 9.
Show that \((\overline{\mathrm{a}} \times \overline{\mathrm{b}}) \times(\overline{\mathrm{a}} \times \overline{\mathrm{c}}) \cdot \overline{\mathrm{d}}=[\overline{\mathrm{a}} \overline{\mathrm{b}} \overline{\mathrm{c}}](\overline{\mathrm{a}} \cdot \overline{\mathrm{d}})\).
Solution:

Question 10.
Show that \(\bar{a} \cdot[(\bar{b}+\bar{c}) \times(\bar{a}+\bar{b}+\bar{c})]=0\)
Solution:

Question 11.
Find λ in order that the four points A(3, 2, 1), B(4, λ, 5), C(4, 2, -2) and D(6, 5, -1) be coplanar.
Solution:

Question 12.
Find the vector equation of the plane passing through the intersection of planes \(\bar{r} \cdot(2 \bar{i}+2 \bar{j}-3 \bar{k})=7, \bar{r} \cdot(2 \bar{i}+5 \bar{j}+3 \bar{k})=9\) and through the point (2, 1, 3)
Solution:

Question 13.
Find the equation of the plane passing through (a, b, c) and parallel to the plane \(\bar{r} \cdot(\bar{i}+\bar{i}+\bar{k})=2\).
Solution:
Given equation plane is \(\bar{r} \cdot(\bar{i}+\bar{i}+\bar{k})=2\)
Let \(\bar{r}=x \bar{i}+y \bar{j}+z \bar{k}\)
∴ \(\bar{r} \cdot(\bar{i}+\bar{i}+\bar{k})=2\)
\((x \bar{i}+y \bar{j}+z \bar{k}) \cdot(i+j+k)=2\)
x + y + z = 2
Required plane equation is x + y + z = k …….(1)
Equation (1) passes through (a, b, c)
∴ a + b + c = k
Substitute ‘k’ in equation (1)
∴ x + y + z = a + b + c

Question 14.
Find the shortest distance between the lines \(\bar{r}=6 \bar{i}+2 \bar{j}+2 \bar{k}+\lambda, \bar{i}-2 \bar{j}+2 \bar{k}\) and \(\bar{r}=-4 \bar{j}-\bar{k}+\mu=3 \bar{j}-2 \bar{j}-2 \bar{k}\).
Solution:
The first line passes through point A(6, 2, 2) and is parallel to the vector b = i – 2j + 2k.
Second line passes through the point C(-4, 0, -1) and is parallel to the vector d = 3i – 2j – 2k

Question 15.
Find the equation of the plane passing through the line of intersection of the planes \(\bar{r} \cdot(\bar{i}+\bar{j}+\bar{k})=1\) and \(\bar{r} \cdot(2 \bar{i}+3 \bar{i}-\bar{k})+4=0\) and parallel to X-axis.
Solution:
Given the equation of planes are

Since it is parallel to X-axis.

Question 16.
Prove that the four points \(4 \bar{i}+5 \bar{j}+\bar{k}\), \(-(\overline{\mathbf{j}}+\overline{\mathbf{k}}), 3 \overline{\mathbf{i}}+9 \overline{\mathbf{j}}+4 \overline{\mathbf{k}}\) and \(-4 \bar{i}+4 \bar{j}+4 \bar{k}\) are coplanar.
Solution:
Let ‘O’ be the origin. A, B, C, D be the given points. Then

Question 17.
If \(\bar{a}, \bar{b}, \bar{c}\) are non – copianar, then show that the vectors \(\overline{\mathbf{a}}-\overline{\mathbf{b}}, \overline{\mathbf{b}}+\overline{\mathbf{c}}\), \(\overline{\mathbf{c}}+\overline{\mathbf{a}}\) are coplanar.
Solution:

Question 18.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are the position vectors of the points A, B and C respectively, then prove that the vector \(\overline{\mathbf{a}} \times \overline{\mathbf{b}}+\overline{\mathbf{b}} \times \overline{\mathbf{c}}+\overline{\mathbf{c}} \times \overline{\mathbf{a}}\) is perpendicular to the plane of ∆ABC.
Solution:

III.

Question 1.
Show that \((\bar{a} \times(\bar{b} \times \bar{c}) \times \bar{c})=(\bar{a} \cdot \bar{c})(\bar{b} \times \bar{c})\) and \((\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \cdot(\overline{\mathbf{a}} \times \overline{\mathbf{c}})+(\overline{\mathbf{a}} \cdot \overline{\mathbf{b}})(\overline{\mathbf{a}} \cdot \overline{\mathbf{c}})\) = \((\overline{\mathbf{a}} \cdot \overline{\mathbf{a}})(\overline{\mathbf{b}} \cdot \overline{\mathbf{c}})\)
Solution:

Question 2.
If A = (1, -2, -1), B = (4, 0, -3), C = (1, 2, -1) and D = (2, -4, -5), find the distance between AB and CD.
Solution:

Question 3.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-2 \overline{\mathbf{j}}+\overline{\mathbf{k}}, \overline{\mathbf{b}}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\) and \(\overline{\mathbf{c}}=\overline{\mathbf{i}}+2 \overline{\mathbf{j}}-\overline{\mathbf{k}}\), find \(\bar{a} \times(\bar{b} \times \bar{c})\) and \(|(\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}|\).
Solution:

Question 4.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-2 \overline{\mathbf{j}}-3 \overline{\mathbf{k}}, \overline{\mathbf{b}}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}}\) and \(\bar{c}=\bar{i}+3 \bar{j}-2 \bar{k}\), verift that \(\overline{\mathbf{a}} \times(\overline{\mathbf{b}} \times \overline{\mathbf{c}}) \neq(\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}\)
Solution:

From (1) and (2), we get
\(\overline{\mathbf{a}} \times(\overline{\mathbf{b}} \times \overline{\mathbf{c}}) \neq(\overline{\mathbf{a}} \times \overline{\mathbf{b}}) \times \overline{\mathbf{c}}\)
i.e., vector multiplication is not associative.

Question 5.
If \(\overline{\mathbf{a}}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}-3 \overline{\mathbf{k}}, \overline{\mathbf{b}}=\overline{\mathbf{i}}-\mathbf{2} \mathbf{j}+\overline{\mathbf{k}}\), \(\bar{c}=-\bar{i}+\bar{j}-4 \bar{k}\) and \(\overline{\mathbf{d}}=\overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\), then compute \(|(\overline{\mathrm{a}} \times \overline{\mathrm{b}}) \times(\overline{\mathrm{c}} \times \overline{\mathrm{d}})|\)
Solution:

Question 6.
If A = (1, a, a²), B = (1, b, b²) and C = (1, c, c²) are non-coplanar vectors and \(\left|\begin{array}{lll}
a & a^{2} & 1+a^{3} \\
b & b^{2} & 1+b^{3} \\
c & c^{2} & 1+c^{3}
\end{array}\right|\) = 0, then show that abc + 1 = 0
Solution:
\(\bar{A}, \bar{B}, \bar{C}\) are non-coplanar vectors.
∆ = \(\left|\begin{array}{lll}
1 & a & a^{2} \\
1 & b & b^{2} \\
1 & c & c^{2}
\end{array}\right|\) ≠ 0 ………..(1)

∆ + (abc) ∆ = 0; ∆(1 + abc) = 0
∆ ≠ 0 ⇒ 1 + abc = 0

Question 7.
If \(\overline{\mathbf{a}}, \overline{\mathbf{b}}, \overline{\mathbf{c}}\) are non-zero vectors, then \(|(\overline{\mathbf{a}} \times \mathbf{b} \cdot \overline{\mathbf{c}})|=|\overline{\mathbf{a}}||\mathbf{b}||\overline{\mathbf{c}}|\) \(\Leftrightarrow \overline{\mathbf{a}} \cdot \overline{\mathbf{b}}=\overline{\mathbf{b}} \cdot \overline{\mathbf{c}}=\overline{\mathbf{c}} \cdot \overline{\mathbf{a}}=\mathbf{0}\)
Solution:

Question 8.
If \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-\mathbf{2} \overline{\mathbf{j}}+3 \overline{\mathbf{k}}, \quad \mathbf{b}=2 \overline{\mathbf{i}}+\overline{\mathbf{j}}+\overline{\mathbf{k}}\), \(\bar{c}=\overline{\mathbf{i}}+\overline{\mathbf{j}}+2 \overline{\mathbf{k}}\) then find \(|(\bar{a} \times \bar{b}) \times \bar{c}|\) and \(|\overline{\mathbf{a}} \times(\mathbf{b} \times \mathbf{c})|\).
Solution:

Question 9.
If \(|\bar{a}|=1,|\bar{b}|=1,|\bar{c}|=2\) and \(\bar{a} \times(a \times \bar{c})+\bar{b}=0\) then find the angle between \(\bar{a}\) and \(\bar{c}\).
Solution:

Question 10.
Let \(\overline{\mathbf{a}}=\overline{\mathbf{i}}-\overline{\mathbf{k}}, \quad \overline{\mathbf{b}}=\mathbf{x} \overline{\mathbf{i}}+\overline{\mathbf{j}}+(\mathbf{1}-\mathbf{x}) \overline{\mathbf{k}}\) and \(\bar{c}=y \bar{i}+x \bar{j}+(1+x-y) \bar{k}\), prove that the scalar triple product \(\left[\begin{array}{lll}
\bar{a} & \bar{b} & \bar{c}
\end{array}\right]\) is independent of both x and y.
Solution:

Question 11.
Let \(\overline{\mathbf{b}}=\mathbf{2} \overline{\mathbf{i}}+\overline{\mathbf{j}}-\overline{\mathbf{k}}, \overline{\mathbf{c}}=\overline{\mathbf{i}}+\mathbf{3} \overline{\mathbf{k}}\). If \(\overline{\mathrm{a}}\) is a unit vector then find the maximum value of \(\left[\begin{array}{lll}
\overline{\mathbf{a}} & \overline{\mathbf{b}} & \bar{c}
\end{array}\right]\).
Solution:
Let \(\bar{a}=x \bar{i}+y \bar{j}+z \bar{k}\) and x² + y² + z² = 1
∵ \(\overline{\mathrm{a}}\) unit vector

Question 12.
Let \(\overline{\mathrm{a}}=\overline{\mathrm{i}}-\overline{\mathrm{i}}, \overline{\mathrm{b}}=\overline{\mathrm{i}}-\overline{\mathrm{k}}, \overline{\mathrm{c}}=\overline{\mathrm{k}}-\overline{\mathrm{i}}\) Find unit vector \(\bar{d}\) such that \(\overline{\mathrm{a}} \cdot \overline{\mathrm{d}}=0=[\bar{b} \overline{\mathrm{c}} \overline{\mathrm{d}}]\)
Solution: