AP SSC 10th Class Maths Solutions Chapter 3 Polynomials Ex 3.4

AP State Board Syllabus AP SSC 10th Class Maths Textbook Solutions Chapter 3 Polynomials Ex 3.4 Textbook Questions and Answers.

AP State Syllabus SSC 10th Class Maths Solutions 3rd Lesson Polynomials Exercise 3.4

10th Class Maths 3rd Lesson Polynomials Ex 3.4 Textbook Questions and Answers

Question 1.
Divide the polynomial p(x) by the polynomial g(x) and find the quotient and remainder in each of the following:
i) p(x) = x³ – 3x² + 5x – 3, g(x) = x² – 2
ii) p(x) = x⁴ – 3×2 + 4x + 5, g(x) = x² + 1 – x
iii) p(x) = x⁴ – 5x + 6, g(x) = 2 – x²
Answer:
i) Given polynomials are
p(x) = x³ – 3x² + 5x – 3 and
g(x) = x² – 2
Here, dividend and divisor are both in standard forms.
So, we have

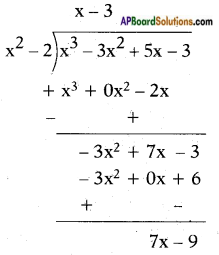
∴ The quotient is x – 3 and the remainder is 7x – 9.

ii) Given polynomials are
p{x) = x⁴ – 3x² + 4x + 5 and
g(x) = x² + 1 – x
Here, the dividend is already in the standard form and the divisor is not in the standard form. It can be written as x² – x + 1.
We have,

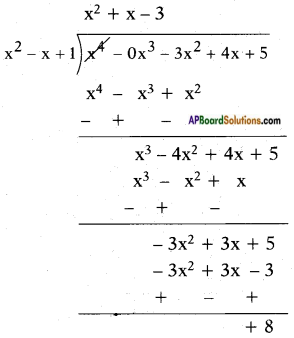
∴ The quotient is x² + x – 3 and the remainder is +8.

iii) Given polynomials are
p(x) = x⁴ – 5x + 6 and
g(x) = 2 – x²
Here, the dividend is already in the standard form and the divisor is not in the standard form. It can be written as -x² + 2.
So, we have

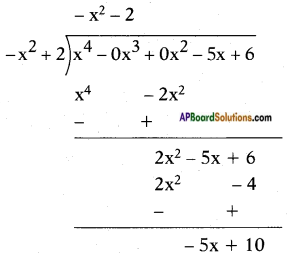
∴ The quotient is -x² – 2 and the remainder is -5x + 10.

Question 2.
Check in which case the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial:
i) t² – 3, 2t⁴ + 3t³ – 2t² – 9t – 12
ii) x² + 3x + 1, 3x⁴ + 5x³ – 7x² + 2x + 2
iii) x³ – 3x + 1, x⁵ – 4x³ + x² + 3x + 1
Answer:
i) Given first polynomial is t² – 3.
Second polynomial is
2t⁴ + 3t³ – 2t² – 9t – 12.
Let us divide 2t⁴ + 3t³ – 2t² – 9t – 12 by t² – 3, we get

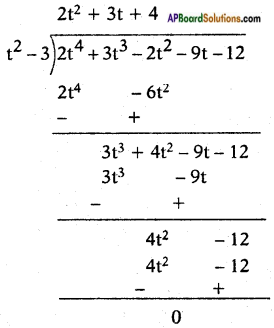
Since the remainder is 0, therefore, t² – 3 is a factor of 2t⁴ + 3t³ – 2t² – 9t – 12.

ii) Given first polynomial is x² + 3x + 1
Second polynomial is 3x⁴ + 5x³ – 7x² + 2x + 2
Let us divide 3x⁴ + 5x³ – 7x² + 2x + 2 by x² + 3x + 1, we get

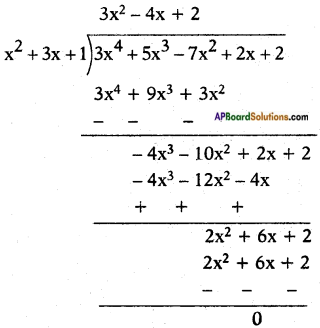
Since the remainder is 0, therefore x² + 3x + 1 is a factor of 3x⁴ + 5x³ – 7x² + 2x + 2.

iii) Given first polynomial = x³ – 3x + 1
Second polynomial = x⁵ – 4x³ + x² + 3x + 1
Let us divide x⁵ – 4x³ + x² + 3x + 1 by x³ – 3x + 1, we get

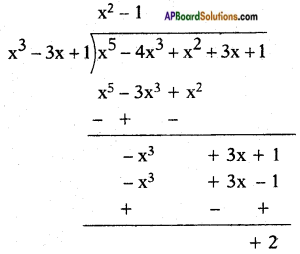
Here, remainder is 2(≠ 0).
Therefore, x³ – 3x + 1 is not a factor of x⁵ – 4x³ + x² + 3x + 1.

Question 3.
Obtain all other zeroes of 3x⁴ + 6x³ – 2x² – 10x – 5, if two of its zeroes are \(\sqrt{\frac{5}{3}}\) and –\(\sqrt{\frac{5}{3}}\).
Answer:
Let the other two zeroes are α and β.
Now compare the given polynomial 3x⁴ + 6x³ – 2x² – 10x – 5 with the standard form ax⁴ + bx³ + cx² + dx + e we get a = 3, b = 6, c = -2, d = -10, e = -5

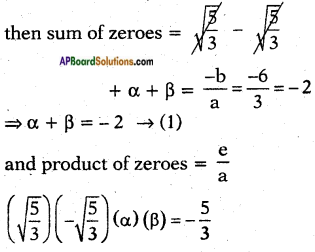
–\(\frac{5}{3}\)αβ = \(\frac{-5}{3}\) ⇒ αβ = 1
now (α – β)² = (α + β)² – 4αβ
= (-2)² – 4(1)
= 4 – 4 = 0
α – β = 0 …. (2)
Now solving (1) and (2) we get

⇒ α = -1, β = -1
Then the remaining the zeroes are -1 and -1.
Hence all zeroes of it = –\(\sqrt{\frac{5}{3}}\), \(\sqrt{\frac{5}{3}}\), -1, -1.

Question 4.
On dividing x³ – 3x² + x + 2 by a polynomial g(x), the quotient and remainder were x – 2 and -2x + 4, respectively. Find g(x).
Answer:
Given, p(x) = x³ – 3x² + x + 2
q(x) = x – 2 and
r(x) = -2x + 4
By division algorithm, we know that Dividend = Divisor × Quotient + Remainder
p(x) = q(x) × g(x) + r(x)
Therefore, x³ – 3x² + x + 2
= (x – 2) × g(x) + (- 2x + 4)
⇒ x³ – 3x² + x + 2 + 2x – 4 = (x – 2) × g(x)
g(x) = \(\frac{x^{3}-3 x^{2}+3 x-2}{x-2}\)
On dividing x³ – 3x² + x + 2, by x – 2, we get

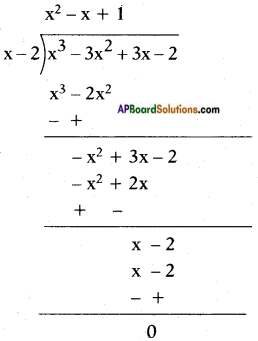
First term of g(x) = \(\frac{\mathrm{x}^{3}}{\mathrm{x}}\) = x²
Second term of g(x) = \(\frac{-x^{2}}{x}\) = -x
Third term of g(x) = \(\frac{x}{x}\) = 1
Hence, g(x) = x² – x + 1.

Question 5.
Give examples of polynomials p(x), g(x), q(x) and r(x), which satisfy the division algorithm and
i) deg p(x) = deg q(x)
ii) deg q(x) = deg r(x)
iii) deg r(x) = 0
Answer:
Let q(x) = 3x² + 2x + 6, degree of q(x) = 2
p(x) = 12x² + 8x + 24, degree of p(x) = 2
Given degree p(x) = degree q(x)
i) Using division algorithm,
We gave, p(x) = q(x) × g(x) + r(x)
On dividing 12x² + 8x + 24 by 3x² + 2x + 6, we get

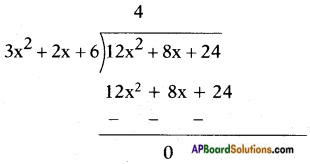
Since, the remainder is zero, therefore 3x² + 2x + 6 is a factor of 12x² + 8x + 24.
∴ g(x) = 4 and r(x)= 0

ii) Let p(x) = x⁵ + 2x⁴ + 3x³ + 5x² + 2
q(x) = x² + x + 1, degree q(x) = 2
Given degree q(x) = degree r(x)
On dividing x⁵ + 2x⁴ + 3x³ + 5x² + 2 by x² + x + 1, we get

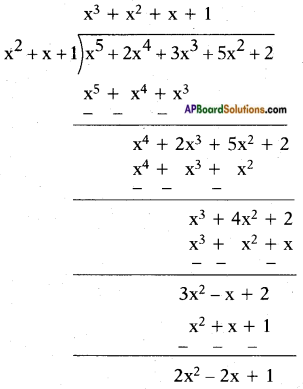
Here, g(x) = x³ + x² + x + 1 and r(x) = 2x² – 2x + 1
degree of r(x) = 2.
∴ deg g(x) = deg r(x).

iii) Let p(x) = 2x⁴ + 8x³ + 6x² + 4x + 12, r(x) = 2
Here, degree r(x) = 0
On dividing 2x⁴ + 8x³ + 6x² + 4x + 12 by 2, we get

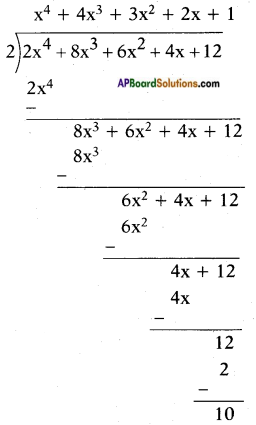
Here, g(x) = x⁴ + 4x³ + 3x² + 2x + 1 and r(x) = 10
so degree of r(x) = 0