Inter 2nd Year Maths 2B Integration Solutions Ex 6(f)

Practicing the Intermediate 2nd Year Maths 2B Textbook Solutions Inter 2nd Year Maths 2B Integration Solutions Exercise 6(f) will help students to clear their doubts quickly.

Intermediate 2nd Year Maths 2B Integration Solutions Exercise 6(f)

I. Evaluate the following integrals.

Question 1.
∫e^x(1 + x²) dx
Solution:
∫e^x(1 + x²) dx = ∫e^x + ∫x² . e^x dx
= e^x + (x².e^x – 2 ∫x.e^xdx)
= e^x + x².e^x – 2(x.e^x – ∫e^x dx
= e^x + x².e^x – 2x. e^x + 2e^x + C
= e^x(x² – 2x + 3) + C

Question 2.
∫x² e^-3x dx
Solution:

Question 3.
∫x³ e^ax dx
Solution:

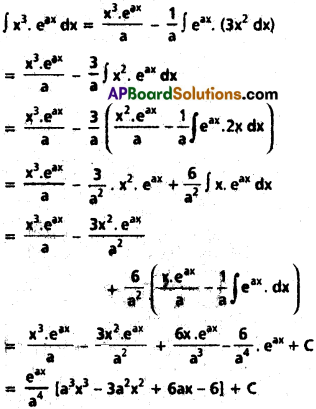
II.

Question 1.
Show that
∫xⁿ.e^-x dx = -xⁿ e^-x + n∫x^n-1.e^-x dx
Solution:
∫xⁿ.e^-x dx = \(\frac{x^ne^{-x}}{(-1)}\) + ∫e^-x. nx^n-1 dx
= -xⁿ . e^-x + n∫x^n-1e^-x dx

Question 2.
If I_n = ∫cosⁿ x dx, than show that
I_n = \(\frac{1}{n}\) cos^n-1 x sin x + \(\frac{n-1}{n}\) I_n-2.
Solution:
I_n = ∫cosⁿ x dx = ∫cos^n-1 x. cos x dx
= cos^n-1x . sin x – ∫sin x.(n – 1)cos^n-2x (-sin x)dx
= cos^n-1x . sin x + (n – 1) ∫cos^n-2x(1 – cos² x)dx
= cos^n-1x . sin x + (n – 1)I_n-2 – (n – 1) I_n
∴ I_n(1 + n – 1) = cos^n-1x. sin x + (n – 1) I_n-2
I_n = \(\frac{\cos ^{n-1} x \sin x}{n}+\frac{n-1}{n}\)I_n-2

III.

Question 1.
Obtain reduction formula for I_n = ∫cotⁿ x dx, n being a positive integer, n ≥ 2 and deduce the value of ∫cot⁴ x dx.
Solution:
I_n = ∫cotⁿ dx = ∫cot^n-2 x . cot² x dx
= ∫cot^n-2 x . (cosec² x – 1) dx
= ∫cot^n-2 x . cosec² x dx – I_n-2
= – \(\frac{cot^{n-1}x}{n-1}\) – I_n-2
n = 4 ⇒ I₄ = –\(\frac{cot^{3}x}{3}\) – I₂
n = 2 ⇒ I₂ = – cot x – I₀ where I₀ = ∫dx = x
I₂ = – cot x – x
I₄ = –\(\frac{cot^{3}x}{3}\) – (-cot x – x) + C
= –\(\frac{cot^{3}x}{3}\) + cot x + x + C

Question 2.
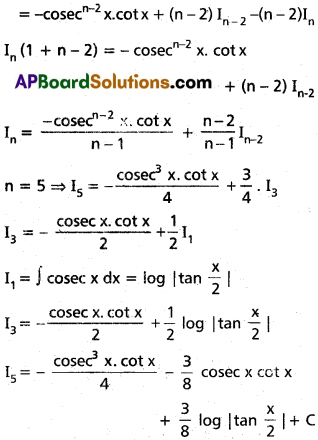
Obtain the reduction formula for I_n = ∫cosecⁿ x dx, n being a positive integer, n ≥ 2 and deduce the value of ∫cosec⁵ x dx.
Solution:
I_n = ∫cosecⁿ x dx
= cosec^n-2 x(-cot x) + ∫cot x. (n – 2)cosec^n-3 x. (cot x)dx
= -cosec^n-2x. cot x + (n – 2)∫cosec^n-2x. (cosec² x – 1)dx

Question 3.
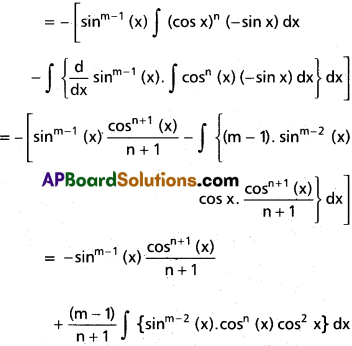
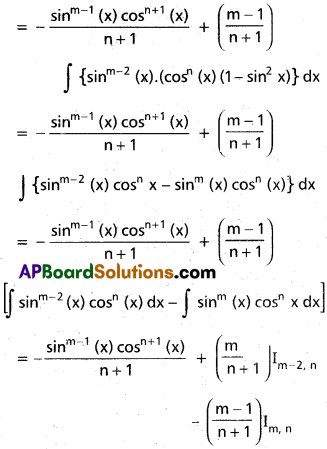
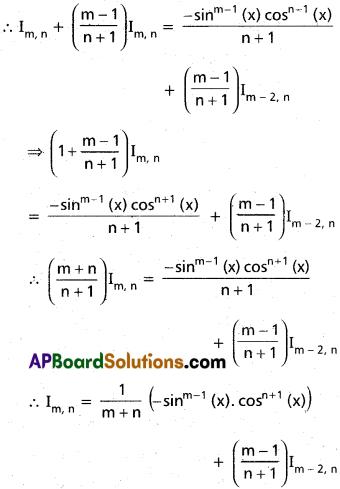
If I_{m, n} = ∫sin^m xcosⁿ xdx, then show that
for a positive integer m ≥ 2.
Solution:
I_{m, n} = ∫(sin^m x) (cosⁿ x) dx
= ∫sin^m-1 (x).(cos x)ⁿ. sin x dx
= ∫sin^m-1 (x)(cos x)ⁿ(-sin x) dx

Question 4.
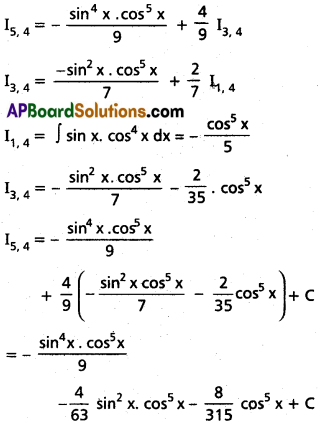
Evaluate ∫sin⁵ x cos⁴ x dx
Solution:
Reduction formula
I_{m, n} = \(\frac{-\sin ^{m-1} x \cdot \cos ^{n+1} x}{m+n}+\frac{m-1}{m+n}\).I_{m-2, n}

 

Question 5.
If I_n = ∫(log x)ⁿ dx, then show that I_n = x(log x)ⁿ – nI_n-1, and hence find
∫(log x)⁴ dx.
Solution:
I_n = ∫(log x)ⁿ dx
= (log x)ⁿ. x – ∫x . n . (log x)^n-1 . \(\frac{1}{x}\)dx
= x.(log x)ⁿ – n∫(log x)^n-1 dx
= x(log x)ⁿ – n . I_n-1
I₄ = x(log x)⁴ – 4 . I₃
I₃ = x(log x)³ – 3 . I₂
I₂ = x(log x)² – 2 . I₁
I₁ = x log x I₀ where I₀ = ∫dx = x
I₁ = x log x – x
I₂ = (x (log x)² – 2x log x = 2x
I₃ = x(log x)³ – 3(x (log x)² – 2x log x + 2x)
= x . (log x)³ – 3x (log x)² + 6x(log x) – 6x
I₄ = x(log x)⁴ – 4[x . (log x)³ – 3x(log x)² + 6x(log x) -6x] + C
= x[(log x)⁴ – 4(log x)³ + 12(log x)² – 24(log x) + 24] + C