Inter 2nd Year Maths 2B Ellipse Solutions Ex 4(b)

Practicing the Intermediate 2nd Year Maths 2B Textbook Solutions Inter 2nd Year Maths 2B Ellipse Solutions Exercise 4(b) will help students to clear their doubts quickly.

Intermediate 2nd Year Maths 2B Ellipse Solutions Exercise 4(b)

I.

Question 1.
Find the equation of tangent and normal to the ellipse x + 8y = 33 at (-1, 2).
Solution:
Equation of the tangent is
\(\frac{xx_1}{a^2}+\frac{yy_1}{b^2}\)
x(-1) + 8y(2) = 33
⇒ -x +16y = 33
⇒ x – 16y + 33 = 0
Equation of the normal is
16x + y + k = 0
It passes through P(-1, 2)
-16 + 2 + k = 0 ⇒ k =14
Equation of the normal is 16x + y + 14 = 0.

Question 2.
Find the equation of tangent and normal to the ellipse
x² + 2y² – 4x + 12y + 14 = 0 at (2, – 1).
Solution:
xx₁ + 2yy₁ – 2(x + x₁) + 6(y + y₁) + 14 = 0
⇒ 2x – 2y – 2(x + 2) + 6(y – 1) + 14 = 0
⇒ 4y + 4 = 0
y = – 1 required equation of tangent.
Slope of tangent is ‘0’
Equation of normal be
y + 1 = \(\frac{-1}{0}\) (x – 2)
x = 2 equation of normal.

Question 3.
Find the equation of the tangents to 9x² + 16y² = 144, which makes equal intercepts on the co-ordianate axis.
Solution:
Equation of the ellipse is 9x² + 16y² = 144
⇒ \(\frac{x^2}{16}+\frac{y^2}{9}\) = 1
Equation of the tangent is
\(\frac{x}{a}\). cos θ + \(\frac{y}{b}\) sin θ = 1
Slope of the tangent = –\(\frac{b \cos \theta}{a \sin \theta}\) = -1
cot θ = \(\frac{a}{b}=\frac{4}{3}\)
cos θ = ± \(\frac{4}{5}\), sin 0 = ± \(\frac{3}{5}\)
Equation of the tangent is
\(\frac{x}{4}\)(±\(\frac{4}{5}\)) + \(\frac{y}{3}\)(±\(\frac{3}{5}\)) = 1
x ± y ± 5 = 0.

Question 4.
Find the co-ordinates for the points on the ellipse x² + 3y² = 37 at which the normal is parallel to the line 6x – 5y = 2.
Solution:
Equation of the ellipse is x² + 3y² = 37
⇒ \(\frac{x^2}{37}+\frac{y^2}{\left(\frac{37}{3}\right)}\) = 1
a² = 37, b² = \(\frac{37}{3}\)
Slope of the normal =

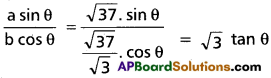
The normal is parallel to 6x – 5y = 2

Case i) The co-ordinates of P are
(a cos θ, b sin θ)

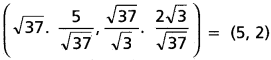
Case ii) The co-ordinates of P are (a cos θ, b sin θ)

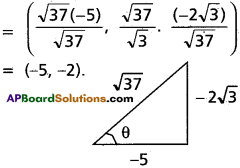
Question 5.
Find the value of k if 4x+y + k = 0isa tangent to the ellipse x² + 3y² = 3.
Solution:
Equation of the ellipse is x² + 3y² = 3
\(\frac{x^2}{3}+\frac{y^2}{1}\) = 1
a² = 3, b² = 1
Equation of the line is 4x + y + k = 0
y = -4x – k
m = -4, c = -k.
Condition for tangency is c² = a² m² + b²
(-k)² = 3(-4)² + 1
k²= 48 + 1 = 49
k = ±7.

Question 6.
Find the condition for the line x cos α + y sin α = p to be a tangent to the ellipse \(\frac{x^2}{a^2}+\frac{y^2}{b^2}\) = 1.
Solution:
Equation of the ellipse is
\(\frac{x^2}{a^2}+\frac{y^2}{b^2}\) ……….. (1)
Equation of the line is x cos α + y sin α = p
y sin α = – x cos α + p cos α p

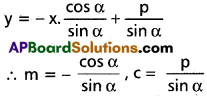
Condition for tangency is c² = a² m² + b²

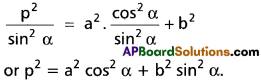
II.

Question 1.
Find the equations of tangent and normal to the ellipse 2x² + 3y² = 11 at the point whose ordinate is 1.
Solution:
Equation of the ellipse is 2x² + 3y² = 11
Given y = 1
2×2 + 3 = 11 ⇒ 2x² = 8
x² = 4
x = ±2
Points on the ellipse are P (2, 1) and
Q(-2, 1)

Case i)P (2, 1)
Equation of the tangent is 2x.2 + 3y.1 = 11
4x + 3y = 11
The normal is perpendicular to the tangent Equation of the normal at P can be taken as 3x – 4y = k.
The normal passes through P (2, 1)
6 – 4 = k ⇒ k = 2
Equation of the normal at P is 3x – 4y = 2.

Case ii) Q (-2, 1)
Equation of the tangent at Q is
2x(-2) + 3y.1 = 11
– 4x + 3y = 11
4x – 3y + 11 = 0
Equation of the normal can be taken as
3x + 4y = k
The normal passes through Q (-2, 1)
-6 + 4 = k ⇒ k = -2
Equation of the normal at Q is 3x + 4y = -2
or 3x + 4y + 2 = 0.

Question 2.
Find the equations to the tangents to the ellipse, x² + 2y² = 3 drawn from the point (1, 2) and also find the angle between these tangents.
Solution:
Equations of the ellipse is x² + 2y² = 3
⇒ \(\frac{x^2}{3}+\frac{y^2}{\left(\frac{3}{2}\right)}\) = 1
a² = 3, b² = \(\frac{3}{2}\)
Suppose m is the slope of the tangent. It passes through P (1, 2)
Equation of the tangent is

y – 2 = m(x – 1)
= mx – m
y = mx + (2 – m)
Condition for tangency is c² = a²m² + b²
(2 – m)² = 3(m²) + \(\frac{3}{2}\)
4 + m² – 4m = 3m² + \(\frac{3}{2}\)
2m² + 4m – \(\frac{5}{2}\)
4m² + 8m – 5 = 0
(2m – 1) (2m + 5) = 0
m = \(\frac{1}{2}\) or –\(\frac{5}{2}\)

Case i) m = \(\frac{1}{2}\)
Equation of the tangent is y = \(\frac{1}{2}\) x + 2 – \(\frac{1}{2}\)
= \(\frac{x}{2}+\frac{3}{2}\)
2y = x + 3
x- 2y + 3 = 0

Case ii) m = –\(\frac{5}{2}\)
Equation of the tangent is
y = – \(\frac{5}{2}\)x + (2 + \(\frac{5}{2}\))
= –\(\frac{5}{2}\)x + \(\frac{9}{2}\)
2y = – 5x + 9
or 5x + 2y – 9 = 0
Angle between the tangents is given by

Question 3.
Fincbthe equation of tangents to the ellipse 2x² + y² = 8 which are
i) Parallel to x – 2y – 4 = 0
Solution:
Slope will be : \(\frac{1}{2}\)
Equation of tangent y = mx ± \(\sqrt{a^2m^2+b^2}\)

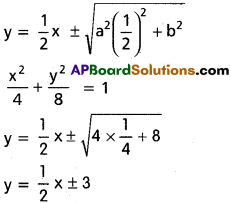
2y – x + 6 = 0 required equation of tangents,
x – 2y ± 6 = 0.

ii) Perpendicular to x + y + 2 = 0
Solution:
Slope of tangent be ‘1’ as it is perpendicular to above line
y = mx ± \(\sqrt{a^2m^2+b^2}\)
y = x ± \(\sqrt{4+8}\)
y = x ± 2√3
⇒ x – y ± 2√3 = 0.

iii) Which makes an angle \(\frac{\pi}{4}\) with x-axis.
Solution:
Equation of tangent y = x ± 2√3.
⇒ x – y ± 2√3 = 0.

Question 4.
A circle of radius 4, is concentric with the ellipse 3x² + 13y² = 78. Prove that a common tangent is inclined to the major axis at an angle \(\frac{\pi}{4}\).
Solution:
Equation of the ellipse is 3x² + 13y² = 78
\(\frac{x^2}{26}+\frac{y^2}{6}\) = 1 ……… (1)
Centre of the ellipse is (0, 0)
Equation of the circle is x² + y² = 16 ………. (2)
Equation of tangent at P(θ) to the circle is
x cos θ + y sin θ = 16 ……. (3)

(3) is a tangent to the ellipse.

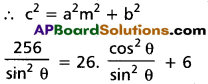
16 = 26 cos² θ + 6 sin² θ
= 26 cos² θ + 6(1 – cos² θ)
= 26 cos² θ + 6-6 cos² θ
20 cos² θ = 10
cos² θ = \(\frac{10}{20}=\frac{1}{2}\)
cos θ = ± \(\frac{1}{\sqrt{2}}\)
θ = \(\frac{\pi}{4}\)

III.

Question 1.
Show that the foot of the perpendicular drawn from the centre on any tangent to the ellipse lies on the curve
(x² + y²)² = a²x² + b²y².
Solution:
Equation of the ellipse is \(\frac{x^2}{a^2}+\frac{y^2}{b^2}\) = 1
Equation of the tangent at P (θ) is
\(\frac{x}{a}\) cos θ + \(\frac{y}{b}\) sin θ = 1

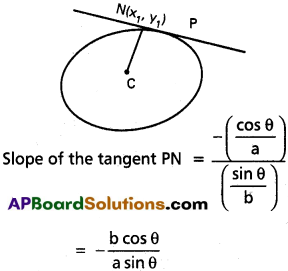
CN is the perpendicular from C on the tangent slope of CN = \(\frac{y_1}{x_1}\)
∴ Slope of PT × slope of CN = -1


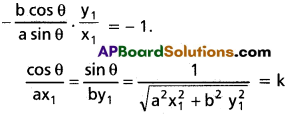
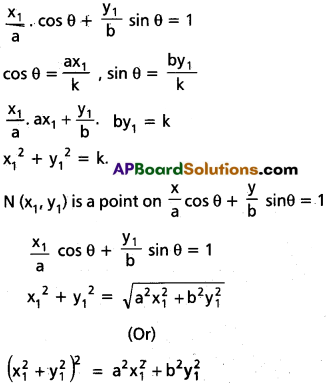
Locus of N (xv y,) is (x² + y²)² = a²x² + b²y²

Question 2.
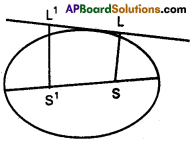
Show that the locus of the feet of the perpendiculars drawn from foci to any tangent of the ellipse is the auxiliary circle.

Solution:
Hint : Equation of the ellipse is \(\frac{x^2}{a^2}+\frac{y^2}{b^2}\)
Equation of the tangent to the ellipse is
y = mx ± \(\sqrt{a^2m^2+b^2}\)
⇒ y – mx = ± \(\sqrt{a^2m^2+b^2}\) ………… (1)
Equation to the perpendicular from either focus (+ae, 0) on this tangent is
y = – \(\frac{1}{m}\) (x ± ae)
my = -(x ± ae)
my + x = ± ae ……….. (2)
Squaring and adding (1) and (2)
(y – mx)² + (my + x)² = a²m² + b² + a²e²
y² + m²x² – 2mxy + m²y² + x² + 2mxy = a²m² + a² – a²e² + a²e²
(x² + y²) (1 + m²) = a² (1 + m²)
⇒ x² + y² = a²
The locus is the auxiliary circle concentric with the ellipse.

Question 3.
The tangent and normal to the ellipse x² + 4y² = 4 at a point P(θ) on it meets the major axis in Q and R respectively. If 0 < θ < \(\frac{x}{2}\) and QR = 2, then show that θ = cos^–\(\frac{2}{3}\)
Solution:

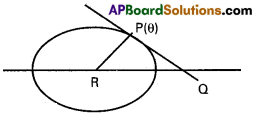
Equation of the ellipse is x² + 4y² = 4
\(\frac{x^2}{4}+\frac{y^2}{1}\) = 1
Equation of the tangent at P (θ) is
\(\frac{x}{2}\).cos θ + \(\frac{y}{1}\) sin θ = 1
Equation of x-axis is y = 0
\(\frac{x}{2}\) cos θ = 1 ⇒ x = \(\frac{2}{\cos\theta}\)
Co-ordinates of Q are (\(\frac{2}{\cos\theta}\), θ)
Equation of the normal at
P(θ) is \(\frac{ax}{\cos\theta}-\frac{by}{\sin\theta}\) = a² – b²
\(\frac{ax}{\cos\theta}-\frac{by}{\sin\theta}\) = 3
Substituting y = 0 we get \(\frac{2x}{\cos\theta}\) = 3
x = \(\frac{3}{2}\). cos θ
Co-ordinates of R are (\(\frac{3}{2}\). cos θ, o)

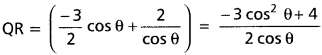
Given QR = 2
\(\frac{-3 \cos ^2 \theta+4}{2 \cos \theta}\) = 2
-3 cos² θ+ 4 = 4 cos θ
3 cos² θ + 4 cos θ – 4 = 0
(3 cos θ – 2) ( cos θ + 2) = 0
3 cos θ – 2 = 0 or cos θ + 2 = 0
cos θ = \(\frac{2}{3}\) or cos θ = – 2
cos θ always lies between -1 and 1
∴ cos θ = \(\frac{2}{3}\)
i.e., θ = cos^-1(\(\frac{2}{3}\))