AP SSC 10th Class Maths Solutions Chapter 1 Real Numbers Optional Exercise

AP State Board Syllabus AP SSC 10th Class Maths Textbook Solutions Chapter 1 Real Numbers Optional Exercise Textbook Questions and Answers.

AP State Syllabus SSC 10th Class Maths Solutions 1st Lesson Real Numbers Optional Exercise

10th Class Maths 1st Lesson Real Numbers Optional Exercise Textbook Questions and Answers

Question 1.
Can the number 6ⁿ, n being a natural number, end with the digit 5? Give reason.
Answer:
Given number = 6ⁿ ; n ∈ N
6ⁿ to be end in 5; it should be divisible by 5
6ⁿ = (2 × 3)ⁿ
The prime factors of 6ⁿ are 2 and 3.
It can’t end with the digit 5.

Question 2.
Is 7 × 5 × 3 × 2 + 3 a composite number? Justify your answer.
Answer:
Given:
7 × 5 × 3 × 2 + 3
= 3 (7 × 5 × 2 + 1)
= 3 × (70 + 1)
= 3 × 71
∴ The given number has two factors namely 3 and 71.
Hence it is a composite number.

Question 3.
Prove that (2√3 + √5 ) is an irrational number. Also check whether (2√3 + √5) (2√3 – √5) is rational or irrational.
Answer:
To prove:
2√3 + √5 is an irrational number. On contrary, let us suppose that 2√3 + √5 be a rational number.
Then 2√3 + √5 = \(\frac{p}{q}\)
Squaring on both sides, we get


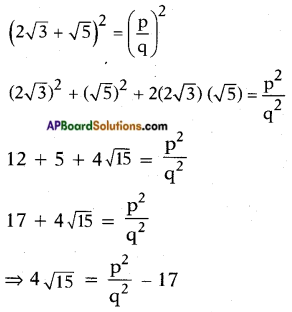
L.H.S = an irrational number.
R.H.S = p, q being integers, \(\frac{p^{2}-17 q^{2}}{4 q^{2}}\) is a rational number.
This is a contradiction to the fact that √l5 is an irrational. This is due to our assumption that 2√3 + √5 is a rational. Hence our assumption is wrong and 2√3 + √5 is an irrational number. Also,
(2√3 + √5) (2√3 – √5)
= (2√3)² – (√5)²
[∵ (a + b) (a – b) = a² – b²]
= 4 × 3 – 5
= 12 – 5 = 7, a rational number.

Question 4.
If x² + y² = 6xy, prove that 2 log (x + y) = log x + log y + 3 log 2.
Answer:
Given: x² + y² = 6xy
x² + y² + 2xy = 6xy + 2xy
(x + y)² = 8xy
Taking logarithms on both sides log (x + y)² = log8xy
⇒ 2log(x + y)= log8 + logx + logy [∵ logx^m = mlogx]
[∵ logxy = logx + logy]
= log2³ + logx + logy
⇒ 2log(x + y) = logx + logy + 3log2

Question 5.
Find the number of digits in 42013, if log₁₀2 = 0.3010.
Answer:
Given:
log₁₀2 = 0.3010
4²⁰¹³ = (2²)²⁰¹³ = 2⁴⁰²⁶
∴ log₁₀ 2⁴⁰²⁶ = 4026 log₁₀2
[∵ log x^m = m log x]
= 4026 × 0.3010 = 1211.826.
So 1211 + 1 = 1212
∴ 4²⁰¹³ has 1212 digits in its expansion.
(∵ characteristic 1211)