AP 10th Class Maths Bits Chapter 8 Similar Triangles with Answers

Practice the AP 10th Class Maths Bits with Answers Chapter 8 Similar Triangles on a regular basis so that you can attempt exams with utmost confidence.

AP State Syllabus 10th Class Maths Bits 8th Lesson Similar Triangles with Answers

Question 1.
In ΔABC, E and F are the points on the sides AB and AC respectively.
If AE = 2 cm, EB = 2.5 cm, AF = 4 cm and FC = 5 cm, then
A) EF ⊥ BC
B) EF ⊥ AB
C) EF || BC
D) EF || AB
Answer:
C) EF || BC

Question 2.
From the given figure, the value represented by ‘x’ is …………………

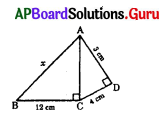
A) 12 cm
B) 11 cm
C) 13 cm
D) 16 cm
Answer:
C) 13 cm

Question 3.
From the given figure, ar (ΔADE): ar (ΔABC) =

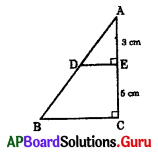
A) 25 : 9
B) 9 : 64
C) 25 : 64
D) 9 : 25
Answer:
B) 9 : 64

Question 4.
The altitude of an equilateral triangle of side ‘x’ cm is
A) \(\frac{\sqrt{3}}{2}\) x
B) \(\frac{2}{\sqrt{3}}\) x
C) \(\frac{\sqrt{3}}{4}\) x²
D) \(\frac{\sqrt{3}}{2}\) x²
Answer:
A) \(\frac{\sqrt{3}}{2}\) x

Question 5.
Areas of two similar triangles are 100 cm² and 64 cm². If the median of bigger triangle is 10 cm, then the median of the smaller triangle is …………………..
A) 10 cm
B) 6 cm
C) 4 cm
D) 8 cm
Answer:
D) 8 cm

Question 6.
If ΔPQR ~ ΔXYZ and ∠X= 30°, ∠Q=50°, then ∠Z = ………………….
A) 100°
B) ∠R
C) both A and B
D) not known
Answer:
C) both A and B

Question 7.
From the given figure, x = ……………….

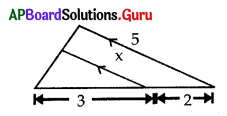
A) 3
B) 2
C) 5
D) 1
Answer:
A) 3

Question 8.
In Heron’s formula, area of triangle = \(\sqrt{\mathbf{S ( S – a ) ( S – b ) ( S – c )}}\), S is ……………… of the triangle.
A) Perimeter
B) Height
C) Half of perimeter
D) None
Answer:
C) Half of perimeter

Question 9.
In Δ ABC with ∠A = 90°; from A, perpendicular AD is drawn on BC. Which one of the following is not correct?
A) ∠A = ∠B
B) Δ DAC ~ Δ DBA
C) Δ ABC ~ Δ DBA
D) Δ ABC ~ Δ DBA ~ Δ DAC .
Answer:
A) ∠A = ∠B

Question 10.
If Δ PQR – ΔXYZ, QR = 3 cm, YZ = 4 cm, ar ΔPQR= 54 cm², then ar ΔXYZ = ………………
A) 13.5 cm²
B) 46 cm²
C) 96 cm²
D) 12 cm²
Answer:
C) 96 cm²

Question 11.
In the adjacent figure, AB = 3 cm, AC = 8 cm, BE = 4.5 cm, then CD = ………………..

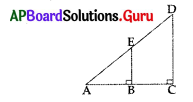
A) 10.5 cm
B) 9.5 cm
C) 16 cm
D) 12 cm
Answer:
D) 12 cm

Question 12.
If ΔABC ~ ΔDEF and area (ΔABC) : area (ΔDEF) = 49 : 100. Then DE : AB = ………………….
A) 9 : 10
B) 10 : 7
C) 10 : 9
D) 7 : 10
Answer:
B) 10 : 7

Question 13.
In ΔABC, \(\overline{\mathrm{DE}} \| \overline{\mathrm{BC}}\) and \(\frac{\mathrm{AD}}{\mathrm{DB}}=\frac{3}{5}\) ,AC = 5.6, then AE =
A) 1.8 cm
B) 3.5 cm
C) 1.2 cm
D) 2.1 cm
Answer:
D) 2.1 cm

Question 14.
ΔABC ~ ΔPQR, ∠A = 50°, then ∠Q + ∠R =
A) 120°
B) 110°
C) 130°
D) 80°
Answer:
C) 130°

Question 15.
Area of regular hexagon of side ‘a’ units is …………….. sq. units
A) \(\frac{6 \sqrt{3}}{4}\) a
B) \(\frac{6 \sqrt{3}}{7}\) a²
C) \(\frac{6 \sqrt{3}}{2}\) a²
D) \(\frac{6 \sqrt{3}}{4}\) a²
Answer:
D) \(\frac{6 \sqrt{3}}{4}\) a²

Question 16.
If ΔABC ~ ΔDEF, BC = 4 cm, EF = 5 cm area of ΔABC = 80 cm², then area of
ΔDFF = ……………. cm².
A) 64
B) 125
C) 144
D) 169
Answer:
B) 125

Question 17.
In a square, the diagonal is ……………… times of its side.
A) √7
B) √3
C) √2
D) 2
Answer:
C) √2

Question 18.
In a quadrilateral ABCD, if AB = CD, BC = AD and AC ≠ BD, then ABCD is ………………
A) parallelogram
B) trapezium
C) rhombus
D) square
Answer:
A) parallelogram

Question 19.
In ΔPQR, ∠R = 90° then hypotenuse is …………………..
A) PQ
B) PR
C) RP
D) PP
Answer:
A) PQ

Question 20.
ΔABC ~ ΔDEF if ∠E + ∠F = 130° then ∠A = …………………..
A) 60°
B) 70°
C) 80°
D) 50°
Answer:
D) 50°

Question 21.
The length of the diagonal of a square is 7√2 cm, then the area of the square is …………….cm².
A) 28
B) 14√2
C) 21
D) 49
Answer:
D) 49

Question 22.
In the given figure DE || BC and AE : EC = 4 : 7, then BC: DE = ………………

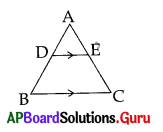
A) 11 : 4
B) 4 : 11
C) 11 : 3
D) 3 : 11
Answer:
A) 11 : 4

Question 23.
In a equilateral triangle ABC, D is a point on side BC, such that BD = \(\frac {1}{3}\) BC.
Then 9 AD² = ………………
A) 3AB²
B) 5AB²
C) 7AB²
D) 4AB²
Answer:
C) 7AB²

Question 24.
The angles of a triangle are 30°, 60° and 90°. The ratio of its sides is ……………
A) 1 : 2 : √3
B) 1 : √3 : 2
C) √3 : 1 : 2
D) 1 : 2 : 3
Answer:
B) 1 : √3 : 2

Question 25.
In a equilateral triangle ABC, if AD ⊥ BC, then …………………..
A) 5AB² = 4AD²
B) 3AB² = 4AD²
C) 4AB² = 3AD²
D) 2AB² = 3AD²
Answer:
B) 3AB² = 4AD²

Question 26.
Two isosceles triangles have equal angles and their areas are in the ratio of 16 : 25, then the ratio of their corresponding heights is …………………
A) 4 : 5
B) 5 : 4
C) 3 : 2
D) 1 : 4
Answer:
A) 4 : 5

Question 27.
In ΔABC, AD is bisector of ∠A if BD = 5 cm, BC = 7.5 cm, then AB: AC = …………..
A) 2 : 1
B) 1 : 2
C) 4 : 5
D) 3 : 5
Answer:
A) 2 : 1

Question 28.
In the quadrilateral DEFG a circle is inscribed touching quadrilateral at R, S, P, Q. If ES = 5 cm, PF = 7 cm, QG = 5 cm, DR = 4 cm; then perimeter of the quadrilateral is …………………..

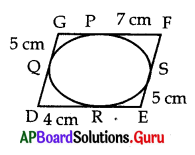
A) 40 cm
B) 21 cm
C) 42 cm
D) 20 cm
Answer:
C) 42 cm

Question 29.
If ΔABC ~ ΔEFD and ∠A = 40°, ∠F = 80° then ∠C = ………………….
A) 40°
B) 80°
C) 50°
D) 60°
Answer:
D) 60°

Question 30.
ΔABC ~ ΔPQR, AB = 3.6 cm, PQ = 24 cm and AC = 8.1 cm, PR = ……………. cm.
A) 3.6
B) 5.4
C) 7.8
D) 7.3
Answer:
B) 5.4

Question 31.
In ΔABC, D and E are midpoints of AB and AC then DE : BC
A) 4 : 1
B) 1 : 1
C) 1 : 2
D) 2 : 1
Answer:
C) 1 : 2

Question 32.
ΔABC ~ ΔDEF and perimeters of ΔABC and ΔDEF are 30 cm and 18 cm respectively. If BC = 9 cm, then EF =………………..
A) 6.3 cm
B) 5.4 cm
C) 7.2 cm
D) 4.5 cm
Answer:
B) 5.4 cm

Question 33.
According Baudhayan theorem AC² = ………………
A) AB/BC
B) AB² + BC²
C) AB + BC + AD
D) None of these
Answer:
B) AB² + BC²

Question 34.
ABC is a right triangle and right angled at C. If p is the length of perpendicular from C to AB with AB = c, BC = a, AC = b, then ………………..
A) \(\frac{1}{\mathrm{a}^{2}}=\frac{1}{\mathrm{~b}^{2}}-\frac{1}{\mathrm{p}^{2}}\)
B) \(\frac{1}{p^{2}}=\frac{1}{a^{2}}-\frac{1}{b^{2}}\)
C) \(\frac{1}{b^{2}}=\frac{1}{p^{2}}-\frac{1}{a^{2}}\)
D) \(\frac{1}{p^{2}}=\frac{1}{a^{2}}+\frac{1}{b^{2}}\)
Answer:
D) \(\frac{1}{p^{2}}=\frac{1}{a^{2}}+\frac{1}{b^{2}}\)

Question 35.
The diagonals of a trapezium ABCD with AB || DC, intersect each other at the point O. If AB = 2 CD, then the ratio of areas of triangles AOB and COD is

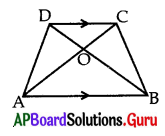
A) 4 : 1
B) 1 : 4
C) 3 : 4
D) 4 : 3
Answer:
A) 4 : 1

Question 36.
AB, CD, PQ are perpendicular to BD. If AB = x, CD = y and PQ = z, then \(\frac{1}{z}\) =

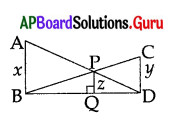
A) \(\frac{1}{x}+\frac{1}{y}\)
B) xy
C) \(\frac{1}{x}-\frac{1}{y}\)
D) 1
Answer:
A) \(\frac{1}{x}+\frac{1}{y}\)

Question 37.
In the given fighre if LM || AB, AL = x – 3, AC = 2x,BM = x – 2 and BC = 2x + 3. Then the value of x is ……………..

A) 7
B) 8
C) 9
D) Cannot be determined
Answer:
C) 9

Question 38.
The diagonals of a quadrilateral ABCD intersect each other at a point O such that \(\frac{\mathrm{AO}}{\mathrm{BO}}=\frac{\mathrm{CO}}{\mathrm{DO}}\) = . Then the quadrilateral ABCD is …………………….
A) trapezium
B) square
C) rectangle
D) parallelogram
Answer:
B) square

Question 39.
In the given figure, if AB || CD || EF given that AB = 7.5 cm, DC = y cm, EF = 4.5 cm, CF = 3 cm BC = x cm, then the value of x is ………………..

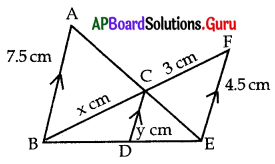
A) 4 cm
B) 5 cm
C) 6 cm
D) None
Answer:
C) 6 cm

Question 40.
If BL and CM are the medians of a triangle ABC right angled at A, then the value of 4(BL² + CM²) =
A) 3BC²
B) 5BC²
C) 7BC²
D) BC²
Answer:
B) 5BC²

Question 41.
If ABD is a triangle right angled at A and AC ⊥ BD, then AC² =
A) BC . BD
B) BD . CD
C) BC. DC
D) AD . AB
Answer:
C) BC. DC

Question 42.
In ΔABC, DE || BC and \(\frac{A D}{D B}=\frac{3}{5} .\). If AC = 5.6 cm, then AE =

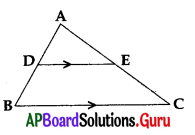
A) 2 cm
B) 2.1 cm
C) 2.2 cm
D) 2.5 cm
Answer:
B) 2.1 cm

Question 43.
In a trapezium ABCD, AB || DC. E and F are points on non-parallel sides AD and BC respectively such that EF || AB. Then \(\frac{\mathrm{AE}}{\mathrm{ED}}\) =

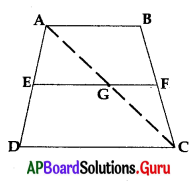
A) \(\frac{\mathrm{FC}}{\mathrm{BF}}\)
B) \(\frac{\mathrm{ED}}{\mathrm{AE}}\)
C) \(\frac{\mathrm{BF}}{\mathrm{FC}}\)
D) None
Answer:
C) \(\frac{\mathrm{BF}}{\mathrm{FC}}\)

Question 44.
Given that ΔABC ~ ΔDEF and their areas are 64 cm² and. 121 cm² respectively. If EF = 15.4 cm, then BC=
A) 2.11 cm
B) 21.1 cm
C) 1.21 cm
D) 11.2 cm
Answer:
D) 11.2 cm

Question 45.
If two triangles have the same base and area then they lies between …………….
A) parallel lines
B) two straight lines
C) perpendicular lines
D) intersecting lines
Answer:
A) parallel lines

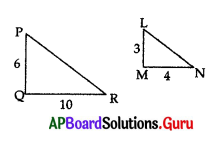
Question 46.
A flagpole 4 m tall casts a 6m shadow. At the same time, a nearby building casts a shadow of 24 m. Then the height of the building is
A) 16 m
B) 20 m
C) 24 m
D) 18 m
Answer:
A) 16 m

Question 47.
In the figure below, PQ || BC. If AP = 3, PB = 4 and AQ = 4, then QC =

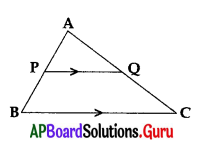
A) \(\frac{7}{3}\)
B) \(\frac{4}{3}\)
C) \(\frac{16}{3}\)
D) \(\frac{3}{4}\)
Answer:
C) \(\frac{16}{3}\)

Question 48.
ΔABC ~ ΔDEF and their areas are 16 cm² and 81 cm² respectively.
If BC = 4 cm, then EF =
A) 5 cm
B) 4 cm
C) 11 cm
D) 9 cm
Answer:
A) 5 cm

Question 49.
In the figure below, DE || BC. If AD = x, AE = x + 2, DB = x – 2 and CE = x – 1, then x =

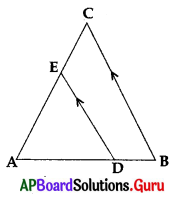
A) 4
B) 5
C) 6
D) 7
Answer:
A) 4

Question 50.
In the figure, x = ………………

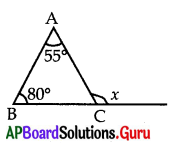
A) 45°
B) 135°
C) 90°
D) 15°
Answer:
B) 135°

Question 51.
In the figure if MN || BC, AN = 3, NC = 18, MB = 24, then AM =

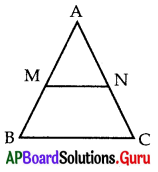
A) 2
B) 4
C) 6
D) 8
Answer:
B) 4

Question 52.
An observer of height 1.8 m is 13.2 m away from a palm tree. The angle of elevation of the top of the tree from his eyes is 45°. Then the height of the palm tree is
A) 13.2 m
B) 15 m
C) 14 m
D) 11.4 m
Answer:
C) 14 m

Question 53.
A boy goes 5m due west and then 5m due north. How far is he from the starting point?
A) 10 m
B) 10 √2 m
C) 5 m
D) 5 √2 m
Answer:
C) 5 m

Question 54.
In the figure CD = DA, and EF || BD, CF then \(\frac{\mathrm{CF}}{\mathbf{F B}}\) = ………………

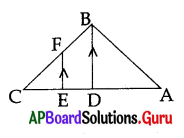
A) \(\frac{\mathrm{CD}}{\mathrm{DA}}\)
B) \(\frac{C E}{D A}\)
C) \(\frac{\mathrm{CE}}{\mathrm{ED}}\)
D) \(\frac{\mathrm{CD}}{\mathrm{CA}}\)
Answer:
C) \(\frac{\mathrm{CE}}{\mathrm{ED}}\)

Question 55.
From the diagram, LM||CB and LN || CD. Which of the following is true?

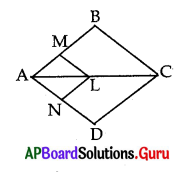
A) \(\frac{\mathrm{AM}}{\mathrm{AB}}=\frac{\mathrm{AN}}{\mathrm{AD}}\)
B) \(\frac{\mathrm{AM}}{\mathrm{MB}}=\frac{\mathrm{AN}}{\mathrm{ND}}\)
C) \(\frac{\mathrm{AL}}{\mathrm{LC}}=\frac{\mathrm{AM}}{\mathrm{AN}}\)
D) \(\frac{\mathrm{AD}}{\mathrm{BC}}=\frac{\mathrm{AD}}{\mathrm{DC}}\)
Answer:
A) \(\frac{\mathrm{AM}}{\mathrm{AB}}=\frac{\mathrm{AN}}{\mathrm{AD}}\)
or
B) \(\frac{\mathrm{AM}}{\mathrm{MB}}=\frac{\mathrm{AN}}{\mathrm{ND}}\)

Question 56.
When we construct a triangle similar to a given triangle as per given scale f actor, we construct on the basis of ……………….
A) SSS similarity
B) AAA similarity
C) Basic proportionality theorem
D) A and C are correct
Answer:
C) Basic proportionality theorem

Question 57.
In ΔABC, DE || BC, If \(\frac{\mathrm{AD}}{\mathrm{DB}}=\frac{2}{3}\) and AC = 18, then AE =
A) 36
B) 3.6
C) 72
D) 7.2
Answer:
D) 7.2

Question 58.
In the figure, DE || BC. AD = x, DB = x – 2, AE = x + 2 and CE = x – 1, then x =

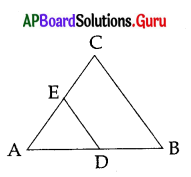
A) 1
B) 2
C) 3
D) 4
Answer:
D) 4

Question 59.
If ΔABC ~ ΔXYZ, ∠C = 60° and ∠B = 75°, then ∠Z = ……………….
A) 45°
B) 60°
C) 75°
D) 90°
Answer:
A) 45°

Question 60.
If ΔABC ~ ΔDEF, ∠A = 50°, then ∠E + ∠F = ………………
A) 100°
B) 125°
C) 130°
D) 140°
Answer:
C) 130°

Question 61.
If ΔABC ~ ΔDEF, ∠A + ∠B = 130°, then ∠F = ……………..
A) 130°
B) 140°
C) 50°
D) 40°
Answer:
C) 50°

Question 62.
ΔABC ~ ΔPQR, If AB = 3.6, PQ = 2.4, PR = 5.4, then AC =
A) 3.6
B) 8.1
C) 5.4
D) 7.8
Answer:
B) 8.1

Question 63.
In ΔABC, \(\overline{\mathbf{P Q}} \| \overline{\mathbf{A B}}\) , P, Q are on BC and AC. If CQ : QA = 1 : 3 and CP = 4, then BC =
A) 8
B) 12
C) 16
D) 4
Answer:
C) 16

Question 64.
If ΔABC ~ ΔDEF, such that 2AB = DE and BC = 8 cm, then EF = ……………. cm
A) 16
B) 12
C) 9
D) 4
Answer:
A) 16

Question 65.
ΔABC ~ ΔPQR, if AB = 6, BC = 4, AC = 8 and PR = 6, then PQ + QR = …………..
A) 8
B) 10
C) 7.5
D) 9
Answer:
C) 7.5

Question 66.
The ratio of perimeters of two similar triangles is 4 : 9, then the ratio of their areas is …………….
A) 16 : 9
B) 2 : 3
C) 16 : 81
D) 61 : 81
Answer:
C) 16 : 81

Question 67.
The perimeters of two similar triangles are 20 cm and 30 cm then the ratio of the areas of the triangles is ………………
A) 2 : 3
B) 3 : 2
C) 4 : 9
D) 9 : 4
Answer:
C) 4 : 9

Question 68.
The areas of two similar triangles are 16 cm² and 25 cm² respectively, then the ratio of their corresponding sides is ………………..
A) 5 : 4
B) 4 : 5
C) 16 : 25
D) 25 : 16
Answer:
B) 4 : 5

Question 69.
ΔABC ~ ΔDEF. If BC = 3 cm, EF = 4 cm and ar (ΔABC) = 54 cm² then ar (ΔDEF)=
A) 66 cm²
B) 79 cm²
C) 100 cm²
D) 96 cm²
Answer:
D) 96 cm²

Question 70.
The ratio of altitudes of two similar triangles is 4 : 9, then ratio of their areas is
A) 2 : 3
B) 4 : 9
C) 81 : 16
B) 4 : 9
D) 16 : 81
Answer:
D) 16 : 81

Question 71.
The areas of two similar triangles are 25 m² and 36 m². Median of smaller triangle is 10m, then median of the bigger triangle is
A) 15 m
B) 18 m
C) 16 m
D) 12 m
Answer:
D) 12 m

Question 72.
The ratio of areas of two similar triangles is 1: 2, then the ratio of their altitudes is
A) 1 : √2
B) 1 : 2
C) 2 : 1
D) √2 : 1
Answer:
A) 1 : √2

Question 73.
D, E are the mid-points of the sides AB and AC of ΔABC., then DE : BC = …………………
A) 3 : 1
B) 1 : 1
C) 2 : 1
D) 1 : 2
Answer:
D) 1 : 2

Question 74.
D, E are the mid-points of the sides AB and AC of ΔABC. Then DE : BC =
A) 8 cm
B) 6 cm
C) 4 cm
D) 12 cm
Answer:
A) 8 cm

Question 75.
In ΔABC, DE intersects AB and AC in the ratio 1 : 3, if DE = 2.4 cm, then BC = ……………..
A) 4.8 cm
B) 7.2 cm
C) 9.6 cm
D) 12.0 cm
Answer:
B) 7.2 cm

Question 76.
The ratios of corresponding medians of two similar triangles is 2 : 3, then the ratio of their perimeters is
A) 2 : 3
B) 3 : 2
C) 1 : 2
D) 2 : 1
Answer:
A) 2 : 3

Question 77.
The ratio of perimeters of two similar triangles is 9 : 16, then ratio of their altitudes is
A) 9 : 16
B) 3 : 4
C) 16 : 9
D) 4 : 3
Answer:
A) 9 : 16

Question 78.
In a ΔABC, if ∠A = 90°, and AD ⊥ BC, then AD² =
A) BD . DC
B) BC . BD
C) BD . BA
D) BA . BC
Answer:
A) BD . DC

Question 79.
Praveen goes 3 m due East and then 4 m due North. Then, his distance from the starting point is
A) 3 m
B) 4 m
C) 5 m
D) 7 m
Answer:
C) 5 m

Question 80.
In an equilateral triangle ABC, AD ⊥ BC, then 4 AD² =
A) 3 AC²
B) 3 AB²
C) 3 BC²
D) 2 BC²
Answer:
B) 3 AB²

Question 81.
In the rhombus ABCD, AB = 6 cm, then AC² + BD² =
A) 36
B) 144
C) 72
D) 90
Answer:
B) 144

Question 82.
ΔABC ~ ΔPQR, AB = 6, BC = 4, AC = 8, PR = 6, then PQ + QR =
A) 8
B) 10
C) 7.5
D) 9
Answer:
C) 7.5

Question 83.
Two figures are said to be similar if they have same
A) shape
B) size
C) size or shape
D) both size and shape
Answer:
A) shape

Question 84.
Two polygons with the same number of sides are similar if
A) corresponding angles are equal
B) corresponding sides are in proportion
C) corresponding angles are not equal
D) corresponding angles are equal and sides are in proportion
Answer:
D) corresponding angles are equal and sides are in proportion

Question 85.
Which of the following are not similar?
A) Allcircies
B) All squares
C) All quadrilaterals
D) All equilateral triangles
Answer:
C) All quadrilaterals

Question 86.
In ΔABC, DE || BC, then
A) \(\frac{\mathrm{AB}}{\mathrm{AC}}=\frac{\mathrm{AD}}{\mathrm{AE}}\)
B) \(\frac{\mathrm{AD}}{\mathrm{DB}}=\frac{\mathrm{EC}}{\mathrm{AE}}\)
C) \(\frac{\mathrm{AD}}{\mathrm{DB}}=\frac{\mathrm{AE}}{\mathrm{EC}}\)
D) \(\frac{\mathrm{AD}}{\mathrm{DE}}=\frac{\mathrm{AE}}{\mathrm{EC}}\)
Answer:
C) \(\frac{\mathrm{AD}}{\mathrm{DB}}=\frac{\mathrm{AE}}{\mathrm{EC}}\)

Question 87.
In ΔABC, l intersects AB and AC in D and E such that \(\) , then
A) DE ⊥ BC
B) DE || BC
C) DE ~ BC
D) DE ≅ BC
Answer:
B) DE || BC

Question 88.
ΔPQR is formed by joining the midpoints of the sides of ΔABC, then the ratio of the areas of ΔPQR and ΔABC is
A) 4 : 1
B) 1 : 2
C) 2 : 1
D) 1 : 4
Answer:
D) 1 : 4

Question 89.
Which of the following is not the sides of a right triangle ?
A) 3, 4, 5
B) 5, 12, 13
C) 7, 24, 25
D) 4, 5, 6
Answer:
D) 4, 5, 6

Question 90.
In ΔABC, BC² + AB² = AC², then is a right angle.
A) ∠A
B) ∠B
C) ∠C
D) ∠A and ∠B
Answer:
B) ∠B

Question 91.
The adjacent figures state ……………… criterion of similarity.

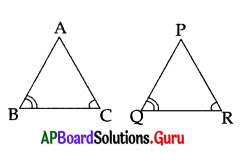
A) AA
B) AAA
C) SAS
D) SSS
Answer:
A) AA

Question 92.
Which of the following figures are similar?

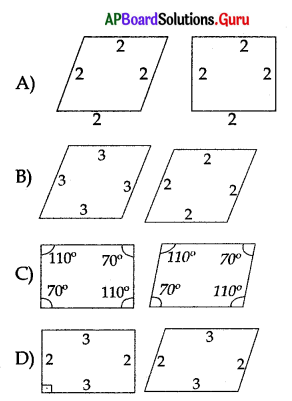
Answer:

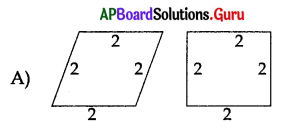
Question 93.
In a ΔXYZ, if the internal bisector of ∠X meets YZ in P. Then
A) \(\frac{X Y}{X Z}=\frac{Y P}{P Z}\)
B) \(\frac{\mathrm{XY}}{\mathrm{PZ}}=\frac{\mathrm{XZ}}{\mathrm{YP}}\)
C) \(\frac{\mathrm{XY}}{\mathrm{XZ}}=\frac{\mathrm{PZ}}{\mathrm{XP}}\)
D) \(\frac{X Z}{X Y}=\frac{Y Z}{Y P}\)
Answer:
A) \(\frac{X Y}{X Z}=\frac{Y P}{P Z}\)

Question 94.
In ΔABC and ΔA’B’C’, A’ corresponds to vertex A. We denote it symbolically as
A) A’ = A
B) A’ ~ A
C) A’ || A
D) A’ ↔ A
Answer:
D) A’ ↔ A

Question 95.
Two figures are said to be congruent if they have same
A) shape
B) size
C) size or shape
D) both size and shape
Answer:
B) size

Question 96.
Two triangles are similar if their corresponding
A) angles are equal
B) sides are in proportion
C) angles are equal (or) sides are in proportion
D) sides are not in proportion
Answer:
C) angles are equal (or) sides are in proportion

Question 97.
Symbol for “is similar to” is
A) =
B) ~
C) ≅
D) ⇒
Answer:
B) ~

Question 98.
Symbol for “is congruent to” is
A) =
B) ~
C) ≅
D) ⇒
Answer:
C) ≅

Question 99.
Basic proportionality theorem is also known as ……………… theorem.
A) Pythagoras
B) Thales
C) Similar
D) Perpendicular
Answer:
B) Thales

Question 100.
In a rhombus ABCD, AC² + BD² =
A) 4 AB²
B) 4 BC²
C) 4 CA²
D) All of these
Answer:
D) All of these

Question 101.
In a trapezium ABCD, AB || DC, and also EF || AB, then \(\frac{\mathrm{AE}}{\mathrm{ED}}\) =
A) \(\frac{\mathrm{AB}}{\mathrm{DC}}\)
B) \(\frac{\mathrm{AB}}{\mathrm{EF}}\)
C) \(\frac{\mathrm{BF}}{\mathrm{AE}}\)
D) \(\frac{\mathrm{BF}}{\mathrm{FC}}\)
Answer:
D) \(\frac{\mathrm{BF}}{\mathrm{FC}}\)

Question 102.
In ΔABC, D is the mid point of BC. Then AB² + AC² =
A) AD² + BD²
B) 2AD² + 2BD²
C) BD² + DC²
D) 2AC² + 2CD²
Answer:
B) 2AD² + 2BD²

Question 103.
In ΔABC, if ∠B = 90°, BD ⊥ AC, then
A) ΔADB ~ ΔBDC
B) ΔADB ~ ΔABC
C) ΔBDC ~ ΔABC
D) All of these
Answer:
D) All of these

Question 104.
Two ……………. are always similar
A) Triangles
B) Rectangles
C) Squares
D) Trapeziums
Answer:
C) Squares

Question 105.
Two ……………. are always similar
A) Triangles
B) Circles
C) Trapeziums
D) Rectangles
Answer:
B) Circles

Question 106.
The perimeters of two similar triangles are 30 cm and 20 cm respectively. If one side of the first triangle is 12 cm, then the corresponding side of the second triangle is …………….. cm
A) 8
B) 10
C) 12
D) 20
Answer:
A) 8

Question 107.
In a ΔABC, the bisector of ∠A intersects BC at D. If BD: DC = 4 : 7 and AC = 3.5 then AB =
A) 2
B) 3
C) 4
D)5
Answer:
A) 2

Question 108.
AD is the angular bisector of ‘A’. If AB = 6 cm, BD = 8 cm and DC = 6 cm, then AC = …………………. cm.
A) 4.5
B) 4
C) 4.8
D) 5.6
Answer:
A) 4.5

Question 109.
ΔABC is similar to ΔDEF is denoted as
A) ΔABC = ΔDEF
B) ΔABC ~ ΔDEF
C) ΔABC ≅ ΔDEF
D) ΔABC || ΔDEF
Answer:
B) ΔABC ~ ΔDEF

Question 110.
ΔABC is congruent to ΔDEF is denoted as
A) ΔABC = ΔDEF
B) ΔABC ~ ΔDEF
C) ΔABC ≅ ΔDEF
D) ΔABC || ΔDEF
Answer:
C) ΔABC ≅ ΔDEF

Question 111.
In the given figure , we observe the following relationship

A) \(\frac{\mathrm{PE}}{\mathrm{EQ}}=\frac{\mathrm{PE}}{\mathrm{FR}}\)
B) EF ⊥ QR
C) EF || QR
D) EF = QR
Answer:
C) EF || QR

Question 112.
In the given figure, DE || BC, If \(\frac{\mathbf{A D}}{\mathbf{D B}}=\frac{1}{2}\) and AE = 1.2 cm then EC = …………….. cm

A) 7.2
B) 4.7
C) 3.6
D) 2.4
Answer:
D) 2.4

Question 113.
In the adjacent figure, x = …………….., if AB || QR.

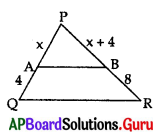
A) 4
B) 8
C) 12
D) 16
Answer:
A) 4

Question 114.
In the figure DE || BC, then x = …………………..

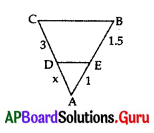
A) 2
B) 3
C) 4
D) 1.5
Answer:
A) 2

Question 115.
Observe the given figures and name the criterion of similarity.

A) AAA
B) ASA
C) SAS
D) No similarity
Answer:
D) No similarity

Question 116.
The adjacent figures state ………………… criterion of similarity.

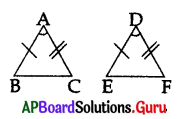
A) AAA
B) SSS A
C) SAS
D) ASA
Answer:
C) SAS

Question 117.
Write the similarity relation in symbolic form.

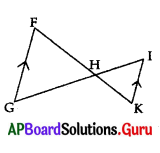
A) Δ FGH ~ Δ HIK
B) Δ FGH ~ Δ KIH
C) Δ GFH ~ Δ HKI
D) Δ FHG ~ Δ IKH
Answer:
B) Δ FGH ~ Δ KIH

Question 118.
Write the similarity relation of triangles in symbolic form.

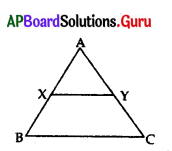
A) Δ AXY ~ Δ ABC
B) Δ XYA ~ Δ BAC
C) Δ YXA ~ Δ BCA
D) Δ XAY ~ Δ CAB
Answer:
A) Δ AXY ~ Δ ABC

Question 119.
In the given similar triangles, the value of x =

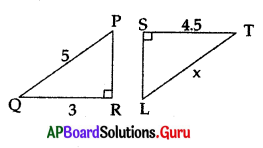
A) 4.5
B) 6.5
C) 7.5
D) 8.5
Answer:
C) 7.5

Question 120.
Find the value of y if the given triangles are similar.

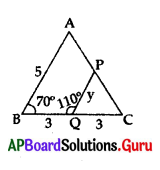
A) 1.5
B) 2.5
C) 3.5
D) 4.5
Answer:
B) 2.5

Question 121.
In the given figure, DE || BC and AD : DB = 5 : 4, then =

A) \(\frac{81}{25}\)
B) \(\frac{5}{9}\)
C) \(\frac{5}{4}\)
D) \(\frac{25}{81}\)
Answer:
D) \(\frac{25}{81}\)

Question 122.
In ΔABC, D, E are the mid-points of the sides AB and AC, then ar (ΔADE ) : ar ( BCED) =
A) 1 : 4
B) 1 : 3
C) 2 : 1
D) 3 : 2
Answer:
B) 1 : 3

Question 123.
In figure, QA ⊥ AB, PB ⊥ AB. If AO = 10 cm, BO = 6 cm and PB = 9 cm, then AQ = …………………. cm

A) 15
B) 30
C) 45
D) 70
Answer:
A) 15

Question 124.
In the given figure, if ar (ΔPOB) =32 cm², then ar(ΔQOA) is

A) 40 cm²
B) 50 cm²
C) 75 cm²
D) 150 cm²
Answer:
B) 50 cm²

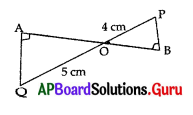
Question 125.
In figure, ΔABC ~ ΔDEF. AB = 5 cm, area (ΔABC) = 20 cm², area (ΔDEF) = 45 cm² then DE =

A) 2.0 cm
B) 4.5 cm
C) 7.5 cm
D) 9.5 cm
Answer:
C) 7.5 cm

Question 126.
In the given figure, CD is

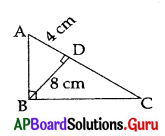
A) 16 cm
B) 64 cm
C) 4 cm
D) 36 cm
Answer:
A) 16 cm

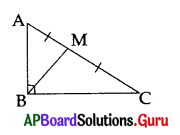
Question 127.
Diagonal of right angled triangle ABC is 8 cm, then its median BM =

A) 2 cm
B) 3 cm
C) 4 cm
D) 5 cm
Answer:
C) 4 cm

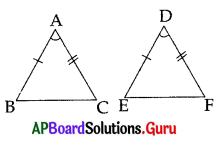
Question 128.
In the fig. ΔABC ~ ΔDEF. Name the criterion of similarity.

A) SSS
B) SAS
C) AAA
D) RHS B
Answer:
B) SAS

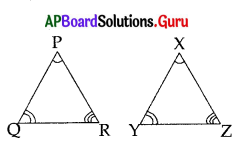
Question 129.
The adjacent figures state …………….. criterion of similarity.

A) SSS
B) SAS
C) AAA
D) AA
Answer:
C) AAA

Question 130.
In the given fig. LM || CB and LN || CD, then

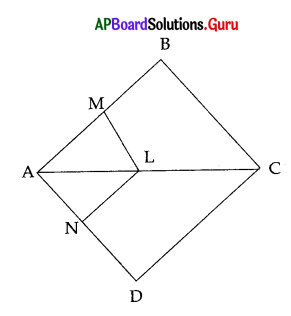
A) \(\frac{A N}{N D}=\frac{A M}{A B}\)
B) \(\frac{\mathrm{AN}}{\mathrm{AL}}=\frac{\mathrm{AM}}{\mathrm{ML}}\)
C) \(\frac{\mathrm{AM}}{\mathrm{MB}}=\frac{\mathrm{AN}}{\mathrm{ND}}\)
D) \(\frac{A M}{M B}=\frac{A N}{A D}\)
Answer:
C) \(\frac{\mathrm{AM}}{\mathrm{MB}}=\frac{\mathrm{AN}}{\mathrm{ND}}\)